Introduction
The migratory locust, Locusta migratoria (Linnaeus) (Orthoptera: Acrididae), is an agricultural pest of major importance in large areas of the Ancient world (COPR, 1982). Most of the time, the species occurs at low densities in the solitarious phase, characterized by relatively cryptic and scattered individuals. During outbreaking periods, it displays huge increases of population densities with actively aggregating and swarming individuals typical of the high-density gregarious phase (Uvarov, Reference Uvarov1966).
It has been proposed that gregarious populations achieve higher growth rates than solitarious populations because they encounter more favourable environments. For instance, in Africa and Madagascar, marked numerical population increases at the beginning of outbreaks have been associated with above-average rainfall (Davey, Reference Davey1956; Waloff, Reference Waloff1962; Dempster, Reference Dempster1963; Têtefort & Wintrebert, Reference Têtefort and Wintrebert1966; Roffey & Popov, Reference Roffey and Popov1968). By stimulating vegetation growth, such conditions result in abundant and high quality food resources for breeding adults. Moreover, higher soil humidity allows successive waves of females to oviposit and decreases egg desiccation and first-instar larval mortality. The absence of egg and larval parasites and predators has also been proposed as a factor favouring the production of locust outbreaks (Roffey & Popov, Reference Roffey and Popov1968; Wintrebert, Reference Wintrebert1970; Farrow, Reference Farrow1974). However, the potential for gregarious populations to maintain a high growth rate following outbreak initiation is often counteracted by their frequent extension into new environments that are unfavourable for sustained reproduction (Batten, Reference Batten1967).
The higher growth rate of gregarious populations may also result from intrinsic differences in survival and/or fecundity between the gregarious phase individuals produced by high density conditions and the solitarious phase individuals generated under low density conditions (Uvarov, Reference Uvarov1966). As a causal factor of fluctuations in population growth rates, a density-related shift in life-history traits could arise from genetic (Chitty, Reference Chitty1967) or non-genetic (Rossiter, Reference Rossiter1996; Ginzburg, Reference Ginzburg, Mousseau and Fox1998) parental effects. Because of the strength of selection required to produce a major change in gene frequency in just one or a few generations, the hypothesis of a genetic causation of short-term fluctuations in population growth rates has received so far little credit (Mitter & Schneider, Reference Mitter, Schneider, Barbosa and Schultz1987; but see Baltensweiler, Reference Baltensweiler1984). On the other hand, a considerable amount of theoretical work indicates that the delayed effects of parental environments are capable of profoundly altering the life-history traits of insect populations (e.g. Rossiter, Reference Rossiter, Hunter, Ohgushi and Price1992) and are likely to generate population outbreaks (e.g. Ginzburg & Taneyhill, Reference Ginzburg and Taneyhill1994; Rossiter, Reference Rossiter1994). Although parental density conditions have been shown to drive behavioural and morphological phase changes in locusts (Islam et al., Reference Islam, Roessingh, Sinervo and McCaffery1994) and in L. migratoria in particular (Chapuis et al., Reference Chapuis, Estoup, Augé-Sabatier, Foucart, Lecoq and Michalakis2008a), empirical evidence for parental effects on the density-related changes in life-history traits of their offspring is still lacking in these species (but see Albrecht et al., Reference Albrecht, Verdier and Blackith1959). To adequately test the hypothesis of parentally-mediated variation in life-history traits among gregarious and solitarious locusts, multigenerational studies controlling for population density, life-history traits and environmental quality are compulsory (Rossiter, Reference Rossiter1994; Hunter, Reference Hunter2002). Because L. migratoria is a highly mobile insect, especially in tropical regions where it displays seasonal migrations, such studies are very difficult to conduct in the field. An alternative approach consists of testing for differences in the life-history traits among the density-related phases by comparing laboratory-induced solitarious and gregarious individuals of the same locust population sample under controlled density conditions.
In the present study, we assessed the influence of solitarious and gregarious parentally-inherited phases on the development, survival and fecundity of females, as well as the quality and sex-ratio of their offspring. We compared two treatment groups of locusts with a known history of controlled isolated and crowded rearing conditions. Life-history traits may not only vary because of genetic and environmental factors acting on previous generations, but also because of the proximal environmental conditions experienced by the individuals under study. Several previous attempts to characterise differences between gregarious and solitarious locusts in life-history traits failed to disentangle the genetic/environmental effects acting on previous generations from proximal environmental effects (e.g. Norris, Reference Norris1950). Our experimental design, by rearing the focal experimental insects from the isolated and crowded treatment groups in the same environment (isolation), manages to cast off the confounding proximal density effects. Thus, any differences between treatments can only be ascribed to the rearing history. We used a population sample from Madagascar, an area characterized by frequent outbreak events and shown, in a previous study, to express parentally-inherited density-dependent behavioural and morphometric phase changes (Chapuis et al., Reference Chapuis, Estoup, Augé-Sabatier, Foucart, Lecoq and Michalakis2008a). We demonstrate that females respond to parental crowding conditions by reproducing earlier and producing larger offspring. Such delayed effects of parental crowding in reproductive traits might have important implications for the control of L. migratoria outbreaks.
Materials and methods
Insects and experimental design
Pre-reproductive adults of L. migratoria were collected in Betioky in southwestern Madagascar. These insects belong to the taxon L. m. capito, but note that the current taxonomy is far from being considered definitive by some authors (see Chapuis et al., Reference Chapuis, Lecoq, Loiseau, Sword, Piry and Estoup2008b). During the 20th century, five intense plagues of highly gregarious locusts and two incipient outbreaks controlled by insecticides have been observed in Madagascar, including the Betioky area (Randriatmanantsoa, Reference Randriatmanantsoa1998). At the moment of sampling, field densities were low (ca. 200 adults per ha; results not shown), well below the critical density for first phase change manifestations (ca. 2000 adults per ha: Lecoq, Reference Lecoq1975). All individuals collected corresponded to the solitarious morph and were sampled three years after the last recorded outbreak event (i.e. after 12 generations, assuming a generation time of four generations per year: Lecoq, Reference Lecoq1975). Populations of southwestern Madagascar might, however, experience high density conditions during the rainy season, and some gregarious populations were occasionally observed in this area, even during recession periods. To control for parental histories, individuals were reared in isolated conditions for two generations after sampling. In Schistocerca gregaria, the behavioural change acquired after short periods of crowding is rapidly lost (Roessingh & Simpson, Reference Roessingh and Simpson1994). Hence, assuming a similar time-course of behavioural phase change in L. migratoria, the insects were solitarious at the end of this reset step. We then reared individuals under isolated or crowded conditions for two subsequent generations. We measured developmental time, survival, fecundity, offspring body size and offspring sex-ratio of female individuals of the next generation (i.e. 5th generation) that had been reared in individual cages. By doing so, we were able to distinguish the effects of rearing history from those of the environment of the assay. Each generation started with 86 to 214 larvae from different egg pods (at least 14). Figure 1 presents an overall view of the experimental protocol and the resulting two groups we obtained, namely individuals with a rearing history of isolation (hereafter referred to as I) and individuals with a rearing history of crowding (C). Locusts with isolation and crowding histories developed highly contrasted behavioural and morphometrical phenotypes, indicating that the protocol used here efficiently induced density-dependent phase changes in this population (Chapuis et al., Reference Chapuis, Estoup, Augé-Sabatier, Foucart, Lecoq and Michalakis2008a).

Fig. 1. Experimental design. I, rearing history=isolation conditions; C, rearing history=crowding conditions; G1 to G5, first to fifth generation of experimental rearing. See text for more details. The numbers of larvae used to initiate each generation and treatment are shown in italicized characters.
Rearing under isolation and crowded conditions
Isolated rearing facilities were similar to those described in Hoste et al. (Reference Hoste, Luyten, Claeys, Clynen, Rahman, De Loof and Breuer2002), with slight modifications. Cages were rendered opaque to visually isolate insects from each other and were ventilated through a holed top. We also homogenized light conditions within each drawer by adding ‘cool’ tube lamps every two shelves. Crowd-reared locusts were kept in a separate room in 19×19×24 cm cages at a density of about 40 individuals per cage. Different cages (at least three) were used for each population at each generation of crowded rearing (i.e. 3rd and 4th generation; fig. 1). Cages were ventilated and lit in a similar way to that of the individual cages. Isolated and crowded locusts were reared under completely identical room and feeding regimes. Rooms were maintained under a 14h:10 h light:dark cycle, a fluctuating 14–10 h temperature regime of 32–28°C, a constant humidity of 50% and one complete air renewal every 3 min to minimize olfactory contact among cages. Insects were fed every two days with seedling wheat, supplemented by wheat bran for adults.
Chitin and resilin depositions in the exoskeleton following imaginal moult and until sexual maturity (Song, Reference Song2004) result in a thicker thorax in mature locusts (Loher, Reference Loher1961; Neville, Reference Neville1963a,Reference Nevilleb). We, hence, determined sexual maturity as the time at which thoracic cuticle had become very hard by manual assessment, and subsequently placed isolated males and females together for 48 h to ensure insemination. Under our rearing conditions (see details above), sexual maturity occurred nine days after emergence for the two groups of Malagasy migratory locust (means±standard errors of 8.94±0.83 and 8.77±0.57 for C and I, respectively). This is within the range reported by other studies, which, using various rearing conditions and culture strains, have reported that adults of L. migratoria reach sexual maturity within one to two weeks after emergence (e.g. seven days: Byers, Reference Byers1991; ten days: Lay et al., Reference Lay, Zissler and Hartmann1999; 14 days: Spieß & Rose, Reference Spieß and Rose2004). A single mating was performed between isolated adults originating from different parents by using their reference numbers. For both isolated and crowded conditions, egg pods were obtained in 100×50 mm diameter plastic tubes filled with moist sand (ten parts sand, one part water). Males used for mating were reared under similar histories to those of measured females.
Survival analysis
Females of the 5th generation with isolation (I) or crowding (C) history were checked during the entire study (122 days). At each check, each female was given a state based on morphological or reproductive characteristics: larva (denoted LAR) before the day of adult moulting, non-reproductive adult (NRA) between the day of adult moulting and the day of first oviposition, and first-time reproductive adult (FRA) after the first oviposition event. Thus, it was possible to reconstitute the life history of each individual. This kind of data is best analysed with Capture-Mark-Recapture (CMR) methods, which are able to estimate different life-history parameters, e.g. survival and maturation (see Lebreton et al., Reference Lebreton, Burnham, Clobert and Anderson1992). Another significant advantage of CMR methods over other methods is the ability of incorporating non-complete (censored) data points: 27 out of the 190 larvae which initiated the survival experiment were found to have escaped, were removed from the experiment or were still alive at the end of the study. Schematically, CMR methods allow selecting the model that best fits the data among a set of candidates, and then using it to infer demographic parameters, such as survival rates. Model selection is based on the Akaike's information criterion corrected for small sample sizes (hereafter AICc: Burnham & Anderson, Reference Burnham and Anderson1998). The AICc is directly related to the deviance (DEV, equals to minus twice the log likelihood of the model, thus providing a relative measure of the fit of the model) and the number of parameters (np) included in the model where AIC=DEV+2np. The model with the lowest AICc is, thus, the best compromise between the lack of precision of the estimates (too many parameters in the model) and bias (too few parameters in the model) among all candidate models. According to Burnham & Anderson (Reference Burnham and Anderson1998), candidate models with an AICc farther than ten from the best model's score should be considered as essentially not supported by the data.
We used a three-states model (LAR, NRA and FRA) to analyse this dataset (see Nichols et al., Reference Nichols, Sauer, Pollock and Hestbeck1992). Twelve parameters were necessary: three probabilities of survival (one per state, denoted Φ), three probabilities of recapture (denoted P and here set to one as all capture histories of the few escaped or removed individuals were actually right-censored) and six probabilities of transition between the three states (denoted Ψ). With regards to transitions, the transition from LAR to FRA and all reverse transitions, i.e. from FRA to NRA, from NRA to LAR and from FRA to LAR, were set to zero. Because the experiment was run under constant controlled conditions, we did not expect time-variation in the probabilities of transition and survival. Besides, because there were few observations as FRA (see Results section), we set the FRA survival and the transition from NRA to FRA constant. However, we expected an age-dependence for the transition probability LAR to NRA since young larvae have a lower probability to become NRA than old larvae. Before moulting to adults, larvae go through five different stages that were not recorded throughout the experiment (Uvarov, Reference Uvarov1966). Because these different larval stages may have different survival rates, we tested for an age effect in the survival of larvae. We, thus, considered age to be a categorical variable with the last age class pooling several late age classes due to small sample sizes. Consequently, each age class, except the last one, thus, covered the time interval between two check occasions. Because hatching was not synchronised and time intervals were not equal between groups I and C (see below), the age classes are not directly comparable between the two groups. Overall, in the set of candidate models, we analysed eight models differing in whether parameters were age dependent or not. We also ran a random effect model on age-dependent survival parameters of larvae to estimate a mean and its variance over all ages from the best model among the previous eight models (Burnham & White, Reference Burnham and White2002). This random effect model also provided a straightforward way for testing for differences in survival of larvae between the two groups by performing a Z test on these estimates. The program MARK was used for model fitting (White & Burnham, Reference White and Burnham1999).
Assessing the fit of such complex models is not an easy task as there is no specific goodness of fit test available for multi-state models with fixed parameters (Pradel et al., Reference Pradel, Wintrebert and Gimenez2003). However, given that the candidate models included only age differences in parameters (see below), we pooled data over cohorts and resorted to a parametric bootstrap approach to assess the goodness of fit of the best models (R. Pradel, personal communication). We simulated 3000 samples using multinomial laws parameterised with the estimates from the best models and using the numbers of individuals ‘released’ in each state at a given age (RANDMULTINOMIAL function, SAS for Windows 9.1. SAS institute, Cary, NC, USA). A chi-square statistic was calculated for each sample. As a goodness of fit test, we assessed the number of samples for which the value of chi-square was superior from the one calculated from original data.
Due to logistic constraints, time schedules were different between the groups I and C: survival of group I was checked in 21 occasions while that of group C was checked in 28 occasions. Further, the time intervals between consecutive checks varied from two to nine days. Because of these differences, the analysis of survival was carried out separately for groups I and C. However, for the sake of comparison between groups, CMR methods allow standardising all survival estimates to the same time interval – here we estimated daily rates. All estimates of life-history parameters are displayed as mean±standard error in the text.
Development and reproduction analysis
We compared developmental time of female larvae (measured by the adult moulting day minus the hatching day), body size (hind femur length of around 40-day-old post-hatching female adults, i.e. after the cuticle had hardened) and reproduction of females of the 5th generation with isolation (I) and crowding (C) histories. All reproductive parameters were measured for the first egg pod only, as in natural solitarious populations of L. migratoria in southwestern Madagascar, most females lay only one pod (average number of oviposition events per female=1.35: Lecoq, Reference Lecoq1975). The timing of reproduction was assessed by monitoring the time necessary for the females to lay their first egg pod once mated (i.e. first oviposition day minus mating day) and the time necessary for the eggs to hatch (i.e. hatching day minus oviposition day). Moreover, we counted the number of laid eggs and estimated hatching rate (i.e. number of hatched eggs divided by number of laid eggs). Finally, we measured hind femur lengths of freshly hatched female and male offspring (<24 h) following Dirsh (Reference Dirsh1953), by using scanning and image analysis tools (UTSCHA, Image Tool version 3.0, developed by the University of Texas Health Science Center, San Antonio, TX, USA). Offspring sex-ratio was assessed by examining external genitalia of freshly hatched larvae (<24 h) following Dirsh (Reference Dirsh1950).
Check intervals for adult moulting and oviposition days varied in time and with treatment (two to nine days; see Survival analysis for further details). We, hence, checked that distributions of time intervals between the observation of the oviposition event and the previous check were similar between the two groups I and C (Wilcoxon two samples test; W=80 and P=0.787). In contrast, the time intervals between the observation of the adult moulting and the previous check, hereafter referred as ‘check interval’, were significantly different between the two treatments (Wilcoxon two samples test; W=1673 and P=0.0003). To account for this methodological bias, we took this interval explicitly into account and analysed developmental times of female larvae using a full factorial ANCOVA with density history as a fixed factor and check interval as a covariate. Times necessary for the eggs to hatch, adult hind femur lengths and egg numbers per pod were analysed using ANOVAs with density history as a fixed factor. Because mating day was controlled by the experimenter, who placed together isolated females and males once they reached sexual maturity (see the Rearing paragraph in the Materials and methods section), we analysed times necessary for the females to lay their first egg pod using a full factorial ANCOVA with density history as a fixed factor and mating day minus sexual maturity day as a covariate (hereafter referred as ‘mating day’). For the former ANOVA and ANCOVA analyses, time data were logarithmically-transformed and adult hind femur lengths were square-transformed to satisfy analysis of variance requirements, and the JMP package was used (SAS Institute, 1995, Carry, NC, USA). Because of unbalanced data, we analysed offspring hind femur lengths using a mixed model with density history and sex as fixed factors and mother as a random factor, using the SAS system (Littell et al., Reference Littell, Milliken, Stroup and Wolfinger1996). For proportions (hatching rate and sex-ratio), analyses of deviance were performed assuming a logit link function and a binomial error (Crawley, Reference Crawley1993) and using the GLIM package (Baker & Nelder, Reference Baker and Nelder1985). Overdispersion was accounted for following Crawley (Reference Crawley1993; pp. 223–225). A Fisher's combined probability test was used to assess the combined significance across all eight measures (see Manly, Reference Manly1985, appendix A.15).
Results
Survival analysis
We initiated the generation of measurements (i.e. 5th generation) for isolation (I) and crowding (C) rearing histories with 212 and 198 first-instar larvae, respectively, from which 104 and 86 were females (fig. 1). There were 1344 observation events of these females recorded in the three different states, 552 and 792 for groups I and C, respectively (see Appendix 1 for a summary of the number of observations in each state and the observed transitions between states in the dataset).
Model selection on survival data ranked the eight models similarly for both I (isolation rearing history) and C (crowding rearing history) groups (see Appendix 2 for a summary of the model selection for groups I and C). Furthermore, the best model was, respectively, for group I and C, 14 and 18 units of AICc from the next best model, implying that all other candidate models were essentially not supported by the data. The best model had age-dependence for survival of larvae and transition from larva to non-reproductive adult. However, survival of non-reproductive adults was constant in the best model. Given the large number of observations of this class, the absence of age-dependence could not be ascribed to lack of data. In both groups, best models (model 8) fitted the data adequately as evaluated by the bootstrap approach (I: P=0.10; C: P=0.41).
For both I and C groups, survival of non-reproductive adults and larvae were largely superior to the survival of reproductive adults (table 1; Besnard et al. Reference Besnard, Piry, Berthier, Lebreton and Streiff2007). Also, as expected, the probability of transition from larva to non-reproductive adult overall increased with age for both groups. Differences in survival of larvae and non-reproductive adults between the groups I and C were minute (table 1; results of the random effects model for larvae I: 0.976±0.009 and C: 0.979±0.008). Larger, though still not significant, differences between the groups I and C were found in survival for reproductive adults (Z=−0.80 and P=0.42; table 1) and in the probability of transition from non-reproductive to reproductive adults (Z=0.74 and P=0.46; table 1). To sum up, our experiment fails to demonstrate any difference in these demographic parameters between groups I and C.
Table 1. Estimates and standard errors of survival (Φ) and transition (Ψ) probabilities of females individuals under the best three-state model (i.e. model 8; see table 1).

The three states are: LAR, larvae; NRA, non-reproductive adult; and FRA, first-time reproductive adult. Survival estimates are standardized to 24 h. The estimates of each age class are presented for the age-dependent parameters (survival of larvae and the transition from larva to non-reproductive adult). Program MARK does not provide estimates of standard error for estimates close to the boundaries of the parameter space (0 or 1). I: isolation rearing history; C: crowding rearing history.
Development and reproduction analysis
Approximately 50% of the 104 and 86 initial female larvae, for groups I and C, respectively, reached the adult stage. Among them, only around 50% survived 40 days, i.e. the age needed for measuring the hind femur length, and 40% reproduced. Some of the reproductive females provided egg-pods in which more than 90% of eggs died, probably because their eggs were not successfully fertilized or for some other uncontrolled reason. Both the low percentage of reproductive females and the presence of non-viable egg-pods led to a small number of observations for egg-related traits (e.g. a total of 14 monitored egg-pods for the traits egg number, hatching rate, offspring sex-ratio and offspring hind femur length; see table 2 for a summary of the number of observations for each trait for groups I and C). The results on these traits must, therefore, be interpreted cautiously.
Table 2. Summary of the density history effect on development and reproductive traits in L. migratoria females.

Means and standard errors were computed using raw (i.e. not transformed) data. F-tests are given for the density history effect only. P-values<0.05 are shown in bold. n, number of observations; I, isolation rearing history; C, crowding rearing history.
F-tests revealed that density history significantly affected the time between mating and first oviposition and the offspring hind femur length (F 1,12=6.08 and P=0.030; F 1,16=5.58 and P=0.031, respectively). Females with a crowding rearing history had the best parameters, with a half-fold decrease in the time to lay the first egg-pod once mated and offspring that emerged with larger hind femurs (table 2). We did not detect effects of the density rearing history on the developmental time, survival, fecundity, and the sex-ratio and the number of offspring (table 2). A Fisher's combined probability test on the eight P-values of growth and reproduction parameters indicates an overall significant difference between I and C groups (χ216=26.87, P=0.043). For offspring hind femur length, comparisons between the two genders did not reveal a significant difference (F 1,16=3.94 and P=0.065; table 2). In contrast, there was a significant among-mother variance component (Wald test: Z=2.82 and P=0.002). We did not detect effects of the experimenter on the developmental time of female larvae (F 1,94=3.59 and P=0.061; F 1,94=0.09 and P=0.768 for check interval and the interaction check interval×rearing history, respectively) and on the times for females to lay the first egg-pod once mated either (F 1,12=3.77 and P=0.076; F 1,12=4.62 and P=0.053 for mating day and the interaction mating day×rearing history, respectively).
All these results indicate that a rearing history of crowding led to reduced female laying times and increased offspring sizes compared to females with isolation history. On the other hand, different rearing histories did not affect significantly developmental time and survival. This also holds for fecundity and the hatching rate, sex-ratio and number of offspring. Our sample size and estimation precision is limited on these latter traits, so these results must be interpreted very cautiously.
Discussion
In this study, we found that females with a crowding history, originating from a historically frequently outbreaking Malagasy population of L. migratoria, reproduced earlier (by reducing oviposition times) and increased offspring quality (by increasing offspring size) than females with an isolation history. Measured females of solitarious and gregarious phases were raised in a common environment (isolation conditions), indicating that trans-generational effects induced by density must be responsible for the observed reproductive difference. Our result parallels that of Lauga & Hatté (Reference Lauga and Hatté1978), in which L. migratoria isolated females hatching in sand used many times for oviposition by crowded females laid heavier and more eggs within their reproductive lifetime than isolated females hatching in sterilized sand. It is also compatible with the increase of the mean weight of hatched offspring produced by parents which had experienced crowding conditions either during their entire lifetime or during their adult lifetime only (Hunter-Jones, Reference Hunter-Jones1958). This latter study showed, however, that the mean weight of hatched offspring did not vary when parents had experienced crowding during their nymph lifetime or during the seven-day interval after their emergence only. Moreover, our result is, at least partly, consistent with the findings of Albrecht et al. (Reference Albrecht, Verdier and Blackith1959). The latter authors reared a crowded stock of L. migratoria individuals in isolation conditions for successive generations and reported that egg weight decreased though female potential fecundity (ovariole number) increased over successive generations. Such delayed effects, whereby gregarious females shorten reproductive timing and increase offspring quality, are likely to increase gregarious population growth rates. They, thus, may be of particular importance in the production and/or duration of outbreaks of this species.
It is worth noting that most previous studies documenting a phase variation in life-history of locusts have shown that solitarious females reared in isolation perform better than gregarious females reared under crowded conditions (e.g. Norris, Reference Norris1950; reviewed in Pener, Reference Pener1991). Because density conditions are predicted to profoundly affect life history traits through competition (e.g. for food or reproductive sites) and stress (e.g. through contact or toxic wastes), previous results on locust life history may rather reflect a constraint (i.e. stressed gregarious females are prevented from achieving their reproductive potential whereas non-stressed solitarious females are not) than an adaptation (i.e. gregarious females can achieve their reproductive potential, that is lower than that of solitarious females). Hence, previous results do not preclude that gregarious females would fare better than solitarious ones under homogeneous environmental conditions. Our present results indicate that they do so under isolation conditions, at least for a historically frequently outbreaking Malagasy population of L. migratoria.
The evolutionary factors underlying phase-level variation in life-history strategies of L. migratoria have still to be identified. Life-history evolutionary theory predicts that increases of early mortality select for increased investments to early reproduction (reviewed in Reznick, Reference Reznick, Bryant and Bashey2002). Selection favouring plasticity in offspring quality, whereby a mother that experiences a resource-limited environment allocates more resources to her offspring, has been evidenced in natural insect populations. For instance, females of seed beetles lay larger eggs in response to increasing adult density, an indicator that their offspring will encounter severe competition for food (reviewed in Fox & Mousseau, Reference Fox, Mousseau, Mousseau and Fox1998). Because gregarious populations attain huge densities and invade new areas, their resource availability is expected to be lower and more unpredictable than that of solitarious populations, with a subsequent negative impact on their survival probability (Batten, Reference Batten1967). It is, hence, possible that the observed phase-level variations in life-history strategies in L. migratoria have evolved through phase-level variations in age-specific mortality.
Our results, however, should be evaluated with caution. We did not detect significant differences in female survival with phase state. We found that producing larger offspring did not come at the cost of producing fewer offspring either, though such trade-offs are commonly observed in natural populations (Smith & Fretwell, Reference Smith and Fretwell1974). Several possibilities might explain the apparent absence of trade-offs. First, our experimental procedure might be conservative to reveal phase-related effects. Measured individuals from both I and C groups were reared in isolation conditions, which can only decrease the differences between the groups due to non-parental effects. Extending the crowded treatment period beyond two generations might have made the locusts more resistant to losing gregariousness in isolation conditions of measurements. Second, the absence of trade-offs may be partly ascribed to limited data for egg-related traits (see Results section). A likely reason for the small number of oviposition events in our study might be that we used insects sampled from a natural population for the purpose of the present experiment only, whereas most laboratory locust studies have been conducted on (often old) laboratory strains. It is, thus, possible that the other studies used animals well adapted and selected under laboratory conditions, while in our case such adaptation had not yet occurred. Third, the significance of parental effects may also depend on the environment the offspring encounter (Bernardo, Reference Bernardo1996; Plaistow et al., Reference Plaistow, Lapsley and Benton2006). In particular, trade-offs are less detectable under benign conditions (Zera & Harshman, Reference Zera and Harshman2001). Our experiment was conducted under benign isolation conditions, since animals were not in competition for food or egg-laying space and were not stressed by contact or toxic wastes of conspecifics. To thoroughly test and measure the parental effects on life-history traits of offspring reared under crowding conditions, the statistical analysis of a large number of cages would be required because individuals grown in the same cage are not independent. Finally, laboratory conditions precluded the effects of stressful natural factors, such as predators or parasitism, which may differentially affect survival of individuals of the two phases. Further experimental work, hence, is needed to detect other potential phase-related life history differences in L. migratoria and confirm the observed trans-generational elevation of population quality with high-density conditions. However, our results do unambiguously show that Malagasy locust females respond to parental crowding conditions at least by reproducing earlier and producing larger offspring. This finding incites for further research elucidating the respective roles of proximate vs. parental environments in altering growth rates of solitarious and gregarious populations and promoting locust outbreaks.
Acknowledgements
MPC was supported by a CNRS BDI grant from Nov. 2002 to Nov. 2005 and has been supported by a Marie-Curie OIF within the Sixth ECFP since Feb. 2007. This work was partly funded by INRA (SPE department) and CIRAD. YM acknowledges support from CNRS and IRD. We benefited from constructive comments on an earlier version of the manuscript from N. Charbonnel (CBGP, France) and G. Sword (University of Sydney, Australia).
Appendices
Appendix 1. Summary of the numbers of observations in each state and the observed transitions between states in the survival data (cumulative over several intervals).
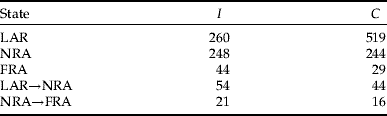
This table gives the number of observations in states LAR (larvae), NRA (non-reproductive adult) and FRA (first-time reproductive adult), as well as the number of transitions between LAR and NRA and, NRA and FRA. I, isolation rearing history; C, crowding rearing history.
Appendix 2. Summary of the model selection for survival data of groups with (a) isolation and (b) crowding rearing histories.
(a)
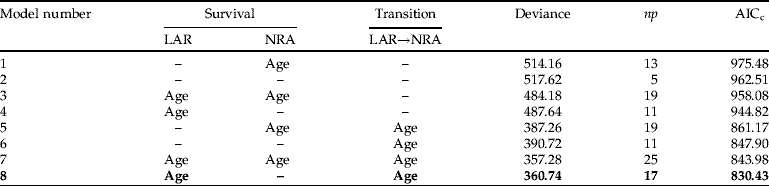
(b)

For all models, the transition NRA→FRA and the survival of state FRA were set constant. The fit of the models was assessed by Akaike's information criterion (AICc), where a lower value shows better fit. Models are ranked by AICc with best model shown in bold. Notations follow Lebreton et al. (Reference Lebreton, Burnham, Clobert and Anderson1992). np gives the number of identifiable parameters in the model under consideration. LAR, larvae; NRA, non-reproductive adult; FRA, first-time reproductive adult; –, constant (intercept only model).







