A much discussed paradox of the 2000 Presidential election is the fact that
despite winning the popular vote, Al Gore lost the election to George W.
Bush. This is, of course, due to the fact that the president is not elected
by a plurality of popular votes cast but rather by a plurality of votes of
the 538-member Electoral College (EC). Each state, except for Maine and
Nebraska, elects its members of the EC by a winner-take-all method, i.e.,
the winner of the plurality vote in a state is entitled to all the electors
from that state.1
Maine and Nebraska give an
elector to the winner of the plurality of votes in each
congressional district and in addition give two electors to the
winner of the plurality of the statewide vote. It is therefore
possible that the five Nebraska electors and Maine's four electors
are split between several candidates.
The only other
winning candidate without a plurality of the popular vote was in the
Harrison v. Cleveland election of 1888. While the 1888 election still
produced a difference of 65 votes in the EC in favor of Harrison, the 2000
election was also very close in the EC. Only the 1876 Hayes v. Tilden
election was closer in EC votes.
The size of each state's delegation to the EC equals the size of the state's
delegation in the House of Representatives plus two for its senators. The
reasons for this method of apportionment of the EC members are rooted in the
Connecticut Compromise of 1787 (Kelly, Harbinson, and
Belz 1970; Koppell 2000). Furthermore,
the 23rd Amendment to the U.S. Constitution, ratified in 1961, gives the
District of Columbia the same number of members in the EC as the smallest
state. Currently, this number is 3, the number of EC members for several
states. Since the size of each state's EC delegation is equal to the size of
its delegation in the House plus two, smaller states have a relatively
larger representation in the EC than they would if EC members were
apportioned based on the population size alone. For example, in the 2000
election the 22 smallest states had a total of 98 votes in the EC while
their combined population was roughly equal to that of the state of
California, which had only 54 votes in the EC. Of those 98 EC votes, 37 went
for Gore while 61 went for Bush. These inequities are well known (Dahl 2002); we will not discuss them further
here.
In 1941, the size of the House was fixed at 435 representatives and has not
been changed since.2
During the 87th Congress
1961–1963, after Hawaii and Alaska had joined the Union, the House
size was 437 to give the two new states representation. However, the
apportionment following the 1960 census was based again on a House
size of 435.
Though the size of the House has not increased
during the last 60 years, the population increased from about 131 million
people in 1940 to about 290 million people in 2000. In 1941, there was
approximately one representative for every 301,000 citizens. In 1990, there
was approximately one representative for every 572,000 citizens. If we
wanted the same ratio of representatives to people today as existed in 1941
then the House, based on the 1990 census, should have had about 830 members
while based on the 2000 census the current House should have about 940
members. Since our interest is in the 2000 presidential election we use the
1990 census figures which were used for the apportionment of electors in the
2000 election. We apportioned a hypothetical House with 830 members and,
using the official results of the 2000 presidential election from all 50
states and the District of Columbia, added up the electoral votes for Bush
and Gore under this scenario. The result of this thought experiment is that
Gore has 471 of the 934 EC votes versus 463 votes for Bush. Hence Gore would
have been the winner of the election if the House size had been 830 in 2000.
It is this surprising result that inspired this article.
We then carried out the apportionment of the House for all House sizes
between 50 and 1000. As our example for the House size 830 indicates, we
expected Gore to win for large House sizes and Bush to win for small House
sizes as he did with the House size at 435. The actual results are more
surprising. As the House size ranges from 50 to 1000, the 2000 election
would have produced ties for the following 25 House sizes: 491, 493, 505,
507, 533, 535, 537, 539, 541, 543, 545, 547, 551, 555, 557, 559, 561, 571,
573, 585, 587, 591, 593, 597, and 655. For all House sizes larger than 597,
save for 655, which results in a tie, Gore would have won the election. For
all House sizes smaller than 491 Bush would have won the election. The
would-be winner for each House size is listed in Table
1.
Perhaps the most troubling aspect of the information in Table 1 is the fact that for House sizes between 492 and 596 the
winner goes back and forth many times without much rhyme or reason. For
those 105 different House sizes, the election ends in a tie 23 times, Gore
wins 29 times, and Bush wins 53 times.
To put it as a punch line: The winner of the 2000 presidential election was
de-termined in 1941 when the House size was fixed at 435. Had the House size
been set at 500 in 1941 (and not been changed since) then Gore would have
won the 2000 election!
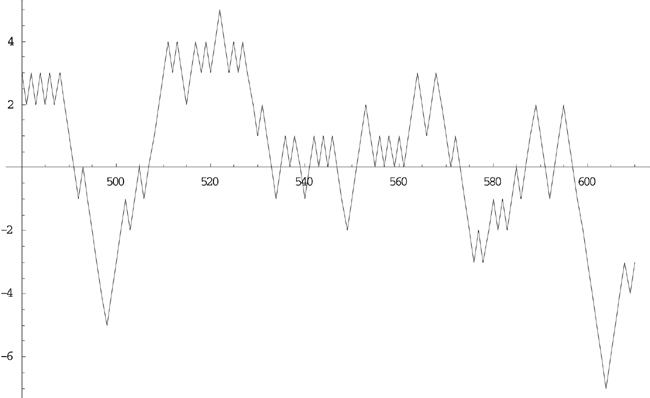
As an illustration, we describe the reasons for the different outcomes for
the House sizes 490, 491, and 492. With a House size of 490, Bush wins the
election by one vote, a House size of 491 results in a tie while a House
size of 492 results in Gore winning the election by one vote. Increasing the
House size from 490 to 491 causes the state of New York to gain an extra
seat while all other states have the same apportionment as they did with a
House size of 490. Since Gore won New York, the election now results in a
tie. When we increase the House size from 491 to 492 the extra seat goes to
Pennsylvania. Since Gore won that state he now wins the election by one
vote. Graph 1 plots House sizes between 50 and 1000 on the horizontal axis
and the corresponding difference in electoral votes on the vertical axis.
Positive differences mean that Bush wins and negative differences mean that
Gore wins.
Though the global picture clearly shows the general tendency for the
difference to decrease this is not necessarily obvious when we look at
smaller intervals. Graph 2 extracts the graph for the House sizes between
480 and 610 for a more in-depth look. Notice the steep increase in the
difference from a House size of 498 and a House size of 511. Over this
interval of House sizes the difference in electoral votes increases from −5
to +4. Over this interval states that gain seats are states Bush won in 2000
with the exception of New York and California.
The number of electoral votes for Bush minus the electoral votes for Gore
changes by one each time the house size increases by one seat.3
While this seems obvious it is not true for all
apportionment methods but is true for the current apportionment
method.
Whether the change is in favor of Bush or in favor
of Gore depends on which state gains the extra seat. As the House size
increases from 491 to 597, the winner changes repeatedly depending on which
order the states gain the additional seats. This order depends intricately
on population sizes and cannot be discerned
a priori. The following
observations explain the behavior for small House sizes and large House
sizes. For large House sizes the relative representation of the states in
the EC becomes closer to their relative representation in the House. Since
Gore won the popular vote, it is hence not surprising that he would have won
the election for large House sizes. Our earlier example shows that for small
House sizes smaller states have a relatively larger percentage of the
members in the EC. This is the reason Bush would have won the election with
small House sizes. The interval between 491 and 597 falls between the two
extremes, which is reflected in the repeated change of the winner from Bush
to Gore and vice versa. Bush's electoral strategy of winning many small
states, without winning the big states of California and New York, worked!
However, it only worked because the House size was small enough. The
non-monotonicity of the difference in the electoral votes for the two
candidates with respect to the House size is not unlike the Alabama paradox,
which led to Hamilton's method being abandoned in 1901 as the method of
apportionment in the U.S. With Hamilton's method of apportionment it may
happen, as it did with Alabama after the 1880 census, that the House size
increases yet one or more states lose a seat (see
Tannenbaum and Arnold 2001, for examples). This, in turn, may
cause the number of electoral votes for a candidate to drop even when the
size of the House is increased by one. With the current apportionment
method, this rather undesirable behavior cannot happen. Nevertheless, the
difference in the number of votes for two candidates shows the same
non-monotonic behavior with respect to the House size that we see with the
Alabama paradox in Hamilton's method.
These observations show that our present method not only goes against the
popular vote in the recent election but this instability further highlights
the fact that the election of our president by a supposedly fair and
trustworthy method depends capriciously on the choice of the House size. We
believe that this is a major flaw of the current method of electing a
president. We offer this fact as an argument that can be used against the
use of the EC in electing the President. Of course, abolishing the current
election method requires a constitutional change and as such would require
the approval of Congress and ratification by 37 states. Most states are
small and benefit from having their proportional share in representation
augmented by those two electoral votes corresponding to Senate seats; it
would seem unlikely that these states would give away this power. More
importantly, perhaps, it would undo a good part of the work of the framers
of the Constitution. Without the Connecticut Compromise there might not even
be a United States and thus no basis for this discussion.
However, this does not prevent us here from playing with a few what-if
scenarios to see how the 2000 election would have turned out under different
circumstances. The first two scenarios would require constitutional changes
and are therefore unlikely to be of any practical significance. The third
scenario does not require a constitutional change.
Electing the president by a plurality of the votes eliminates the EC and its
idiosyncrasies altogether. One drawback to a direct vote is the “Nader
factor”; a 3rd party candidate that draws votes disproportionately away from
one candidate over the other, thereby influencing the election. Undoubtedly,
the “Nader factor” played a role in the 2000 election in Florida but it was
not strong enough for Gore to lose the popular vote in the whole country.
The perils of voting theory are probably better known through Arrow's
Theorem (Arrow 1951) and through the more recent
work of Saari (Saari 1994 and 2001). A direct election of the president does offer the
advantage that it is independent of the House size.
Another possibility is a modified EC with the size of each state's delegation
equal to the number of its representatives. Under this scenario, Gore would
have been elected by the EC with a vote total of 225 to 211 for Bush. In
other words, Gore wins 51.6% of electors; somewhat more than the 48.4% of
the popular vote he won. This method is also dependent on the House size and
the described problems with the current EC in the 2000 election already
occur for smaller House sizes.
The final alternative we discuss here does not require a constitutional
change. All it would require is for all states to change the way they
allocate their electoral votes. The U.S. Constitution gives the states broad
powers as to the method of choosing their electors. Currently Maine and
Nebraska give an elector to the winner of the plurality of votes in each
congressional district and give an additional two electors to the winner of
the plurality of the statewide vote. Under this scenario, in the 2000
presidential election Bush would have won 19 of California's 54 electors
since he won the plurality of votes in 19 of California's congressional
districts. Gore would have won the remaining 35 electors; 33 of those as a
result of winning the plurality of votes in congressional districts and two
of those for winning the plurality of votes in the state. Under the current
system, Gore won all 54 electors. In all 50 states plus the District of
Columbia, Bush would have won 289 of the electoral votes to Gore's 249.
Table 2 lists the results of the presidential
election under this scenario for all states. Minnesota's 10 electors are
split equally between the two candidates; Bush winning in five congressional
districts and Gore winning three congressional districts and the plurality
of votes in the state. In all other states, the winner of the statewide
plurality vote is the candidate with more electoral votes. Note that Gore
would also win the three electors for D.C.
Districts with Republican representatives tended to vote Republican in the
presidential election, while districts with Democratic representatives
tended to vote Democratic with the exception being mostly southern districts
held by conservative Democrats that voted Republican. At first sight, this
method promises to allocate electors to the candidates at numbers closer to
the actual percentage of votes a candidate receives in a given state. This
is in fact true for some of the bigger states. In Illinois, Bush won 43% of
the vote and would have won 41% of its electors under this method while Gore
won 53% of the votes and would have won 56% of its electors. These numbers
compare favorably to the 0% of the electors for Bush and 100% of the
electors for Gore allocated in the actual election. However, while this
method allows a big state to achieve a more equitable allocation of its
electors it hardly changes the allocation of electors in a small state. In
the 10 smallest states, only one elector would have been allocated
differently. Since more of the small states are solidly Republican this
method would present serious challenges for Democratic presidential
politics. A deficit of four electoral votes would grow to a deficit of 43
votes. This method also depends on the House size and very heavily on the
composition of the resulting districts in the states. Its effects with
regard to a changing House size are very hard to estimate.
Some readers may wonder if we are calling for a revision of our electoral
system. We are not, since, as we point out above, it is politically unlikely
that the system will change and there are some positive reasons to support
the current system. We present the above arguments as a contribution to
informed discussion. We do not pretend to know what other considerations
might arise in the future but feel that careful consideration of our
electoral system requires understanding rather than unfaulting advocacy. It
is not the nature of our system to rest upon divine or unquestioned
perfection of the rules but to believe in ongoing consideration. We are
reminded of Robert Nozick's (Nozick 1968, pp.
xii-xiv) comments: “Works of philosophy are written as though their authors
believe them to be the absolutely final word on their subject…. Why do they
strive to force everything into that one fixed perimeter? What does having
everything within a perimeter do for us? Why do we want it so?… I propose to
give it all to you: the doubts, and worries and uncertainties as well as the
beliefs, convictions, and arguments.”
Appendix
Though apportionment appears at first sight to be a straightforward
problem that should allow for a satisfactory answer, the real story is
quite a bit more complex. During the last 225 years of U.S. history
several methods of apportionment have been in use. Tannenbaum and
Arnold's book (Tannenbaum and Arnold 2001)
gives a nice summary of dates of importance to the history of
apportionment in the U.S. We can only offer a small glimpse at the
subtleties of apportionment. We refer the interested reader to Balinski
and Young's definitive treatment (Balinski and Young
2001). The U.S. Constitution prescribes the apportionment of
the EC based on the apportionment of the House but it does not specify a
method of apportionment for the House.
The first attempt at solving the apportionment problem usually revolves
around the idea of the natural divisor. For any given size of the House,
which we call H, and a given total population of the U.S., which we call
P, we define the natural divisor D = P/H. The natural divisor D measures
the number of people per representative. If D = 450,000 then we say that
there is one representative for every 450,000 citizens. If we now divide
the population of each state by D we get a natural quota for each state.
As the reader will have probably noticed, the natural quota is almost
never a whole number but involves fractional parts.
Unfortunately, it is impossible to come up with good rules to deal with
the inevitable fractional parts of the natural quotas. For example, if a
state's natural quota is 11.567 then we need to decide if the state
should get 11 or 12 representatives. Rounding conventionally, i.e.,
rounding up above.5 and rounding down below.5, leaves us in most cases
with either too many or too few representatives and is therefore not an
option.
Alexander Hamilton proposed the following apparently sensible method:
Give each state its natural quota rounded down, in our example 11, and
then apportion any extra seats based on the size of the fractional parts
of the natural quota, with the states with largest fractional parts each
getting one extra seat until the correct number is reached.
Hamilton's, aka Vinton's, method leads to the following paradox, called
the Alabama Paradox. In 1880, with the size of the House at 299, Alabama
would have been entitled to eight seats while, with the size of the
House at 300, Alabama would have been entitled to only seven seats. It
seemed highly undesirable for a state's apportionment to go down as the
size of the House increases. This problem finally doomed Hamilton's
method in 1911 after Congress had adopted it in 1852. Any replacement of
Hamilton's method needed to have the “House size property,” i.e., an
increase in the size of the House cannot lower the apportionment of any
state. Balinski and Young (Balinski and Young
2001) have shown that any apportionment method based on
natural quotas will produce the Alabama paradox in some instances.
The other idea used to solve the apportionment problem revolves around
the district sizes. If a state's apportionment is N representatives
while its population is P, then the average district size is P/N. In
fact, the courts have ruled that in drawing their districts the states
insure that every district size is very close to the quotient P/N.
An obvious goal now is to make the district sizes in all states “as equal
as possible.” However, it is this notion of “as equal as possible” that
is the cause for disagreement; several competing methods have been
proposed on how to measure “as equal as possible.” We describe two
different measurements that lead to different apportionment methods
followed by an example to illustrate their difference. First, we owe the
reader an explanation of the terminology. The absolute difference of two
positive numbers A and B, where A is greater than B, is A – B. Their
relative difference is (A – B)/B.
Dean's method apportions to each state a number of seats such that no
transfer of one seat from one state to another state lowers the absolute
difference of the average district sizes of the two states, i.e., the
absolute differences of the average district sizes are minimized. The
Method of Equal Proportions or Huntington-Hill method is different only
in that it uses the relative differences as the criterion for a transfer
of seats.
The apportionment method currently in use is the Method of Equal
Proportions or Huntington-Hill method. How it got to be named Method of
Equal Proportion is a story told very well by Balinski and Young (Balinski and Young 2001). Let it be said here
only that naming it the Method of Equal Proportions was for the purpose
of making it appear to be the only unbiased among several apportionment
methods. That it is, in fact, biased has been argued repeatedly (see,
e.g., Balinski and Young 2001; Poston 1997).
The following example explains the difference in the two methods. It gave
rise to the Supreme Court case U.S. Department of Commerce v. Montana,
No. 91–860. In the 1990 census, Montana's population was determined to
be 803,655 and the state of Washington's population was determined to be
4,887,941. Montana was apportioned one seat while Washington received
nine seats. The district size for Montana was 803,655 while the district
size for Washington was 4,887,941/9 = 543,104.55. The absolute
difference of these two numbers is 260,550.44 while their relative
difference is 0.480.
Now consider a transfer of one seat from Washington to Montana, i.e.,
Montana has now two seats while Washington has only eight. The new
district sizes are 401,827.5 for Montana and 610,992.625 for Washington.
The absolute difference of the new district sizes is 209,165.125 while
the relative difference now is 0.521.
Thus, a transfer of one seat from Washington to Montana results in a
decrease of the absolute difference of the district sizes and according
to Dean's method this transfer should then happen. However, the same
transfer leads to an increase in the relative difference of the district
sizes and hence violates the stipulation of the Huntington-Hill method.
The Supreme Court agreed with the Department of Commerce by ruling that
the Huntington-Hill method meets constitutional requirements and Montana
did not gain a second seat.
