1. Introduction
To ensure high growth performance in plants, a continuous and reliable water supply to photosynthetically active organs is essential. Long-distance water transport in trees occurs along the soil–plant–atmosphere continuum that creates a continuous pathway of liquid water extending from the roots to the leaves (Tyree & Zimmermann Reference Tyree and Zimmermann2002). Along this gradient, water is moved by following the descending water potential gradient through an interconnected network of xylem conduits (Lambers et al. Reference Lambers, Chapin and Pons2008). The efficiency of this network to transport water varies between tree species and depends on their plant hydraulic architecture (Kotowska et al. Reference Kotowska, Hertel, Rajab, Barus and Schuldt2015, Olson et al. Reference Olson, Soriano, Rosell, Anfodillo, Donoghue, Edwards, Gómez, Dawson, Martinez, Castorena, Echeverria, Espinosa, Fajardo, Gazol, Isnard, Lima, Marcati and Alonzo2018, Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013). Along this hydraulic pathway, hydraulic efficiency and thus the resistance of a given segment is described by the potential hydraulic conductivity (K p), which depends on the number and diameter of vessels per cross-section as hydraulic conductivity scales with the fourth power according to the Hagen–Poiseuille equation (Tyree & Ewers Reference Tyree and Ewers1991, Tyree & Zimmermann Reference Tyree and Zimmermann2002). To increase hydraulic conductivity, plants might therefore enhance the vessel lumen fraction, i.e. the lumen-to-sapwood area ratio (A l:A x), by either producing larger vessel diameters or by increasing the number of vessels, or both.
How high water needs to be transported to reach the leaves thereby plays a critical role in the construction, structure and stability of the hydraulic network as large stature poses challenges for trees, both from a mechanical and hydraulic perspective (Larjavaara Reference Larjavaara2014, Ryan & Yoder Reference Ryan and Yoder1997). Accordingly, tree height has substantial implications for other plant functions, such as canopy and stem dimensions (Sterck & Bongers Reference Sterck and Bongers2001, Larjavaara Reference Larjavaara2014), leaf area index and water use (Falster et al. Reference Falster, Brännström, Dieckmann and Westoby2011, Horna et al. Reference Horna, Schuldt, Brix and Leuschner2011) as well as carbon gain and storage (Feldpausch et al. Reference Feldpausch, Lloyd, Lewis, Brienen, Gloor, Monteagudo Mendoza, Lopez-Gonzalez, Banin, Abu Salim, Affum-Baffoe, Alexiades, Almeida, Amaral, Andrade, Aragão, Araujo Murakami, Arets, Arroyo, Aymard, Baker, Bánki, Berry, Cardozo, Chave, Comiskey, Alvarez, De Oliveira, Di Fiore, Djagbletey, Domingues, Erwin, Fearnside, França, Freitas, Higuchi, Honorio, Iida, Jiménez, Kassim, Killeen, Laurance, Lovett, Malhi, Marimon, Marimon-Junior, Lenza, Marshall, Mendoza, Metcalfe, Mitchard, Neill, Nelson, Nilus, Nogueira, Parada, Peh, Pena, Cruz, Peñuela, Pitman, Prieto, Quesada, Ramírez, Ramírez-Angulo, Reitsma, Rudas, Saiz, Salomão, Schwarz, Silva, Silva-Espejo, Silveira, Sonké, Stropp, Taedoumg, Tan, Ter Steege, Terborgh, Torello-Raventos, Van Der Heijden, Vásquez, Vilanova, Vos, White, Willcock, Woell and Phillips2012, Kotowska et al. Reference Kotowska, Link, Röll, Hertel, Hölscher, Waite, Moser, Tjoa, Leuschner and Schuldt2021), but also drought-induced mortality (Bennett et al. Reference Bennett, Mcdowell, Allen and Anderson-Teixeira2015, O’Brien et al. Reference O’Brien, Engelbrecht, Joswig, Pereyra, Schuldt, Jansen, Kattge, Landhäusser, Levick, Preisler, Väänänen and Macinnis-Ng2017). Because top canopy trees tend to receive a higher light intensity than their smaller neighbours (Cavaleri et al. Reference Cavaleri, Oberbauer, Clark, Clark and Ryan2010), tree height seems more important in terms of competitive fitness than standing biomass (Falster et al. Reference Falster, Westoby and Falster2003). This facilitates a disproportionate share of resources, which increases their competitiveness (Feng et al. Reference Feng, Raza, Li, Chen, Bin, Du, Liu, Wu, Song, Yu, Zhang, Yuan, Yang and Yang2019, Toldi et al. Reference Toldi, Gyugos, Darkó, Szalai, Gulyás, Gierczik, Székely, Boldizsár, Galiba, Müller, Simon-Sarkadi and Kocsy2019, Weiner Reference Weiner1990), while shorter trees may have greater adaptation capacity and higher drought resistance (Fajardo et al. Reference Fajardo, McIntire and Olson2019). On the other hand, large stature poses several challenges for trees. While taller trees are more wind-exposed and thus more vulnerable to breaking (Jackson et al. Reference Jackson, Shenkin, Majalap, Bin Jami, Bin Sailim, Reynolds, Coomes, Chandler, Boyd, Burt, Wilkes, Disney and Malhi2020), water transport from roots to the leaves is increasingly challenged by gravity and path-length resistance.
While wide and conductive vessels allow greater rates of sap movement (Kotowska et al. Reference Kotowska, Link, Röll, Hertel, Hölscher, Waite, Moser, Tjoa, Leuschner and Schuldt2021), they also leave more space for fibres, which mainly serve for mechanical support (Chave et al. Reference Chave, Coomes, Jansen, Lewis, Swenson and Amy2009, Martínez-Cabrera et al. Reference Martínez-Cabrera, Jochen Schenk, Cevallos-Ferriz and Jones2011, Ziemińska et al. Reference Ziemińska, Butler, Gleason, Wright and Westoby2013). This might trade-off with the density of the woody tissue, and in fact wood density has been associated with vessel features and thus water transport capacitance in various ecosystems (Hoeber et al. Reference Hoeber, Leuschner, Köhler, Arias-aguilar and Schuldt2014, Pfautsch et al. Reference Pfautsch, Harbusch, Wesolowski, Smith, Macfarlene, Tjoelker, Reich and Adam2016, Preston et al. Reference Preston, Cornwell, Denoyer and Preston2006). Hard-wooded trees have often been associated with greater hydraulic safety, longevity and reduced growth rate, while fast growth comes at the cost of elevated mortality risk due to hydraulic failure and lower resistance to environmental hazards and pathogens (e.g. Eller et al., Reference Eller, de Barros, Bittencourt, Rowland, Mencuccini and Oliveira2018, King et al., Reference King, Davies, Supardi and Tan2006; Kraft et al., Reference Kraft, Metz, Condit and Chave2010). In evergreen wet tropical forests, on the other hand, wood density was found to vary independent of any hydraulic trait (Baraloto et al. Reference Baraloto, Morneau, Bonal, Blanc and Ferry2007, Fan et al. Reference Fan, Zhang, Hao, Slik and Cao2012, McCulloh et al. Reference McCulloh, Sperry, Lachenbruch, Meinzer, Reich and Voelker2010, Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013), with tree size and hydraulic efficiency playing the major role for carbon gain and water use (Kotowska et al. Reference Kotowska, Link, Röll, Hertel, Hölscher, Waite, Moser, Tjoa, Leuschner and Schuldt2021). Therefore, hydraulic efficiency has been identified as the pivotal role in terrestrial plant productivity from the individual tree to the ecosystem level (Brodribb Reference Brodribb2009).
As trees grow tall, they are thus facing hydraulic limitations, which might result in reduced transpiration and lower photosynthesis rates resulting in reduced growth (Ambrose et al. Reference Ambrose, Baxter, Martin, Francis, Asner, Nydick and Dawson2018, Koch et al. Reference Koch, Stillet, Jennings and Davis2004, Ryan & Yoder Reference Ryan and Yoder1997). To ensure sufficient water transport to the upper leaves, the development of an optimal well-adapted water flow pathway is therefore required in order to support the potential maximum tree height and water demand a given species can reach. Because the terminal twigs are exposed to the steepest gradients in evaporative demand and the highest gravitational force and friction, narrow conduits seem advantageous in order to prevent hydraulic dysfunction (Olson et al. Reference Olson, Soriano, Rosell, Anfodillo, Donoghue, Edwards, Gómez, Dawson, Martinez, Castorena, Echeverria, Espinosa, Fajardo, Gazol, Isnard, Lima, Marcati and Alonzo2018). As a consequence, basipetal conduit widening from the apex towards the stem base is a universal pattern, and tall trees consequently have larger vessels at the stem base than smaller trees to allow for high flow rates (Fang et al. Reference Fang, Turner, Xu, Jin, He and Li2013, Kotowska et al. Reference Kotowska, Link, Röll, Hertel, Hölscher, Waite, Moser, Tjoa, Leuschner and Schuldt2021, Olson et al. Reference Olson, Anfodillo, Rosell, Petit, Crivellaro, Isnard, León-Gómez, Leonardo and Matiss2014, Reference Olson, Rosell, Martinez-Perez, Leon-Gomez, Fajardo, Isnard, Cervantes-Alcayde, Echeverria, Figueroa-Abundiz, Segovia-Rivas, Trueba and Vasquez-Segovia2020, Poorter et al. Reference Poorter, Mcdonald, Fichtler, Licona, Alarco, Sterck, Villegas and Sass-klaassen2010, Toft et al. Reference Toft, Alam, Wilkie and Topp2019). This tip-to-base conduit diameter widening with tree height was predicted by hydraulic optimality models (West et al. Reference West, Brown and Enquist1999) and reported to follow a power law throughout many studies (Anfodillo et al. Reference Anfodillo, Carraro, Carrer, Fior and Rossi2006, Coomes & Allen Reference Coomes and Allen2007, Olson et al. Reference Olson, Anfodillo, Gleason and McCulloh2021, Petit et al. Reference Petit, Pfautsch, Anfodillo and Adams2010, Petit & Crivellaro Reference Petit and Crivellaro2014, Rosell et al. Reference Rosell, Olson and Anfodillo2017). In contrast, the adjustments of hydraulic traits at the upper canopy in relation to the actual and potential tree height a species can reach are less documented, but available studies indicate that vessel diameters in sun-exposed top canopy branches increase with height, mainly across species (Kotowska et al. Reference Kotowska, Hertel, Rajab, Barus and Schuldt2015, Liu et al. Reference Liu, Gleason, Hao, Hua and He2019, Olson et al. Reference Olson, Anfodillo, Rosell, Petit, Crivellaro, Isnard, León-Gómez, Leonardo and Matiss2014, Reference Olson, Anfodillo, Gleason and McCulloh2021, Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013, Zach et al. Reference Zach, Schuldt, Brix, Horna, Culmsee and Leuschner2010). At first glance, this seems to contradict the assumption that hydraulic efficiency must decline vertically in order to compensate for the increase in gravitational force and resistance friction with height (Burgess et al. Reference Burgess, Pittermann and Dawson2006, Halis & Djehichi Reference Halis and Djehichi2012). Indeed, Woodruff et al. (Reference Woodruff, Meinzer and Lachenbruch2008) observed a linear intra-specific decline in vessel diameters in upper-canopy branches with height in a tall-growing conifer, but comparable data are scarce.
In order to test whether the tree height and canopy position a given species may reach at maturity influences hydraulics traits of terminal twigs of tropical angiosperm trees, we collected sun-exposed samples from the uppermost canopy of 279 tree species from a phylogenetically and structurally diverse Indonesian lowland rainforest. We analysed the influences of actual (H) and maximal tree height (H max), diameter at breast height (DBH) and stem wood density (WD) on wood anatomical and derived hydraulic traits in these terminal twigs. We hypothesized that (i) trees from tall species have disproportionately wider vessels in the upper-most canopy branches to optimize for maximal conductive demand, (ii) while their mechanical stability expressed as wood density is unrelated to vessel sizes and does not trade-off with hydraulic conductance in this humid tropical environment.
2. Materials and methods
2.1. Study sites and macroclimate conditions
The research was carried out in two tropical lowland rainforests remnants (50–60 m a.s.l) of Jambi Province, Sumatra. The study sites are located in two protected areas: Harapan rainforest (HR) of Muara Bulian Regency (S 2º0´ E 103º24´) and Bukit Duabelas National Park (BD) of Sarolangun Regency (S 1º56´ E 102º34´). Both protected areas correspond to former logging concessions and have been protected for more than 20 years. The forests can be considered as logged-over primary lowland forest. Stand characteristics measured in 2019 for all trees with a DBH >10 cm did not differ between the two landscapes (HR and BD, respectively) (t = −0.36, p > 0.05, Table 1). Acrisols are the dominant soils in both sites, with clay texture in BD and a sandy loam texture in HR (Allen et al. Reference Allen, Corre, Tjoa and Veldkamp2015). The climate is moist tropical with a mean annual precipitation of 2,552 mm yr−1, a mean daily temperature of 24ºC and 25.3ºC, and a mean daily relative humidity of 92% and 90% at BD and HR sites, respectively (Drescher et al. Reference Drescher, Rembold, Allen, Beckscha, Buchori, Clough, Faust, Fauzi, Gunawan, Hertel, Irawan, Jaya, Klarner, Kleinn, Knohl, Kotowska, Krashevska, Krishna, Leuschner, Lorenz, Meijide, Melati, Steinebach, Tjoa, Tscharntke, Wick, Wiegand, Kreft and Scheu2016).
Table 1. Stand characteristics for all trees with DBH >10 cm for the two landscapes Harapan (HR) and Bukit 12 (BD) measured in 2019. Given are mean values ± SD for the four plots per landscapes

2.2. Tree selection and height measurements
The twig samples were collected at eight 50 m × 50 m forest inventory plots at each location from tree individuals with a DBH ≥ 10 cm. We aimed at sampling one mature individual of each species, which represents the maximum height reached by that species across all plots. In total, 279 species belonging to 142 genera and 56 families were sampled (Table S1). Due to logistic reasons, however, it was not always possible to sample the tallest individual per species. We therefore additionally estimated the maximum height of all tree species from our inventory data covering 3517 forest tree individuals in total. Across our species sample, actual tree height (H, m) and maximal tree height (H max, m) were highly interrelated (Fig. 1), and in most cases we indeed managed to collect samples from the tallest individuals. Sun-exposed upper-canopy branches were collected by using a slingshot (Big Shot, Notch Equipment, USA) to pull up a hand chain saw (RoNa, Düsseldorf, Germany) for cutting-off the branch. Across all species, similar-sized twigs with a diameter of 8.84 ± 1.61 mm (mean ± SD) were selected and stored in 70% ethanol for subsequent wood anatomical analysis. Tree height was measured using a Vertex III height meter (Haglof, Sweden).

Figure 1. Linear regression analysis between actual and reported maximum height for the 199 tropical tree species from lowland forests on Sumatra (a), as well as histograms showing the number of species per selected tree height class for actual tree height (b) and maximum tree height (c).
2.3. Vessels anatomy and potential hydraulic conductivity
The complete samples were sectioned using a sliding microtome (G.S.L.1, WSL Bismendorf, Switzerland). The resulting semi-thin cross-sections were stained using a mixture of 0.65% alcian blue and 0.35% safranin (w/v), and subsequently digitalized using a stereo-microscope with an automatic stage equipped with a digital camera (SteREOV20, Carl Zeiss MicroImaging GmbH).
Image processing was done with the software Adobe Photoshop CS6 (Adobe Systems Inc., U.S.A.) and ImageJ v1.5.2. for analyzing the diameter and number of all xylem vessels in the complete cross-section by the automated particle analysis function and to calculate the corresponding xylem area. All vessels of a cross-section (xylem area mean ± SD: 45.4 ± 24.0 mm2) were analyzed, yielding 600–20,000 measured vessels per sample. We estimated vessel diameter (D, µm) from major (a) and minor (b) vessel radii according to D i = ((32 × (a × b)3)/(a2 + b2))¼ (White & Majdalani Reference White and Majdalani1999), the hydraulically weighted vessel diameter (D h) according to D h = ∑D i5/∑D i4 (Sperry & Saliendra Reference Sperry and Saliendra1994), vessel density (VD, n mm−2) and the lumen-to-sapwood area ratio (A l:A x, in percentage; see Table 2 for descriptions). As the flow rate of water along a tube is proportional to the fourth power of its radius, a small increase in conduit diameter causes a substantial increase in flow rate or conductivity (Sperry et al. Reference Sperry, Hacke and Pittermann2006). The hydraulically weighted vessel diameter corresponds to the lumen of average Hagen–Poiseuille conductivity for the sampled cross-section area and thus is a better predictor of hydraulic conductivity than the non-weighted mean vessel diameter (Hacke et al. Reference Hacke, Spicer, Schreiber and Plavcová2017). We further calculated the potential hydraulic conductivity (K p, kg m−1 MPa−1 s−1) of the twig xylem based on the Hagen–Poiseuille’s law as K p = π × ρ × Σ(D i4)/(128 η × A xylem), where D i is the diameter of each single vessel i, η the water viscosity (1.002×10−9 MPa s) and ρ the density of water (998.2 kg m−3), both at 20 °C, and A xylem (m2) the analyzed sapwood area.
Table 2. List of tree structural and twig wood anatomical variables with definition and units employed.

2.4. Statistical analyses
Statistical analyses were performed using R software version 3.6.1 (R Core Team 2019) and the packages corrmorant (Link Reference Link2020), ggplot2 (Wickham Reference Wickham2016), and ggpubr (Kassambara Reference Kassambara2020). We investigated the effect of tree height on hydraulically weighted vessel diameter (D h), VD, lumen-to-sapwood area ratio (A l:A x) and potential hydraulic conductivity (K p) of twigs using linear regression analyses. We additionally grouped given species according to their measured actual tree height (H) and documented maximum tree height per species in the region (H max). We created eight tree height classes for H (5.0–9.9 m, n = 10; 10.0–14.9 m, n = 34; 15.0–19.9 m, n = 75; 20.0–24.9 m, n = 72; 25.0–29.9 m, n = 44; 30.0–34.9 m, n = 23; 35.0–39.9 m, n = 12; >40.0 m, n = 9) and eight tree height classes for H max (<14.9 m, n = 16; 15.0–19.9 m, n = 62; 20.0–24.9 m, n = 57; 25.0–29.9 m, n = 57; 30.0–34.9 m, n = 37; 35.0–39.9 m, n = 31; 40.0–44.9 m, n = 8; >45.0, n = 11). For a linear regression analysis across height classes, H or H max and the variable of interest was averaged, ignoring the unbalanced species number per height class. A first inspection of the dataset was done with the help of the correlation matrix shown in Fig. S1, and linear regression analyses were used to identify a significant effect of tree height on the variable of interest. Kruskal–Wallis test were performed to test whether height classes differ significantly from each other for the hydraulic trait of interest. Non-normally distributed data were log-transformed.
3. Results
Across the 279 tropical rainforest tree species of our sample, actual tree height (H) was closely related to the maximum tree height (H max) reported for a given species, and to their DBH (Figure 1a, Figiure S1). Although we observed quite a variability across species and a low coefficient of determination, D h and K p of terminal twigs increased significantly with tree height (p < 0.001 and p < 0.05, respectively) (Fig. 2). We found no effect of tree height on VD and lumen-to-sapwood area ratio (Fig. 2). According to the linear regression analysis, log transformed D h and K p were increasing with tree height by 0.64 µm and 0.34 kg m−1 MPa−1 s−1 per meter across species, respectively. In addition to the actual height of a given tree (H), D h and K p likewise increased with the maximum height (H max) of the species (R 2 = 0.05, p < 0.001 and R 2 = 0.02, p < 0.01, respectively). Unlike with the actual tree height, we found that VD decreased (R 2 = 0.01, p < 0.05) with H max (Fig. S2). As with the actual height, no correlation was observed between A l:A x and H max (R 2 < 0.01, p = 0.31).

Figure 2. Actual height of the 279 tropical rainforest species in relationship to vessel density (a), hydraulically weighted vessel diameter (c), and potential hydraulic conductivity (d). Red lines are regression curves of linier regression model (a, c, d) while dashed line represents a non-significant influence (b). Grey areas show the 95% confident interval of the models.
When pooled across species within their given tree height class, D h and K p likewise differed significantly between height classes according to a Kruskal–Wallis test (p < 0.01 and p < 0.05, respectively; Fig. 3). Ignoring the variable number of species per tree height class (refer to Figure 1b, c), a linear regression analysis between mean tree height per height class likewise revealed a strong relationship for both D h and K p (R 2 = 0.68, p < 0.05 and R 2 = 0.60, p < 0.05, respectively; Fig. S3). This relation became even stronger for categories created according to the mean maximal tree height per height class documented for each species in the region against D h and K p (R 2 = 0.75, p < 0.001 and R 2 = 0.85, p < 0.001, respectively). However, when accounting for each class variance, Kruskall–Wallis test was non-significant in the case of Kp across H max classes. According to the regression analysis across the mean of each classes, hydraulically weighted vessel diameters increased from 53.9 ± 4.0 µm at 11.8 ± 0.6 m H max to 79.3 ± 15.0 µm at 42.6 ± 0.6 m H max (mean ± SE), i.e. D h increased by 0.82 µm per meter across species (data not shown). In contrast, no significant differences between height classes with respect to VD and A l:A x were found (Fig. 3).

Figure 3. Differences of hydraulic traits across aggregated actual tree height classes for the lumen-to-sapwood area ratio (a), hydraulically weighted vessel diameter (b), vessel density (d) and potential hydraulic conductivity (e), as well as the hydraulically weighted vessel diameter (c) and potential hydraulic conductivity (f) across tree height classes based on the maximum tree height of a given species in the region.
We further observed a weak but highly significant relationship between D h and the stem DBH as well as WD (Fig. 4). The latter remained significant even after excluding the two most light-wooded species. Although the r-square values are likewise low across the 279 tree species (R 2 < 0.1), D h increased (p < 0.01) while WD declined (p < 0.01) with increasing height.

Figure 4. Diameter at breast height (a) and wood density (b) in relationship to the hydraulically-weighted vessel diameter of twigs across a wide range of tropical tree species.
Across species differing in height, we found several different vessel diameter distributions (Fig. 5). For example, the wood of Dichapetalum gelonioides (Roxb) Engl – a rather small species reaching 14 m height in our sample – is characterized by small vessels (10 – 40 µm in diameter), while Syzygium acuminatissimum (Blume) DC (21 m height) had a wider range of different vessel sizes and overall larger vessels (20–80 µm). In contrast, Mezzettia parviflora (Becc), which reaches different heights with S. acuminatissimum and D. gelonioides (46 m height), showed a different pattern than the two previous species by having vessel diameters ranging from 80 to 90 µm up to >100 µm.
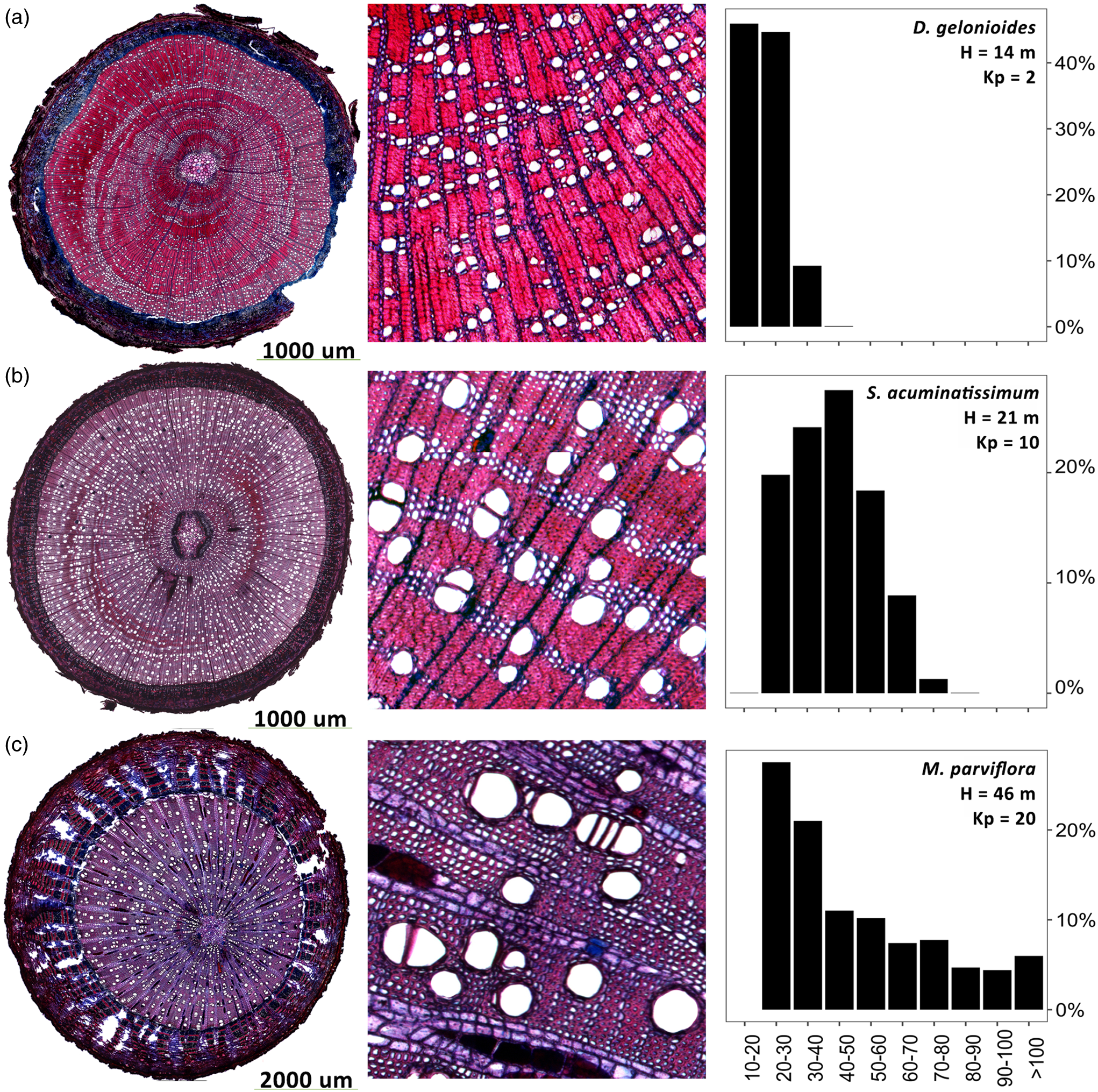
Figure 5. Vessel anatomy and frequency of three tropical tree species differing in their vascular architecture. Shown are three representatives for species with relatively small (Dichapetalum gelonioides (a)), medium (Syzygium acuminatissimum (b)), and large (Mezzetia parviflora (c)) vessel diameters.
4. Discussion
In our study across 279 lowland tropical tree species, we found a significant increase in hydraulically weighted vessel diameter (D h) and potential hydraulic conductivity (K p) with tree height. Because of the close relationship between tree height and the DBH, the latter was likewise closely related to D h. Besides the well-described pattern of basipetal conduit widening from the apex towards the stem base in plants of all sizes and genera (Olson et al. Reference Olson, Anfodillo, Gleason and McCulloh2021), the frequently observed phenomenon that tall trees show relatively larger vessels in their terminal twigs (cf. Kotowska et al. Reference Kotowska, Hertel, Rajab, Barus and Schuldt2015, Liu et al. Reference Liu, Gleason, Hao, Hua and He2019, Olson et al. Reference Olson, Anfodillo, Rosell, Petit, Crivellaro, Isnard, León-Gómez, Leonardo and Matiss2014, Reference Olson, Anfodillo, Gleason and McCulloh2021, Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013, Zach et al. Reference Zach, Schuldt, Brix, Horna, Culmsee and Leuschner2010) has not yet been elucidated. Although there are good arguments to expect conduit diameter at the same twig position to stagnate or even decline with increasing height, it is important to consider what organizational level has been analyzed. Our results are supportive for the assumption that the terminal twig anatomy is predetermined by the water demand a given species will reach at maturity, which explains why the grouped data according to the reported maximum tree height of a given species in the region revealed the closest relationship with D h. This would imply a relatively close genetic control of initial vessel sizes of tree species when corrected for tree size and sampling position; with vessel diameters and potential conductivities of twigs showing much less variability within provenances and within species than other physiological and morphological traits associated with hydraulic functioning such as turgor loss point, δ13C, 50% of loss of hydraulic conductivity, and leaf morphological traits (Schreiber et al. Reference Schreiber, Hacke, Hamann and Thomas2011; Hajek et al. Reference Hajek, Kurjak, von Wühlisch, Delzon and Schuldt2016) and the expression of traits associated with hydraulic safety to be genetically controlled (Pritzkow et al. Reference Pritzkow, Williamson, Szota, Trouvé and Arndt2020). Across species, on the one hand, the terminal twig anatomy thus may reflect the growth potential and ecological niche (understory versus overstory or emergent) of a given species.
In the stem wood on the other hand, two gradients simultaneously affect vascular differentiation, namely the radial cambial age gradient that most likely can be attributed to different concentrations in plant hormones such as auxin (Hacke et al. Reference Hacke, Spicer, Schreiber and Plavcová2017) and the vertical cell turgor gradient (cf. Li et al. Reference Li, Li, Link, Li, Deng, Schuldt, Jiang, Zhao, Zheng, Li and Yin2019). In similar-sized branches with equal distance to the tip, only the vertical cell turgor gradient affects the vascular differentiation (Lockhart Reference Lockhart1965, Woodruff et al. Reference Woodruff, Bond and Meinzer2004, Reference Woodruff, Meinzer and Lachenbruch2008). Within the canopy of five tall European beech trees, for example, Gottschall et al. (Reference Gottschall, Davids, Newiger‐Dous, Auge, Cesarz and Eisenhauer2019) observed a linear decline in vessel diameter in similar-sized branches from 17.3 ± 0.3 µm in 20 m height to 15.5 ± 0.3 µm 40 m height, i.e. by 11% only. In trees of the same species, the xylem anatomy of terminal twigs should therefore be more or less comparable as described above, because basipetal path length is the main driver and the most influential factor affecting xylem vessel diameter variation (Fajardo et al. Reference Fajardo, Martínez-Pérez, Cervantes-Alcayde and Olson2020, Olson et al. Reference Olson, Anfodillo, Rosell, Petit, Crivellaro, Isnard, León-Gómez, Leonardo and Matiss2014). However, studies currently show contrasted results, and it seems that vascular differentiation could also result from adaptive causes rather than non-adaptative ones such as vertical cell turgor gradient (Olson et al., Reference Olson, Anfodillo, Gleason and McCulloh2021).
A classic principle of plant hydraulic architecture postulates that the wood of trees growing in wet environments is composed of wider xylem conduits than the wood of trees from dry environments (Baas Reference Baas1973, Carlquist Reference Carlquist1977, Chenlemuge et al. Reference Chenlemuge, Schuldt, Hertel, Dulamsuren, Hauck and Leuschner2015, Fajardo et al. Reference Fajardo, Martínez-Pérez, Cervantes-Alcayde and Olson2020, Martinez-Cabrera et al. Reference Martinez-Cabrera, Jones, Espino and Schenk2009, Pfautsch Reference Pfautsch2016, Schuldt et al. Reference Schuldt, Knutzen, Delzon, Jansen, Müller‐Haubold, Burlett, Clough and Leuschner2016). Only recently, however, it has been shown that water limitation does not directly translate into smaller xylem vessels, but that drought-exposure results in reduced growth and height and thus flow-path length from the stem base towards the distal twigs (Olson et al. Reference Olson, Anfodillo, Rosell, Petit, Crivellaro, Isnard, León-Gómez, Leonardo and Matiss2014, Reference Olson, Soriano, Rosell, Anfodillo, Donoghue, Edwards, Gómez, Dawson, Martinez, Castorena, Echeverria, Espinosa, Fajardo, Gazol, Isnard, Lima, Marcati and Alonzo2018, Reference Olson, Rosell, Martinez-Perez, Leon-Gomez, Fajardo, Isnard, Cervantes-Alcayde, Echeverria, Figueroa-Abundiz, Segovia-Rivas, Trueba and Vasquez-Segovia2020, Reference Olson, Anfodillo, Gleason and McCulloh2021, Rosell et al. Reference Rosell, Olson and Anfodillo2017). The tree species in this study evolved in humid tropical environments with precipitation rates beyond 2,000 mm yr−1 and do normally not face frequent drought stress, which can decrease water transport efficiency and might lower potential hydraulic conductivity of the xylem vessels. As an efficient and highly conductive xylem is indeed a perquisite for high sap flux densities in these trees (Kotowska et al. Reference Kotowska, Link, Röll, Hertel, Hölscher, Waite, Moser, Tjoa, Leuschner and Schuldt2021) in response to a vertical gradient in evaporative demand within the stand (Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013), it seems beneficial for tall-growing overstory tree species to produce a highly efficient xylem that enables high transpiration rates during the diurnal peak in evaporative demand. Our results are in line with another tropical study (Zach et al. Reference Zach, Schuldt, Brix, Horna, Culmsee and Leuschner2010) and a global meta-analysis (Liu et al. Reference Liu, Gleason, Hao, Hua and He2019).
Because of controversial reports in the literature, we further tested whether the terminal twig anatomy was related to WD. We assume that WD is indicative of twig wood density because this wood trait does not vary greatly along the flow path in tropical trees (Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013). In general, fast-growing species tend to have less compact wood than slow-growing species, which often is associated with a higher hydraulic efficiency (Enquist et al. Reference Enquist, West, Charnov, Brown, Santa, Road, Fe, Division and Ms1999, King et al. Reference King, Davies, Supardi and Tan2006, Muller-Landau Reference Muller-Landau2004, Poorter et al. Reference Poorter, Right, Az, Ckerly, Ondit, Quez and Arms2008, Reference Poorter, Mcdonald, Fichtler, Licona, Alarco, Sterck, Villegas and Sass-klaassen2010), but lower mechanical strength (Fan et al. Reference Fan, Sterck, Zhang, Fu, Hao and Fan2017). While several studies have shown that wood density is related to xylem anatomy as well as hydraulic properties (Hoeber et al. Reference Hoeber, Leuschner, Köhler, Arias-aguilar and Schuldt2014, McCulloh et al. Reference McCulloh, Meinzer, Sperry, Lachenbruch, Voelker, Woodruff and Domec2011, Roque & Tomazelo-Filho Reference Roque and Tomazelo-Filho2007), others could not confirm these findings (Kotowska et al. Reference Kotowska, Hertel, Rajab, Barus and Schuldt2015, Martinez-Cabrera et al. Reference Martinez-Cabrera, Jones, Espino and Schenk2009, Poorter et al. Reference Poorter, Mcdonald, Fichtler, Licona, Alarco, Sterck, Villegas and Sass-klaassen2010, Russo et al. Reference Russo, Jenkins, Wiser, Uriarte, Duncan and Coomes2010). It was speculated that a given drought-stress exposure is needed to observe such a relationship (Schuldt et al. Reference Schuldt, Leuschner, Brock and Horna2013). Although the tree species of our study are not exposed to frequent edaphic or climatic aridity, we likewise observed a weak but significant negative relationship between D h and wood density in contrast to the assumption by Schuldt et al. (Reference Schuldt, Leuschner, Brock and Horna2013). The reason for this remains speculative because vessel diameter variation is only one component that affects differences in wood density across species due to its effect on the lumen-to-sapwood area ratio, and thus the space available for carbon storage. In addition, fibres and parenchymatic tissue strongly influence wood density variation (Ziemińska et al. Reference Ziemińska, Butler, Gleason, Wright and Westoby2013, Reference Ziemińska, Westoby and Wright2015).
5. Conclusions
The purpose of the current study was to analyze the effect of tree height on xylem anatomical traits of terminal twigs from fully grown individuals of 279 tropical lowland tree species. We show that hydraulically weighted vessel diameter (D h) and potential hydraulic conductivity (K p) increase with tree height across a wide range of species and values were largest in the species reaching the upper canopy. Evidence from this study points towards a differentiation of hydraulic strategies between functional groups with tree species reaching upper canopy or emergent position in tropical forests having distinct vascular anatomical adaptions i.e. larger vessel diameter in their terminal wood anatomy than tree species occurring in the understorey. The resulting highly conductive xylem allows to maximize for higher sap flux rates per cross sectional area and meet the water demand of the sun-exposed canopy experiencing pronounced fluctuations of vapor pressure deficits. To determine whether these observations are indeed based on a conservative trait expression under mainly genetic control, we emphasize the priorities of follow-up studies. These should focus on incorporating data on within-species variability, assembling one the one hand data on vascular anatomy along the whole flow path starting from leaf veins, to leaf petioles and following down to the stem while correcting for tree height and distance to leaf tip, and on the other hand, comparing whether various ontogenetic stages such as seedings, sapling and adult trees of tree species from different functional groups already show anatomical perquisite for their future ecological niche. Furthermore, incorporating phylogenetic information and – if feasible – using progeny trials will allow to determine the proportion of variance in vessel anatomy explained by phenotypic plasticity versus genetic control.
Supplementary material
To view supplementary material for this article, please visit https://doi.org/10.1017/S0266467422000335
Acknowledgments
This study is part of the German-Indonesian collaborative research centre (CRC) 990/EFForTS. The samples were collected under 25/EXT/SIP/FRP/SM/III/2013-2015, 42/EXT/SIP/FRP/E5/Dit. Ki/VII/2016, 56/EXT/SIP/FRP/E5/Dit. Ki/IX/2017, 2012/EXT/SIP/FRP/E5/Dit.KI/VII/2018. We thank the Indonesia Ministry of Finance and Indonesia Endowment Fund for Education (LPDP) for financial support in terms of research funds and scholarship. Thanks to the rangers in particular Khairul Anwar who supported us during the field research, the EFForTS A03 and Z02 subprojects for sharing climate data, PT REKI and Bukit Duabelas National Park authorities as well as the local assistants who helped collecting the samples.
Author contributions
B.S., M.K., and T.T. designed the study. F.B. supervised the field sampling campaign and P.-A.W. the wood anatomical sectioning. J.J. performed the wood anatomical and statistical analysis with the support of P-A.W, M.K., and T.T., and wrote the first version of the manuscript together with T.T and S.S., which was intensively discussed and revised by all authors.










