Bilinguals are faced with a cognitive challenge during speech production: words in both languages become active to some degree and may compete for selection (e.g., Kroll, Bobb, Misra & Guo, Reference Kroll, Bobb, Misra and Guo2008), suggesting that cognitive control mechanisms must be in place to handle this cross-language activation. Some theorists have argued that inhibitory control may serve this role during bilingual speech production (e.g., Costa, Santesteban & Ivanova, Reference Costa, Santesteban and Ivanova2006; Green, Reference Green1998). Evidence in support of the inhibitory control account comes from a number of sources, including behavioral and neurocognitive studies of language switching (e.g., Jackson, Swainson, Cunnington & Jackson, Reference Jackson, Swainson, Cunnington and Jackson2001; Meuter & Allport, Reference Meuter and Allport1999) and a growing body of research demonstrating that a lifetime of being bilingual incurs benefits to domain-general inhibitory control abilities (e.g., Bialystok, Reference Bialystok2009; Colzato, Bajo, van den Wildenberg, Paolieri, Nieuwenhuis, La Heij & Hommel, Reference Colzato, Bajo, van den Wildenberg, Paolieri, Nieuwenhuis, La Heij and Hommel2008). However, no study to date has reported a clear, direct connection between inhibitory control and online speech production. This study provides evidence of a relationship between domain-general inhibitory control and trilingual speech production in a language switching task.
When a monolingual views a picture, activation spreads from the conceptual representation to the associated lexical and phonological representations before verbalization can take place. This seemingly simple procedure becomes much more complex for multilingual individuals, since more than one lexical representation maps onto a given concept.Footnote 1 When a multilingual names a picture of a dog in one of his/her languages, words in all languages are activated to some degree, thereby requiring the individual to select the intended word for production. Eventually the multilingual verbalizes the correct word that matches the target language, suggesting that there must be a selection process that facilitates this procedure. Similarly, there must also be a cognitive control mechanism that prevents the wrong words from being produced. According to Green's (Reference Green1998) Inhibitory Control Model, all words contain language tags specifying to which language they belong. When words in the non-target language are activated during lexical access, they are inhibited by virtue of their non-target language tag, thereby allowing words in the target language to be selected and ultimately produced. Critically, the amount of inhibition applied to representations in the non-target language(s) is proportional to the extent to which they are activated, such that more inhibition is needed to suppress words belonging to a more dominant language. Thus, for example, when an unbalanced bilingual speaks in the less dominant second language (L2), it is assumed that first language (L1) translation equivalents are inhibited to a greater extent than L2 translation equivalents are when speaking in the L1 (e.g., Meuter & Allport, Reference Meuter and Allport1999).
Much of the evidence in support of the Inhibitory Control Model has come from language switching experiments that allow researchers to compare the amount of time it takes to switch into more- and less-dominant languages. Meuter and Allport (Reference Meuter and Allport1999) conducted a seminal language switching study in which language learners named lists of numerals in their L1 and L2 while switching back and forth between the two languages. The results suggested that switching into the L1 was more difficult because it was strongly inhibited during the previous trial in which the L2 was produced. Switching into the L2 was apparently less difficult because it did not require as much inhibition and was therefore faster to be reactivated. Meuter and Allport were the first to demonstrate asymmetrical language switch costs between L1 and L2, which have been assumed to reflect the differential amounts of time required to reactivate less- vs. more-dominant languages and have been interpreted as empirical indicators of inhibitory control (but see but see Gollan & Ferreira, Reference Gollan and Ferreira2009, for a discussion on how asymmetries can occur from involuntary switching, or Verhoef, Roelofs & Chwilla, Reference Verhoef, Roelofs and Chwilla2009, for an exploration of L1-repetition benefits).
Converging evidence of inhibitory control comes from both behavioral and neuroscience methods. For instance, Guo, Liu, Misra and Kroll (Reference Guo, Liu, Misra and Kroll2011) reported event-related potential (ERP) evidence of persisting inhibition in bilinguals well after a language switch, suggesting that global inhibitory control supports language switching and that the suppression of the L1 may have consequences for how bilinguals process the L1. Some of the first studies investigating inhibitory control among trilingual speakers have explored n–2 repetition costs (e.g., comparing L1–L2–L1 vs. L3–L2–L1 switch costs) as a direct measure of lingering inhibition in a trilingual experimental context and found additional support for persisting inhibition of abandoned languages (Koch, Gade, Schuch & Philipp, Reference Koch, Gade, Schuch and Philipp2010; Philipp, Gade & Koch, Reference Philipp, Gade and Koch2007; Philipp & Koch, Reference Philipp and Koch2009).
While each of these trilingual studies has made progress towards a better understanding of the reliance on inhibitory control in speech production, they have yet to address the extent to which switching performance is affected by the language on the trial immediately preceding a language switch – a factor that Schwieter and Sunderman (Reference Schwieter and Sunderman2011) recently noted may impact switching performance. Given the predictions of the Inhibitory Control Model (Green, Reference Green1998), our working hypothesis for the trilingual case is that separate task schemas support naming in each language, and each non-target language is suppressed independently. For example, activation of the L3 naming task schema enables successful L3 picture naming by increasing activation of L3 lemmas while simultaneously inhibiting both the L1 naming and L2 naming task schemas. For the lemmas in the more dominant L1, competing lemmas require additional inhibition relative to competing L2 lemmas, which is satisfied via greater reactive inhibition of activated L1 lemmas by the L3 naming task schema. To the extent that competing L2 lemmas become activated during speech planning, the L3 task schema will also reactively inhibit those lemmas, but to a much lesser degree. The same asymmetry in inhibition holds when naming pictures in an L2 that is less dominant than the L1. This framework leads to the prediction that, when switching into a less dominant L2 or L3, switch costs should be greater when switching from the dominant L1 than when switching between the less dominant languages – a prediction specific to the trilingual case that has implications for any theory of language switching (see also Philipp et al., Reference Philipp, Gade and Koch2007). That is, the amount of inhibition required on the current trial depends, in part, on the language of naming on the previous trial. A competing hypothesis could be that the language task schema is agnostic to the language membership of non-target language representations, and instead it simply inhibits activated language representations in any non-target language. By this account, the language of the previous trial should not affect the amount of inhibition applied to the competing representations.
Language dominance should also constrain the extent to which inhibitory control supports language switching. Specifically, since language switching is argued to rely more heavily on inhibitory control when there is a larger discrepancy in language dominance (Green, Reference Green1998; Meuter & Allport, Reference Meuter and Allport1999), better inhibitory control abilities should predict reduced switch costs when switching between highly unbalanced languages but not when switching between two less dominant languages. Trilingual language switching studies provide an opportunity unavailable in studies with bilingual participants by examining the potential effects of both the prior language of naming and language dominance within individuals, and this is one main contribution of the current study.
Another contribution of the current study is related to issues of domain-general inhibitory control abilities. In a recent examination of individual differences in language control, Festman, Rodríguez-Fornells and Münte (Reference Festman, Rodríguez-Fornells and Münte2010) compared two groups of late bilinguals, defined by their susceptibility to cross-language interference as indexed by language errors during a picture naming task (i.e., naming the picture in the incorrect language). They found that the bilingual group with better language control abilities had a cognitive advantage on tasks measuring executive functions (including inhibition) and verbal subtests of intelligence, providing preliminary evidence that individual differences in executive functions are related to language control.
There is also a growing body of research showing that a lifetime of experience being bilingual incurs cognitive benefits, with bilingual children and aging adults outperforming their age-matched monolingual counterparts on inhibitory control tasks (Bialystok, Reference Bialystok2009). These results have been found using a number of non-linguistic measures of inhibitory control, including the Simon task (e.g., Bialystok, Craik, Klein & Viswanathan, Reference Bialystok, Craik, Klein and Viswanathan2004), ambiguous figures (Bialystok & Shapero, Reference Bialystok and Shapero2005), and a modified antisaccade task (Bialystok, Craik & Ryan, Reference Bialystok, Craik and Ryan2006). Young adult bilinguals have also shown benefits relative to monolinguals in other related executive functions, such as attentional control in the attentional network task (Costa, Hernández & Sebastián-Gallés, Reference Costa, Hernández and Sebastián-Gallés2008) and shifting between mental sets during a task switching task (Prior & MacWhinney, Reference Prior and MacWhinney2010). Furthermore, a number of neurocognitive studies of bilinguals and L2 learners have found that online speech production is supported by frontal lobe regions that have been linked to inhibitory control and conflict resolution (for a review, see Abutalebi & Green, Reference Abutalebi and Green2008).
Present study
Taken together, these results implicate inhibitory control as a domain-general cognitive mechanism that supports bilingual speech production. However, most of the extant evidence has relied on group comparisons between monolinguals and bilinguals or between less and more proficient bilinguals. Festman et al. (Reference Festman, Rodríguez-Fornells and Münte2010) provided the first analysis of individual differences within one sample of late bilinguals, although they also relied on group-comparison analyses of executive functioning tasks between participants that were grouped based on language errors. Moreover, although language errors provide one measure of language processing difficulties, a reaction time (RT) analysis can provide a more sensitive measure of online language processing that may illuminate subtle differences between individuals. Specifically, we analyzed the range of scores on an inhibition task and examined whether those scores accounted for individual variation in switch costs in three languages. If speech production is indeed supported by a domain-general inhibitory control mechanism (e.g., Green, Reference Green1998), then individuals with greater inhibitory control abilities should be able to more efficiently perform language switches – that is, they should show smaller switch costs. We tested the hypotheses that (i) better inhibitory control is related to smaller switch costs in all languages, and that (ii) this relationship is particularly strong when switching into the L1, when inhibitory control has been theorized to be particularly important (Green, Reference Green1998). We operationalized inhibitory control using the Simon task (e.g., Simon & Rudell, Reference Simon and Rudell1967; Bialystok et al., Reference Bialystok, Craik, Klein and Viswanathan2004), a measure of one's ability to inhibit a prepotent response that is cued by distracting, irrelevant stimulus features. Critically, the Simon task is a non-linguistic task – participants must make a right or left button press based on the color of the stimulus while ignoring the spatial location of the stimulus. Thus, we contend that any relationship between performance on the Simon task and language switch costs would suggest that both tasks rely on a domain-general inhibitory control mechanism.
Method
Participants
Fifty-six native English (L1) speakers learning French (L2) and Spanish (L3) were recruited from a large public university in an English-speaking region of Ontario, Canada. These participants were currently enrolled in both L2 and L3 language courses: third and fourth year (fifth through eighth semester) advanced French language courses and second year (third and fourth semester) intermediate Spanish language courses. The average age of the participants was around 21 years and they reported learning their L1 from birth, the L2 from around the age of 7 (due to obligatory French in public schools), and the L3 from around the age of 17 (either in high school or university). To gather information about language use and proficiency level, the participants completed a language questionnaire. Table 1 reports key participant characteristics, including age of acquisition, self-ratings of fluency, and verbal fluency in all three languages. Paired t-tests revealed significant differences between the overall ratings for all three languages: L1 and L2, t(55) = 11.48, p < .001; L2 and L3, t(55) = 9.02, p < .001; and L1 and L3, t(55) = 20.95, p < .001.
Table 1. Means (SDs) of participant characteristics and self-ratings.

L1 = native language (English); L2 = second language (French); L3 = third language (Spanish)
Notes: Self-ratings were given on a ten-point Likert scale ranging from 1 (“not fluent”) to 10 (“very fluent”). Lexical robustness is indicated by the total number of category exemplars produced in the verbal fluency task. Scores for each language were computed as the sum across ten categories. Planned contrasts indicated significant differences in the lexical robustness scores between all three languages (all ps < .001). See Schwieter and Sunderman (Reference Schwieter and Sunderman2011) for further details.
Procedure
The participants were each tested individually and were given verbal instructions by the researchers in addition to the reading the instructions on the computer screen for the tasks. They first completed the informed consent form followed by the language questionnaire. All participants then completed a verbal fluency task, the details of which are presented elsewhere (see Schwieter & Sunderman, Reference Schwieter and Sunderman2008, Reference Schwieter and Sunderman2009, Reference Schwieter and Sunderman2011). In this task, participants were presented with a category (five semantic, five letter), and for each category they were instructed to produce as many exemplars as possible within sixty seconds. Categories were blocked by language to avoid language switching. The total number of unique category exemplars produced by the participant has been taken as an indicator of lexical robustness, an element of global language proficieny related to the familiarity and frequency of lexical items and the automaticity of retrieval (Costa et al., Reference Costa, Santesteban and Ivanova2006; Schwieter & Sunderman, Reference Schwieter and Sunderman2008, Reference Schwieter and Sunderman2009). The participants’ verbal fluency performance further suggests that they were L1-dominant trilinguals with higher proficiency in the L2 than in the L3 (see Table 1). Paired t-tests revealed significant differences between verbal fluency scores in each of the three languages: L1 and L2, t(55) = 17.10, p < .001; L2 and L3, t(55) = 11.59, p < .001; and L1 and L3, t(55) = 26.47, p < .001. Futhermore, correlation analyses found significant correlations between the lexical robustness scores and the self-ratings scores in both the L2, r(55) = .499, p < .001, and L3, r(55) = .484, p < .001. The proficiency self-ratings and verbal fluency scores suggest that participants had significantly different levels of dominance in each of their languages.
Finally, participants completed a picture-naming task and the Simon task. The specific experimental procedures for each of these tasks are described in the next section.
Picture-naming task
A number of studies have used a variant of the picture-naming task reported in the present study (e.g., Costa & Santesteban, Reference Costa and Santesteban2004; Schwieter & Sunderman, Reference Schwieter and Sunderman2008). However, the addition of a third response language was included to explore trilingual language processing and reliance on inhibitory control within the same experiment (see also Schwieter & Sunderman, Reference Schwieter and Sunderman2011). In this trilingual adaption of the task, ten standardized black and white line drawings (Snodgrass & Vanderwart, Reference Snodgrass and Vanderwart1980) of a pencil, house, car, dog, book, cat, chair, table, bear, and heart were presented individually on a computer screen in 60 lists ranging from 5–14 pictures in length.Footnote 2 Within the lists, each trial was classified as either a non-switch trial (the previous trial was named in the same language) or a switch trial (the previous trial was named in a different language). Each of the ten pictures appeared 60 times such that, of the 600 total trials, 420 (70%) were non-switch trials and 180 (30%) were switch trials. Switch trials were equally distributed across the six possible language pairings (e.g., L1-to-L2, L1-to-L3, etc.), resulting in 30 switches per type. Each list included anywhere from 0–4 switch trials, and for lists with 5–10 pictures in length, no picture appeared twice. For lists with 11–14 pictures, the repeated pictures were placed at least three trials away from their first presentation.
Each of the lists of pictures were structured as follows: (i) a fixation point (+) was presented in the center of the screen on a white background for 500 ms; (ii) the first picture appeared on either a blue, red, or yellow background for 2000 ms or until the participant responded into the microphone; (iii) a fixation point (+) appeared on a white background for 500 ms; and (iv) another picture (either a switch or non-switch trial) was shown, and the cycle was repeated until the end of the list, at which point an asterisk was presented at fixation for 1000 ms to provide a brief pause prior to beginning the next list. All responses were timed by the computer and recorded and coded for accuracy on a master key by the researchers.
Participants were instructed to name the pictures in one of their three languages as specified by the background color of the computer screen. The participants named the pictures in their L1 when presented on blue background screens, in their L2 on red background screens, and in their L3 on yellow background screens. There was equal production of all three languages in the experiment (i.e., 200 responses were elicited in each language). Before the experimental lists, participants practiced the task on six practice lists, with each list presenting each of the ten pictures at least twice in all three languages to familiarize the participants with the pictures prior to the experimental lists. A brief break was given between the practice and experimental lists and also at the completion of every 10 lists (approximately every five minutes) to avoid fatigue.
Simon task
We selected the Simon task as our measure of inhibitory control since it has recently been used in a number of bilingual studies (e.g., Bialystok et al., Reference Bialystok, Craik, Klein and Viswanathan2004; Bialystok, Craik, Grady, Chau, Ishii, Gunji & Pantev, Reference Bialystok, Craik, Grady, Chau, Ishii, Gunji and Pantev2005). Moreover, we wanted to avoid inhibitory control tasks involving linguistic interference – like the Stroop task (Stroop, Reference Stroop1935) – to better examine the role of domain-general rather than language-oriented inhibitory control. In the Simon task, a series of red and blue boxes was presented on screen in one of three locations (central, left of center, or right of center). Participants were instructed to respond with a left or right button press based on the color of the stimulus while ignoring the stimulus location. On congruent trials, the locations of the stimulus and the correct response matched (e.g., a box presented left of center requiring a left button press). On incongruent trials, the stimulus and response locations were mismatched, such that the stimulus was presented on the opposite side of the screen from the location of the correct response (e.g., a box presented left of center requiring a right button press). Neutral trials – where the stimulus was presented at the center of the screen – were also presented but were excluded from the analysis. Participants completed three blocks of 42 trials (14 each of congruent, neutral, and incongruent trials).
Response latencies are typically longer on incongruent trials than on congruent trials due to the mismatch between the stimulus and response locations (Simon & Rudell, Reference Simon and Rudell1967). The magnitude of this difference in RTs, termed the Simon effect, is interpreted as an indicator of an individual's ability to inhibit the prepotent tendency to respond based on the (task-irrelevant) location of the stimulus.
Data scoring and analysis
For the Simon task, the Simon effect is often computed as the difference between mean RTs on incongruent trials and mean RTs on congruent trials. However, preliminary analyses indicated that the standard Simon effect scores for this sample were not normally distributed. To correct for normality, trial-level RTs were first log-transformed, then the median log-RTs were computed for congruent and incongruent trials.Footnote 3 For the picture-naming task, inaccurate trials as well as trials with RTs faster than 250 ms or slower than 2500 ms were excluded from all analyses. RTs were then log-transformed prior to analysis to obtain a more normal distribution.
Picture-naming performance was analyzed using mixed effects modeling, which offers a number of benefits over Analysis of Variance (ANOVA). First, by-subject (F1) and by-item (F2) analyses can be combined within one analytic framework, thereby more appropriately modeling the data and allowing generalization of results to other people and items (e.g., Baayen, Davidson & Bates, Reference Baayen, Davidson and Bates2008). Second, the trial-level RT data can be analyzed without aggregation across conditions, as is necessary for ANOVAs. Third, mixed effects models allow an examination of predictors at multiple levels, as was the case with the predictors of interest in this study: the current language and previous language (L1, L2, or L3) were manipulated at the trial level, whereas inhibitory control was measured at the subject level. Mixed models were implemented using the lme4 package (Bates & Maechler, Reference Bates and Maechler2010) within the R statistical computing environment (R Development Core Team, 2010).
Model interpretation
When fitting mixed effects models with a dummy coded categorical variable, one level of that factor serves as the baseline condition against which all other levels of that factor are compared. For example, for these picture naming data, language of naming has three levels (L1, L2 and L3). If a model with only language and inhibitory control (IC) were fit to these data with L1 as the baseline condition, the intercept would estimate mean RT in the L1 condition, whereas the L2 and L3 parameters would estimate the difference between mean RT in L1 and mean RT in L2 or L3, respectively. In this example model, the IC effect estimates the magnitude and direction of the relationship between IC and naming in the L1 condition (i.e., the IC slope). The interpretation of a significant positive slope is that better inhibitory control (indicated by a smaller Simon effect) predicts faster picture naming (smaller RTs). The two-way interactions – L2 × IC, and L3 × IC – indicate whether the IC slope differs in the L2 and L3 (relative to the L1), and the interpretation of the parameters is the difference in the IC slope between L1 and L2 or L3 conditions, respectively. For example, a significant negative L2 × IC parameter indicates that the IC slope is significantly smaller (i.e., less steep) for L2 relative to L1. However, this does not indicate whether the IC slope differs from zero in the L2 condition. To conduct that hypothesis test, one refits the model with L2 as the baseline condition and then examines the significance test for the IC slope. Note that refitting the model does not affect the goodness of fit of the model or the type-I error rate, but rather it simply estimates the parameters with a different reference point (now, L2 naming) and modifies the parameters’ interpretations accordingly (Gelman & Hill, Reference Gelman and Hill2007).
For models with multiple categorical variables and higher level interactions, the model parameters are again interpreted in reference to the baseline level of each categorical variable. For example, a model with current language (baseline: L1) and prior language (baseline: L1), the intercept estimates the mean RT for L1 trials preceded by L1 trials (i.e., L1 non-switches). The prior language-L2 parameter indicates whether RTs were different when L1 naming was preceded by L2 naming, and is interpreted as the difference in mean RT between L1 non-switches and L2–L1 switches – that is, the L2-to-L1 switch cost. As such, a significant positive prior language-L2 parameter indicates that participants had significant L2-to-L1 switch costs. The IC parameter indicates whether the IC slope significantly predicts RTs on L1 non-switch trials; the interpretation of a positive IC parameter is that better inhibitory control (i.e., a smaller Simon effect) predicts faster L1 naming on non-switch trials. The IC × prior language-L2 interaction indicates whether inhibitory control predicts L2-to-L1 switch costs, and the interpretation of a positive interaction term is that better inhibitory control predicts smaller L2-to-L1 switch costs.
With L1 as the baseline condition for current language and prior language, to estimate the switch costs and IC slopes for the L2 (or L3), one must refit the models with L2 (or L3) as the baseline condition for both current language and prior language. The model parameters are then interpreted as described above. That is, to directly test the hypothesis that inhibitory control predicts switch costs in the L2 (or L3), one refits the model with L2 (or L3) as the baseline for both current language and prior language variables, then examines the significance tests for the parameters identified above.
For the two main analyses of this paper, different variable coding schemes were implemented to facilitate direct tests of the hypothesized effects. These coding differences are noted below with the corresponding model results.
Results
Language switching performance
Reaction times and percent accuracy for the picture-naming task are presented in Table 2. On average, participants were faster in their L1 than in their L2 or L3. Participants showed asymmetric switch costs across the three languages, with smaller switch costs in the L3 relative to the more dominant L1 or L2 – a pattern that replicates previous language switching results involving trilinguals who were highly proficient in their L1 and L2 but much less proficient in their L3 (e.g., Costa et al., Reference Costa, Santesteban and Ivanova2006).
Table 2. Correct reaction times (in ms) and accuracy (in percent) for the picture-naming task, by Language and Condition.

L1 = native language (English); L2 = second language (French); L3 = third language (Spanish)
Notes: Reaction times reported as geometric means to ease interpretation, but analyses were conducted on log-transformed RTs. Accuracy standard deviations reported in parentheses. Bold indicates magnitude of switch costs to naming latencies or accuracy.
These patterns were confirmed by the mixed model analysis (Table 3). For this model, L1 trials served as the baseline condition, such that L2 and L3 parameters indicate contrasts with the L1 condition. Switch and non-switch trials were coded with contrast coding (non-switch = –.5, switch = .5), so that the intercept estimates the mean log RT across both switch and non-switch conditions and the Switch Cost parameter estimates the switch cost magnitude in log RT. The significant Switch Cost parameter indicates that participants showed a reliable switch cost in L1 (the baseline language condition). At first glance, it appears that very similar switch costs were found in L1 and L2, based on the raw RTs reported in Table 2. However, on the log RT scale, switch costs were significantly larger in L1 than in either L2 or L3, as indicated by significant Switch Cost × L2 and Switch Cost × L3 parameters, respectively.
Table 3. Estimated coefficients from mixed effects model of switch costs.

L1 = native language (English); L2 = second language (French); L3 = third language (Spanish); SE = standard error of parameter estimate; * p < .05
Notes: The table lists maximum likelihood estimates. Current trial switch condition was contrast-coded (.5 = switch trial; –.5 = non-switch trial), making the coefficient interpretations as follows: intercept = estimated mean log-RT on L1 trials for a participant with the sample-average inhibitory control abilities; Switch Cost = magnitude of switch cost; IC = slope for inhibitory control (magnitude of Simon effect, in log-RT), centered at the sample mean to ease interpretation of the other model parameters (Raudenbush & Bryk, Reference Raudenbush and Bryk2002); t-ratio = Coefficient/SE, with t-ratio values over 2.0 indicating the coefficient is significantly different from zero (Gelman & Hill, Reference Gelman and Hill2007). Bold indicates coefficients that are significantly different from zero.
Does inhibitory control predict switch costs?
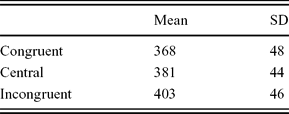
Mean raw RTs from the Simon task are reported for the congruent, incongruent, and central conditions in Table 4. In order to address the primary question of interest in this study, Inhibitory Control was also included as a simple effect and in interaction terms in the mixed effects model reported in Table 3. For L3 naming only, better inhibitory control predicted faster RTs in general (i.e., across switch and non-switch trials), as indicated by the significant IC × L3 interaction term. Critically, better inhibitory control abilities predicted smaller switch costs above and beyond this general effect, but only in the L1 (see Figure 1). The significant and positive Switch Cost ×IC parameter indicates that, for the L1, better inhibitory control was related to smaller switch costs. The two negative three-way interaction terms indicate that the inhibitory control effect was smaller in the L2 and L3. In order to directly test whether inhibitory control predicted switch costs in the L2 or L3, the model was refit first with L2 as the baseline condition, then with L3 as the baseline condition, in order to estimate the Switch Cost × IC parameter for these languages directly. Neither parameter was significantly different from zero (L2: t = –1.06, ns; L3: t = 1.3, ns). These results suggest that better inhibitory control predicts smaller switch costs, but only when switching into the L1.
Table 4. Simon task raw reaction times by condition.
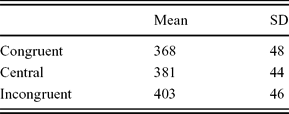
Note: Each participant's Simon effect score was computed by log-transforming RTs, calculating the median log-RT for each condition, then taking the difference in median log-RTs for the incongruent and congruent conditions, in order to normalize the distribution of scores (see text).

Figure 1. Relationship between inhibitory control and switch costs.
Prior language effects
The above analysis examined switch costs in the typical manner implemented in language switching studies, based solely on the language being switched into. However, doing so ignores the language being switched from – a factor that is likely to impact performance but has not yet been examined in the literature, as briefly mentioned in the introduction. Indeed, the unbalanced proficiency levels of the participants may be obfuscating the role of inhibitory control in the L2 and L3, where the participants were presumably most likely to experience cross-language interference and therefore potentially needed to engage inhibitory control (e.g., Green, Reference Green1998). Thus, we conducted an exploratory post-hoc analysis to directly address this question. In this analysis, the Switch Cost variable was replaced with a variable coding for the previous trial's language of naming (Prior Language). The purpose of this exploratory analysis was two-fold. First, we aimed to directly test whether prior language affects switching performance. Second and more directly relevant to the main research question of this study, we wanted to examine whether the inhibitory control effects reported above would still hold after accounting for the prior language in our model.
Mean RTs for the nine conditions suggest that the prior language indeed affected the magnitude of switch costs (see Table 5). Specifically, L1 switch costs appear to be larger when switching from the L2. Switch costs in the L2 were larger when switching from the less dominant L3 (107 ms, on average) than from the dominant L1 (32 ms, on average), whereas L3 switch costs were larger when switching from the L2 than the dominant L1.
Table 5. Correct reaction times (in ms) and accuracy (in percent) for the picture-naming task, by preceding trial and current trial language.

L1 = native language (English); L2 = second language (French); L3 = third language (Spanish)
Notes: Reaction times reported as geometric means to ease interpretation, but analyses were conducted on log-transformed RTs. Accuracy standard deviations reported in parentheses.
But does the effect of inhibitory control on switch costs depend on the prior language? For the analysis, Prior Language and Current Langauge were both dummy coded with L1 as the baseline condition. Note that, due to the increased complexity of the model, the interpretation of model parameters becomes much less straightforward than in the previous analysis. The intercept now represents mean log RT on L1 non-switch trials (Prior Language baseline = L1, Current Language baseline = L1), and the Prior Language and Current Language parameters represent contrasts between different conditions. For example, the Prior L2 parameter estimates the difference between L1 non-switch and L2-to-L1 switch conditions; the Prior L2 × Current L3 parameter estimates the difference between L2-to-L1 switch and L2-to-L3 switch conditions).
Thus, to ease the interpretation of the model, we first begin with a visual inspection of the data (Figure 2), which suggests that the inhibitory control and prior language effects vary for each language. In the L1, inhibitory control seems to predict switch costs similarly for both prior languages. In the L2 and L3, inhibitory control only appears to predict switch costs when switching from the L1. Curiously, the direction of the relationship differs for the L2 and L3: better inhibitory control appears to predict smaller switch costs when switching from the L1 into the L2 but larger switch costs when switching from the L1 into the L3.

Figure 2. Relationship between inhibitory control and switch costs, conditional on the language of naming on the previous trial.
These patterns were largely supported by the mixed effects model (see Table 6). These results indicate that better inhibitory control significantly predicted smaller switch costs when switching into the L1 from the L2 (Prior L2 × IC effect) and from the L3 (Prior L3 × IC effect). Note that inhibitory control did not predict RTs on L1 non-switch trials – a dissociation we return to in the discussion.
Table 6. Estimated coefficients from mixed effects model of prior language effects.

L1 = native language (English); L2 = second language (French); L3 = third language (Spanish); * p < .05
Notes: The table lists maximum likelihood estimates. Prior trial language and current trial language were both dummy coded with L1 as the baseline condition. Thus, Intercept = estimated mean log-RT on L1 non-switch trials for a participant with the sample-average inhibitory control abilities; IC = slope for inhibitory control (magnitude of Simon effect, in log-RT), centered at the sample mean to ease interpretation of the other model parameters (Raudenbush & Bryk, Reference Raudenbush and Bryk2002). See text for details on interpretation of critical interaction parameters. t-ratio = coefficient/SE, with t-ratio values over 2.0 indicating the coefficient is significantly different from zero (Gelman & Hill, Reference Gelman and Hill2007). Bold indicates coefficients that are significantly different from zero.
To directly examine the IC slopes in L2 and L3, the model was refit first with L2 as the baseline condition for Prior Language and Current Language, then with L3 as the baseline for both factors. For the L2, inhibitory control did not significantly predict RTs on non-switch trials, t = 1.65, ns. Furthermore, inhibitory control did not predict switch costs from the L3 (t = 0.06, ns) or the L1 (t = –1.69, ns). For the L3, better inhibitory control significantly predicted faster RTs on non-switch trials (parameter = 0.65, t = 2.15, p < .05). Critically, inhibitory control predicted switch costs when switching from the L1 (t = 2.60, p < .05) but not from the L2 (t = –0.64, ns).
Discussion
The present study was designed to examine the relationship between domain-general inhibitory control abilities and performance during a multilingual language switching task. Two hypotheses were tested: (1) inhibitory control should predict switch costs in general, and (2) this relationship should be strongest when switching into the L1. A series of analyses provided clear support for the second hypothesis: in a traditional analysis of switch costs (ignoring the language of naming on the previous trial), inhibitory control was related to the magnitude of switch costs for the L1 only, with better inhibitory control predicting smaller L1 switch costs. This pattern was replicated in the post-hoc analysis that included prior language, with a similar magnitude inhibitory control effect on L1 switching when switching from L2 and L3. These results support our second hypothesis that efficient inhibitory control abilities are most critical when switching into the more dominant L1 – when there should be the greatest amount of cross-language competition (e.g., Green, Reference Green1998).
The examination of L2 and L3 switch costs produced a more nuanced picture. In the traditional analysis, inhibitory control had no reliable relationship with switch costs in either the L2 or L3. Based on this analysis alone, one might be tempted to conclude that better domain-general inhibitory control does not facilitate switching into non-native languages. However, when taking into account the language of naming on the immediately preceding trial, inhibitory control effects emerged for L3 switch costs, but only when switching from the L1. Specifically, better inhibitory control predicted smaller switch costs. This L3 effect was in the expected direction, since naming in the L3 is precisely the condition when these participants were likely to experience the greatest amounts of cross-language competition – and particularly from the dominant L1. This pattern of results supports our hypothesis that the language task schemas apply inhibition to competing representations in a language-specific manner. Specifically, we hypothesized that a given task schema inhibits each non-target language independently, such that there may be greater reliance on inhibitory control for particular languages. By this account, the L3 naming task schema more strongly inhibited the L1 than the L2. Greater reliance on inhibitory control during L1-to-L3 switches provided more opportunity for individual differences in inhibitory control abilities to impact switching performance relative to L2-to-L3 switches. These results provide constraints on theories of language control that are unavailable from studies focusing solely on bilingual language switching.
It is important to note that inhibitory control was related to naming latencies overall in the L3. This finding contrasts with emerging results in the literature on the cognitive benefits of bilingualism. In a number of studies, bilinguals have been found to outperform monolinguals on a range of conflict resolution tasks, but these effects have been found in both conflict and non-conflict conditions. The working hypothesis is that bilingualism incurs benefits to the executive functioning system (not inhibitory control in particular), thus leading to an overall benefit for bilinguals when performing even in non-conflict conditions. In this sample of unbalanced trilinguals, differences in inhibitory control are most important in a conflict condition – specificially, when naming in the less dominant L3. This finding is congruent with competition-for-selection models of speech production (e.g., Kroll, Bobb & Wodniecka, Reference Kroll, Bobb and Wodniecka2006). According to these models, the greatest amount of cross-language competition should occur when naming in a less dominant language. For these participants, the L3 is much less dominant than both the L1 and L2, and therefore we would expect the greatest amount of competition to be when naming in the L3. The fact that better inhibitory control predicted faster RTs when naming in the L3 supports claims that inhibitory processes may serve as one method for reducing this cross-language competition (e.g., Kroll et al., Reference Kroll, Bobb, Misra and Guo2008) and in particular for relatively weak languages (Schwieter & Sunderman, Reference Schwieter and Sunderman2008). Future studies might consider examining inhibitory control alongside other executive functions (e.g., task switching, working memory updating) to determine their relative contributions to multilingual language switching.
Our post-hoc analysis found reliable prior language effects in line with theoretical considerations. These results constrain the benefits of better inhibitory control to conditions involving the dominant L1 only. The robust inhibitory control–L1 switch cost effects suggest that inhibitory control is clearly relevant when re-engaging the L1 after having previously abandoned it. This finding is congruent with recent findings of asymmetric n–2 repetition costs that were largest in the L1, which has been interpreted as direct evidence of the impact of lingering inhibition, particularly on the dominant L1 (e.g., Koch et al., Reference Koch, Gade, Schuch and Philipp2010; Philipp et al., Reference Philipp, Gade and Koch2007). However, Koch and colleagues argued that persisting activation of a non-dominant language, and not inhibition (as measured by the n–2 repetition cost), was most responsible for the switch cost asymmetry in their study. Our findings are in direct contrast with this claim, as better inhibitory control in fact predicted smaller switch costs in the L1 but not in the L2, and only when switching into the L3 from the L1. These effects on L1 switches were robust over both prior language conditions. That is, the effects of inhibitory control were constrained to conditions in which there was a large discrepancy in language dominance. However, we measured inhibition with an independent, domain-general measure of inhibitory control, whereas the n–2 repetition effect could be seen as a domain-specific inhibitory mechanism. More research is needed to further disentangle the relative contributions of persisting activation, domain-specific inhibition (e.g., n–2 repetition costs), and domain-general inhibition.
Based on inhibitory accounts of the switch cost asymmetry (e.g., Meuter & Allport, Reference Meuter and Allport1999), one might expect ‘better inhibitors’ to deploy more inhibition to the L1 during L3 naming, thereby inducing larger L1 switch costs due to the increase in lingering L1 inhibition. But better inhibitory control does not necessarily imply more inhibition of competitors. Rather, better inhibitors may more rapidly deploy inhibition to L1 lemmas during L3 naming, thereby limiting the activation of these L1 competitors. By this account, better inhibitors may therefore require less inhibition of L1 competitors to support successful L3 naming. In contrast, slower deployment of inhibition allows the L1 competitors to become more highly activated, and thus selection of the target lemma would require greater inhibition of these L1 competitors. This mechanism could be incorporated into the Inhibitory Control Model (Green, Reference Green1998) to account for individual differences in inhibitory control abilities: naming in less dominant languages requires more inhibition than naming in the dominant language, but the amount of inhibition required to support non-dominant language naming is reduced in a more efficient inhibitory control system. Specifically, this extension of Green's Inhibitory Control Model would predict that more efficient inhibitory control is related to (i) smaller costs to switching from the L1 to a less dominant non-native language (due to more rapid deployment of L1 inhibition when switching out of the L1), and (ii) smaller costs to switching from the less dominant non-native language to the dominant L1 (due to the reduction in the amount of lingering L1 inhibition from the previous trial). In the present study, both of these patterns were observed when participants switched between the dominant L1 and the much less proficient L3.
Individual differences in inhibitory control did not account for switching from L2 to L3, despite the fact that these participants were clearly more dominant in the L2 than the L3. For these participants, the L2 may have still required effortful processing relative to the automatized L1. Or they may not have developed a sufficient level of fluency in the L2 to require inhibitory processes to support the disengagement of the L2. In any case, both accounts are congruent with competition-for-selection models, if one assumes that activation of the L2 did not reach a threshold to induce enough cross-language competition to require inhibitory processes to help resolve that competition. Although domain-general inhibitory control abilities did not account for these switch costs, switching into the L2 from the L3 clearly induced a cost, which numerically was larger than when switching from the more dominant L1. Language switching is likely supported by multiple control mechanisms. Indeed, Prior and Gollan (Reference Prior and Gollan2011) recently found that bilinguals with smaller language switching costs also had smaller switch costs in a non-linguistic task switching paradigm, suggesting that both switching tasks rely on similar control mechanisms. These different mechanisms – including different types of inhibitory control processes (e.g., Friedman & Miyake, Reference Friedman and Miyake2004) as well as other executive functions (e.g., task switching, working memory updating) – may differentially contribute to language switching performance based on a number of factors, such as the relative dominance or automaticity of the languages. This is clearly a ripe area for continued research into the role of executive functions in supporting language control.
These results provide a direct demonstration that more efficient domain-general inhibitory control ability is related to smaller switch costs when switching between languages. This benefit was constrained to switching into the dominant L1 and switching out of the dominant L1 into a much weaker L3. Festman et al. (Reference Festman, Rodríguez-Fornells and Münte2010) approached this question from a different perspective: they identified participants as having high or low language control ability (non-switchers and switchers, respectively) based on the number of language errors committed during a picture naming task, then compared these groups on a range of tasks measuring executive functions and intelligence. Their study provided a key first piece of evidence that better language control is related to executive functions. The current study provides a more fine-grained analysis of this relationship by linking inhibitory control abilities to individual differences in online lexical access during speech production.
Conclusions
This study examined the relationship between domain-general inhibitory control and online language processing during a language switching task. Taking an individual differences approach, these results provide evidence of a direct link between inhibitory control abilities and language switching capabilities – particularly when switching into the more dominant L1 or when switching from the L1 into a much less dominant L3. However, inhibitory control showed no relationship when switching into the relatively proficient L2, suggesting important constraints on the conditions under which domain-general inhibitory control abilities are related to language switching. This research takes an important step towards directly mapping executive functions to particular aspects of online language processing performance. This line of work promises to advance theories of bilingual cognitive control and further elucidates the precise conditions under which executive functions are and are not called upon to guide behavior.