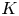1. Introduction
Throughout the article, the ground field is assumed to be the field of complex numbers. Let $S$![]() be a codimension $c$
be a codimension $c$![]() complete intersection of type $(d_1,\, \ldots,\, d_c)$
complete intersection of type $(d_1,\, \ldots,\, d_c)$![]() in a weighted projective space $\mathbb {P}(a_0,\, \ldots,\, a_n)$
in a weighted projective space $\mathbb {P}(a_0,\, \ldots,\, a_n)$![]() that is quasi-smooth, well-formed and $a_0\leq a_1\leq \cdots \leq a_n < d_1\leq \cdots \leq d_c$
that is quasi-smooth, well-formed and $a_0\leq a_1\leq \cdots \leq a_n < d_1\leq \cdots \leq d_c$![]() . Suppose that $S$
. Suppose that $S$![]() is a log del Pezzo surface. Then we have exactly two possibilities:
is a log del Pezzo surface. Then we have exactly two possibilities:
(A) Either $n=3$
 and $S\subset \mathbb {P}(a_0,\,a_1,\,a_2,\,a_3)$
and $S\subset \mathbb {P}(a_0,\,a_1,\,a_2,\,a_3)$ is a hypersurface of degree
\[ d< a_0+a_1+a_2+a_3 \]with amplitude $I=a_0+a_1+a_2+a_3-d$
is a hypersurface of degree
\[ d< a_0+a_1+a_2+a_3 \]with amplitude $I=a_0+a_1+a_2+a_3-d$

(B) Or $n=4$
 and $S\subset \mathbb {P}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$
and $S\subset \mathbb {P}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$ is a complete intersection of two hypersurfaces of degrees $d_1$
is a complete intersection of two hypersurfaces of degrees $d_1$ and $d_2$
and $d_2$ such that
\[ d_1+d_2< a_0+a_1+a_2+a_3+a_4 \]with amplitude $I=a_0+a_1+a_2+a_3+a_4-d_1-d_2$
such that
\[ d_1+d_2< a_0+a_1+a_2+a_3+a_4 \]with amplitude $I=a_0+a_1+a_2+a_3+a_4-d_1-d_2$
 .
.
In the case (A), Johnson and Kollár [Reference Johnson and Kollár9] found the complete list of all possibilities for the quintuple $(a_0,\,a_1,\,a_2,\,a_3,\,d)$![]() in the case when the amplitude $I$
in the case when the amplitude $I$![]() is one. Moreover, they computed the $\alpha$
is one. Moreover, they computed the $\alpha$![]() -invariants and proved the existence of the orbifold Kähler–Einstein metrics in the case when the quintuple $(a_0,\, a_1,\, a_2,\, a_3,\, d)$
-invariants and proved the existence of the orbifold Kähler–Einstein metrics in the case when the quintuple $(a_0,\, a_1,\, a_2,\, a_3,\, d)$![]() is not one of the following four quintuples
is not one of the following four quintuples
To prove the above statement they used the criterion that a log del Pezzo surface $S$![]() admits an orbifold Kähler–Einstein metric whenever the $\alpha$
admits an orbifold Kähler–Einstein metric whenever the $\alpha$![]() -invariant of $S$
-invariant of $S$![]() is bigger than $\frac {2}{3}$
is bigger than $\frac {2}{3}$![]() . Later, Araujo [Reference Araujo1] computed the $\alpha$
. Later, Araujo [Reference Araujo1] computed the $\alpha$![]() -invariants for two of these four cases to show the existence of an orbifold Kähler–Einstein metric when $(a_0,\, a_1,\, a_2,\, a_3,\, d) = (1,\, 2,\, 3,\, 5,\, 10)$
-invariants for two of these four cases to show the existence of an orbifold Kähler–Einstein metric when $(a_0,\, a_1,\, a_2,\, a_3,\, d) = (1,\, 2,\, 3,\, 5,\, 10)$![]() or $(a_0,\, a_1,\, a_2,\, a_3,\, d) = (1,\, 3,\, 5,\, 7,\, 15)$
or $(a_0,\, a_1,\, a_2,\, a_3,\, d) = (1,\, 3,\, 5,\, 7,\, 15)$![]() and the defining equation contains the monomial $yzt$
and the defining equation contains the monomial $yzt$![]() where $x$
where $x$![]() , $y$
, $y$![]() , $z$
, $z$![]() and $t$
and $t$![]() are coordinates with weights $\operatorname {wt}(x) = a_0$
are coordinates with weights $\operatorname {wt}(x) = a_0$![]() , $\operatorname {wt}(y) = a_1$
, $\operatorname {wt}(y) = a_1$![]() , $\operatorname {wt}(z) = a_2$
, $\operatorname {wt}(z) = a_2$![]() and $\operatorname {wt}(t) = a_3$
and $\operatorname {wt}(t) = a_3$![]() . Finally, Cheltsov, Park and Shramov [Reference Cheltsov, Park and Shramov2] computed the $\alpha$
. Finally, Cheltsov, Park and Shramov [Reference Cheltsov, Park and Shramov2] computed the $\alpha$![]() -invariants for the remaining families.
-invariants for the remaining families.
For the case (A) every log del Pezzo surface $S$![]() admits an orbifold Kähler–Einstein metric except possibly the case when $(a_0,\,a_1,\,a_2,\,a_3,\,d) = (1,\,3,\,5,\,7,\,15)$
admits an orbifold Kähler–Einstein metric except possibly the case when $(a_0,\,a_1,\,a_2,\,a_3,\,d) = (1,\,3,\,5,\,7,\,15)$![]() and the defining equation does not contain the monomial $yzt$
and the defining equation does not contain the monomial $yzt$![]() whose $\alpha$
whose $\alpha$![]() -invariant is $\frac {8}{15}(<\tfrac {2}{3})$
-invariant is $\frac {8}{15}(<\tfrac {2}{3})$![]() .
.
Recently Fujita and Odaka introduced $\delta$![]() -invariant which gives a strong criterion showing the uniform $K$
-invariant which gives a strong criterion showing the uniform $K$![]() -stability of ${{\mathbb {Q}}}$
-stability of ${{\mathbb {Q}}}$![]() -Fano varieties (see [Reference Fujita and Odaka8]).
-Fano varieties (see [Reference Fujita and Odaka8]).
Theorem 1.1 Let $X$![]() be a $\mathbb {Q}$
be a $\mathbb {Q}$![]() -Fano variety. Then X is uniformly $K$
-Fano variety. Then X is uniformly $K$![]() -stable if and only if $\delta (X) > 1$
-stable if and only if $\delta (X) > 1$![]() .
.
The estimation of the $\delta$![]() -invariant has been investigated on several log del Pezzo surfaces in [Reference Cheltsov, Rubinstein and Zhang4–Reference Fujita, Liu, Süß, Zhang and Zhuang7, Reference Park and Won14, Reference Park and Won15]. Moreover Li, Tian and Wang generalized in [Reference Li, Tian and Wang13] the result of Chen, Donaldson, Sun and Tian for the $K$
-invariant has been investigated on several log del Pezzo surfaces in [Reference Cheltsov, Rubinstein and Zhang4–Reference Fujita, Liu, Süß, Zhang and Zhuang7, Reference Park and Won14, Reference Park and Won15]. Moreover Li, Tian and Wang generalized in [Reference Li, Tian and Wang13] the result of Chen, Donaldson, Sun and Tian for the $K$![]() -polystability and the existence of the Kähler–Einstein metric to some singular Fano varieties. In virtue of the $\delta$
-polystability and the existence of the Kähler–Einstein metric to some singular Fano varieties. In virtue of the $\delta$![]() -invariant method and the result [Reference Li, Tian and Wang13], the paper [Reference Cheltsov, Park and Shramov3] completes the problem of the existence of the (orbifold) Kähler–Einstein metric on del Pezzo hypersurfaces with $I=1$
-invariant method and the result [Reference Li, Tian and Wang13], the paper [Reference Cheltsov, Park and Shramov3] completes the problem of the existence of the (orbifold) Kähler–Einstein metric on del Pezzo hypersurfaces with $I=1$![]() , case (A):
, case (A):
Theorem 1.2 [Reference Cheltsov, Park and Shramov3]
Let $S$![]() be a quasi-smooth hypersurface in ${{\mathbb {P}}}(1,\,3,\,5,\,7)$
be a quasi-smooth hypersurface in ${{\mathbb {P}}}(1,\,3,\,5,\,7)$![]() of degree $15$
of degree $15$![]() such that its defining equation does not contain $yzt$
such that its defining equation does not contain $yzt$![]() . Then the surface $S$
. Then the surface $S$![]() admits an orbifold Kähler–Einstein metric.
admits an orbifold Kähler–Einstein metric.
Corollary 1.3 Every quasi-smooth hypersurface with $I=1$![]() admits an orbifold Kähler-Einstein metric.
admits an orbifold Kähler-Einstein metric.
In [Reference Kim and Park10] and [Reference Kim and Won11], we classified the log del Pezzo surfaces $S$![]() for the case (B) when $S\subset {{\mathbb {P}}}(a_0,\, a_1,\, a_2,\, a_3,\, a_4)$
for the case (B) when $S\subset {{\mathbb {P}}}(a_0,\, a_1,\, a_2,\, a_3,\, a_4)$![]() are quasi-smooth and well-formed complete intersection log del Pezzo surfaces given by two quasi-homogeneous polynomials of degrees $d_1$
are quasi-smooth and well-formed complete intersection log del Pezzo surfaces given by two quasi-homogeneous polynomials of degrees $d_1$![]() and $d_2$
and $d_2$![]() with amplitude $1$
with amplitude $1$![]() , and not being the intersection of a linear cone with another hypersurface. Then there are 42 families. We denote family No. $i$
, and not being the intersection of a linear cone with another hypersurface. Then there are 42 families. We denote family No. $i$![]() as the number $i$
as the number $i$![]() in the first column $\Gamma$
in the first column $\Gamma$![]() of the table which is represented in [Reference Kim and Won11, section 5].
of the table which is represented in [Reference Kim and Won11, section 5].
Suppose that the log del Pezzo surface $S$![]() is not one of the following:
is not one of the following:
• No. 3 : a complete intersection of two hypersurfaces of degrees $6$
 and $8$
and $8$ embedded in ${{\mathbb {P}}}(1,\,2,\,3,\,4,\,5)$
embedded in ${{\mathbb {P}}}(1,\,2,\,3,\,4,\,5)$ such that the defining equation of the hypersurface of degree 6 does not contain the monomial $yt$
such that the defining equation of the hypersurface of degree 6 does not contain the monomial $yt$ , where $y$
, where $y$ is the coordinate function of weight $2$
is the coordinate function of weight $2$ and $t$
and $t$ is the coordinate function of weight $4$
is the coordinate function of weight $4$ .
.• No. 40 : a complete intersection of two hypersurfaces of degree $2n$
 embedded in ${{\mathbb {P}}}(1 ,\,1,\, n,\, n,\, 2n-1)$
embedded in ${{\mathbb {P}}}(1 ,\,1,\, n,\, n,\, 2n-1)$ where $n$
where $n$ is a positive integer.
is a positive integer.
Then the $\alpha$![]() -invariant of $S$
-invariant of $S$![]() is bigger than $\tfrac {2}{3}$
is bigger than $\tfrac {2}{3}$![]() , in fact they are bigger or equal to one, so that it admits an orbifold Kähler–Einstein metric (see [Reference Kim and Park10, theorem 1.9] and [Reference Kim and Won11, theorem 1.2]).
, in fact they are bigger or equal to one, so that it admits an orbifold Kähler–Einstein metric (see [Reference Kim and Park10, theorem 1.9] and [Reference Kim and Won11, theorem 1.2]).
The present article completes the existence of the orbifold Kähler–Einstein metric of the remaining two cases.
Theorem 1.4 Let $S$![]() be a quasi-smooth member of family No. $i$
be a quasi-smooth member of family No. $i$![]() with $i\in \{3,\,40\}$
with $i\in \{3,\,40\}$![]() . Then the log del Pezzo surface $S$
. Then the log del Pezzo surface $S$![]() is uniformly $K$
is uniformly $K$![]() -stable so that it admits an orbifold Kähler–Einstein metric.
-stable so that it admits an orbifold Kähler–Einstein metric.
Corollary 1.5 Every quasi-smooth weighted complete intersection with $I=1$![]() admits an orbifold Kähler–Einstein metric.
admits an orbifold Kähler–Einstein metric.
2. Preliminary
2.1 Notation
Throughout the paper we use the following notations:
• For positive integers $a_0$
 , $a_1$
, $a_1$ , $a_2$
, $a_2$ , $a_3$
, $a_3$ and $a_4$
and $a_4$ , ${{\mathbb {P}}}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$
, ${{\mathbb {P}}}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$ is the weighted projective space. We assume that $a_0\leq a_1\leq a_2\leq a_3\leq a_4$
is the weighted projective space. We assume that $a_0\leq a_1\leq a_2\leq a_3\leq a_4$ .
.• We usually write $x$
 , $y$
, $y$ , $z$
, $z$ , $t$
, $t$ and $w$
and $w$ for the weighted homogeneous coordinates of ${{\mathbb {P}}}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$
for the weighted homogeneous coordinates of ${{\mathbb {P}}}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$ with weights $\operatorname {wt}(x)=a_0$
with weights $\operatorname {wt}(x)=a_0$ , $\operatorname {wt}(y)=a_1$
, $\operatorname {wt}(y)=a_1$ , $\operatorname {wt}(z)=a_2$
, $\operatorname {wt}(z)=a_2$ , ${\operatorname {wt}(t)=a_3}$
, ${\operatorname {wt}(t)=a_3}$ and $\operatorname {wt}(w)=a_4$
and $\operatorname {wt}(w)=a_4$ .
.• $S\subset {{\mathbb {P}}}(a_0,\,a_1,\,a_2,\,a_3,\,a_4)$
 denotes a quasi-smooth complete intersection log del Pezzo surface given by quasi-homogeneous polynomials of degrees $d_1$
denotes a quasi-smooth complete intersection log del Pezzo surface given by quasi-homogeneous polynomials of degrees $d_1$ and $d_2$
and $d_2$ .
.• The integer $I = a_0 + a_1 + a_2 + a_3 + a_4 - d_1 - d_2$
 is called the amplitude of $S$
is called the amplitude of $S$ .
.• $H_{*}$
 is the hyperplane section on the log del Pezzo surface $S$
is the hyperplane section on the log del Pezzo surface $S$ cut out by the equation $* = 0$
cut out by the equation $* = 0$ .
.• $\mathsf p_x$
 denotes the point on $S$
denotes the point on $S$ given by $y=z=t=w=0$
given by $y=z=t=w=0$ . The points $\mathsf p_y$
. The points $\mathsf p_y$ , $\mathsf p_z$
, $\mathsf p_z$ , $\mathsf p_t$
, $\mathsf p_t$ and $\mathsf p_w$
and $\mathsf p_w$ are defined in a similar way.
are defined in a similar way.• $-K_S$
 denotes the anti-canonical divisor of $S$
denotes the anti-canonical divisor of $S$ .
.
2.2 Foundation
$X$![]() is ${{\mathbb {Q}}}$
is ${{\mathbb {Q}}}$![]() -Fano variety, i.e., a normal projective ${{\mathbb {Q}}}$
-Fano variety, i.e., a normal projective ${{\mathbb {Q}}}$![]() -factorial variety with at most terminal singularities such that $-K_X$
-factorial variety with at most terminal singularities such that $-K_X$![]() is ample.
is ample.
Definition 2.1 Let $(X,\,D)$![]() be a pair, that is, $D$
be a pair, that is, $D$![]() is an effective ${{\mathbb {Q}}}$
is an effective ${{\mathbb {Q}}}$![]() -divisor, and let $\mathsf p \in X$
-divisor, and let $\mathsf p \in X$![]() be a point. We define the log canonical threshold (LCT, for short) of $(X,\,D)$
be a point. We define the log canonical threshold (LCT, for short) of $(X,\,D)$![]() and the log canonical threshold of $(X,\,D)$
and the log canonical threshold of $(X,\,D)$![]() at $\mathsf p$
at $\mathsf p$![]() to be the numbers
to be the numbers
respectively. We define
and for a subset $\Sigma \subset X$![]() , we define
, we define
The number $\alpha (X) := \operatorname {lct}_X (X)$![]() is called the global log canonical threshold (GLCT, for short) or the $\alpha$
is called the global log canonical threshold (GLCT, for short) or the $\alpha$![]() -invariant of $X$
-invariant of $X$![]()
Let $S$![]() be a surface with at most cyclic quotient singularities, and let $D$
be a surface with at most cyclic quotient singularities, and let $D$![]() be an effective ${{\mathbb {Q}}}$
be an effective ${{\mathbb {Q}}}$![]() -divisor on $X$
-divisor on $X$![]() .
.
Lemma 2.2 [Reference Kollár12]
Let $\mathsf p$![]() be a smooth point of $S$
be a smooth point of $S$![]() . Suppose that the log pair $(S,\, D)$
. Suppose that the log pair $(S,\, D)$![]() is not log canonical at the point $\mathsf p$
is not log canonical at the point $\mathsf p$![]() . Then $\operatorname {mult}_{\mathsf p}(D) > 1$
. Then $\operatorname {mult}_{\mathsf p}(D) > 1$![]() .
.
Suppose that $S$![]() has a cyclic quotient singular point $\mathsf q$
has a cyclic quotient singular point $\mathsf q$![]() of type $\frac {1}{r}(a,\,b)$
of type $\frac {1}{r}(a,\,b)$![]() . Then there is an orbifold chart $\pi \colon \bar {U}\to U$
. Then there is an orbifold chart $\pi \colon \bar {U}\to U$![]() for some open set $\mathsf q\in U$
for some open set $\mathsf q\in U$![]() on $S$
on $S$![]() such that $\bar {U}$
such that $\bar {U}$![]() is smooth and $\pi$
is smooth and $\pi$![]() is a cyclic cover of degree $r$
is a cyclic cover of degree $r$![]() branched over $\mathsf q$
branched over $\mathsf q$![]() .
.
Lemma 2.3 [Reference Kollár12]
Let $\bar {\mathsf q}\in \bar {U}$![]() be the point such that $\pi (\bar {\mathsf q}) = \mathsf q$
be the point such that $\pi (\bar {\mathsf q}) = \mathsf q$![]() . Then the log pair $(U,\, D|_U)$
. Then the log pair $(U,\, D|_U)$![]() is log canonical at the point $\mathsf q$
is log canonical at the point $\mathsf q$![]() if and only if the log pair $(\bar {U},\, \bar {D}|_{\bar {U}})$
if and only if the log pair $(\bar {U},\, \bar {D}|_{\bar {U}})$![]() is log canonical at the point $\bar {\mathsf q}$
is log canonical at the point $\bar {\mathsf q}$![]() where $\bar {D}=\pi ^{*}(D|_{U})$
where $\bar {D}=\pi ^{*}(D|_{U})$![]() .
.
Definition 2.4 [Reference Fujita and Odaka8]
Let $k$![]() be a positive integer. We set $h=h^{0}(S,\,-kK_S)$
be a positive integer. We set $h=h^{0}(S,\,-kK_S)$![]() . Given any basis
. Given any basis
of $H^{0}(S,\,-kK_S)$![]() , taking the corresponding divisors $D_1,\,\ldots,\,D_h$
, taking the corresponding divisors $D_1,\,\ldots,\,D_h$![]() with $D_i\sim -kK_S$
with $D_i\sim -kK_S$![]() , we get an anti-canonical ${{\mathbb {Q}}}$
, we get an anti-canonical ${{\mathbb {Q}}}$![]() -divisor
-divisor
We call this kind of anti-canonical ${{\mathbb {Q}}}$![]() -divisor an anti-canonical ${{\mathbb {Q}}}$
-divisor an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type.
-basis type.
Then we can define the $\delta$![]() -invariant of $S$
-invariant of $S$![]() using an anti-canonical ${{\mathbb {Q}}}$
using an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type. The definition of the $\delta$
-basis type. The definition of the $\delta$![]() -invariant of a Fano variety is the following.
-invariant of a Fano variety is the following.
Definition 2.5 [Reference Fujita and Odaka8]
For $k\in {{\mathbb {Z}}}_{>0}$![]() , set
, set
Moreover, we define
It is called the $\delta$![]() -invariant of $S$
-invariant of $S$![]() .
.
Definition 2.6 Let $X$![]() be an irreducible projective variety of dimension $n$
be an irreducible projective variety of dimension $n$![]() , and let $D$
, and let $D$![]() be a Cartier divisor on X. The volume of $D$
be a Cartier divisor on X. The volume of $D$![]() is defined to be the non-negative real number
is defined to be the non-negative real number
For a ${{\mathbb {Q}}}$![]() -divisor $D$
-divisor $D$![]() on the surface $S$
on the surface $S$![]() we can define its volume using the identity
we can define its volume using the identity
for an appropriate positive rational number $\lambda$![]() .
.
Let $D$![]() be an anti-canonical ${{\mathbb {Q}}}$
be an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type with $k\gg 1$
-basis type with $k\gg 1$![]() , and let $C$
, and let $C$![]() be an irreducible reduced curve on $S$
be an irreducible reduced curve on $S$![]() . We write
. We write
where $a$![]() is non-negative real number and $\Delta$
is non-negative real number and $\Delta$![]() is an effective ${{\mathbb {Q}}}$
is an effective ${{\mathbb {Q}}}$![]() -divisor such that $C\not \subset \operatorname {Supp}(\Delta )$
-divisor such that $C\not \subset \operatorname {Supp}(\Delta )$![]() . Let
. Let
In the case that $D$![]() is an ample ${{\mathbb {Q}}}$
is an ample ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type with $k\gg 1$
-basis type with $k\gg 1$![]() we can find a better bound for $a$
we can find a better bound for $a$![]() . One such estimate is given by the following very special case of [Reference Fujita and Odaka8, lemma 2.2].
. One such estimate is given by the following very special case of [Reference Fujita and Odaka8, lemma 2.2].
Theorem 2.7 [Reference Cheltsov, Park and Shramov3, theorem 2.9]
Suppose that $D$![]() is a big ${{\mathbb {Q}}}$
is a big ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type for $k\gg 1$
-basis type for $k\gg 1$![]() . Then
. Then
where $\epsilon _k$![]() is a small constant depending on $k$
is a small constant depending on $k$![]() such that $\epsilon _k\to 0$
such that $\epsilon _k\to 0$![]() as $k\to \infty$
as $k\to \infty$![]() .
.
Corollary 2.8 [Reference Cheltsov, Park and Shramov3, corollary 2.10]
Suppose that $D$![]() is a big ${{\mathbb {Q}}}$
is a big ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type for $k\gg 0,$
-basis type for $k\gg 0,$![]() and
and
for some positive rational number $\mu$![]() . Then
. Then
where $\epsilon _k$![]() is a small constant depending on $k$
is a small constant depending on $k$![]() such that $\epsilon _k \to 0$
such that $\epsilon _k \to 0$![]() as $k\to \infty$
as $k\to \infty$![]() .
.
3. Family No. $3$
In this section we prove the following theorem:
Theorem 3.1 Let $S$![]() be a quasi-smooth member of family No. $3$
be a quasi-smooth member of family No. $3$![]() . Then $\delta (S) \geq \frac {5}{4}$
. Then $\delta (S) \geq \frac {5}{4}$![]() . Moreover, $S$
. Moreover, $S$![]() admits an orbifold Kähler–Einstein metric.
admits an orbifold Kähler–Einstein metric.
Proof. Let $D$![]() be an anti-canonical ${{\mathbb {Q}}}$
be an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type on $S$
-basis type on $S$![]() with $k\gg 0$
with $k\gg 0$![]() . By lemmas 3.2–3.4 the log pair $(S,\, \frac {5}{4}D)$
. By lemmas 3.2–3.4 the log pair $(S,\, \frac {5}{4}D)$![]() is log canonical. Therefore $\delta (S)\geq \frac {5}{4}$
is log canonical. Therefore $\delta (S)\geq \frac {5}{4}$![]() .
.
We divide the proof of the above theorem into a sequence of lemmas. Let $S\subset {{\mathbb {P}}}(1,\,2,\,3,\,4,\,5)$![]() be a quasi-smooth complete intersection log del Pezzo surface given by two quasi-homogeneous polynomials of degrees $6$
be a quasi-smooth complete intersection log del Pezzo surface given by two quasi-homogeneous polynomials of degrees $6$![]() and $8$
and $8$![]() . By suitable coordinate change we may assume that $S$
. By suitable coordinate change we may assume that $S$![]() is given by
is given by
where $\xi$![]() is a constant and $g(x,\,y)$
is a constant and $g(x,\,y)$![]() is a quasi-homogeneous polynomial of degree $8$
is a quasi-homogeneous polynomial of degree $8$![]() . Then $S$
. Then $S$![]() is singular only at the point $\mathsf p_w$
is singular only at the point $\mathsf p_w$![]() , which is a cyclic quotient singularity of type $\tfrac {1}{5}(4,\,3)$
, which is a cyclic quotient singularity of type $\tfrac {1}{5}(4,\,3)$![]() . Since the defining equation of degree $6$
. Since the defining equation of degree $6$![]() of a member of family No. $3$
of a member of family No. $3$![]() does not contain the monomial $ty$
does not contain the monomial $ty$![]() , $\xi = 0$
, $\xi = 0$![]() . Thus $S$
. Thus $S$![]() is given by
is given by

Let $H_x$![]() be the hyperplane section given by $x = 0$
be the hyperplane section given by $x = 0$![]() . Then it is isomorphic to the variety embedded in ${{\mathbb {P}}}(2,\,3,\,4,\,5)$
. Then it is isomorphic to the variety embedded in ${{\mathbb {P}}}(2,\,3,\,4,\,5)$![]() given by
given by
where $\zeta = g(0,\,1)$![]() . We consider the open set $U = S \setminus H_w$
. We consider the open set $U = S \setminus H_w$![]() where $H_w$
where $H_w$![]() is the hyperplane section given by $w=0$
is the hyperplane section given by $w=0$![]() . $H_x|_U$
. $H_x|_U$![]() is isomorphic to the ${{\mathbb {Z}}}_5$
is isomorphic to the ${{\mathbb {Z}}}_5$![]() -quotient of the affine curve given by
-quotient of the affine curve given by
in ${{\mathbb {A}}}^{2}$![]() . From the equation (3.1), we can see that $H_x$
. From the equation (3.1), we can see that $H_x$![]() is irreduciblyreduced and singular at the point $\mathsf p_w$
is irreduciblyreduced and singular at the point $\mathsf p_w$![]() . Also, we have $\operatorname {lct}(S,\, H_x) = \frac {7}{12}$
. Also, we have $\operatorname {lct}(S,\, H_x) = \frac {7}{12}$![]() .
.
Let $D$![]() be an anti-canonical ${{\mathbb {Q}}}$
be an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type on $S$
-basis type on $S$![]() with $k\gg 0$
with $k\gg 0$![]() . We put $\lambda = \frac {5}{4}$
. We put $\lambda = \frac {5}{4}$![]() .
.
Lemma 3.2 The log pair $(S,\, \lambda D)$![]() is log canonical along $H_x\setminus \{\mathsf p_w\}$
is log canonical along $H_x\setminus \{\mathsf p_w\}$![]() .
.
Proof. Suppose that the log pair $(S,\, \lambda D)$![]() is not log canonical at some point $\mathsf p\in H_x\setminus \{\mathsf p_w\}$
is not log canonical at some point $\mathsf p\in H_x\setminus \{\mathsf p_w\}$![]() . We write
. We write
where $a$![]() is non-negative rational number and $\Delta$
is non-negative rational number and $\Delta$![]() is an effective divisor such that $H_x\not \subset \operatorname {Supp}(\Delta )$
is an effective divisor such that $H_x\not \subset \operatorname {Supp}(\Delta )$![]() . By corollary 2.8 we have $a\leq \frac {1}{3} + \epsilon _k < \frac {9}{25}$
. By corollary 2.8 we have $a\leq \frac {1}{3} + \epsilon _k < \frac {9}{25}$![]() for $k \gg 0$
for $k \gg 0$![]() . Since $\lambda a\leq 1$
. Since $\lambda a\leq 1$![]() the log pair $(S,\, H_x + \lambda \Delta )$
the log pair $(S,\, H_x + \lambda \Delta )$![]() is not log canonical at the point $\mathsf p$
is not log canonical at the point $\mathsf p$![]() . By the inversion of adjunction formula the log pair $(H_x,\, \lambda \Delta |_{H_x})$
. By the inversion of adjunction formula the log pair $(H_x,\, \lambda \Delta |_{H_x})$![]() is not log canonical at point $\mathsf p$
is not log canonical at point $\mathsf p$![]() . We have the inequalities
. We have the inequalities
which imply that $a< -1$![]() . This is impossible. Therefore the log pair $(S,\, \lambda D)$
. This is impossible. Therefore the log pair $(S,\, \lambda D)$![]() is log canonical along $H_x\setminus \{\mathsf p_w\}$
is log canonical along $H_x\setminus \{\mathsf p_w\}$![]() .
.
Lemma 3.3 The log pair $(S,\, \lambda D)$![]() is log canonical long $S\setminus H_x$
is log canonical long $S\setminus H_x$![]() .
.
Proof. Suppose that the log pair $(S,\, \lambda D)$![]() is not log canonical at some point $\mathsf p\in S\setminus H_x$
is not log canonical at some point $\mathsf p\in S\setminus H_x$![]() . By suitable coordinate change we can assume that $\mathsf p = \mathsf p_x$
. By suitable coordinate change we can assume that $\mathsf p = \mathsf p_x$![]() .
.
Let $C$![]() be the curve on $S$
be the curve on $S$![]() cut out by the equation $y=0$
cut out by the equation $y=0$![]() . Then $C$
. Then $C$![]() passes through the point $\mathsf p$
passes through the point $\mathsf p$![]() . Since the curve $C$
. Since the curve $C$![]() is smooth at $\mathsf p_w$
is smooth at $\mathsf p_w$![]() and $C\cdot H_x= \frac {4}{5}$
and $C\cdot H_x= \frac {4}{5}$![]() , it is irreducible and reduced. Let $\mathcal {L}$
, it is irreducible and reduced. Let $\mathcal {L}$![]() be the pencil cut out by the equations $\alpha xy + \beta z = 0$
be the pencil cut out by the equations $\alpha xy + \beta z = 0$![]() where $[\alpha : \beta ]\in {{\mathbb {P}}}^{1}$
where $[\alpha : \beta ]\in {{\mathbb {P}}}^{1}$![]() . The base locus of $\mathcal {L}$
. The base locus of $\mathcal {L}$![]() is given by $z=yx=0$
is given by $z=yx=0$![]() . Since $S\cap H_x \cap H_z = \{\mathsf p_y\}$
. Since $S\cap H_x \cap H_z = \{\mathsf p_y\}$![]() and $S\cap H_y \cap H_z = \{\mathsf p_x,\, \mathsf p_w\}$
and $S\cap H_y \cap H_z = \{\mathsf p_x,\, \mathsf p_w\}$![]() we have $\operatorname {BS}(\mathcal {L}) = \{\mathsf p_x,\, \mathsf p_y,\, \mathsf p_w\}$
we have $\operatorname {BS}(\mathcal {L}) = \{\mathsf p_x,\, \mathsf p_y,\, \mathsf p_w\}$![]() . Thus there is a general member $M\in \mathcal {L}$
. Thus there is a general member $M\in \mathcal {L}$![]() such that $\mathsf p\in M$
such that $\mathsf p\in M$![]() and $C\not \subset \operatorname {Supp}(M)$
and $C\not \subset \operatorname {Supp}(M)$![]() . We have
. We have
It implies that $\operatorname {mult}_{\mathsf p}(C)$![]() is either $1$
is either $1$![]() or $2$
or $2$![]() . We write
. We write
where $b$![]() is non-negative rational number and $\Sigma$
is non-negative rational number and $\Sigma$![]() is an effective ${{\mathbb {Q}}}$
is an effective ${{\mathbb {Q}}}$![]() -divisor such that $C\not \subset \operatorname {Supp}(\Sigma )$
-divisor such that $C\not \subset \operatorname {Supp}(\Sigma )$![]() . By Corollary 2.8, we have $b\leq \frac {1}{6} + \epsilon _k < \frac {1}{3}$
. By Corollary 2.8, we have $b\leq \frac {1}{6} + \epsilon _k < \frac {1}{3}$![]() for $k \gg 0$
for $k \gg 0$![]() .
.
We assume that $\operatorname {mult}_{\mathsf p}(C) = 1$![]() . Since $\lambda b\leq 1$
. Since $\lambda b\leq 1$![]() the log pair $(S,\, C + \lambda \Sigma )$
the log pair $(S,\, C + \lambda \Sigma )$![]() is not log canonical at the point $\mathsf p$
is not log canonical at the point $\mathsf p$![]() . By the inversion of adjunction formula the log pair $(C,\, \lambda \Sigma |_C)$
. By the inversion of adjunction formula the log pair $(C,\, \lambda \Sigma |_C)$![]() is not log canonical at the point $\mathsf p$
is not log canonical at the point $\mathsf p$![]() . We have the inequalities
. We have the inequalities
They imply that $b<0$![]() . It is impossible. Thus $\operatorname {mult}_{\mathsf p}(C) = 2$
. It is impossible. Thus $\operatorname {mult}_{\mathsf p}(C) = 2$![]() . From lemma 2.2 we have the following inequalities
. From lemma 2.2 we have the following inequalities
Then we have $\frac {1}{3}< b$![]() . It is impossible. Thus the log pair $(S,\, \lambda D)$
. It is impossible. Thus the log pair $(S,\, \lambda D)$![]() is log canonical along $S\setminus H_x$
is log canonical along $S\setminus H_x$![]() .
.
Lemma 3.4 The log pair $(S,\, \lambda D)$![]() is log canonical at $\mathsf p_w$
is log canonical at $\mathsf p_w$![]() .
.
Proof. Suppose that the log pair $(S,\, \lambda D)$![]() is not log canonical at $\mathsf p_w$
is not log canonical at $\mathsf p_w$![]() . We consider the open set $U$
. We consider the open set $U$![]() given by $w\neq 0$
given by $w\neq 0$![]() . Then we may regard $y$
. Then we may regard $y$![]() and $t$
and $t$![]() are local coordinates with weights $\operatorname {wt}(y) = 4$
are local coordinates with weights $\operatorname {wt}(y) = 4$![]() and $\operatorname {wt}(t) = 3$
and $\operatorname {wt}(t) = 3$![]() in $U$
in $U$![]() . Let $\pi \colon \bar {S}\to S$
. Let $\pi \colon \bar {S}\to S$![]() be the weighted blow-up at $\mathsf p_w$
be the weighted blow-up at $\mathsf p_w$![]() with weights $\operatorname {wt}(y) = 4$
with weights $\operatorname {wt}(y) = 4$![]() and $\operatorname {wt}(t) = 3$
and $\operatorname {wt}(t) = 3$![]() . Then $\bar {S}$
. Then $\bar {S}$![]() has the singular points $\mathsf q_1$
has the singular points $\mathsf q_1$![]() and $\mathsf q_2$
and $\mathsf q_2$![]() of types $\frac {1}{4}(1,\,1)$
of types $\frac {1}{4}(1,\,1)$![]() and $\frac {1}{3}(1,\,1)$
and $\frac {1}{3}(1,\,1)$![]() , respectively. We have
, respectively. We have
where $\bar {H_x}$![]() is the strict transform of $H_x$
is the strict transform of $H_x$![]() and $E$
and $E$![]() is the exceptional divisor of $\pi$
is the exceptional divisor of $\pi$![]() . We write
. We write
where $a$![]() is a non-negative rational number and $\Delta$
is a non-negative rational number and $\Delta$![]() is an effective ${{\mathbb {Q}}}$
is an effective ${{\mathbb {Q}}}$![]() -divisor such that $H_x\not \subset \operatorname {Supp}(\Delta )$
-divisor such that $H_x\not \subset \operatorname {Supp}(\Delta )$![]() . By corollary 2.8, we have
. By corollary 2.8, we have
for $k\gg 0$![]() . We also have
. We also have
where $\bar {\Delta }$![]() is the strict transform of $\Delta$
is the strict transform of $\Delta$![]() and $m$
and $m$![]() is a non-negative rational number. To obtain a bound of $m$
is a non-negative rational number. To obtain a bound of $m$![]() we consider the inequality
we consider the inequality
Since $\Delta \cdot H_x = (D - aH_x)\cdot H_x = \frac {2}{5} - \frac {2}{5}a$![]() and $E^{2} = -\frac {5}{12}$
and $E^{2} = -\frac {5}{12}$![]() , we have
, we have
Meanwhile, we have
where
It implies that the log pair $(\bar {S},\, \lambda (a \bar {H_x} + \bar {\Delta }) + \mu E)$![]() is not log canonical at some point $\mathsf q\in E$
is not log canonical at some point $\mathsf q\in E$![]() . From the inequalities (3.2) and (3.3) we have $\mu \leq 1$
. From the inequalities (3.2) and (3.3) we have $\mu \leq 1$![]() . It implies that the log pair $(\bar {S},\, \lambda (a \bar {H_x} + \bar {\Delta }) +E)$
. It implies that the log pair $(\bar {S},\, \lambda (a \bar {H_x} + \bar {\Delta }) +E)$![]() is not log canonical at the point $\mathsf q$
is not log canonical at the point $\mathsf q$![]() . We consider the case that $E$
. We consider the case that $E$![]() is smooth at the point $\mathsf q$
is smooth at the point $\mathsf q$![]() . By the inversion of adjunction formula the log pair $(E,\, \lambda (a \bar {H_x} + \bar {\Delta })|_E)$
. By the inversion of adjunction formula the log pair $(E,\, \lambda (a \bar {H_x} + \bar {\Delta })|_E)$![]() is not log canonical at $\mathsf q$
is not log canonical at $\mathsf q$![]() . If $\mathsf q\not \in \bar {H_x}$
. If $\mathsf q\not \in \bar {H_x}$![]() then the log pair $(E,\, \lambda \bar {\Delta }|_E)$
then the log pair $(E,\, \lambda \bar {\Delta }|_E)$![]() is not log canonical at $\mathsf q$
is not log canonical at $\mathsf q$![]() . From this we have the inequalities
. From this we have the inequalities
They imply that $\frac {48}{25}< m$![]() . From the inequality (3.3), it is impossible. Thus $\mathsf q\in \bar {H_x}$
. From the inequality (3.3), it is impossible. Thus $\mathsf q\in \bar {H_x}$![]() . From lemma 2.2 and the inequality (3.3) we have the inequalities
. From lemma 2.2 and the inequality (3.3) we have the inequalities
They imply that $\frac {19}{25}< a$![]() . From the inequality (3.2), it is impossible. Thus $E$
. From the inequality (3.2), it is impossible. Thus $E$![]() is singular at the point $\mathsf q$
is singular at the point $\mathsf q$![]() . Also, the point $\mathsf q$
. Also, the point $\mathsf q$![]() is either $\mathsf q_1$
is either $\mathsf q_1$![]() or $\mathsf q_2$
or $\mathsf q_2$![]() .
.
Suppose that $\mathsf q = \mathsf q_1$![]() . Then there is a cyclic cover $\varphi \colon \tilde {U}\to \bar {U}$
. Then there is a cyclic cover $\varphi \colon \tilde {U}\to \bar {U}$![]() of degree $4$
of degree $4$![]() branched over $\mathsf q$
branched over $\mathsf q$![]() for some open set $\mathsf q\in \bar {U}$
for some open set $\mathsf q\in \bar {U}$![]() on $\bar {S}$
on $\bar {S}$![]() such that $\tilde {U}$
such that $\tilde {U}$![]() is smooth. From lemma 2.3, the log pair $(\tilde {U},\, \lambda \tilde {\Delta } + \tilde {E})$
is smooth. From lemma 2.3, the log pair $(\tilde {U},\, \lambda \tilde {\Delta } + \tilde {E})$![]() is not log canonical at some point $\tilde {\mathsf q}$
is not log canonical at some point $\tilde {\mathsf q}$![]() where $\tilde {\Delta } = \varphi ^{*}(\Delta |_U)$
where $\tilde {\Delta } = \varphi ^{*}(\Delta |_U)$![]() , $\tilde {E} = \varphi ^{*}(E|_U)$
, $\tilde {E} = \varphi ^{*}(E|_U)$![]() and $\varphi (\tilde {\mathsf q}) = \mathsf q$
and $\varphi (\tilde {\mathsf q}) = \mathsf q$![]() . By the inversion of adjunction formula the log pair $(\tilde {E},\, \lambda \tilde {\Delta }|_{\tilde {E}})$
. By the inversion of adjunction formula the log pair $(\tilde {E},\, \lambda \tilde {\Delta }|_{\tilde {E}})$![]() is not log canonical at the point $\tilde {\mathsf q}$
is not log canonical at the point $\tilde {\mathsf q}$![]() . From this we have the inequalities
. From this we have the inequalities
They imply that $\frac {12}{25} < m$![]() . From the inequality (3.3), it is impossible. Thus $\mathsf q = \mathsf q_2$
. From the inequality (3.3), it is impossible. Thus $\mathsf q = \mathsf q_2$![]() . Similarly, we can see that this case is impossible. Therefore the log pair $(S,\, \lambda D)$
. Similarly, we can see that this case is impossible. Therefore the log pair $(S,\, \lambda D)$![]() is log canonical at the point $\mathsf p_w$
is log canonical at the point $\mathsf p_w$![]() .
.
By the above lemmas we prove that the log pair $(S,\,\lambda D)$![]() is log canonical.
is log canonical.
4. On smooth points of family No. $40$
Let $S_n\subset {{\mathbb {P}}}(1,\,1,\,n,\,n,\,2n-1)$![]() be a quasi-smooth complete intersection log del Pezzo surface given by two quasi-homogeneous polynomials of degree $2n$
be a quasi-smooth complete intersection log del Pezzo surface given by two quasi-homogeneous polynomials of degree $2n$![]() , where $n$
, where $n$![]() is a positive integer bigger than $1$
is a positive integer bigger than $1$![]() . By suitable coordinate change we may assume that $S_n$
. By suitable coordinate change we may assume that $S_n$![]() is given by
is given by
where $f_i$![]() , $\hat {f}_i$
, $\hat {f}_i$![]() , $g_i$
, $g_i$![]() and $\hat {g}_i$
and $\hat {g}_i$![]() are homogeneous polynomials of degree $i$
are homogeneous polynomials of degree $i$![]() . Then $S_n$
. Then $S_n$![]() is only singular at the point $\mathsf p_w$
is only singular at the point $\mathsf p_w$![]() of type $\frac {1}{2n-1}(1,\,1)$
of type $\frac {1}{2n-1}(1,\,1)$![]() . In the paper [Reference Kim and Park10], we have $\alpha (S_2) = 7/10$
. In the paper [Reference Kim and Park10], we have $\alpha (S_2) = 7/10$![]() . It implies that $S_2$
. It implies that $S_2$![]() admits an orbifold Kähler–Einstein metric. Thus we only consider the cases that $n \geq 3$
admits an orbifold Kähler–Einstein metric. Thus we only consider the cases that $n \geq 3$![]() .
.
Let $D$![]() be an anti-canonical ${{\mathbb {Q}}}$
be an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type on $S_n$
-basis type on $S_n$![]() with $k\gg 0$
with $k\gg 0$![]() . We set $\lambda = \frac {6n}{4n+3}$
. We set $\lambda = \frac {6n}{4n+3}$![]() . To prove that $\delta (S_n) > 1$
. To prove that $\delta (S_n) > 1$![]() along the smooth points of $S_n$
along the smooth points of $S_n$![]() , we consider the following.
, we consider the following.
Lemma 4.1 The log pair $(S_n,\, \lambda D)$![]() is log canonical along $S_n\setminus \{\mathsf p_w\}$
is log canonical along $S_n\setminus \{\mathsf p_w\}$![]()
Proof. For the convenience, we set $S = S_n$![]() . Suppose that the log pair $(S,\, \lambda D)$
. Suppose that the log pair $(S,\, \lambda D)$![]() is not log canonical at some point $\mathsf p\in S\setminus \{\mathsf p_w\}$
is not log canonical at some point $\mathsf p\in S\setminus \{\mathsf p_w\}$![]() . Let $\mathcal {L}=|-K_S|$
. Let $\mathcal {L}=|-K_S|$![]() be the pencil cut out on $S$
be the pencil cut out on $S$![]() by the equations $\alpha x + \beta y = 0$
by the equations $\alpha x + \beta y = 0$![]() where $[\alpha : \beta ]\in {{\mathbb {P}}}^{1}$
where $[\alpha : \beta ]\in {{\mathbb {P}}}^{1}$![]() . Since the point $\mathsf p$
. Since the point $\mathsf p$![]() is not the point $\mathsf p_w$
is not the point $\mathsf p_w$![]() , there is the unique curve $C\in \mathcal {L}$
, there is the unique curve $C\in \mathcal {L}$![]() passing through $\mathsf p$
passing through $\mathsf p$![]() . Without loss of generality we can assume that $\mathsf p$
. Without loss of generality we can assume that $\mathsf p$![]() is contained in the open set $U_x$
is contained in the open set $U_x$![]() given by $x = 1$
given by $x = 1$![]() . Then $C$
. Then $C$![]() is given by the equation $y = \xi x$
is given by the equation $y = \xi x$![]() on $S$
on $S$![]() where $\xi$
where $\xi$![]() is a constant. On the open set $U_x$
is a constant. On the open set $U_x$![]() , the affine curve $C|_{U_x}$
, the affine curve $C|_{U_x}$![]() is given by
is given by
Thus it is isomorphic to the variety given by
where $\xi _1\ldots,\,\xi _4$![]() are constants. Since $S$
are constants. Since $S$![]() is quasi-smooth at least one $\xi _i$
is quasi-smooth at least one $\xi _i$![]() in $i\in \{1,\,2,\,3,\,4\}$
in $i\in \{1,\,2,\,3,\,4\}$![]() is non-zero. It implies that the rank of the quadratic equation (4.1) is either $1$
is non-zero. It implies that the rank of the quadratic equation (4.1) is either $1$![]() or $2$
or $2$![]() . We assume that $C$
. We assume that $C$![]() is irreducible. By the quadratic equation (4.1), $C$
is irreducible. By the quadratic equation (4.1), $C$![]() is smooth at the point $\mathsf p$
is smooth at the point $\mathsf p$![]() . We write
. We write
where $\Delta$![]() is an effective ${{\mathbb {Q}}}$
is an effective ${{\mathbb {Q}}}$![]() -divisor such that $C\not \subset \operatorname {Supp}(\Delta )$
-divisor such that $C\not \subset \operatorname {Supp}(\Delta )$![]() and $a$
and $a$![]() is a non-negative constant. By corollary 2.8 we have $\lambda a \leq 1$
is a non-negative constant. By corollary 2.8 we have $\lambda a \leq 1$![]() . By the inversion of adjunction formula, the log pair $(C,\, \lambda \Delta |_C)$
. By the inversion of adjunction formula, the log pair $(C,\, \lambda \Delta |_C)$![]() is not log canonical at $\mathsf p$
is not log canonical at $\mathsf p$![]() . Then we have the inequalities
. Then we have the inequalities
The above inequalities imply that $a$![]() is negative. This is impossible. Thus $C$
is negative. This is impossible. Thus $C$![]() is reducible. We now turn to the case that $C$
is reducible. We now turn to the case that $C$![]() is the sum of two irreducible curves $L_1$
is the sum of two irreducible curves $L_1$![]() and $L_2$
and $L_2$![]() , that is, we write
, that is, we write
Then $L_1$![]() and $L_2$
and $L_2$![]() satisfy the following intersection numbers:
satisfy the following intersection numbers:
Without loss of generality we can assume that $\mathsf p\in L_1$![]() . We write
. We write
where $\Sigma$![]() is an effective ${{\mathbb {Q}}}$
is an effective ${{\mathbb {Q}}}$![]() -divisor such that $L_1\not \subset \operatorname {Supp}(\Sigma )$
-divisor such that $L_1\not \subset \operatorname {Supp}(\Sigma )$![]() and $b$
and $b$![]() is a non-negative number. By theorem 2.7, we have
is a non-negative number. By theorem 2.7, we have
where $\epsilon _k$![]() is a small constant depending on $k$
is a small constant depending on $k$![]() such that $\epsilon _k\to 0$
such that $\epsilon _k\to 0$![]() as $k\to \infty$
as $k\to \infty$![]() . Since
. Since
and $L_2^{2} < 0$![]() , we have $\operatorname {vol}(D-xL_1)=0$
, we have $\operatorname {vol}(D-xL_1)=0$![]() for $x\geq 1$
for $x\geq 1$![]() . It implies that $\tau (L_1) = 1$
. It implies that $\tau (L_1) = 1$![]() . Meanwhile, the equalities
. Meanwhile, the equalities
imply that $(D - xL_1)$![]() is nef whenever $\frac {1}{n}\geq x$
is nef whenever $\frac {1}{n}\geq x$![]() . Thus
. Thus
for $\frac {1}{n}\geq x$![]() . We next consider the volume of $D - xL_1$
. We next consider the volume of $D - xL_1$![]() for $1\geq x \geq \frac {1}{n}$
for $1\geq x \geq \frac {1}{n}$![]() . Let
. Let
be the nef divisor for $1\geq x \geq \frac {1}{n}$![]() . Then we write
. Then we write
Since $P\cdot L_2 = 0$![]() , the right-hand side of the above equation is the Zariski decomposition of $D - xL_1$
, the right-hand side of the above equation is the Zariski decomposition of $D - xL_1$![]() . Thus
. Thus
for $1\geq x \geq \frac {1}{n}$![]() . Then we have
. Then we have

Thus we obtain
It implies that $\lambda b \leq 1$![]() . By the inversion of adjunction formula we have
. By the inversion of adjunction formula we have
It implies that
This is impossible. Therefore the log pair $(S,\, \lambda D)$![]() is log canonical along $S\setminus \{\mathsf p_w\}$
is log canonical along $S\setminus \{\mathsf p_w\}$![]() .
.
5. On the singular point of family No. $40$
In this section we prove the following theorem.
Theorem 5.1 Let $S_n\subset {{\mathbb {P}}}(1,\,1,\,n,\,n,\,2n-1)$![]() be a quasi-smooth member of family No. $40$
be a quasi-smooth member of family No. $40$![]() where $n$
where $n$![]() is a positive integer. Then $\delta (S_n) > \frac {6n}{4n+3}$
is a positive integer. Then $\delta (S_n) > \frac {6n}{4n+3}$![]() . Moreover, $S_n$
. Moreover, $S_n$![]() admits an orbifold Kähler–Einstein metric.
admits an orbifold Kähler–Einstein metric.
We divide the proof of the above theorem into a sequence of lemmas.
5.1 Basis
Let $\mathcal {L} = H^{0}(S_n,\, \mathcal {O}_{S_n}(k))$![]() be the vector space where $k$
be the vector space where $k$![]() is a positive integer. In this subsection, we find a monomial basis of $\mathcal {L}$
is a positive integer. In this subsection, we find a monomial basis of $\mathcal {L}$![]() . We define a subset of $\mathcal {L}$
. We define a subset of $\mathcal {L}$![]() as follows:
as follows:
where ${{\mathbb {C}}}[x,\,y,\,z,\,t,\,w]_k$![]() is the set of quasi-homogeneous polynomials of degree $k$
is the set of quasi-homogeneous polynomials of degree $k$![]() with weights $\operatorname {wt}(x) = \operatorname {wt}(y) = 1$
with weights $\operatorname {wt}(x) = \operatorname {wt}(y) = 1$![]() , $\operatorname {wt}(z) = \operatorname {wt}(t) = n$
, $\operatorname {wt}(z) = \operatorname {wt}(t) = n$![]() and $\operatorname {wt}(w) = 2n - 1$
and $\operatorname {wt}(w) = 2n - 1$![]() . The equations
. The equations
and
hold in $S_n$![]() . From the equations (5.1) and (5.2), we can obtain
. From the equations (5.1) and (5.2), we can obtain
From the equations (5.1), (5.2) and (5.3) we can see that $\mathcal {L}$![]() is generated by $\mathcal {B}$
is generated by $\mathcal {B}$![]() on $S_n$
on $S_n$![]() .
.
Claim. The set $\mathcal {B}$![]() is the basis of $\mathcal {L}$
is the basis of $\mathcal {L}$![]() .
.
In a neighbourhood $U$![]() of $S_n$
of $S_n$![]() at $\mathsf p_w$
at $\mathsf p_w$![]() , we may regard $z$
, we may regard $z$![]() and $t$
and $t$![]() are local coordinates with weights $\operatorname {wt}(z) = 1$
are local coordinates with weights $\operatorname {wt}(z) = 1$![]() and $\operatorname {wt}(t) = 1$
and $\operatorname {wt}(t) = 1$![]() . Then $U$
. Then $U$![]() is isomorphic to the quotient of ${{\mathbb {C}}}^{2}$
is isomorphic to the quotient of ${{\mathbb {C}}}^{2}$![]() by the action $\zeta \cdot (z,\, t) \mapsto (\zeta z,\, \zeta t)$
by the action $\zeta \cdot (z,\, t) \mapsto (\zeta z,\, \zeta t)$![]() where $\zeta$
where $\zeta$![]() is a primitive $(2n-1)$
is a primitive $(2n-1)$![]() -th root of unity. We have the isomorphism $\sigma \colon {{\mathbb {C}}}/{{\mathbb {Z}}}_{2n-1}\to U$
-th root of unity. We have the isomorphism $\sigma \colon {{\mathbb {C}}}/{{\mathbb {Z}}}_{2n-1}\to U$![]() given by $(z,\,t)\mapsto (z^{2} + f_{>2n},\, t^{2} + g_{> 2n},\,z,\,t)$
given by $(z,\,t)\mapsto (z^{2} + f_{>2n},\, t^{2} + g_{> 2n},\,z,\,t)$![]() where $f_{>2n}$
where $f_{>2n}$![]() and $g_{>2n}$
and $g_{>2n}$![]() are power series such that the orders are greater than $2n$
are power series such that the orders are greater than $2n$![]() . Then for a section $s(x,\, y,\, z,\, t,\, w)\in \mathcal {L}$
. Then for a section $s(x,\, y,\, z,\, t,\, w)\in \mathcal {L}$![]() the local equation in $U$
the local equation in $U$![]() is given by $\sigma ^{*}(s(x,\, y,\, z,\, t,\, 1))$
is given by $\sigma ^{*}(s(x,\, y,\, z,\, t,\, 1))$![]() . We consider the following set:
. We consider the following set:
Let $\mathbf {x} = x^{a}y^{b}z^{c}t^{d}w^{e}$![]() be a monomial in $\mathcal {L}$
be a monomial in $\mathcal {L}$![]() . Then $\sigma ^{*}(\mathbf {x})$
. Then $\sigma ^{*}(\mathbf {x})$![]() is
is
where $h(z,\,t)$![]() is the power series such that the order of $h(z,\,t)$
is the power series such that the order of $h(z,\,t)$![]() is greater than $2a+2b+c+d$
is greater than $2a+2b+c+d$![]() . Thus the Zariski tangent term of $\sigma ^{*}(\mathbf {x})$
. Thus the Zariski tangent term of $\sigma ^{*}(\mathbf {x})$![]() is $z^{2a + c}t^{2b + d}$
is $z^{2a + c}t^{2b + d}$![]() . It implies that every element of $\mathcal {T}$
. It implies that every element of $\mathcal {T}$![]() is a monomial in ${{\mathbb {C}}}[z,\,t]$
is a monomial in ${{\mathbb {C}}}[z,\,t]$![]() .
.
Lemma 5.2 The number of elements of the set $\mathcal {T}$![]() is equal to the number of elements of the set $\mathcal {B}$
is equal to the number of elements of the set $\mathcal {B}$![]() .
.
Proof. Let $\mathbf {x_1}=x^{a_1}y^{b_1}z^{c_1}t^{d_1}$![]() and $\mathbf {x_2}=x^{a_2}y^{b_2}z^{c_2}t^{d_2}$
and $\mathbf {x_2}=x^{a_2}y^{b_2}z^{c_2}t^{d_2}$![]() be monomials in the set $\mathcal {B}$
be monomials in the set $\mathcal {B}$![]() such that the Zariski tangent terms of $\sigma ^{*}(\mathbf {x_1})$
such that the Zariski tangent terms of $\sigma ^{*}(\mathbf {x_1})$![]() and $\sigma ^{*}(\mathbf {x_2})$
and $\sigma ^{*}(\mathbf {x_2})$![]() are equal. Then we have
are equal. Then we have
Since the two monomials $\mathbf {x_1}$![]() and $\mathbf {x_2}$
and $\mathbf {x_2}$![]() have same degree, we have
have same degree, we have
From the above equations, we obtain the equations
If $a_1 = a_2$![]() then we have $b_1 = b_2$
then we have $b_1 = b_2$![]() , $c_1 = c_2$
, $c_1 = c_2$![]() and $d_1 = d_2$
and $d_1 = d_2$![]() . Thus we can assume that $a_1 > a_2$
. Thus we can assume that $a_1 > a_2$![]() . Then we have $b_1 < b_2$
. Then we have $b_1 < b_2$![]() , $c_1 < c_2$
, $c_1 < c_2$![]() and $d_1 > d_2$
and $d_1 > d_2$![]() . We can write the two monomials $\mathbf {x_1}$
. We can write the two monomials $\mathbf {x_1}$![]() and $\mathbf {x_2}$
and $\mathbf {x_2}$![]() as
as
They imply that $2(a_1 - a_2) = c_2 - c_1$![]() and $2(b_2 - b_1) = d_1 - d_2$
and $2(b_2 - b_1) = d_1 - d_2$![]() . We also have $a_1-a_2 = b_2 - b_1$
. We also have $a_1-a_2 = b_2 - b_1$![]() and $c_2-c_1 = d_1 - d_2$
and $c_2-c_1 = d_1 - d_2$![]() . Thus the two monomials $\mathbf {x_1}$
. Thus the two monomials $\mathbf {x_1}$![]() and $\mathbf {x_2}$
and $\mathbf {x_2}$![]() are
are
However monomials of the form $(yz^{2})^{\xi } x^{a}y^{b}z^{c}t^{d}$![]() are not contained in the set $\mathcal {B}$
are not contained in the set $\mathcal {B}$![]() where $\xi$
where $\xi$![]() is a positive integer. Therefore the two monomials $\mathbf {x_1}$
is a positive integer. Therefore the two monomials $\mathbf {x_1}$![]() and $\mathbf {x_2}$
and $\mathbf {x_2}$![]() are equal.
are equal.
By lemma 5.2, we obtain the following.
Corollary 5.3 The set $\mathcal {B}$![]() is the basis of $\mathcal {L}$
is the basis of $\mathcal {L}$![]() .
.
Proof. We consider the following set:
It is obvious that $\dim _{{{\mathbb {C}}}} \mathcal {Z}\leq \dim _{{{\mathbb {C}}}}\mathcal {L}$![]() . Since $\mathcal {T}\subset \mathcal {Z}$
. Since $\mathcal {T}\subset \mathcal {Z}$![]() , we have $|\mathcal {T}|\leq \dim _{{{\mathbb {C}}}} \mathcal {Z}$
, we have $|\mathcal {T}|\leq \dim _{{{\mathbb {C}}}} \mathcal {Z}$![]() . We also have $\dim _{{{\mathbb {C}}}}\mathcal {L} \leq |\mathcal {B}|$
. We also have $\dim _{{{\mathbb {C}}}}\mathcal {L} \leq |\mathcal {B}|$![]() . By lemma 5.2 we have $\dim _{{{\mathbb {C}}}}\mathcal {L} = |\mathcal {B}|$
. By lemma 5.2 we have $\dim _{{{\mathbb {C}}}}\mathcal {L} = |\mathcal {B}|$![]() . Consequently, $\mathcal {B}$
. Consequently, $\mathcal {B}$![]() is the basis of $\mathcal {L}$
is the basis of $\mathcal {L}$![]() .
.
5.2 Monomial
We consider the ring ${{\mathbb {C}}}[z,\,t]$![]() . The order of monomials in the ring ${{\mathbb {C}}}[z,\,t]$
. The order of monomials in the ring ${{\mathbb {C}}}[z,\,t]$![]() is the graded lexicographic order with $z< t$
is the graded lexicographic order with $z< t$![]() . We set $l=h^{0}(S_n,\, \mathcal {O}_{S_n}(k))$
. We set $l=h^{0}(S_n,\, \mathcal {O}_{S_n}(k))$![]() . All elements of the basis $\mathcal {B}$
. All elements of the basis $\mathcal {B}$![]() can be written
can be written
in the order of their Zariski tangent terms. we set $a=\sum _{i=1}^{l} a_i$![]() , $b=\sum _{i=1}^{l} b_i$
, $b=\sum _{i=1}^{l} b_i$![]() , $c=\sum _{i=1}^{l} c_i$
, $c=\sum _{i=1}^{l} c_i$![]() , $d=\sum _{i=1}^{l} d_i$
, $d=\sum _{i=1}^{l} d_i$![]() and $e=\sum _{i=1}^{l} e_i$
and $e=\sum _{i=1}^{l} e_i$![]() .
.
Lemma 5.4 For every basis $\{s_1,\,\ldots s_l\}$![]() of $\mathcal {L},$
of $\mathcal {L},$![]() the Newton polygon of the power series by applying the coordinate change $z\mapsto z-\sum _{j>0} \alpha _j t^{j}$
the Newton polygon of the power series by applying the coordinate change $z\mapsto z-\sum _{j>0} \alpha _j t^{j}$![]() and $t\mapsto t$
and $t\mapsto t$![]() to the power series $\prod _{i=1}^{l} \sigma ^{*}(s_i(x,\,y,\,z,\,t,\,1))$
to the power series $\prod _{i=1}^{l} \sigma ^{*}(s_i(x,\,y,\,z,\,t,\,1))$![]() contains the point corresponding to the monomial $z^{c+2a}t^{d+2b}$
contains the point corresponding to the monomial $z^{c+2a}t^{d+2b}$![]() .
.
Proof. We set $\xi _i = \sigma ^{*}(x^{a_i}y^{b_i}z^{c_i}t^{d_i}w^{e_i})$![]() for each $i$
for each $i$![]() . Then the Zariski tangent term of $\xi _i$
. Then the Zariski tangent term of $\xi _i$![]() is the monomial $z^{c_i+2a_i}t^{d_i+2b_i}$
is the monomial $z^{c_i+2a_i}t^{d_i+2b_i}$![]() for each $i$
for each $i$![]() . Let $\zeta _i$
. Let $\zeta _i$![]() be the power series by applying the coordinate change $z\mapsto z-\sum _{j>0} \alpha _j t^{j}$
be the power series by applying the coordinate change $z\mapsto z-\sum _{j>0} \alpha _j t^{j}$![]() and $t\mapsto t$
and $t\mapsto t$![]() to $\xi _i$
to $\xi _i$![]() for each $i$
for each $i$![]() . And let $T$
. And let $T$![]() be the $l\times l$
be the $l\times l$![]() matrix whose entry in row $i$
matrix whose entry in row $i$![]() and column $j$
and column $j$![]() is the coefficient of the monomial $z^{c_j+2a_j}t^{d_j + 2b_j}$
is the coefficient of the monomial $z^{c_j+2a_j}t^{d_j + 2b_j}$![]() of $\zeta _i$
of $\zeta _i$![]() . Since the Zariski tangent terms of $\zeta _i$
. Since the Zariski tangent terms of $\zeta _i$![]() are $(z-\alpha _1 t)^{c_i+2a_i}t^{d_i + 2b_i}$
are $(z-\alpha _1 t)^{c_i+2a_i}t^{d_i + 2b_i}$![]() , all monomials less than $z^{c_i+2a_i}t^{d_i + 2b_i}$
, all monomials less than $z^{c_i+2a_i}t^{d_i + 2b_i}$![]() in the monomial ordering are not contained in $\zeta _i$
in the monomial ordering are not contained in $\zeta _i$![]() for each $i$
for each $i$![]() . Thus the matrix $T$
. Thus the matrix $T$![]() is the upper triangular matrix whose every diagonal entry is $1$
is the upper triangular matrix whose every diagonal entry is $1$![]() .
.
For any $l\times l$![]() invertible matrix $M$
invertible matrix $M$![]() there is a permutation matrix $P$
there is a permutation matrix $P$![]() such that $PMT$
such that $PMT$![]() is the upper triangular matrix. Then the power series $\eta _i$
is the upper triangular matrix. Then the power series $\eta _i$![]() with $i=1,\,\ldots l$
with $i=1,\,\ldots l$![]() given by
given by

contain the monomial $z^{c_i+2a_i}t^{d_i + 2b_i}$![]() . Thus the Newton polygon of $\prod _{i=1}^{l} \eta _i$
. Thus the Newton polygon of $\prod _{i=1}^{l} \eta _i$![]() contains the point corresponding to the monomial $z^{c+2a}t^{d+2b}$
contains the point corresponding to the monomial $z^{c+2a}t^{d+2b}$![]() .
.
Lemma 5.5 The inequalities $\frac {1}{kl}(c + 2a) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$![]() and $\frac {1}{kl}(d + 2b) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$
and $\frac {1}{kl}(d + 2b) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$![]() hold where $\epsilon _k$
hold where $\epsilon _k$![]() is a small constant depending on $k$
is a small constant depending on $k$![]() such that $\epsilon _k \to 0$
such that $\epsilon _k \to 0$![]() as $k\to \infty$
as $k\to \infty$![]() .
.
Proof. We consider the monomials
of the basis $\mathcal {B}$![]() . Let $B_i$
. Let $B_i$![]() be the effective Cartier divisor given by $x^{a_i}y^{b_i}z^{c_i}t^{d_i}w^{e_i} = 0$
be the effective Cartier divisor given by $x^{a_i}y^{b_i}z^{c_i}t^{d_i}w^{e_i} = 0$![]() for each $i$
for each $i$![]() . Then
. Then
is the anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type. Moreover $klB$
-basis type. Moreover $klB$![]() is given by $x^{a}y^{b}z^{c}t^{d}w^{e} = 0$
is given by $x^{a}y^{b}z^{c}t^{d}w^{e} = 0$![]() where $a=\sum _{i=1}^{l} a_i$
where $a=\sum _{i=1}^{l} a_i$![]() , $b=\sum _{i=1}^{l} b_i$
, $b=\sum _{i=1}^{l} b_i$![]() , $c=\sum _{i=1}^{l} c_i$
, $c=\sum _{i=1}^{l} c_i$![]() , $d=\sum _{i=1}^{l} d_i$
, $d=\sum _{i=1}^{l} d_i$![]() and $e=\sum _{i=1}^{l} e_i$
and $e=\sum _{i=1}^{l} e_i$![]() . By corollary 2.8 we have the following inequalities:
. By corollary 2.8 we have the following inequalities:
where $\epsilon _k$![]() is a small constant depending on $k$
is a small constant depending on $k$![]() such that $\epsilon _k \to 0$
such that $\epsilon _k \to 0$![]() as $k\to \infty$
as $k\to \infty$![]() . Thus we have the inequalities $\frac {1}{kl}(c + 2a) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$
. Thus we have the inequalities $\frac {1}{kl}(c + 2a) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$![]() and $\frac {1}{kl}(d + 2b) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$
and $\frac {1}{kl}(d + 2b) \leq \frac {1}{3n} + \frac {2}{3} + \epsilon _k$![]() .
.
5.3 The proof of the theorem 5.1
By using lemmas 4.1 and 5.6 we prove that the log pair $(S_n,\, \lambda D)$![]() is log canonical, that is, $\delta (S_n) \geq \frac {1}{\lambda } > 1$
is log canonical, that is, $\delta (S_n) \geq \frac {1}{\lambda } > 1$![]() .
.
Lemma 5.6 Let $D$![]() be an anti-canonical ${{\mathbb {Q}}}$
be an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type on $S_n$
-basis type on $S_n$![]() with $k\gg 0$
with $k\gg 0$![]() . The log pair $(S_n,\, \lambda D)$
. The log pair $(S_n,\, \lambda D)$![]() is log canonical at the point $\mathsf p_w$
is log canonical at the point $\mathsf p_w$![]() .
.
Proof. Let $D$![]() be an anti-canonical ${{\mathbb {Q}}}$
be an anti-canonical ${{\mathbb {Q}}}$![]() -divisor of $k$
-divisor of $k$![]() -basis type on $S_n$
-basis type on $S_n$![]() with $k\gg 0$
with $k\gg 0$![]() . Then there is a basis $\{s_1,\,\ldots,\,s_l\}$
. Then there is a basis $\{s_1,\,\ldots,\,s_l\}$![]() of the space $H^{0}(S_n,\, \mathcal {O}_{S_n}(k))$
of the space $H^{0}(S_n,\, \mathcal {O}_{S_n}(k))$![]() such that
such that
where $D_i$![]() is the effective divisor of the section $s_i$
is the effective divisor of the section $s_i$![]() for each $i$
for each $i$![]() . In the open set $U$
. In the open set $U$![]() , the effective divisor $\sum _{i=1}^{l} D_i$
, the effective divisor $\sum _{i=1}^{l} D_i$![]() is given by the equation $s{:=} \prod _{i=1}^{l} s_i(x,\,y,\,z,\,t,\,1) = 0$
is given by the equation $s{:=} \prod _{i=1}^{l} s_i(x,\,y,\,z,\,t,\,1) = 0$![]() . We consider the Newton polygon $N$
. We consider the Newton polygon $N$![]() of $\sigma ^{*}(s)$
of $\sigma ^{*}(s)$![]() in the coordinates $(u,\,v)$
in the coordinates $(u,\,v)$![]() of ${{\mathbb {R}}}^{2}$
of ${{\mathbb {R}}}^{2}$![]() . Let $\Lambda$
. Let $\Lambda$![]() be the edge of the Newton polygon $N$
be the edge of the Newton polygon $N$![]() that intersects the diagonal line given by $u=v$
that intersects the diagonal line given by $u=v$![]() . If the edge $\Lambda$
. If the edge $\Lambda$![]() is either vertical or horizontal then the log canonical threshold of the log pair $(S_n,\, \sum _{i=1}^{l} D_i)$
is either vertical or horizontal then the log canonical threshold of the log pair $(S_n,\, \sum _{i=1}^{l} D_i)$![]() at $\mathsf p_w$
at $\mathsf p_w$![]() is determined by the edge $\Lambda$
is determined by the edge $\Lambda$![]() (see [Reference Park and Won14, step A]). By lemma 5.4 the point corresponding to the monomial $z^{c+2a}t^{d+2b}$
(see [Reference Park and Won14, step A]). By lemma 5.4 the point corresponding to the monomial $z^{c+2a}t^{d+2b}$![]() is contained in the Newton polygon $N$
is contained in the Newton polygon $N$![]() . Thus we have
. Thus we have
By lemma 5.5 we then have
Thus the log pair $(S_n,\, \lambda D)$![]() is log canonical at the point $\mathsf p_w$
is log canonical at the point $\mathsf p_w$![]() .
.
Suppose that the edge $\Lambda$![]() is neither vertical nor horizontal. By [Reference Park and Won14, step C], we can obtain a power series $\eta$
is neither vertical nor horizontal. By [Reference Park and Won14, step C], we can obtain a power series $\eta$![]() applying a change of coordinates $z\mapsto z-\sum _{j>0} \alpha _j t^{j}$
applying a change of coordinates $z\mapsto z-\sum _{j>0} \alpha _j t^{j}$![]() and $t\mapsto t$
and $t\mapsto t$![]() to $\sigma ^{*}(s)$
to $\sigma ^{*}(s)$![]() such that the edge $\Lambda '$
such that the edge $\Lambda '$![]() of the Newton polygon $N'$
of the Newton polygon $N'$![]() of the power series $\eta$
of the power series $\eta$![]() that intersects the diagonal line given by $u=v$
that intersects the diagonal line given by $u=v$![]() determine the log canonical threshold of the log pair $(S_n,\, \sum _{i=1}^{l} D_i)$
determine the log canonical threshold of the log pair $(S_n,\, \sum _{i=1}^{l} D_i)$![]() at $\mathsf p_w$
at $\mathsf p_w$![]() . By lemma 5.4 the point corresponding to the monomial $z^{c+2a}t^{d+2b}$
. By lemma 5.4 the point corresponding to the monomial $z^{c+2a}t^{d+2b}$![]() is contained in the Newton polygons $N'$
is contained in the Newton polygons $N'$![]() of the power series $\eta$
of the power series $\eta$![]() , we have
, we have
By lemma 5.5 we then have
Therefore the log pair $(S_n,\, \lambda D)$![]() is log canonical at the point $\mathsf p_w$
is log canonical at the point $\mathsf p_w$![]() .
.
Acknowledgments
The authors are very grateful to the referee for valuable suggestions and comments. I.-K. Kim and J. Won were supported by NRF grant funded by the Korea government (MSIT) (I.-K. Kim: NRF-2020R1A2C4002510, J. Won: NRF-2020R1A2C1A01008018). J. Won was supported by the Ewha Womans University Research Grant of 2022.