A Cauchy problem for the Tartar equation
Published online by Cambridge University Press: 12 July 2007
Abstract
In order to study weak limits of quadratic expressions of oscillatory solutions of partial differential equations, there was proposed a construction of H-measures defined on the space of positions and frequencies. The present paper is devoted to the investigation of the Tartar equation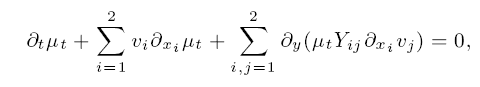 which describes the evolution of the H-measure μt associated with a sequence of oscillatory solutions of the linear transport equation
which describes the evolution of the H-measure μt associated with a sequence of oscillatory solutions of the linear transport equation
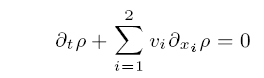 in cases when a given solenoidal velocity field v(x, t) is sufficiently smooth. Here, (t, x, y) ∈ (0, T) × Ω × S1, 0 < T < +∞, Ω is a bounded open subset of R2 and S1 is the unit circle in R2, given coefficients Yij = Yij(y) are infinitely smooth.
in cases when a given solenoidal velocity field v(x, t) is sufficiently smooth. Here, (t, x, y) ∈ (0, T) × Ω × S1, 0 < T < +∞, Ω is a bounded open subset of R2 and S1 is the unit circle in R2, given coefficients Yij = Yij(y) are infinitely smooth.
Assuming that v belongs to 
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 132 , Issue 2 , April 2002 , pp. 395 - 418
- Copyright
- Copyright © Royal Society of Edinburgh 2002
- 2
- Cited by


