We look for standing waves for nonlinear Schrödinger equations

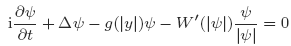
with cylindrically symmetric potentials g vanishing at infinity and non-increasing, and a C1 nonlinear term satisfying weak assumptions. In particular, we show the existence of standing waves with non-vanishing angular momentum with prescribed L2 norm. The solutions are obtained via a minimization argument, and the proof is given for an abstract functional which presents a lack of compactness. As a specific case, we prove the existence of standing waves with non-vanishing angular momentum for the nonlinear hydrogen atom equation.