Article contents
Generalized Hensel's lemma
Published online by Cambridge University Press: 20 January 2009
Extract
Let (K, v) be a complete, rank-1 valued field with valuation ring Rv, and residue field kv. Let vx be the Gaussian extension of the valuation v to a simple transcendental extension K(x) defined by 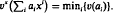 The classical Hensel's lemma asserts that if polynomials F(x), G0(x), H0(x) in Rv[x] are such that (i) vx(F(x) – G0(x)H0(x)) > 0, (ii) the leading coefficient of G0(x) has v-valuation zero, (iii) there are polynomials A(x), B(x) belonging to the valuation ring of vx satisfying vx(A(x)G0(x) + B(x)H0(x) – 1) > 0, then there exist G(x), H(x) in K[x] such that (a) F(x) = G(x)H(x), (b) deg G(x) = deg G0(x), (c) vx(G(x)–G0(x)) > 0, vx(H(x) – H0(x)) > 0. In this paper, our goal is to prove an analogous result when vx is replaced by any prolongation w of v to K(x), with the residue field of wa transcendental extension of kv.
The classical Hensel's lemma asserts that if polynomials F(x), G0(x), H0(x) in Rv[x] are such that (i) vx(F(x) – G0(x)H0(x)) > 0, (ii) the leading coefficient of G0(x) has v-valuation zero, (iii) there are polynomials A(x), B(x) belonging to the valuation ring of vx satisfying vx(A(x)G0(x) + B(x)H0(x) – 1) > 0, then there exist G(x), H(x) in K[x] such that (a) F(x) = G(x)H(x), (b) deg G(x) = deg G0(x), (c) vx(G(x)–G0(x)) > 0, vx(H(x) – H0(x)) > 0. In this paper, our goal is to prove an analogous result when vx is replaced by any prolongation w of v to K(x), with the residue field of wa transcendental extension of kv.
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 42 , Issue 3 , October 1999 , pp. 469 - 480
- Copyright
- Copyright © Edinburgh Mathematical Society 1999
References
REFERENCES
- 2
- Cited by


