Hostname: page-component-6bf8c574d5-xtvcr Total loading time: 0 Render date: 2025-02-21T21:10:13.296Z Has data issue: false hasContentIssue false
  • English
  • Français

Existentially closed locally cofinite groups

Published online by Cambridge University Press:  20 January 2009

Felix Leinen
Felix Leinen
Affiliation:
Fachbereich 17-MathematikJohannes Gutenberg-UniversitätSaarstr. 21D-6500 Mainz, Germany
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 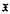be a class of finite groups. Then a c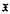-group shall be a topological group which has a fundamental system of open neighbourhoods of the identity consisting of normal subgroups with 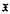-factor groups and trivial intersection. In this note we study groups which are existentially closed (e.c.) with respect to the class Lc 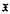of all direct limits of c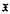-groups (where 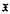satisfies certain closure properties). We show that the so-called locally closed normal subgroups of an e.c. Lc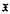-group are totally ordered via inclusion. Moreover it turns out that every ∀2-sentence, which is true for countable e.c. L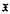-groups, also holds for e.c. Lc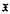-groups. This allows it to transfer many known properties from e.c. L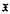-groups to e.c. Lc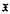-groups.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 35 , Issue 2 , June 1992 , pp. 233 - 253
Copyright
Copyright © Edinburgh Mathematical Society 1992

References

REFERENCES

1.Baumslag, B., On the residual finiteness of generalized free products of nilpotent groups, Trans. Amer. Math. Soc. 106 (1963), 193–209.CrossRefGoogle Scholar
2.Baumslag, B. and Tretkoff, M., Residually finite HNN-extensions, Comm. Algebra 6 (1978), 179–194.Google Scholar
3.Dugundji, J., Topology (Allyn and Bacon, Boston 1966).Google Scholar
4.Gerstenhaber, M. and Rothaus, O. S., The solution of sets of equations in groups, Proc. Nat. Acad. Sci USA 48 (1962), 1531–1533.Google Scholar
5.Golod, E. S., On nil-algebras and finitely approximable p-groups, AMS Translations (2) 48 (1965), 103–106.Google Scholar
6.Grigorchuk, R. I., Construction of p-groups of intermediate growth that have a continuum of quotient groups, Algebra and Logic 23 (1984), 265–273.CrossRefGoogle Scholar
7.Hall, P., Some constructions for locally finite groups, J. London Math. Soc. 34 (1959), 305–319.CrossRefGoogle Scholar
8.Hartley, B., Profinite and residually finite groups, Rocky Mountain Math. J. 7 (1977), 193–217.Google Scholar
9.Haug, F., Existenziell abgeschlossene LFC-Gruppen (Dissertation, Tübingen 1987).Google Scholar
10.Hewitt, E. and Ross, K. A., Abstract harmonic analysis I (Springer, Berlin, 1963).Google Scholar
11.Hiogins, P. J., An introduction to topological groups (LMS Lecture Note Series 15, Cambridge, 1974).Google Scholar
12.Higman, G., Amalgams of p-groups, J. Algebra 1 (1964), 301–305.Google Scholar
13.Hirschfeld, J. and Wheeler, W. H., Forcing, arithmetic, division rings (Springer Lecture Notes 454, Berlin, 1975).Google Scholar
14.Kegel, O. H. and Wehrfritz, B. A. F., Locally finite groups (North-Holland, Amsterdam, 1973).Google Scholar
15.Leinen, F., Existentially closed groups in locally finite group classes, Comm. Algebra 13 (1985), 1991–2024.CrossRefGoogle Scholar
16.Leinen, F., Existentially closed L -groups, Rend. Sem. Mat. Univ. Padova 75 (1986), 191–226.Google Scholar
17.Leinen, F., ExistentiaDy closed locally finite p-groups, J. Algebra 103 (1986), 160–183.Google Scholar
18.Leinen, F., Uncountable existentially closed groups in locally finite group classes, Glasgow J. Math. 32 (1990), 153–163.CrossRefGoogle Scholar
19.Leinen, F. and E, R., Phillips, Existentially closed central extensions of locally finite p-groups, Math. Proc. Cambridge Philos. Soc. 100 (1986), 281–301.Google Scholar
20.Lyndon, R. C. and Schupp, P. E., Combinatorial group theory (Springer, Berlin, 1977).Google Scholar
21.Maier, B., Existenziell abgeschlossene lokal endliche p-Gruppen, Arch. Math. 37 (1981), 113–128.CrossRefGoogle Scholar
22.Neumann, B. H., On amalgams of periodic groups, Proc. Roy. Soc. London (A) 255 (1960), 477–489.Google Scholar
23.Ribes, L., On amalgamated products of profinite groups, Math. Z. 123 (1971), 357–364.Google Scholar
24.Zel'manov, E. I., Solution of the restricted Burnside problem for groups of odd exponent, Math. USSR Izvestiya 36 (1991), 41–60.Google Scholar
25.Zel'Manov, E. I., Solution of the restricted Burnside problem for 2-groups, Mat. Sb., to appear.Google Scholar