No CrossRef data available.
Published online by Cambridge University Press: 12 October 2020
von Neumann [(1951). Various techniques used in connection with random digits. National Bureau of Standards Applied Math Series 12: 36–38] introduced a simple algorithm for generating independent unbiased random bits by tossing a (possibly) biased coin with unknown bias. While his algorithm fails to attain the entropy bound, Peres [(1992). Iterating von Neumann's procedure for extracting random bits. The Annals of Statistics 20(1): 590–597] showed that the entropy bound can be attained asymptotically by iterating von Neumann's algorithm. Let $b(n,p)$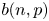 denote the expected number of unbiased bits generated when Peres’ algorithm is applied to an input sequence consisting of the outcomes of $n$
denote the expected number of unbiased bits generated when Peres’ algorithm is applied to an input sequence consisting of the outcomes of $n$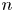 tosses of the coin with bias $p$
tosses of the coin with bias $p$ . With $p=1/2$
. With $p=1/2$ , the coin is unbiased and the input sequence consists of $n$
, the coin is unbiased and the input sequence consists of $n$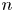 unbiased bits, so that $n-b(n,1/2)$
unbiased bits, so that $n-b(n,1/2)$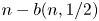 may be referred to as the cost incurred by Peres’ algorithm when not knowing $p=1/2$
may be referred to as the cost incurred by Peres’ algorithm when not knowing $p=1/2$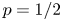 . We show that $\lim _{n\to \infty }\log [n-b(n,1/2)]/\log n =\theta =\log [(1+\sqrt {5})/2]$
. We show that $\lim _{n\to \infty }\log [n-b(n,1/2)]/\log n =\theta =\log [(1+\sqrt {5})/2]$ (where $\log$
(where $\log$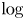 is the logarithm to base $2$
is the logarithm to base $2$ ), which together with limited numerical results suggests that $n-b(n,1/2)$
), which together with limited numerical results suggests that $n-b(n,1/2)$ may be a regularly varying sequence of index $\theta$
may be a regularly varying sequence of index $\theta$ . (A positive sequence $\{L(n)\}$
. (A positive sequence $\{L(n)\}$ is said to be regularly varying of index $\theta$
is said to be regularly varying of index $\theta$ if $\lim _{n\to \infty }L(\lfloor \lambda n\rfloor )/L(n)=\lambda ^\theta$
if $\lim _{n\to \infty }L(\lfloor \lambda n\rfloor )/L(n)=\lambda ^\theta$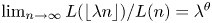 for all $\lambda > 0$
for all $\lambda > 0$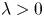 , where $\lfloor x\rfloor$
, where $\lfloor x\rfloor$ denotes the largest integer not exceeding $x$
denotes the largest integer not exceeding $x$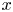 .) Some open problems on the asymptotic behavior of $nh(p)-b(n,p)$
.) Some open problems on the asymptotic behavior of $nh(p)-b(n,p)$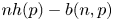 are briefly discussed where $h(p)=-p\log p- (1-p)\log (1-p)$
are briefly discussed where $h(p)=-p\log p- (1-p)\log (1-p)$ denotes the Shannon entropy of a random bit with bias $p$
denotes the Shannon entropy of a random bit with bias $p$ .
.