Introduction
Clinopyroxene minerals generally contain various transition-metal ions. In calcium clinopyroxenes, Tschermak-type substitutions (VIM 2+ + IVSi4+ ↔ VIM 3+ + IVM’3+) play an essential role in the incorporation of trivalent transition-metal ions. Thus, these clinopyroxenes have been investigated repeatedly in order to understand the: (1) stability of clinopyroxenes with calcium Tschermak-type end-member compositions; (2) solubility of each end-member component in diopside; (3) distribution of trivalent transition-metal ions between octahedral and tetrahedral sites in calcium clinopyroxene; and (4) effect of the Tschermak-type substitutions on the pyroxene structure. Examples of this research in the literature include: synthetic clinopyroxenes in the join CaMgSi2O6 (diopside: Di)–CaFe3+AlSiO6 (esseneite: Ess) (Hijikata, Reference Hijikata1968; Hijikata and Onuma, Reference Hijikata and Onuma1969; Huckenholz et al., Reference Huckenholz, Lindhuber and Springer1974; Oba and Onuma, Reference Oba and Onuma1978; Ghose et al., Reference Ghose, Okamura and Ohashi1986; Kurepin et al., Reference Kurepin, Polshin and Alibekov1981; Shinno and Maeda, Reference Shinno and Maeda1989; and others); in the join Di–CaFe3+2SiO6 (Huckenholz et al., Reference Huckenholz, Schairer and Yoder1967; Hafner and Huckenholz, Reference Hafner and Huckenholz1971); in the join Di–CaTiAl2O6 (‘titan pyroxene’: Tp) (Yagi and Onuma, Reference Yagi and Onuma1967; Onuma et al., Reference Onuma, Hijikata and Yagi1968); in the join Di–Ess–Tp (Akasaka and Onuma, Reference Akasaka and Onuma1980; Akasaka et al., Reference Akasaka, Ohashi, Shinno, Gupta, Onuma and Arima1997); in the join Ess–Tp (Onuma and Akasaka, Reference Onuma and Akasaka1980; Akasaka, Reference Akasaka1983); in the join Di–Tp–CaAl2SiO6 (kushiroite: Ksh) (Yang, Reference Yang1973, Reference Yang1976; Onuma and Kimura, Reference Onuma and Kimura1978); in the join Di–Ksh–Ess (Onuma et al., Reference Onuma, Akasaka and Yagi1981); synthetic Ca(V3+,Al)AlSiO6-pyroxene (Ohashi and Sato, Reference Ohashi, Sato and Ohashi2003) and CaSc3+AlSiO6-pyroxene (Ohashi and Ii, Reference Ohashi and Ii1978).
As well as the case of clinopyroxenes containing trivalent transition-metal ions, such as Fe3+, V3+ and Sc3+, by calcium Tschermak-type substitutions, diopside incorporates Cr3+ ions as calcium Tschermak-type CaCr3+Cr3+SiO6 (chromium Tschermak's component: CrTs) or CaCr3+AlSiO6 (chromium–aluminium Tschermak's component: CrAlTs) components. Ikeda and Yagi (Reference Ikeda and Yagi1977) determined the maximum solubility of CrTs component in diopside to be 6.7 wt.% at 1 atm and 940°C, and found that the CrTs-bearing diopsides are always blue in colour, in contrast to the green colour of clinopyroxene in the join Di–NaCrSi2O6 (lkeda and Yagi Reference Ikeda and Yagi1972; Ikeda and Ohashi, Reference Ikeda and Ohashi1974) and natural Cr-bearing diopside (e.g. Naumov et al., Reference Naumov, Kamenetsky, Thomas, Kononkova and Ryzhenko2008). They suggested that the blue colour of the CrTs-bearing diopside is due to tetrahedral Cr3+ ions in a low-spin state. The low-spin state of Cr3+ ions in CrTs-bearing diopside was proven using magnetic susceptibility measurements by Ikeda and Yagi (Reference Ikeda and Yagi1978). Furthermore, Ikeda and Yagi (Reference Ikeda and Yagi1982) synthesised diopsides in the join Di−CrAlTs from glasses containing a CrAlTs component up to 5 wt.% (4.48 mol.%), based on the estimated maximum solubility of the CrAlTs component in diopside of ca. 14 wt.% (ca. 13 mol.%) at 1200°C and 1 atm after Dickey et al. (Reference Dickey, Yoder and Schairer1971), and found that Di99CrAlTs1-, Di97CrAlTs3-, and Di95CrAlTs5-diopsides crystallised at temperatures <1160°C are blue in colour, whereas those synthesised at temperatures >1160°C are green in colour. According to the results of the crystal field spectral analysis of the CrAlTs-bearing diopsides by Ikeda and Yagi (Reference Ikeda and Yagi1982), both octahedrally coordinated high-spin (HS) and tetrahedrally coordinated low-spin (LS) Cr3+ ions are present in the blue diopside, whereas, in the green diopside, nearly all Cr3+ ions are in the HS state and are present in the octahedral sites. Moreover, they indicated that the occupancy of Cr3+ at the tetrahedral sites decreases with increasing crystallisation temperature from 800°C to 1160°C, and drops to nearly zero at 1160°C.
However, different interpretations for the cause of the blue colour of Cr-bearing diopside have also been proposed: Dickey et al. (Reference Dickey, Yoder and Schairer1971) and Mao et al. (Reference Mao, Bell and Dickey1972) explained that the blue colour of the synthetic blue diopside is mainly due to the valence change of Cr3+ ions to Cr2+; Schreiber (Reference Schreiber1977, Reference Schreiber1978) and Burns (Reference Burns1993) argued that the blue colour is due to Cr4+ ions. Therefore, in order to solve the discrepancy of the above views on the cause of the blue colour of synthetic Cr-bearing diopside, additional data on the distribution of Cr3+ ions in the octahedral and tetrahedral sites of Cr3+-bearing diopside is required. No structural evidence of different distribution of Cr3+ ions in the octahedral and tetrahedral sites between the blue and green diopsides has been reported because of the lack of structural data on the CrAlTs-bearing diopsides. The purpose of our investigation is to determine the distribution of Cr3+ in the CrAlTs-bearing diopside by powder X-ray diffraction for comparison with the results from crystal field spectroscopy in Ikeda and Yagi (Reference Ikeda and Yagi1982), and to clarify the influence of Cr3+ and Al3+ distribution between the octahedral and tetrahedral sites on the crystal structure. In order to achieve this aim, a diopside with a composition of Di95CrAlTs5 (mol.%) was crystallised at 800, 1000 and 1200°C from glass of this composition, and its crystal structure was refined using Rietveld refinement of the powder X-ray diffraction data.
Experimental method
Samples
In the present study, a CrAlTs-bearing diopside with a composition of Di95CrAlTs5 in mol.% was synthesised from a glass with this composition, for two reasons: (1) the quantity of Cr in this composition is enough for the determination of Cr population in the octahedral and tetrahedral sites in the diopside structure; and (2) the results from the CrAlTs-bearing diopside with this composition can be compared with that of Di95CrAlTs5 (in wt.%)-diopside in Ikeda and Yagi (Reference Ikeda and Yagi1982).
Reagent-grade chemicals CaCO3, MgO, Al2O3, and Cr2O3, and synthetic pure cristobalite were used as the source materials. CaCO3 and Cr2O3 were dried at 110°C for 24 hr, and MgO and Al2O3 were ignited at 1400 and 1350°C, respectively, for 3 hr. Cristobalite was produced by heating of a regent grade amorphous SiO2 at 1350°C for 3 hr and then rapid cooling. A mixture consisting of synthetic cristobalite and CaCO3, MgO, Al2O3, and Cr2O3, formulated with a composition of Di95CrAlTs5 in mol.%, was melted in air in a Pt-crucible at 1590–1600°C using a SILICONIT high-temperature Muffle furnace with an electronic temperature controller (Department of Geoscience, Shimane University). Homogenous glass was obtained after two fusions with intermediate quenching and grinding. The glass exhibits a green colour (Fig. 1a) and has the same chemical composition as the oxide mixture with Di95CrAlTs5 (mol.%) composition within the experimental and analytical error (Table 1). Powdered glass was crystallised at 800°C in air for 2 days using a Muffle furnace, with a heating element of Kanthal heating wire and an electronic temperature controller (Department of Geoscience, Shimane University). The product was a single-phase of blue diopside with a trace amount of wollastonite-2M, and was used as a starting material for the heating experiments at 800, 1000 and 1200°C.
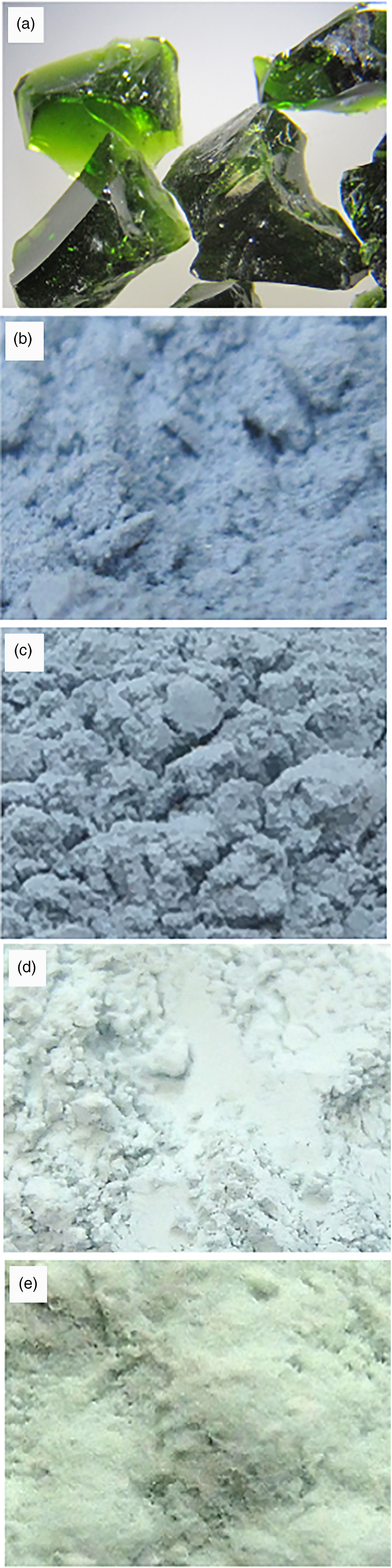
Fig. 1. Photographs of the glass and synthetic diopsides with Di95CrAlTs5 composition: (a) glass; (b) Di95CrAlTs5-diopside synthesised at 800°C for 19 days of Run No. 1; (c) 1000°C for 7 days of Run No. 2; (d) 1200°C for 3 days of Run No. 3; and (e) 1200°C for 7 days of Run No. 4. Width of view = 1 cm.
Table 1. EMPA data (wt.%) of a glass prepared from an oxide mixture with the composition of Di95CrAlTs5 (mol.%)*.
* Di: CaMgSi2O6 component; CrAlTs: CaCr3+AlSiO6 component.
By heating the starting material at 800°C for another 19 days, the run product at 800°C was obtained. The run products at 1000 and 1200°C were obtained by heating of the starting material for 7 days.
Chemical analysis
The chemical compositions of the glass and the diopsides were analysed using a JEOL JXA-8530F field emission electron microprobe analyser (EMPA) at the Department of Geoscience, Shimane University. The EMPA was operated at an accelerating voltage of 15 kV with a beam current of 20 nA and a beam diameter of 1 µm. Standard materials used were synthetic oxides: SiO2 for Si, Al2O3 for Al, Cr2O3 for Cr, and MgO for Mg; and synthetic CaSiO3 for Ca. The ZAF method was applied for data correction.
Crystal field spectra measurement
In order to compare the crystal field spectra of synthetic blue and green diopsides in the present study with those by Ikeda and Yagi (Reference Ikeda and Yagi1982), diffused reflectance spectra of the diopsides in the 300–1600 nm region were measured with a Shimadzu UV-vis-NIR Recording Spectrophotometer Model UV-3100, equipped with an integrating sphere, at the Department of Material Science, Shimane University. Reflectance spectra were plotted against the semi-absorbance scale.
Powder X-ray diffraction data collection and Rietveld analysis
Each fine powder sample of the diopside synthesised at 800, 1100 and 1200°C was mounted in a glass holder with a 20 mm × 18 mm × 0.5 mm cavity by loading the powder from the front of the holder.
Powder X-ray diffraction data were collected using a RIGAKU RINT diffractometer system at the Department of Geoscience, Shimane University, equipped with 1° divergence and scatter slits, a 0.15 mm receiving slit and a curved graphite diffracted-beam monochromator. The Cu X-ray tube generator was operated at 35 kV and 25 mA. For phase identification, the powder X-ray diffraction pattern was taken between 5° and 60° in 2θ using the continuous scan mode with a scan speed of 2° in 2θ per minute. The X-ray diffraction data for Rietveld analysis were measured between 5° and 120° in 2θ using the step-scan mode with a step interval of 0.02° and a step counting time of 4 s.
The crystal structure was refined using the Rietveld program RIETAN-FP (Izumi and Momma, Reference Izumi and Momma2007). The peaks were defined using the ‘Modified split pseudo-Voigt’ function in the RIETAN-FP program. The preferred orientation was corrected with the March–Dollase function (Dollase, Reference Dollase1986). A nonlinear least-squares calculation using the Marquardt method was followed by the conjugate-direction method to check the convergence at a local minimum (Izumi, Reference Izumi and Young1993). The VESTA program of Momma and Izumi (Reference Momma and Izumi2011) was used for calculation of the distortion parameters defined by Robinson et al. (Reference Robinson, Gibbs and Ribbe1971) and the volumes of octahedral and tetrahedral coordination polyhedra. The quadratic elongation parameter <λtet> of the TO4-tetrahedron is defined as <λtet> =
$\mathop \sum \nolimits_{i = 1}^4 \lpar {l_i/l_0} \rpar ^2/4{\rm \;} $(l i is the length of the line in the strained state; l 0 is the centre-to-vertex distance for an tetrahedron with D4h symmetry whose volume is equal to that of the strained tetrahedron with bond lengths l i); the variance of the tetrahedral angle as σθ(tet)2 = 
$\mathop \sum \nolimits_{i = 1}^6 \lpar {{\rm \theta}_i-109.47^\circ} \rpar ^2/5$ (θi; O–T–O angle); the quadratic elongation parameter <λoct> of the M1O6-octahedron is defined as <λoct> =
$\mathop \sum \nolimits_{i = 1}^6 \lpar {l_i/l_0} \rpar ^2/6\; $(l i is the length of the line in the strained state; l 0 is the centre-to-vertex distance for an octahedron with O h symmetry whose volume is equal to that of the strained octahedron with bond lengths l i); and the variance of the octahedral angle is defined as σθ(oct)2 = 
$\mathop \sum \nolimits_{i = 1}^{12} \lpar {{\rm \theta}_i-90^\circ} \rpar ^2/11$ (θi; O–M1–O angle).
Results
Samples
The run product obtained by heating the blue diopside starting material at 800°C for 19 days was blue in colour (Table 2 Run No. 1; Fig. 1b). The product at 1000°C for 7 days also remained blue (Table 2 Run No. 2; Fig. 1c). By heating the blue diopside starting material at 1200°C, the blue diopside changed to a bluish green product after 3 days (Table 2 Run No. 3; Fig. 1d) and to green after 7 days (Table 2 Run No. 4; Fig. 1e). Using powder X-ray diffraction analysis, all products were identified as a diopside single phase with trace amounts of wollastonite-2M (Table 2).
Table 2. Run products* at 800, 1000 and 1200°C in air.
* Starting material: Blue diopside crystallised from the Di95CrAlTs5-glass at 800°C for 2 days.
The crystal field spectra of the blue diopsides synthesised at 800 and 1000°C (Nos. 1 and 2 in Fig. 2, respectively) and of the green diopside at 1200°C (No. 3 in Fig. 2) resemble those of the blue and green Di95CrAlTs5-diopsides (in wt.%) reported by Ikeda and Yagi (Reference Ikeda and Yagi1982), respectively.
Fig. 2. Crystal field spectra of blue diopside of: (1) Run No.1 at 800°C for 19 days; (2) blue diopside of Run No. 2 at 1000°C for 7 days; and (3) green diopside of Run No. 4 at 1200°C for 7 days.
All diopsides have a stoichiometric composition of Di95CrAlTs5 within the experimental and analytical errors (Table 3).
Table 3. EMPA data (wt.%) of the synthesised diopside solid solutions.
X-ray Rietveld analysis
The Rietveld analysis of the X-ray diffraction data was carried out for the diopsides crystallised at 800, 1000 and 1200°C. As a trace amount of wollastonite-2M was detected in the run products, the Rietveld refinement was performed for both diopside and wollastonite-2M. Details of the X-ray data collection are listed in Table 4. The X-ray single-crystal structural data of diopside with a space group C2/c by Cameron et al. (Reference Cameron, Sueno, Prewitt and Papike1973) and of wollastonite-2M with P21/a by Trojer (Reference Trojer1968) were used as structural models of these minerals for the Rietveld refinement. As shown in Table 4, the run products consisted of diopside with mass fractions of 96.8–99.9 wt.% and wollastonite-2M with 0.1–3.2 wt.%, indicating that the products essentially consist of single-phase diopside. In the structural refinement, occupancies of Ca at the 8-coordinated M2 site, Mg at the octahedral M1 site and Si at the tetrahedral T site were fixed to 1.0, 0.95 and 0.975, respectively, because diopside in each run product has a stoichiometric chemical composition of Di95CrAlTs5 (mol.%). Site occupancies of Cr and Al in the M1 and T sites were refined using the following constraints: Al(M1) = 1.0 – Mg(M1) – Cr(M1), Cr(T) = [0.05 – Cr(M1)]/2, and Al(T) = 1.0 – Si(T) – Cr(T). The atomic positions and isotropic atomic displacement parameters of the wollastonite-2M were fixed to the initial values. The refined unit-cell parameters, R-factors, goodness-of-fit (S = R wp/R e) and the Durbin-Watson d statistic are shown in Table 4, and the observed and calculated powder X-ray diffraction patterns of the samples are given in Fig. 3.
Fig. 3. Observed and calculated powder X-ray diffraction patterns for the run products of: (a) Run No. 1 at 800°C for 19 days; (b) Run No. 2 at 1000°C for 7 days; (c) Run No. 3 at 1200°C for 3 days; and (d) Run No. 4 at 1200°C for 7 days. The crosses are the observed data, the solid line is the calculated pattern, and the vertical bars mark all possible Bragg reflections (Kα1 and Kα2) for diopside with space group C2/c (upper bars) and wollastonite-2M with space group P21/a (lower bars). The difference between the observed and calculated pattern is shown at the bottom.
Table 4. Details of data collection and structure refinement*.
* Abbreviations: R p – R-pattern; R wp – R-weighted pattern; R e – R-expected; S – Goodness-of-fit (= R wp/R e); R B – R-Bragg factor; R F – R-structure factor (Young, Reference Young and Young1993). D–W d – Durbin–Watson d statistic (Hill and Flack, Reference Hill and Flack1987). Woll. – Wollastonite.
The refined site occupancies, atomic positions and atomic displacement parameters of the Di95CrAlTs5-diopsides crystallised at 800, 1000 and 1200°C are shown in Table 5, and selected bond lengths are given in Table 6. Errors are shown by estimated standard deviations of 1σ.
Table 5. Site occupancies (occ.), atomic coordinates (x, y, z) and isotropic atomic displacement parameters (U eq in Å2) of the synthesised diopsides.
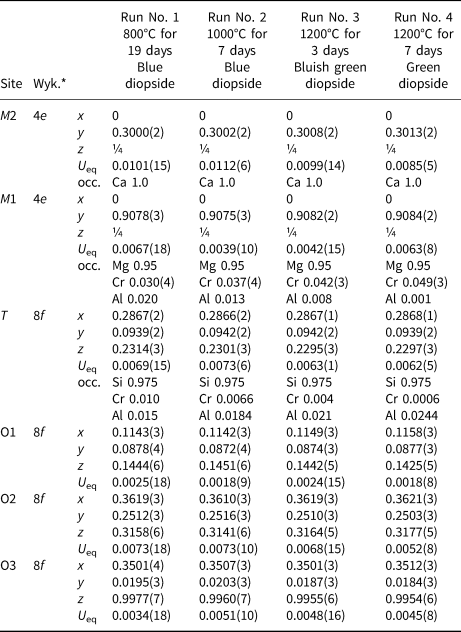
* Wyk. – Wyckoff site notation.
Table 6. Selected bond lengths (Å).
Resulting site occupancies in the M1 and T sites of the Di95CrAlTs5-diopside are: (1) M1[Mg0.95Cr0.030(4)Al0.020] and T[Si0.975Cr0.010Al0.015] for the blue diopside of Run No. 1 at 800°C for 19 days; (2) M1[Mg0.95Cr0.037(4)Al0.013] and T[Si0.975Cr0.0066Al0.0184] for the blue diopside of Run No. 2 at 1000°C for 7 days; (3) M1[Mg0.95Cr0.042(3)Al0.008] and T[Si0.975Cr0.004Al0.021] for the bluish green diopside of Run No. 3 at 1200°C for 3 days; and (4) M1[Mg0.95Cr0.049(3)Al0.001] and T[Si0.975Cr0.0006Al0.0244] for the green diopside of Run No. 4 at 1200°C for 7 days. These site occupancies result in the following site populations in the M1 and T sites: (1) M1[Mg0.95Cr0.030(4)Al0.020]T[Si1.950Cr0.020Al0.030] in atoms per formula unit (O = 6) for the blue diopside of Run No. 1; (2) M1[Mg0.95Cr0.037(4)Al0.013]T[Si1.950Cr0.0132Al0.0368] for the blue diopside of Run No. 2; (3) M1[Mg0.95Cr0.042(3)Al0.008]T[Si1.950Cr0.008Al0.042] for the bluish green diopside of Run No. 3; and (4) M1[Mg0.95Cr0.049(3)Al0.001]T[Si1.950Cr0.0012Al0.0488] for the green diopside of Run No. 4. The validity of the determined site populations was examined in terms of the electrostatic valence principle after Pauling (Reference Pauling1929). In the present study, bond strength s ij (bond valence after Donnay and Allmann, Reference Donnay and Allmann1970) of the bond between cation i and anion j was calculated using a correlation between bond strength and bond length (Brown and Altermatt, Reference Brown and Altermatt1985): s ij = exp[(l o – l ij)/B], where l o is the bond valence parameter from Brown and Altermatt (Reference Brown and Altermatt1985) and Brese and O'Keeffe (Reference Brese and O'Keeffe1991); l ij is the observed bond length between cation i and anion j; and B is 0.37 Å. The valence of cation i, V i, was calculated using the equation after Brown and Shannon (Reference Brown and Shannon1973), V i =
$ \mathop \sum \nolimits_j s_{ij}$. As shown in Table 7, the bond-valence sums of the cation sites, ΣV(A), and anion site, ΣV(C), for O1 correspond approximately to the oxidation state of the sites. However, the bond-valence sums of O2 and O3 are 1.87–1.88 and 2.10–2.12 valence units (vu), deviating slightly from the ideal oxidation state. Brown and Shannon (Reference Brown and Shannon1973) previously pointed out that rather low bond-valence sums for O2 (1.87–1.88 vu), and high values for O3 (2.12–2.15 vu), are common in clinopyroxene, and attributed its reason to uncertainties of the bond lengths and to real effects such as the second-nearest neighbours which cannot be explained as yet. In recent studies (e.g. Tribaudino et al., Reference Tribaudino, Mantovani, Mezzadri, Calestani and Bromiley2018), the characteristic behaviour of O2 and O3 in C2/c pyroxene structure has been recognised: the highest local disorder of the O3 oxygen; limitations of the increase in Si–O3 bond distances by kinking limiting the tetrahedral and octahedral chains; and the under-bonded O2 oxygen atoms. The deviation of the bond-valence sums of O2 and O3 from the ideal oxidation state may be due to the state of oxygen atoms at the O2 and O3 positions.
Table 7. Bond-valence sums (in vu) of blue diopside of Run No. 1 at 800°C, blue diopside of Run No. 2 at 1000°C, bluish green diopside of Run No. 3 at 1200°C and green diopside of Run No. 4 at 1200°C.*
* Multiplicity is indicated by ×↓. ΣV(C) is the valence of bonds reaching anions. ΣV(A) is the valence of bonds emanating from cations summed over the bonded anions.
The distortion parameters defined by Robinson et al. (Reference Robinson, Gibbs and Ribbe1971) and the volumes of octahedral and tetrahedral coordination polyhedra in the blue and green diopsides are shown in Table 8 with four significant digits based on the accuracy of the bond lengths (Table 6). The TO4 tetrahedron in the blue diopside has larger volume and more regular form than that in the green diopside, whereas the M1O6 octahedron of the blue diopside is smaller in volume and more distorted than that of the green diopside.
Table 8. Average bond lengths, polyhedral volumes, quadratic elongations and bond angle variances* of TO4 and M1O6 coordination polyhedra in the synthesised diopsides of Run Nos. 1, 2 and 4.
* Polyhedral volume, quadratic elongation and bond angle variance were calculated using the VESTA program (Momma and Izumi, Reference Momma and Izumi2011). Quadratic elongation and bond angle variance are defined by Robinson et al. (Reference Robinson, Gibbs and Ribbe1971). The definitions are given in the text.
Discussion
Site populations of Cr3+ ions in the octahedral and tetrahedral sites
The interpretation that the blue colour of the synthetic blue diopside is mainly due to the valence change of chromium from a trivalent to divalent state was suggested by Dickey et al. (Reference Dickey, Yoder and Schairer1971) and was supported by the crystal field spectroscopic study of Mao et al. (Reference Mao, Bell and Dickey1972). Burns (Reference Burns1993) also mentioned that energies of several absorption bands measured by Mao et al. (Reference Mao, Bell and Dickey1972) and Ikeda and Yagi (Reference Ikeda and Yagi1982) for Cr-bearing blue diopsides are similar to those observed for aqueous Cr2+ ions. However, the Cr2+ ion is stable only at very low oxygen fugacity conditions at high temperatures (Ikeda and Yagi, Reference Ikeda and Yagi1977) and/or at very high pressures and low oxygen fugacity conditions (Burns, Reference Burns1975). Thus, Cr2+ ions are not stable in the Cr-bearing blue diopsides synthesised in air at 1 atm. On the other hand, Schreiber (Reference Schreiber1977, Reference Schreiber1978) interpreted that the blue colour of Cr-bearing diopside synthesised in his study is due to Cr4+ ions. Burns (Reference Burns1993) agreed with the Schreiber's interpretation, because: (1) the Cr4+ ion with the 3d 2 configuration has relatively high crystal field stabilisation energy (CFSE) in the tetrahedral coordination; and (2) the tetrahedral ionic radius of Cr4+ (0.41 Å, Shannon, Reference Shannon1976), is comparable to that of Al3+ (0.39 Å). However, as the blue diopside synthesised by Schreiber (Reference Schreiber1977) was crystallised from glasses with chemical compositions deviating from pyroxene stoichiometry, the consistency between the Cr distribution in his synthetic blue diopside and the estimated oxidation state of Cr cannot be evaluated in terms of the pyroxene composition and its stoichiometry.
Schreiber (Reference Schreiber1977) and Burns (Reference Burns1993) envisaged that Cr3+ enters preferentially into the octahedral sites of diopside but not into the tetrahedral sites. However, Ikeda and Yagi (Reference Ikeda and Yagi1977) proved a distribution of Cr3+ between the octahedral and tetrahedral sites by successful synthesis of stoichiometric CrTs-bearing blue diopside. Moreover, based on the crystal field spectroscopic data of the diopsides in the joins Di–CrTs (Ikeda and Yagi, Reference Ikeda and Yagi1977) and Di–CrAlTs (Ikeda and Yagi, Reference Ikeda and Yagi1982) and magnetic susceptibility data for CrTs-bearing diopside (Ikeda and Yagi, Reference Ikeda and Yagi1978), Ikeda and Yagi (Reference Ikeda and Yagi1982) found that the Cr3+ ions in the tetrahedral sites are in the low-spin state, and concluded that the blue colour of the CrTs- and CrAlTs-bearing diopsides is due to tetrahedral LS Cr3+. As the crystal field spectra of the blue and green diopsides measured in this study resemble those in Ikeda and Yagi (Reference Ikeda and Yagi1982), the results of the crystal field spectroscopic analysis by Ikeda and Yagi (Reference Ikeda and Yagi1982) are applicable to the spectra in our study.
Ikeda and Yagi (Reference Ikeda and Yagi1982) formulated a determinative curve for the site populations of Cr3+ ions in CrAlTs-bearing diopsides from the spectra of CrTs-bearing diopsides given in Ikeda and Yagi (Reference Ikeda and Yagi1977), and determined the site populations of Cr3+ ions in the octahedral and tetrahedral sites of the CrAlTs-bearing blue diopsides. As shown in Fig. 4, their result on the Di95.52CrAlTs4.48 (mol.%)-diopside indicates that the atomic ratio of Cr3+ at the tetrahedral site versus total Cr3+ is 38 at.% at 800°C, which decreases with increasing temperature and drops down to 2 at.% at temperatures >1160°C. According to our results from Rietveld analysis of the Di95CrAlTs5-diopside, the Cr3+ abundances in the tetrahedral sites at the temperatures of 800, 1000 and 1200°C are 40, 26 and 2 at.% of the total Cr3+, respectively; and in contrast, 60, 74 and 98 at.% Cr3+ of total Cr3+ distribute in the octahedral M1 sites at 800, 1000 and 1200°C, respectively (Fig. 4). Therefore, our result is very consistent with, and supports that of Ikeda and Yagi (Reference Ikeda and Yagi1982).
Fig. 4. Ratios of Cr abundance at the octahedral and tetrahedral sites against total Cr in Di95CrAlTs5 (mol.%)-diopside in this study (red closed circle mark) and Di95CrAlTs5 (wt.%)-diopside after Ikeda and Yagi (Reference Ikeda and Yagi1982) (open diamond mark). The numbers 1, 2, 3 and 4 correspond to the run numbers shown in Table 2.
Structural difference of the M1O6 octahedra and TO4 tetrahedra between the blue and green Cr3+-bearing diopsides
The distributions of Cr3+ and Al3+ between the octahedral and tetrahedral sites in the Di95CrAlTs5-diopside affect the volumes of coordination polyhedra. The larger volume of the TO4 tetrahedron of the blue diopside (2.251 and 2.258 Å3 at 800 and 1000°C, respectively) than the green diopside (2.237 Å3 at 1200°C) is attributed to the higher Cr3+:Al3+ ratio at the tetrahedral sites of the blue diopside (Cr3+:Al3+ = 0.010:0.015 and 0.007:0.018 in occupancy value at 800 and 1000°C, respectively) than the green diopside (Cr3+:Al3+ = 0.0006:0.0244 at 1200°C), because the ionic radius of the tetrahedral LS Cr3+ ion (0.46 Å, Ikeda and Yagi, Reference Ikeda and Yagi1982), is larger than that of tetrahedral Al3+ (0.39 Å). In contrast, the higher Cr3+:Al3+ ratio at the M1 site in the green diopside (Cr3+:Al3+ = 0.049:0.001 in occupancy value) than that in the blue diopside (Cr3+:Al3+ = 0.030:0.020 and 0.037:0.013 at 800 and 1000°C, respectively) results in the larger volume of the M1O6 octahedron of the green diopside (11.86 Å3) than that of the blue diopside (11.77 and 11.74 Å3 at 800 and 1000°C, respectively).
The site distortions of the M1O6 octahedron and TO4 tetrahedron are also influenced by the distributions of Cr3+ and Al3+ in the octahedral and tetrahedral sites. The TO4 tetrahedron in the blue diopside with <λtet> =1.006 and σθ(tet)2 = 24.37–24.69 is more regular in form than that of the green diopside with <λtet> = 1.007 and σθ(tet)2 = 27.94, whereas the M1O6 octahedron in the blue diopside with <λoct> =1.006 and σθ(oct)2 = 20.39–21.13 is more distorted than that of the green diopside with <λoct> = 1.005 and σθ(oct)2 = 17.58 (Table 8). Therefore, the structural properties of the Di95CrAlTs5-diopside are well correlated with the distributions of Cr3+ and Al3+ between the octahedral and tetrahedral sites.
Ikeda and Yagi (Reference Ikeda and Yagi1982) found that transformation of LS Cr3+ ions of the tetrahedral site to HS ones of the octahedral site is attained at 1160°C, and suggested that the rearrangement of Cr3+ and Al3+ ions between the octahedral and tetrahedral sites in the CrAlTs-bearing diopside depends on the kinetics of diffusion of Cr3+ and Al3+ ions in the specimens. In our heating experiment on blue diopside at 1200°C for 3 days, the colour and the Cr3+–Al distribution between the octahedral and tetrahedral sites changed to an intermediate state between the blue and green diopsides (Figs 1 and 4). Such gradual change from the blue diopside to the green diopside with increasing run durations at 1200°C is consistent with Ikeda and Yagi's suggestion.
Implications
As explained by Ikeda and Yagi (Reference Ikeda and Yagi1982), the reason why Cr-bearing blue diopsides have not been found in natural rocks is due to the slow kinetics of transformation from octahedral HS Cr3+ to tetrahedral LS Cr3+ on cooling. The lack of driving force from CFSE differences and slowness of the diffusion required for the transformation from octahedral HS Cr3+ to tetrahedral LS Cr3+ means that Cr-bearing diopside formed at high temperatures retains its Cr3+ in octahedral coordination when cooled to ambient temperature, resulting in the green colour. This interpretation is consistent with the experimental results that the green diopside is formed by heating of the blue diopside at temperatures >1160°C but a reverse reaction from the green diopside to the blue diopside has not been successful (Ikeda and Yagi, Reference Ikeda and Yagi1982).
Such behaviour of the Cr3+ ion with 3d 3 configuration is quite different from that of Fe3+ with 3d 5 configuration. In contrast to Cr3+, Fe3+ in minerals and chemical compounds is commonly in a HS state (Shannon, Reference Shannon1976), and the translation from HS state to LS state occurs only at the extremely high pressures of lower mantle conditions (e.g. Mashino et al., Reference Mashino, Ohtani, Hirao, Mitsui, Masuda, Seto, Sakai, Takahashi and Nakano2014; Sinmyo et al., Reference Sinmyo, McCammon and Dubrovinsky2017); also the case for Fe2+ (e.g. Hamada et al., Reference Hamada, Kamada, Ohtani, Mitsui, Masuda, Sakamaki, Suzuki, Maeda and Akasaka2016). Moreover, despite the similarity of ionic radii between Fe3+ (HS VIFe3+ and HS IVFe3+ are 0.645 and 0.49 Å, respectively) and Cr3+ (HS VICr3+ and LS IVCr3+ are 0.62 and 0.46 Å, respectively), the distribution scheme of Fe3+ ions between octahedral and tetrahedral sites is also different from that of Cr3+ ions: in clinopyroxene in the join Di−CaFe3+AlSiO6 synthesised at temperatures above 800°C, Fe3+ ions distribute not only in the octahedral site but also in the tetrahedral site at ambient temperatures (Kurepin et al., Reference Kurepin, Polshin and Alibekov1981; Akasaka, Reference Akasaka1983; Ghose et al., Reference Ghose, Okamura and Ohashi1986; Shinno and Maeda, Reference Shinno and Maeda1989; Akasaka et al., Reference Akasaka, Ohashi, Shinno, Gupta, Onuma and Arima1997). Because the CFSEs of Fe3+ at octahedral and tetrahedral sites are zero, the distribution of Fe3+ and Al3+ between octahedral and tetrahedral sites is not influenced by the crystal field effect, and, thus, is controlled by the volumes of coordination polyhedra and the difference in ionic radii between Fe3+ and Al3+ (Akasaka et al., Reference Akasaka, Ohashi, Shinno, Gupta, Onuma and Arima1997). Accordingly, the distinctive behaviour of Cr3+ in the diopside structure is attributed to the spin state of Cr3+ ions with 3d 3 configuration and the crystal field effect as well as its ionic radius at the octahedral and tetrahedral sites.
Conclusions
The results of our Rietveld analysis study on the Cr3+ distribution between the octahedral and tetrahedral sites in the CrAlTs-bearing blue and green diopsides are consistent with those determined by means of crystal field spectral analysis by Ikeda and Yagi (Reference Ikeda and Yagi1982): Cr3+ abundance in the tetrahedral sites decreases from 40 at.% of total Cr3+ at 800°C to 2 at.% of total Cr3+ at 1200°C through 26 at.% at 1000°C; in contrast, 60, 74 and 98 at.% Cr3+ of total Cr3+ distribute in the octahedral M1 sites at 800, 1000 and 1200°C, respectively. Such different Cr3+ distribution in the octahedral and tetrahedral sites between the blue and green diopsides causes the difference of the volumes and distortions of the M1O6 and TO4 polyhedra in these diopsides: the TO4 tetrahedron in the blue diopside has larger volume and more regular form than that in the green diopside, whereas the M1O6 octahedron of the blue diopside is smaller in volume and more distorted than that of the green diopside.
Acknowledgements
The authors thank Professor Emeritus Dr. Ko Ikeda of Yamaguchi University for his critical reading of the manuscript and valuable comments and for his help with this study, Dr. Fujio Izumi of the National institute for Materials Science, Tsukuba, and Dr. Koichi Momma of the National Museum of Nature and Science, Tokyo, for their permission to use the RIETAN-FP and VESTA programs. The authors also thank Dr. Stuart Mills, Principal Editor, two anonymous reviewers, and Dr. Helen Kerbey, Production Editor, for critical and constructive comments.
