In this paper, we study a finite connected graph which admits a quasi-monomorphism to hyperbolic spaces and give a geometric bound for the Cheeger constants in terms of the volume, an upper bound of the degree, and the quasi-monomorphism.

We provide primitive recursive bounds for the finite version of Gowers’ $c_{0}$ theorem for both the positive and the general case. We also provide multidimensional versions of these results.

This paper presents a recent result for the problem introduced eleven years ago by Fraenkel and McLeod [A diffusing vortex circle in a viscous fluid. In IUTAM Symposium on Asymptotics, Singularities and Homogenisation in Problems of Mechanics, Kluwer (2003), 489–500], but described only briefly there. We shall prove the following, as far as space allows. The vorticity ${\it\omega}$ of a diffusing vortex circle in a viscous fluid has, for small values of a non-dimensional time, a second approximation ${\it\omega}_{A}+{\it\omega}_{1}$ that, although formulated for a fixed, finite Reynolds number ${\it\lambda}$ and exact for ${\it\lambda}=0$ (then ${\it\omega}={\it\omega}_{A}$), tends to a smooth limiting function as ${\it\lambda}\uparrow \infty$. In §§1 and 2 the necessary background and apparatus are described; §3 outlines the new result and its proof.

We obtain density estimates for subsets of the $n$-dimensional integer lattice lacking four-term matrix progressions. As a consequence, we show that a subset of the grid $\{1,2,\dots ,N\}^{2}$ lacking four corners in a square has size at most $\mathit{CN}^{2}(\log \log N)^{-c}$. Our proofs involve the density increment method of Roth [J. London Math. Soc.28 (1953), 104–109] and Gowers [Geom. Funct. Anal.11(3) (2001), 465–588], together with the $U^{3}$-inverse theorem of Green and Tao [Proc. Edinb. Math. Soc. (2) 51(1) (2008), 73–153].

Two players take it in turn to claim edges from a graph $G$. The first player (“Maker”) wins if at any point he has claimed $s$ edges at a vertex without the second player (“Breaker”) having claimed a single edge at that vertex. If, by the end of play, this does not occur we say that Breaker wins. Our main aim is to show that for every $s$ there is a graph $G$ in which Maker has a winning strategy.

We address a question raised by Anderson, Hayman and Pommerenke relating to a classical result on univalent functions $f$ in the unit disc due to Spencer, and involving the size of the set of ${\it\theta}\in [-{\it\pi},{\it\pi}]$ for which we have $\log |f(r\text{e}^{\text{i}{\it\theta}})|\neq o(\log (1/(1-r)))$ as $r\rightarrow 1.$ An answer is given in terms of a certain generalized capacity, and also in terms of Hausdorff measure. Further results regarding the radial growth of univalent functions are also established, and some examples are constructed which relate to the sharpness of these results.

Let $A\subset \{1,\dots ,N\}$ be a set of prime numbers containing no non-trivial arithmetic progressions. Suppose that $A$ has relative density ${\it\alpha}=|A|/{\it\pi}(N)$, where ${\it\pi}(N)$ denotes the number of primes in the set $\{1,\dots ,N\}$. By modifying Helfgott and De Roton’s work [Improving Roth’s theorem in the primes. Int. Math. Res. Not. IMRN2011 (4) (2011), 767–783], we improve their bound and show that

$$\begin{eqnarray}{\it\alpha}\ll \frac{(\log \log \log N)^{6}}{\log \log N}.\end{eqnarray}$$

We study solvability of convolution equations for functions with discrete support in $\mathbf{R}^{n}$, a special case being functions with support in the integer points. The more general case is of interest for several grids in Euclidean space, like the body-centred and face-centred tessellations of 3-space, as well as for the non-periodic grids that appear in the study of quasicrystals. The theorem of existence of fundamental solutions by de Boor et al is generalized to general discrete supports, using only elementary methods. We also study the asymptotic growth of sequences and arrays using the Fenchel transformation.

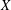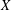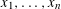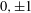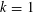Given a finite-dimensional Banach space $X$ and an Auerbach basis $\{(x_{k},x_{k}^{\ast }):1\leqslant k\leqslant n\}$ of $X$, it is proved that there exist $n+1$ linear combinations $z_{1},\ldots ,z_{n+1}$ of $x_{1},\ldots ,x_{n}$ with coordinates $0,\pm 1$, such that $\Vert z_{k}\Vert =1$, for $k=1$, 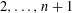$2,\ldots ,n+1$ and 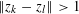$\Vert z_{k}-z_{l}\Vert >1$, for 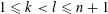$1\leqslant k<l\leqslant n+1$.

In this paper we present a new family of identities for multiple harmonic sums which generalize a recent result of Hessami Pilehrood et al  [Trans. Amer. Math. Soc. (to appear)]. We then apply it to obtain a family of identities relating multiple zeta star values to alternating Euler sums. In such a typical identity the entries of the multiple zeta star values consist of blocks of arbitrarily long 2-strings separated by positive integers greater than two while the largest depth of the alternating Euler sums depends only on the number of 2-string blocks but not on their lengths.

We study a fast method for computing potentials of advection–diffusion operators $-{\rm\Delta}+2\mathbf{b}\boldsymbol{\cdot }{\rm\nabla}+c$ with $\mathbf{b}\in \mathbb{C}^{n}$ and $c\in \mathbb{C}$ over rectangular boxes in $\mathbb{R}^{n}$. By combining high-order cubature formulas with modern methods of structured tensor product approximations, we derive an approximation of the potentials which is accurate and provides approximation formulas of high order. The cubature formulas have been obtained by using the basis functions introduced in the theory of approximate approximations. The action of volume potentials on the basis functions allows one-dimensional integral representations with separable integrands, i.e. a product of functions depending on only one of the variables. Then a separated representation of the density, combined with a suitable quadrature rule, leads to a tensor product representation of the integral operator. Since only one-dimensional operations are used, the resulting method is effective also in the high-dimensional case.

In 1940 Paul Erdős made a conjecture about the distribution of reduced residues. Here we study the distribution of $k$-tuples of reduced residues.

During the past 55 years substantial progress concerning sharp constants in Poincaré-type and Steklov-type inequalities has been achieved. Original results of H. Poincaré, V. A. Steklov and his disciples are reviewed along with the main further developments in this area.

Many classical geometric inequalities on functionals of convex bodies depend on the dimension of the ambient space. We show that this dimension dependence may often be replaced (totally or partially) by different symmetry measures of the convex body. Since these coefficients are bounded by the dimension but possibly smaller, our inequalities sharpen the original ones. Since they can often be computed efficiently, the improved bounds may also be used to obtain better bounds in approximation algorithms.

We consider the first boundary value problem for a second-order parabolic equation with variable coefficients in the domain $K\times \mathbb{R}^{n-m}$, where $K$ is an $m$-dimensional cone. The main results of the paper are pointwise estimates of the Green’s function.

We give a new proof of a recent result of Kohnen–Martin on a characterization of degree 2 Siegel cusp forms by the growth of their Fourier coefficients. Our main tools are Koecher–Maass series, Imai’s converse theorem and the theory of singular modular forms.

We give a short and elementary proof of an inverse Bernstein-type inequality found by S. Khrushchev for the derivative of a polynomial having all its zeros on the unit circle. The inequality is used to show that equally-spaced points solve a min–max–min problem for the logarithmic potential of such polynomials. Using techniques recently developed for polarization (Chebyshev-type) problems, we show that this optimality also holds for a large class of potentials, including the Riesz potentials $1/r^{s}$ with $s>0.$

Let $Q$ be an infinite subset of $\mathbb{N}$. For any ${\it\tau}>2$, denote $W_{{\it\tau}}(Q)$ (respectively $W_{{\it\tau}}$) to be the set of ${\it\tau}$ well-approximable points by rationals with denominators in $Q$ (respectively in $\mathbb{N}$). We consider the Hausdorff dimension of the liminf set $W_{{\it\tau}}\setminus W_{{\it\tau}}(Q)$ after Adiceam. By using the tools of continued fractions, it is shown that if $Q$ is a so-called $\mathbb{N}\setminus Q$-free set, the Hausdorff dimension of $W_{{\it\tau}}\setminus W_{{\it\tau}}(Q)$ is the same as that of $W_{{\it\tau}}$, i.e. $2/{\it\tau}$.

The aim of the paper is to establish estimates in weighted Sobolev spaces for the solutions of the Dirichlet problems on snowflake domains, as well as uniform estimates for the solutions of the Dirichlet problems on pre-fractal approximating domains.