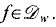In this paper we carry out a linear stability analysis within the Stokes layer that, under suitable conditions, forms at the surface of a circular cylinder in periodic orbital motion. The analysis is related to that performed by Seminara [1,2] in the Stokes layer on a torsionally oscillating cylinder and by Hall [3] in the Stokes layer at the surface of a cylinder in purely oscillatory motion. In all cases we find that the minimum critical Taylor number is located where the flow at the edge of the Stokes layer has maximum speed in each period of the motion.

A particular solution to the biharmonic equation is described which represents a slow viscous flow near a sharp edge. It shows separation streamlines which are tangential to the plate at the edge, when the dominant behaviour there is a combination of the flow around the edge (which provides zero vorticity on the plate) plus a simple linear shear.

For a fixed integer k ≥ 2 suppose we have functions Li (x) satisfying the following conditions.

Let W denote a positive, increasing and continuous function on [1, ∞]. We write to denote the Dirichlettype space of functions f that are holomorphic in the unit disc 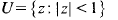and for which

Where 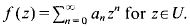If W(x) = x for all x, then is the classicial Dirichlet space for which 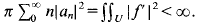Note also that for every so, by Fatu's theoreum, every function in . ha finite radial(and angular) limits a.e. on the boundary of U. The question of the existence a.e. on ∂U of certain tangential limits for functions in has been considered in [6,11], but we shall be concerned here with the radial variation

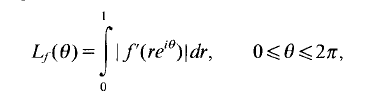
i.e., the length of the image of the ray from 0 to eiθ under the mapping w = f(z), and, in particular, with the size of the set of values of θ for which Lf(θ) can be infinite when

Ramanujan's work on the asymptotic behaviour of the hypergeometric function has been recently refined to the zero-balanced Gaussian hypergeometric function F(a, b; a + b; x) as x→1.We extend these results for F(a, b; c; x) when a, b, c>0 and c<a + b.

It is known that if A and B are nontriangular 2 × 2 non-negative integral matrices similar over the integers and –tr A ≤det A, then A and B are strongly shift equivalent. Suppose that A and B are 2 × 2 non-negative integral matrices similar over the integers. In this article it is shown that if –2 tr A≤det A <– tr A and if | det A | is not a prime, then A and B are strongly shift equivalent.

We show that a measure on ℝd is linearly rectifiable if, and only if, the lower l-density is positive and finite and agrees with the lower average l-density almost everywhere.

A random polytope, Kn, is the convex hull of n points chosen randomly, independently, and uniformly from a convex body It is shown here that, with high probability, Kn can be obtained by taking the convex hull of m = o(n) points chosen independently and uniformly from a small neighbourhood of the boundary of K.

The forms under discussion are integral positive definite quadratic forms in three variables. Such a form g is called regular if g represents every integer represented by the genus of g. This can be recast in elementary terms: g is regular if the solvability of g≡a (mod n) for every n implies the solvability of g = a.

In [CKR], Chan, Kim and Raghavan determine all universal positive ternary integral quadratic forms over real quadratic number fields. In this context, universal means that the form represents all totally positive elements of the ring of integers of the underlying field. This generalizes the usage of the term introduced by Dickson for the case of the ring of rational integers [D]. In the present paper, we will continue the investigation of quadratic forms with this property, considering positive quaternary forms over totally real number fields. The main goal of the paper is to prove that if E is a totally real number field of odd degree over the field of rational numbers, then there are at most finitely many inequivalent universal positive quaternary quadratic forms over the ring of integers of E. In fact, the stronger result will be proved that this finiteness holds for those forms which represent all totally positive multiples of any fixed totally positive integer. The necessity of the assumption of oddness of the degree of the extension for a general result of this type can be seen from the existence of universal ternary forms over certain real quadratic fields (for example, the sum of three squares over the field ℚ(√5), as first shown by Maass [M]).

For i = 1,…, n let ai be a homogeneous polynomial of degree ri(>0) in the graded polynomial ring R[x1, …, xm], or R[x] for short, where R is a commutative ring with unity and x1, …, xm are indeterminates of degree 1. Let of degree - 1 be a formal inverse of xj and let U denote the graded R[x]-module In [2, §2] we introduced a graded complex of r-modules.

A long time ago Ju. E. Vapne ([2], [3]) and, independently, the author ([4], [5]) classified those standard and complete wreath products that have faithful representations of finite degree over (commutative) fields. See [6] pages 37 40 & 150–154 for an account of this. Recently, in connection with finitary linear groups, I needed a more general wreath product. Somewhat to my surprise neither the classification nor the proof for these generalized wreath products was a straightforward translation from the standard case. The situation is intrinsically more complex and it seems worthwhile recording it separately.

Given a p-subgroup P of a finite group G we express the number of p-blocks of G with defect group P as the p-rank of a symmetric integer matrix indexed by the N(P)/P-conjugacy classes in PC(P)/P. We obtain a combinatorial criterion for P to be a defect group in G.

We investigate the distribution of the hitting time T defined by the first visit of the Brownian motion on the Sierpiński gasket at geodesic distance r from the origin. For this purpose we perform a precise analysis of the moment generating function of the random variable T. The key result is an explicit description of the analytic behaviour of the Laplace- Stieltjes transform of the distribution function of T. This yields a series expansion for the distribution function and the asymptotics for t →0.

In this paper the method of inner and outer sums [5], together with the computational power of computer symbolic manipulation, are used to extend to high order the asymptotic expansions in an appropriate limit of some infinite series arising in low Reynolds-number fluid mechanics. The enhanced applicability of the expansions is demonstrated, and the method is extended to treat alternating series.

Rabinowitz' global bifurcation theorem shows that for a large class of nonlinear eigenvalue problems a continuum (i.e., a closed, connected set) of solutions bifurcates from the trivial solution at each eigenvalue (or characteristic value) of odd multiplicity of the linearized problem (linearized at the trivial solution). Each continuum must either be unbounded, or must meet some other eigenvalue. This paper considers a class of such nonlinear eigenvalue problems having simple eigenvalues and a “weak” nonlinear term. A result regarding the location of the continua is obtained which shows, in particular, that in this case the bifurcating continua must be unbounded. Also, under further differentiability conditions it is shown that the continua are smooth, 1-dimensional curves and that there are no non-trivial solutions of the equation other than those lying on the bifurcating continua.