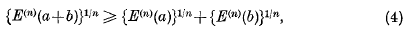Let a1, …, am and b1, … bm be non-negative real numbers. The well-known inequality of Minkowski states that

if n ≥ 1. If n is a positive integer, this inequality asserts a property of a particular symmetric form (i.e. homogeneous polynomial) in m variables, namely the sum of the n-th powers of the variables. Some time ago, Prof. A. C. Aitken conjectured that similar properties are possessed by certain other symmetric forms. In particular, let E(n)(a) denote the n-th elementary symmetric function of a1, …, am and let C(n)(a) denote the n-th complete symmetric function of a1, …, am, the formal definitions being


Then Prof. Aitken conjectured that