INTRODUCTION
The remarkable differences in labor market performance between continental Europe and the United States has sparked a large amount of research on the labor market effects of employment protection legislation (EPL). The existing literature has shown that EPL has important effects on labor market dynamics, since more stringent EPL reduces worker flows in and out of unemployment and increases the average duration of unemployment. However, the relationship between EPL and average unemployment is ambiguous overall, since the reduction in unemployment incidence and the increase in duration tend to offset each other. Although the ambiguous relationship between unemployment and EPL is in line with early theoretical predictions [Bentolila and Bertola (1990)] and the more recent cross-country studies [OECD (1999)], the empirical research of the past decade has identified new results. First, the constructed measures of aggregate job creation and destruction has turned out to be surprisingly similar across countries, suggesting that permanent adjustments in labor demand are hardly affected by the stringency of EPL. Second, worker flows between jobs, or job-to-job movements, do not differ considerably across countries.
This paper argues that permanent adjustments in labor demand cannot be indefinitely avoided by restrictive legislation, since firms always have the option to permanently shut down production at some costs. Nevertheless, EPL plays an important role even when permanent shocks affect labor demand at the firm level, since it can significantly delay the timing of labor adjustment. Indeed, the OECD (1999) suggests that the stringency of EPL has several dimensions, and different dimensions are likely to have different impacts on labor market outcomes. The administrative procedure that firms must follow before notifying a dismissal, the requirements to discuss the separation with the union or with the local authorities, the actual length of the notice period, and the risk that a judge may rule a dismissal unfair are dimensions of EPL that transform firing into a time-consuming process. In light of this complex process, the time lag between the firing decision and the actual separation is sizable and endogenous since it depends on the probability that a worker will find a job without experiencing an unemployment spell. In other words, firms' desired adjustments in labor demand may take place through different combinations of unemployment flows and job-to-job movements, and the more time-consuming the desired adjustment is, the more important the job-job components are likely to be.
As a way to capture in a simple model the time-consuming dimensions of EPL, this paper proposes a search unemployment model in which firms have different options as to how to adjust their employment level.1
On the one hand, firms can always shut down production at some exogenous cost. On the other hand, they can initiate a lengthy separation procedure through the issuance of an exogenously set advance notice. Since the choice of the firing technology is an endogenously determined variable, the actual level of firing costs is also endogenous, and it depends on the aggregate state of the labor market. This paper shows that when aggregate conditions are sufficiently tight, firms have an incentive to issue notice warnings, since they can reduce the expected firing costs when a worker quits before the expiration of the advance notice.
Our simple model brings several new insights. First, the theory we propose incorporates time to fire into the theory of unemployment, which has so far modeled firing restrictions as a simple fixed firing cost to be incurred when separation takes place [Bentolila and Bertola (1990), Bertola and Rogerson (1997)]. Second, and more important, our theory may rationalize the various empirical regularities on the aggregate effects of firing restrictions. In a world without advance notice, firms' desired labor adjustments are more likely to take place instantaneously, and induce workers through an unemployment spell. Thus, job turnover is mainly accommodated by unemployment inflows and outflows. As notice length increases, job separation becomes time-consuming, and the fraction of desired employment adjustment accounted for by job-to-job movements increases. Further, longer advance notice induces an increase in unemployment duration, and a reduction in unemployment turnover.
The paper proceeds as follows. Section 2 reviews the existing evidence on the strictness of EPL, emphasizing its time-consuming dimension. Section 3 reviews the existing literature, pointing out the novelty of the present paper. Section 4 presents the setup of the model, derives its solution, and discusses some of the key simplifying assumptions. Section 5 presents a set of simulations and discusses the predictions of the model in terms of job-to-job flows. Section 6 looks at the empirical relationships between EPL and the structure of job flows for nine OECD economies, emphasizing how the model is consistent with such evidence. Section 7 summarizes and concludes.
Time to Fire and Employment Protection Legislation
This section briefly reviews the multiple dimensions of EPL, for the following nine OECD countries: United States, United Kingdom, Canada, New Zealand, Denmark, France, Sweden, Italy, and Germany. Although the choice of country is constrained by data availability on job and worker flows, the sample we exploit represents the entire spectrum of countries in terms of the strictness of job-security provisions. The OECD (1999) has constructed summary indicators for a country's strictness of employment protection legislation. The indicators are constructed through a scoring method, that assigns different quantitative scores to different institutional dimensions. Summary indicators of the strictness of EPL for regular employment for the nine countries we focus on are provided in the top part of Table 1. The overall EPL index is the average value of three subindicators, which refer, respectively, to the difficulty of dismissal, the size of notice and severance payments, and the regular procedural inconvenience. As is clear from Table 1, the United States, Canada, and United Kingdom are countries with low job-security provisions whereas France, Germany, Sweden, and Italy are countries with strict EPL. Denmark and New Zealand are somewhat in between. In the rest of the paper, we refer to Anglo-Saxon countries (United States, United Kingdom, Canada, New Zealand) as countries with low job-security provisions, and to European countries (Italy, Germany, France, Sweden, Denmark) as countries with high job-security provisions. With the exception of Denmark and New Zealand, whose degree of job-security provision is fairly similar, Table 1 suggests that the two groups of countries are associated with high and low degrees of job-security provisions.
Employer-initiated job separations are restricted in several ways. The most applied form of restriction in OECD countries is the requirement to provide workers with severance payments, that is, with a fixed monetary compensation equal to several months of salary. With severance payments, firing can take place at any time, but it involves a monetary transfer. Advance notice, the other common form of firing restriction, requires firms to provide the worker with several weeks/months of advance warnings, and it forces the firm to keep the worker employed during the entire notice period. As suggested in Table 1, from the decision to dismiss up to the actual termination of the contract, several procedural requirements must be followed: There has to be a sequence of previous warnings, an interview has to be scheduled with the employee, and a third party (work council or the competent local authority) must be notified and consulted, and/or must ultimately approve the dismissal. The average mandatory notice length for dismissing an individual employee is 2 months for the EU countries and 15 days for the Anglo-Saxon countries. In addition, Table 1 shows that the regulatory inconveniences mentioned earlier are much tighter in Europe. Such procedural inconveniences necessarily translate into further time delay, but the OECD does not offer an estimate of the average delay that such procedures impose on job separation.
In the case of collective dismissals, procedural inconvenience and time delays are likely to be more important, as suggested in Table 1. However, with respect to collective dismissals, the difference between the two groups of countries is less sizable. In the case of the United States, the WARN (Worker Adjustment and Retraining Notification) Act, requires employers to give workers written notice of a plant closure with at least 2 months' notice. Further, collective dismissals require a bargaining between the union and the firm, and ex-ante firms do not know the exact timing of the bargaining process. Since collective bargaining is much more pervasive in European countries, the actual delay imposed by such negotiations is likely to be more important in Europe. Overall, it appears that the sum of the notice length and the additional delays imposed by collective dismissals imply a time to fire of some 3 months for the European countries, and 1 month or so for Anglo-Saxon economies.
This paper argues that these dimensions of EPL, albeit relevant in reality, are not traditionally captured by the existing literature, which is reviewed in the next section. Nevertheless, if we want to investigate how job turnover is accommodated in terms of unemployment flows and job-to-job movements, it is certainly necessary take such dimensions into account.
LITERATURE REVIEW
This paper fits into the macroeconomic literature that studies the relationships between labor market flows and EPL, albeit such literature does not recognize the relation between time to fire and the structure of labor market flows. Bertola and Rogerson (1997) argued that the similarity of job turnover rates between North American and European countries should not be surprising, since these countries differ not only in terms of job-security provisions but also in terms of wage-setting mechanisms. However, Bertola and Rogerson (1997) do not attempt to explain why we observe similar turnover rates and markedly different unemployment flows. The latter property, which is emphasized in the current paper, has been observed also by Boeri (1999) who argues that job-to-job movements in Europe are as high as job-to-job movements in North American markets. Boeri's explanation for the similarity of turnover rates rests on the partial deregulation observed in Europe, and on the growing importance of the incidence of temporary contracts. The large turnover rates in Europe, according to Boeri, would be linked to job-to-job transitions of workers employed in temporary contracts, who switch jobs without an intervening unemployment spell. Blanchard and Portugal (2001) carefully analyze the differences between job turnover and worker turnover in the United States and Portugal and find that job turnover at yearly frequencies is very similar in the two countries. However, they find that the proportion of turnover coming from firms' closures in the depressed Portuguese market is much larger than in the North American one. Such finding is fairly consistent with our theoretical perspective, which implies that firms are more likely to shut down production in a more depressed labor market.
There is also an empirical literature that emphasizes the relationship between advance notice and on-the-job search, a key feature of our theoretical perspective. Addison and Blackburn (1994, 1997) have studied the labor market effects of the WARN Act. A key finding of such literature is that advance notice has a significant effect in increasing the probability of avoiding unemployment. Indeed, Addison et al. (1992) and Burgess and Stuart (1992) find that the monotonic relationship between the length of notice and the probability of avoiding joblessness is fairly robust, and continues to hold after controlling for several factors. However, the literature also discovered that advance notice, albeit important in reducing the probability of entering unemployment, seemed to positively affect the length of the unemployment spell once it had started. This puzzle was solved by Addison and Chilton (1997), who showed that the statistical puzzle was linked to the failure of previous studies to appropriately incorporate the predisplacement search time of notified workers. Thus, the solution to the notice puzzle provides further evidence of the importance of on-the-job search for notified workers. Although it is not possible to have a direct estimate of the proportion of job-to-job movers who have received prior advance notice, Ehrenberg and Jakubson (1988) found that in the United States 10% of all displaced workers experience no spells of unemployment, and move directly to a new job. In a more European context, Pfann (2001a) studies theoretically and empirically the behavior of a firm during a slow downsizing. He finds that when workers are heterogeneous, firms choose first to dismiss workers with lower firing costs, and that, following the layoff announcements, the number of workers who quit increases substantially.
Finally, a more theoretically oriented literature has studied whether there is underprovision of advance notice, since the institution appears extremely valuable to workers. Kuhn (1992) proposes a partial equilibrium model in which a firm's profitability is private information, and mandatory notice acts as a signaling device. He shows that there are conditions in which firms would be willing to guarantee advance notice to their to workers, but cannot do so because they lack the means to enforce that promise. Conversely, Addison and Chilton (1997) propose a model in which the commitment problems rest with the workers' inability to alienate their right to quit, and show that there is no underprovision of notice in equilibrium, and that a mandatory notice can have, at most, redistributive effects. Since there are no clear predictions from such literature, and since the interest of the present paper rests more on the macroeconomic consequences of the time-consuming firing process, we assumed that the level and functioning of EPL is simply determined outside the model, and taken as given by firms and workers.
BASIC SET UP
We consider an economy populated by a homogeneous mass of risk-neutral workers, normalized to one for simplicity. Each worker can be in two states: employed or unemployed. As in conventional search equilibrium models, a job is a productive opportunity owned by a firm, and is capable of producing output only when it is matched to a job-seeking worker. If the job is vacant the firm actively searches for a worker, and offers the job to the first worker who meets its needs. Opening a vacancy does not involve any fixed costs, but searching for a worker and keeping a vacancy open entails a flow cost equal to γ.
Existing jobs can be in two states: good or bad. Jobs start their life in the good state, and produce a net flow of production equal to yg, but are subject to permanent adverse idiosyncratic business shocks at rate λ. A good job that turns bad yields a flow of production equal to [phi]yg, with [phi]<1. Firms pay workers a fixed wage throughout the employment relationship, and we let the wage be a fraction β of the marginal product in a good job, so that w=βyg. Further, we assume that β>[phi], so that bad jobs have negative net present value from the firm's standpoint. As a consequence, a firm in a bad business condition will always try to terminate the employment relationship.
There are two ways for downsizing and terminating an employment relationship. On the one hand, firms can shut down production at cost −T. On the other hand, they can start a lengthy separation procedure that requires issuing an institutionally determined advance notice. If the firm opts for the lengthy procedure, a worker cannot be made unemployed unless he or she has been given an advance notice exogenously set to τ*. During the entire notice period, the wage must be fully paid, and firms run marginal losses. A key feature behind the firm's choice of the firing technology is the fact that the requirement to give advance notice applies only to employer-initiated separations, and is consistent with a voluntary quit. In the model, similar to that of Pissarides (1994), we let workers search on-the-job. Thus, if a worker quits before the notice period expires, there are no additional costs involved, beyond the wage paid during the notice time. In this respect, the distinction between quitting and being laid off is consistent with the influential work of Mclaughin (1991), who distinguishes between efficient quits and efficient layoffs on the basis of which of the two parties initiates the job termination. Since all vacant jobs are good jobs, a worker employed in a good firm has no incentive to search on-the-job. Conversely, a worker employed in a bad firm always has the incentive to search on-the-job for a vacant good job.
Whereas closing costs are exogenously given and equal to −T, the expected costs of the advance warning are endogenously determined, since they will depend on the probability that a worker will find a new job during the notice period. We show that a firm's decision to use the lengthy procedure depends on the state of the labor market, and is fully described by a reservation strategy. For analytical reasons, we solve the model with fixed aggregate conditions, and we let firms take the aggregate business conditions as given.
The number of contacts between searching firms and job seekers is given by the matching technology
where x is the total number of matches in a given instant, v is the number of vacancies, u is the unemployment rate, and nb is employment in bad jobs, a measure of the employed job seekers.
2 Equation (1) implicitly assumes that there is no on-the-job search by workers employed in good job. As we show later, this is true in equilibrium.
In equation (1), all measures are expressed as fractions of the fixed labor force. The matching technology
x is assumed to be homogeneous of degree 1 and increasing and concave in both of its arguments. The transition rates from different labor market states are derived from equation (1) after dividing the number of total contacts by the relevant stock of job seekers. Thus, the instant probability that a job seeker finds a vacant job is given by
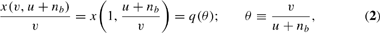
where [theta] is a measure of market tightness from the firm's standpoint, and q([theta]) is a decreasing function of [theta], so that q′([theta])<0. Making use of (2), the total number of contacts between unemployed job seekers and vacant jobs is
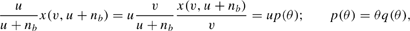
where p([theta]) is the probability that any job seeker will find a vacant job, with p′([theta])>0. Finally, the total number of contacts between workers employed in bad jobs and vacant firms is simply
We next turn to a formal derivation of the model, while in Section 4.4 we discuss the relevance of our assumptions and point out the implications of alternative settings.
MODEL
We present and solve the model in three steps. First, we present and solve the firm's optimization decision with respect to job creation and the optimal separation policy. Second, we define the general equilibrium and derive the equilibrium market tightness. Third, we specify the flow balance conditions and we briefly characterize the equilibrium.
Job Creation, Job Destruction, and Optimal Separation Policy
The firm's expected profit from operating a vacant job of good quality is denoted by Vg and reads
where r is the firm's (and worker's) discount rate, Jg is the firm's value of a good job, and q([theta]) is the firm's probability of finding a job seeker, either unemployed or employed in a bad job. Equation (3) describes the return of a vacant job as the sum of a flow cost γ and a probability q([theta]) of a capital gain equal to Jg−Vg. Since there are no fixed costs in creating a vacancy, firms will open up new job opportunities until the full exhaustion of rents, and free entry in the job market implies Vg=0. Substituting for Vg=0 in (3) yields
Equation (4) is the first key equation of the model, and implies that the value of a new job is equal to the expected search costs. Since wages are exogenously fixed and equal to βyg, worker behavior is summarized by the decision to search on-the-job in low-productivity jobs. If the firm issues the mandatory notice when business conditions turn bad, Jg satisfies the following asset valuation:
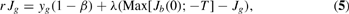
where yg(1−β) is the net marginal profit and λ is the idiosyncratic arrival rate of adverse business conditions. Equation (5) shows that a good job yields a net dividend equal to the difference between the value of the labor product and the wage, and an expected capital loss that depends on the firing technology chosen by the firm. Conditional on the productivity of the job turning bad, the firm has to choose between permanently shutting down production at cost −T or notifying the worker. The expression Jb(0) keeps track of the value of bad jobs at notice time h=0, when the maximum notice period is equal to τ*. In general, if we indicate with Jb(h) the value of bad job at notice time h, its asset valuation function reads
where ([phi]−β)yg is the net marginal loss in bad jobs,
is the capital gain associated with the elapsing of notice time, and
is the expected capital gain associated with a worker-initiated quit. The value of having a bad job at notice time h reads
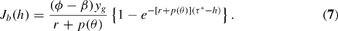
Equation (7) shows that the value of a bad job is a negative function, monotonically increasing in notice time. The value of a bad firm is discounted by two factors, the pure discount rate r and the probability that the worker will find a good job p([theta]). The Appendix reports simple comparative static results. Intuitively, the (negative) value of a bad job decreases monotonically with the length of the maximum notice τ*. Furthermore, since Jb(h)<0, the deadweight loss increases with the interest rate. However, the most important comparative static result is the positive link between market tightness and the cost of separation
. Since workers employed in bad jobs search for employment in good jobs, the higher the market tightness (i.e., the higher [theta]) the higher the probability that a worker under advance notice will be matched to a good job and will quit before the expiration of the notice time. As a result, the value of a bad job becomes less negative as [theta] increases. Equation (7) implies that firing costs decrease with market tightness.
This discussion suggests that the firm's choice between closing down [at cost −T] and advance notice [at cost Jb(0)] depends on the aggregate state of the market [theta]. Since the relationship between Jb(h) and [theta] is monotonic, the choice of the firing strategy satisfies the reservation property, and firms use advance notice for values of market tightness larger than a reservation value [theta]T, where [theta]T solves
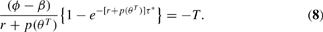
Assuming the parameters of the model are such that equation (8) is solved for an interior value [theta]T>0, it is possible to derive few comparative static exercises for the firing margin [theta]T. In particular, the firing margin increases with the notice length τ*, and decreases with the closing cost −T. We are now in a position to define the equilibrium.
DEFINITION 1.
A steady-state market equilibrium is a n-tuple ([theta],[theta]T,u,ng,nb) satisfying
- free entry on the parts of firms [equation 4],
- optimal separation policy [equation 8],
- aggregate consistency,
- steady-state balance flow condition
The free entry condition on the part of firms is the vacancy posting condition, or equation (4), which shows that the value of a job is equal to the expected search costs. The optimal firing policy is described by equation (8), or by a reservation market tightness [theta]T above which using notice is the optimal separation policy. Aggregate consistency requires that the market tightness obtained by the free entry condition (4) is consistent with the market tightness that characterizes the optimal separation policy (8). The steady-state balance flow conditions are derived in Section 4.3, after we show how to determine market tightness [theta].
Equilibrium Market Tightness
To determine the equilibrium in the labor market, we need to derive market tightness [theta]. This is done by simultaneously solving the free entry condition and the optimal separation policy. Substituting equation (4) into equation (5), equilibrium market tightness is the solution to
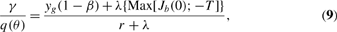
where Jb(0) is given by equation (6) evaluated at h=0. Since the maximization in equation (9) depends on a reservation value of [theta]=[theta]T, the solution to the previous equation can be obtained in four steps. First, we solve for the separation policy [theta]T. Second, we guess whether the equilibrium [theta] is above or below the reservation market tightness [theta]T. We let
be such a guess. Third, we solve the following nonlinear equation in [theta], whose formal expression depends on whether
is above or below [theta]T:
Fourth, we check whether the equilibrium level of [theta] is consistent with the guessed value
. In other words, we check whether the equilibrium value is indeed larger or smaller than the reservation market tightness [theta]T. The left-hand side of equation (10) is a measure of the average search cost, an increasing function of [theta]. The right-hand side is the value of a good job, which can or cannot depend on [theta]. Indeed, the right-hand side of the preceding equation is independent of [theta] when
, or when firms shut down production at cost T. Conversely, the right-hand side is an upward-sloping function of [theta] when [theta]>[theta]T and firms use the lengthy procedure. This positive relationship is due to the monotonic link between Jb(h) and [theta].
Stock and Flows with Advance Notice
To close the model we need to keep track of the distribution of employment between good and bad jobs and of the distribution of bad jobs at different notice times τ. For values of [theta] lower than [theta]T, the distribution of bad jobs is trivial, because firms shut down production and immediately fire the workers. This implies that nb=0 if
. Conversely, if
, bad jobs and good jobs coexist. In what follows, we indicate with
, the time t number of workers employed in bad jobs with notice period less than or equal to τ. Over time, it must be true that
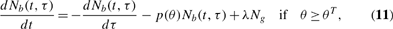
where the first term refers to the number of workers whose duration becomes higher than τ, the second term refers to the fraction of workers who found a good job, and the last term refers to the number of good jobs that issue a new notice warning at time t. In a steady-state, the distribution of bad jobs at different notice times must be constant and, in equation (11),
. The steady-state density of unemployment duration solves the following differential equation in notice time τ:
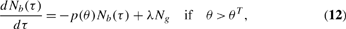
where the time t index, irrelevant in a steady-state, has been omitted for analytical convenience. The solution to (12) reads
where the second relationship highlights the fact that, for values of [theta] below the reservation market tightness, firms shut down production when conditions turn bad and there are no workers employed in bad jobs. Unemployment is constant when inflows are equal to outflows. Unemployment outflows are simply given by p([theta])u, whereas unemployment inflows depend on whether firms use notice or closing costs as the selected separation strategy. In the former case, unemployment inflows are equal to the proportion of bad jobs that reach maximum notice τ*, whereas in the latter case they are just the proportion of good jobs hit by the shock λ. In formulas, unemployment is constant if
where
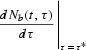
is the fraction of bad jobs that reach duration τ* at time t. Further, the labor force constraint must be binding, which implies that ng,u and nb continuously sum to 1, so that
where
is the aggregate measure of bad jobs. Equations (13), (14), and (15) form a system of three equations in three unknowns, which can be solved to yield the equilibrium number of good jobs as
the equilibrium number of bad jobs as
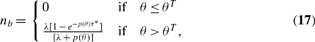
and equilibrium unemployment as
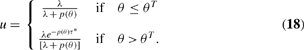
Equation (18) implies a negative relationship between market tightness and unemployment, a sort of Beveridge curve, which holds independently of the separation strategy being used. Nevertheless, the relationship between EPL and unemployment is fairly complex since it depends on the separation strategy being used. As long as closing costs are the optimal separation policy ([theta]<[theta]T), there is a positive link between T and unemployment, because larger closing costs reduce market tightness. Conversely, when firms use the lengthy procedure ([theta]>[theta]T), the relationship between unemployment and advance notice is ambiguous overall because there is a direct effect, working through τ* in equation (18), and an indirect effect, working through the effect of τ* on [theta]. A longer notice period τ* directly increases unemployment incidence while it increases unemployment duration through its effect on [theta]. The overall effect of τ* on unemployment is thus ambiguous, and it depends on the particular set of parameters.
Discussion
Before turning to a formal simulation of the model, we discuss four issues linked to our basic setup: wage determination, the timing of advance notice, the cost of separation, and the role of aggregate shocks. In the rest of the section, we discuss these four issues in turn.
Throughout the derivation of the model, we have assumed a fixed wage, independent of business conditions, in a way consistent with the work of Bentolila and Bertola (1990). Alternatively, we could solve the model with endogenous wage bargaining and let firm–worker pairs split the surplus from the job. Inevitably, such wage structure would entail large wage drops when conditions turn bad. We did not pursue such a more complicated wage structure for at least three reasons. First, there is microeconometric evidence that workers in downsizing firms do not experience massive wage drops. Pfann (2001b) studied the downsizing behavior of a large Dutch aircraft manufacturer and found that, throughout the 10 years of downsizing there was continuous wage growth and, during the final year of the firm's life, there was hardly any noticeable change in the firm's wage distribution. Second, there is also evidence of more general downward nominal wage rigidity, as reported by Kahn (1997), among others. Finally, the model we solve is analytically very simple. Overall, we believe that our simple wage structure is empirically reasonable for a model that focuses mainly on the separation decision.
In our model, the reservation market tightness [theta]T describes the separation choice between closing down production at cost −T and issuing a notice warning when conditions turn bad. At least theoretically, however, one may consider the possibility that firms issue the notice warning when conditions are still good, as a way to reduce the cost of separation if conditions turn bad. If such policy was completely costless, good firms would have an incentive to put workers on notice, just to ask them to ignore such warnings if business conditions remain favorable. Such practice is not observed in reality, probably because it is illegal or because there are further productivity losses incurred when a worker is put on advance notice. Although, in this paper, we ruled out such possibility, Garibaldi (1999) shows that as long as the productivity loss linked to advance notice is high enough, firms do not pursue this alternative strategy in equilibrium.
In deriving the equilibrium, we have assumed that, upon the expiration of the advance notice, the firm can dismiss the worker at no cost. As an extension, it is possible to account for costly separation upon the expiration of the notice, by assuming that, at τ*, firms may separate at cost πT, where π is a parameter less than or equal to 1. In this latter case, the optimal firing policy solves
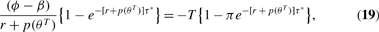
where equation (8) is obtained as a special case when π=0. Two consequences stem from the introduction of firing costs at the expiration of the notice time τ*. First, the larger π, the less attractive is the lengthy separating procedure because the latter now involves the cost of running a bad job plus the termination cost πT. Second, a larger advance notice has ambiguous impact on the value of a bad job and on the reservation market tightness [theta]T. Indeed, a larger τ* certainly increases the cost of running a bad job, but it reduces (in present-value terms) the time at which the additional firing cost has to be paid. Since the two effects work in different directions, the overall relationship between the notice length τ* and the reservation market tightness [theta]T is now ambiguous and some of the comparative static exercises carried out in the Appendix cannot be signed. In the simulation below, we avoid this further complication, and we solve the model for π=0.
The final issue to be addressed is the behavior of the model out of steady-state. In the paper, we assume that aggregate business conditions are constant, and that each (small) firm takes the aggregate conditions as given. The latter assumption is consistent with the productivity shock being fully idiosyncratic, exactly as we assume in our setup. Further, our equilibrium definition is consistent with rational expectation because equilibrium market tightness is consistent with the optimal separation policy [theta]T. Still, one may consider what happens to the model out of steady-state, and assume that alongside idiosyncratic shocks, firms are hit by adverse aggregate shocks. Unfortunately, in such a business-cycle setting, the distribution of jobs between good and bad times would be history dependent, and the model would require numerical solutions. Yet, such extension would certainly enrich the analysis since, at the outset of recession, when aggregate conditions turn bad, we would observe a discrete mass of firms willing to initiate a firing procedure. In terms of the optimal separation policy, such aggregate shocks would be associated with a fall in market tightness, and a subsequent reduction in the incentive to use advance notice. As a result, the incentive to use advance notice would be countercyclical since the probability that workers quit falls during recession. These and other extensions are certainly interesting, but they appear beyond the scope of the present paper. With these limitations in mind, we now turn to the results of our steady-state simulations.
SIMULATIONS
This section presents the results of a simulation of the model for different maximum notice time τ*. Even though the simulations are meant to be suggestive, they allow us to assess quantitatively the relationship between the notice's length τ*, the unemployment stock, job creation, unemployment inflows and outflows, and job-to-job flows. Table 2 reports the values of the parameters used in the baseline simulation. The value of the notice length τ* varies from a minimum value of one tenth of a quarter to a maximum value of 1.4 quarters, a range in notice length similar to the time to fire featured by the OECD economies studied in Section 2. The parameters are set for quarterly rates, but we report statistics for annualized quarterly rate, so as to compare them easily with the empirical evidence presented in Section 6.
Table 3 reports the detailed results of the simulation statistics for a steady-state economy whose maximum notice time is given in each column of Table 3. Table 3 shows that, across different values of τ*, our economy features an average unemployment rate of 4% and a job turnover rate of 24% of the labor force. The table suggests that advance notice has important effects on the composition of job turnover between unemployment flows and job-to-job movements. In the rest of this section, we look in more detail at the different statistics.
Consistent with the comparative static results of the preceding section, the relationship between unemployment and EPL depends on the separation strategy being used. For values of τ* ranging from 0.1 up to 1 quarter, unemployment varies between 3% and 4% of the labor force; it increases to 6% when closing costs (−T) are the optimal separation policy. The simulations suggest that an increase in the notice period has no quantitative impact on the job turnover rate, but it significantly affects unemployment flows and job-to-job movements. With very small advance notice (τ*=0.1), Table 3 shows that unemployment flows are almost identical to job turnover, and the economy does not experience sizable job-to-job flows. Conversely, as the length of advance notice increases, the composition of job turnover in terms of unemployment flows and job-to-job flows changes, and with a notice length of one quarter, job-to-job movements account for more than 40% of total job turnover. More formally, the effect of advance notice on turnover is driven by two opposing forces. On the one hand, an increase in the notice period τ* reduces [theta]. Since job creation is given by
, lower [theta] tends to reduce turnover. On the other hand, an increase in the notice period increases nb, the number of job-searching employees. As a result, the turnover in regulated markets is quantitatively as high as the turnover in unregulated markets, whereas the corresponding unemployment flows are much smaller. The average duration of unemployment, which is 1.3 quarters in an economy with no advance notice, increases to almost 2 quarters as τ* reaches 1.4. Overall, these results are consistent with the view that, with permanent shocks to labor demand, EPL has little effect on job turnover. Nevertheless, the simulations suggest that the composition of job turnover in terms of unemployment flows and job-to-job movements depends crucially on time to fire, which in our model is summarized by the maximum notice time τ*.
To summarize, the model has three key implications. First, job turnover is independent of time to fire and the degree of job-security provisions. Second, unemployment turnover is much lower in countries with high job-security provisions and lengthy firing procedures. Third, job-to-job movements account for a larger proportion of job turnover in countries with strict EPL. In the next section, we look at the cross-country empirical evidence, and we show that our results are consistent with average differences between European and Anglo-Saxon countries.
JOB FLOWS AND WORKER FLOWS ACROSS COUNTRIES
In this section we review the empirical relationships between job flows, worker flows, and EPL for the same countries for which we discuss the strictness of EPL in Table 1. We first review the existing evidence, and then discuss the links between the theoretical predictions and the available evidence. Throughout the section, we distinguish between Anglo-Saxon and European countries, in a way similar to what we did in Table 1.
Table 4 reports average rates of job creation and destruction for our nine countries. Job flows are measured from firm-level data, and job creation (destruction) is defined as the sum of all positive (negative) employment changes at the micro level. From the data reported in Table 4, it is clear that the average rates of gross job creation and destruction are very similar across countries. The average rate of job turnover is 23% of the labor force in both Anglo-Saxon countries and European countries. Although the comparability of the data may be a cause for concern, the OECD has tried to standardize data as much as possible, and the figures reported are all from the OECD (1994) data set.
Table 5 reports average rates of unemployment inflows and unemployment outflows for our set of countries during the same period of analysis. Unemployment flows are typically measured from labor force surveys. The unemployment inflow is the sum of all individual entries into unemployment, either from employment or from out of the labor force. Similarly, the unemployment outflow is the sum of all individual exits from unemployment, either into employment or out of the labor force. Table 5 shows that, in terms of unemployment flows, the difference between the two groups of countries is remarkable.3
In the empirical literature, as well as in Table 2, unemployment inflows are often proxied by the number of unemployed, with unemployment duration lower than a month.
. Note that, in general, worker flows are larger than job flows, since workers can change jobs for many reasons, and only some of those changes are linked to firms' changes in the desired level of employment.
Table 6 shows also that the average duration of unemployment, measured in months, is twice as large in Anglo-Saxon countries as in European countries. Along this dimension, however, the heterogeneity within the two groups of countries is more sizable, since the United Kingdom, grouped in the Anglo-Saxon countries, has a fairly large average duration of unemployment whereas Sweden has a very low duration.
The ratio of unemployment turnover to job turnover, as reported in Table 5, summarizes the difference between the two groups of countries. The proportion of job flows that is accounted for by unemployment flows is 50% in European countries, whereas it is more than 2 in Anglo-Saxon countries. This suggests that, in Europe, job-to-job movements play an important role in accommodating the observed rates of job turnover. For Anglo-Saxon countries, job-to-job movements can certainly be sizable, but the observations of Table 5 suggest that unemployment flows by themselves fully account for the necessary flows induced by changes in labor demand.
The problem with job-to-job flows, however, is that they are very difficult to measure because labor force surveys do not allow scholars to observe whether an employed worker has changed employer over successive rounds of surveys. However, for most European countries, there is an indirect possibility of estimating job-to-job flows. The procedure works as follows. First, one obtains an estimate of the hiring rates by counting the number of employment relationships with tenure of less than a month. Second, it is possible to estimate employment inflows, which are defined as the number of employed workers who were in a different labor market state in the previous labor force survey. Finally, by taking the difference between these two measures, one obtains a proxy of job-to-job movements. This is what is done in Table 7, using some of the information originally compiled by Boeri (1999). The results of Table 7 suggest that job-to-job flows in European countries are as large as job-to-job flows in Anglo-Saxon countries.4
In Table 7, there are only two observations for Anglo-Saxon countries. Although estimates for other countries do exist, they are obtained with very different methodologies and comparison with the ones presented in Table 7 is impossible. For the U.S. labor market, for example, Blanchard and Diamond (1990) estimate job-to-job flows at 20% of hiring rates. Although this result would be consistent with the findings of Table 7, it was derived with a very different method.
is more consistent with the similarities of job turnover rates observed in
Table 4 than with the remarkable differences observed in
Table 5, where we observed that unemployment flows in Anglo-Saxon countries were four times larger than in European countries.
We can summarize the main empirical regularities in the following way. Countries with different job-security provisions experience very similar rates of job creation and destruction, suggesting that permanent changes in demand are not significantly affected by the strictness of EPL. Nevertheless, EPL significantly affects the composition of worker flows with respect to job flows. In countries with long time to fire, job turnover is accounted for in similar quantity by unemployment flows and job-to-job flows. Conversely, countries with loose EPL and no time to fire experience unemployment flows that are much larger than job flows, and more than compensate for the underlying job turnover. In addition, job-to-job flows do not differ across countries.
How does the empirical literature compare with our theoretical perspective? Our model clearly predicts that job turnover is similar across countries with different degrees of job-security provisions. In the simulations reported in Table 3, job turnover is around 24%, independently of the strictness of EPL, in a way consistent with the results reported in Table 4. The simulations reported in Table 3 are also consistent with substantial differences in unemployment flows. Indeed, our theory predicts that unemployment inflows should be much higher in countries with low job-security provisions. Further, in the simulation of Table 3, the ratio between unemployment inflows and job turnover falls as job-security provisions increase, in a way consistent with the evidence reported in Table 5. Yet, in our model, such ratio is constrained to be less than one, since the only determinant of worker flows is an underlying job flow. In real labor markets, as we argued earlier, this is not necessarily the case, and the ratio can be much larger than 1 (Table 5). Finally, our simulations suggest that job-to-job flows should be larger in countries with strict EPL. This prediction is not fully supported by the evidence of Table 7, where we show that job-to-job flows are similar across different groups of countries. To further improve the match between the model and the real data, one would need to add other types of shocks, so as to generate job-to-job movements that are unrelated to job destruction. Nevertheless, it is clear that our model provides a good rationalization of the empirical links between job flows and worker flows across countries.
CONCLUSIONS
This paper was motivated by several empirical regularities in labor market flows across countries. The first observation concerns the behavior of unemployment flows. Unemployment inflows and outflows are much larger in North America than in Europe and, consequently, the incidence of long-term unemployment is much smaller in North America than in Europe. These marked differences in unemployment flows appear consistent with the view that high job-security provisions in Europe reduce unemployment turnover and increase the average duration of unemployment. The second observation concerns the behavior of job and worker turnover. On average, job turnover in highly regulated European markets appears similar to the turnover of Anglo-Saxon countries, suggesting that European labor markets create a number of jobs similar to those in the North American markets. This paper has argued that the existence of permanent shocks to labor demand along with time-consuming firing practices in European labor markets, is consistent with both sets of observations.
Time to fire in the job separation process appears an important determinant of the relationship between job-security provisions, labor market flows, and unemployment duration. Even though time to fire reduces firms' incentives to create new jobs, it provides workers with an opportunity to switch to high-productivity jobs without experiencing an unemployment spell. In equilibrium, an increase in time to fire (modeled as advance notice) causes two effects on turnover in the labor market. On the one hand, higher advance notice causes firms to reduce the number of vacancies, thus inducing a reduction in turnover. On the other hand, higher notice increases the number of workers who search on-the-job, causing an increase in job turnover. As a result, turnover in highly regulated markets can be quantitatively as high as turnover in unregulated markets. However, an increase in advance notice unambiguously reduces the number of people who enter and exit unemployment.
Future work should try to solve one further puzzle in the international comparison of labor market flows. As Blanchard and Portugal have shown, job turnover in highly regulated markets is as high as job turnover in flexible markets only when we look at yearly data. At higher frequency, job turnover in Portugal is much lower than job turnover in the United States, suggesting that the effect of job-security provisions on employment is very different when shocks are temporary than when shocks are permanent.
ACKNOWLEDGEMENT
I am indebted to Gaia Narciso for competent research assistance, and to the editor and an anonymous referee for useful comments. I also thank Giuseppe Bertola, Giovanni Dell'Ariccia, Chris Pissarides, Luis Cubeddu, and participants at various seminars for insightful comments, and Paula Adam for helping me in putting together the various data. A previous version of this paper circulated as CEPR discussion paper 2164, and it was prepared when the author was affiliated with the International Monetary Fund.
