Hostname: page-component-745bb68f8f-cphqk Total loading time: 0 Render date: 2025-02-11T12:11:02.635Z Has data issue: false hasContentIssue false
  • English
  • Français

Mitigation of electromagnetic instabilities in fast ignition scenario

Published online by Cambridge University Press:  02 June 2005

CLAUDE DEUTSCH ,
ANTOINE BRET  and
PATRICE FROMY
CLAUDE DEUTSCH
Affiliation:
Laboratoire de Physique des Gaz des Plasmas (UMR-CNRS), Université Paris XI, Orsay Cedex, France
ANTOINE BRET
Affiliation:
Laboratoire de Physique des Gaz des Plasmas (UMR-CNRS), Université Paris XI, Orsay Cedex, France
PATRICE FROMY
Affiliation:
Centre de Ressources Informatiques, Université Paris XI, Orsay Cedex, France
Rights & Permissions [Opens in a new window]

Abstract

We address the issues of collective stopping for intense relativistic electron beams (REB) used to selectively ignite precompressed deuterium + tritium (DT) fuels. We investigate the subtle interplay of electron collisions in target as well as in beam plasmas with quasi-linear electromagnetic growth rates. Intrabeam scattering is found effective in taming those instabilities, in particular for high transverse temperatures.

Keywords

CollisionsFast IgnitionQuasi-linear theoryWeibel instability
Type
Research Article
Information
Laser and Particle Beams , Volume 23 , Issue 1 , March 2005 , pp. 5 - 8
Copyright
2005 Cambridge University Press

1. Introduction

The interaction processes involved in the stopping of intense relativistic electron beams (REB) for the fast ignition scenario (FIS) (Tabak et al., 1994; Deutsch, 2003a, 2003b, 2004; Mulser & Bauer, 2004) are monitored by a competition between collisionally dominated stopping mechanisms (Deutsch et al., 1996), and nearly instantaneous beam energy loss due to fast rising electromagnetic instabilities (Bret et al., 2005, 2004).

Let us now consider a current neutral beam-plasma system. The relativistic REB propagates with the velocity vdb and the plasma return current flows with vdp. It is reasonable to assume that an electromagnetic mode has k normal to vdb, perturbed electric field E parallel to vdb, and perturbed magnetic field B normal to both vdb and E. So, the total asymmetric f0 consists of nonrelativistic background electrons and relativistic beam electrons (Okada & Niu, 1980)

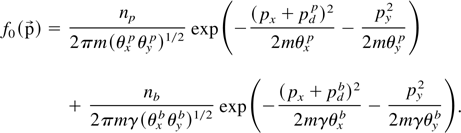
Here θx and θy are the temperature components parallel to the x and y directions, Pd is the drift momentum, superscripts p and b represents the plasma and the beam electron, respectively. From the linearized Vlasov equation with collision term n and linearized Maxwell's equations, we get linear dispersion relations for a purely growing mode. Collision term n = np + nb is explained as a superposition of target and beam plasma contributions. In Eq. (1) the drift momentum should read as

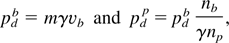
in terms of γ = (1 − vb2/c2)−1/2 and vb, beam velocity.

2. DISPERSION RELATIONS

Our analytical scheme could be implemented in the most transparent fashion through a string of dimensionless variables and parameters. Weibel electromagnetic instability (WEI) growth rates and wave number obviously take then the form (ωp is the plasma frequency)

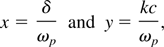
with corresponding normalized collision frequencies

Transverse velocities play a pivotal role in the WEI growth rate analysis. They read as

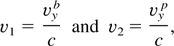
altogether with beam target density ratio

With these expressions, one can then specialize the evaluation of the plasma Fried-Conte dielectric function through suitable asymptotic expansions. This procedure then leads to four typical beam-target combinations based on the asymmetry parameters,

3. QUASI-LINEAR EXTENSIONS (QL)

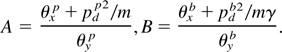
Up to now we were restricted to a linear WEI analysis. More accurate growth rates (GR) are expected by retaining particle motion in target plasma under local electric and magnetic fields. Sophisticated treatments going back to Dupree-Weinstock analysis (Kono & Ichikawa, 1973) of so-called weak turbulence. In the present context these considerations lead us to complete definitions of A and B with

where X denotes the largest solution of

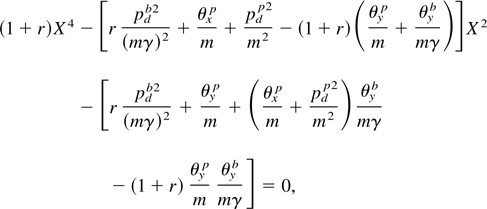
and D = 511r(1 − γ−2). Tp and Tb are in keV.

4. GROWTH RATES (GR)

A typical REB target interaction of FIS interest is depicted in Figs. 1a and 1b with linear (L) and quasi-linear (QL) growth rates (GR). Linear GR are seen monotonously increasing for any collisionality n (target) or n1 (beam) in the whole wavelength range. Quasi-linear GR turn negative as soon as kc/ωp ≥ 0.6. It has to be appreciated that the retaining collisions only in target plasma enhance GR at kc/ωp ≤ 0.6, while restricting them to beam plasma produces the lowest GR. Keeping them in target and beam plasmas still keep the growth rate within acceptable values for permitting an efficient target ignition through beam collisional stopping. Further results are reported on Figs. 1c and 1d for cooler beam and target temperature (Tb = Tp = 100 eV). Other parameters remaining unchanged, collisional contributions n and n1 are thus expected stronger. Indeed, quasi-linear GR (Figs. 1b and 1d) with nonzero n and n1 are manifestly the lowest.

Linear (a) and quasi-linear (b) electromagnetic growth rates for 1 MeV REB with nb = 1022 e-cm−3 and Tb = 1 keV impinging an electron target with np = 10 nb and Tp = 1 keV. Curves are parametrized w.r.t to normalized collision frequencies n (target) and n1 (beam). (c) and (d): same plots for cooler beam and target temperature with Tb = Tp = 100 eV.

Increasing significantly the target plasma density and the beam temperature (Fig. 2) make negligible the intrabeam collision term fulfilling now n1 n. The very high Tb value, in MeV range, erases very efficiently any positive growth rate, thus featuring a beam-target interaction stable at any plasma wave number k.

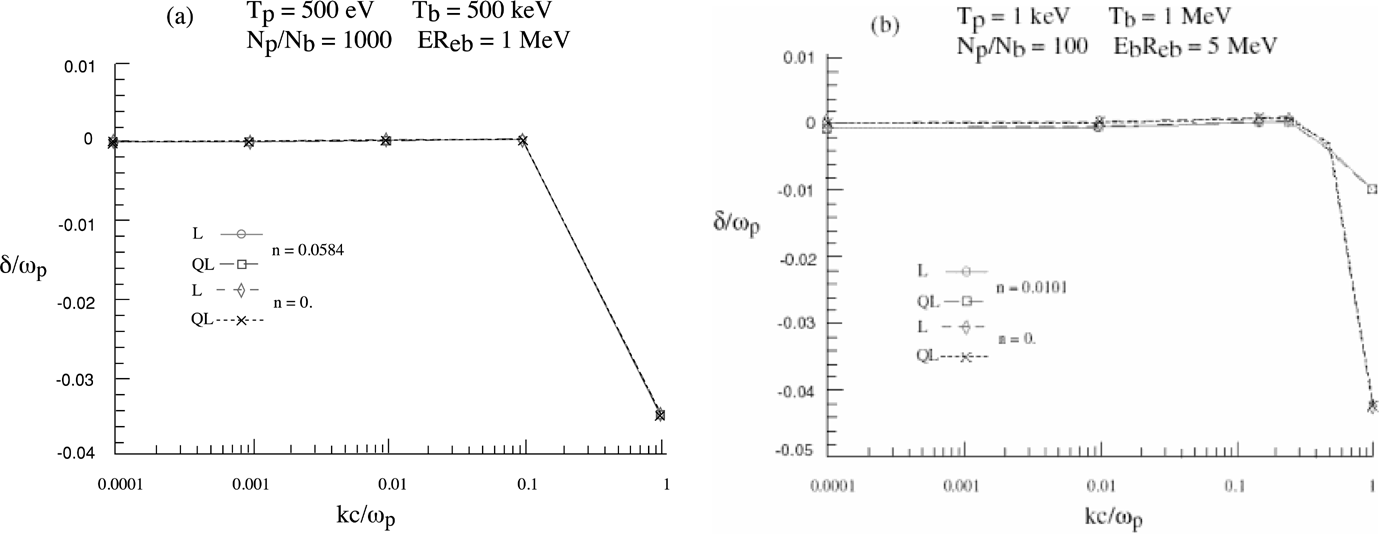
Same captions as in Figs. 1 for higher target densities and beam temperature with negligible intrabeam scattering. (a) np = 1025 e-cm−3, Tp = 0,5 keV with Tb = 500 keV. (b) np = 1024 e-cm−3, Tp = 1 keV with Tb = 1 MeV and 5 MeV REB.

These preliminary results highlight the cone-angle scenario (Kodama, 2001; Deutsch, 2003a, 2003b) with laser produced electrons close to highest density core in precompressed DT fuel.

References

REFERENCES

Bret, A., Deutsch, C. & Firpo, M.C. (2005). Bridging the gap between two stream and filamentation instabilities. Laser Part. Beams 23, In press.CrossRefGoogle Scholar
Bret, A., Firpo, M.C. & Deutsch, C. (2004). Collective electromagnetic modes for beam-plasma interaction in whole k space. Phys. Rev. E 70, 46401.Google Scholar
Deutsch, C., Furukawa, H., Mima, H., Murakami, K. and Nishihara, K. (1996). Interaction physics of the fast ignition concept. Phys. Rev. Lett. 77, 2483.CrossRefGoogle Scholar
Deutsch, C. (2003a). Fast ignition schemes for inertial confinement fusion. Eur. Phys. J. Appl. Phys. 24, 95.CrossRefGoogle Scholar
Deutsch, C. (2003b). Transport of Megaelectron Volt protons for fast ignition Laser Part. Beams 21, 33–35.CrossRefGoogle Scholar
Deutsch, C. (2004). Penetration of charged particle beams in the outer layers of precompressed thermonuclear fuel. Laser Part. Beams 22, 115–120.Google Scholar
Kodama, R., Norreys, P.A., Mima, K., Dangor, A.E., Evans, R.G., Fujita, H., Kitagawa, Y., Krushelnik, K., Miyakoshi, T., Miyanada, N., Normatsu, T., Rose, S.J., Shozaki, T., Shigemori, K., Sunahara, A., Tampo, M., Tanaka, K.A., Toyama, Y., Yamanaka, T. & Zepf, M. (2001). Fast heating of ultrahigh-density plasma as a step towards laser fusion ignition. Nature 412, 798.CrossRefGoogle Scholar
Kono, M. & Ichikawa, Y.H. (1973). Renormalization of the wave-particle interaction in weakly turbulent plasmas. Prog. Theor. Phys. 49, 754.CrossRefGoogle Scholar
Mulser, P. & Bauer, D. (2004). Fast ignition of fusion pellets with superintense lasers: Concepts problems and prospective. Laser Part. Beams 22, 5–12CrossRefGoogle Scholar
Okada, T. & Niu, K. (1980). Electromagnetic instability and stopping power of plasma for relativistic electron beams. J. Plasma Phys. 23, 423.CrossRefGoogle Scholar
Tabak, M., Hammer, J., Glinsky, M.E., Kruer, W.L., Wilks, S.C., Woodworth, J., Campbell, M.E., Perry, M.D. & Mason, R.J. (1994). Ignition and high gain with ultra powerful lasers. Phys. Plasmas 1, 1626.Google Scholar
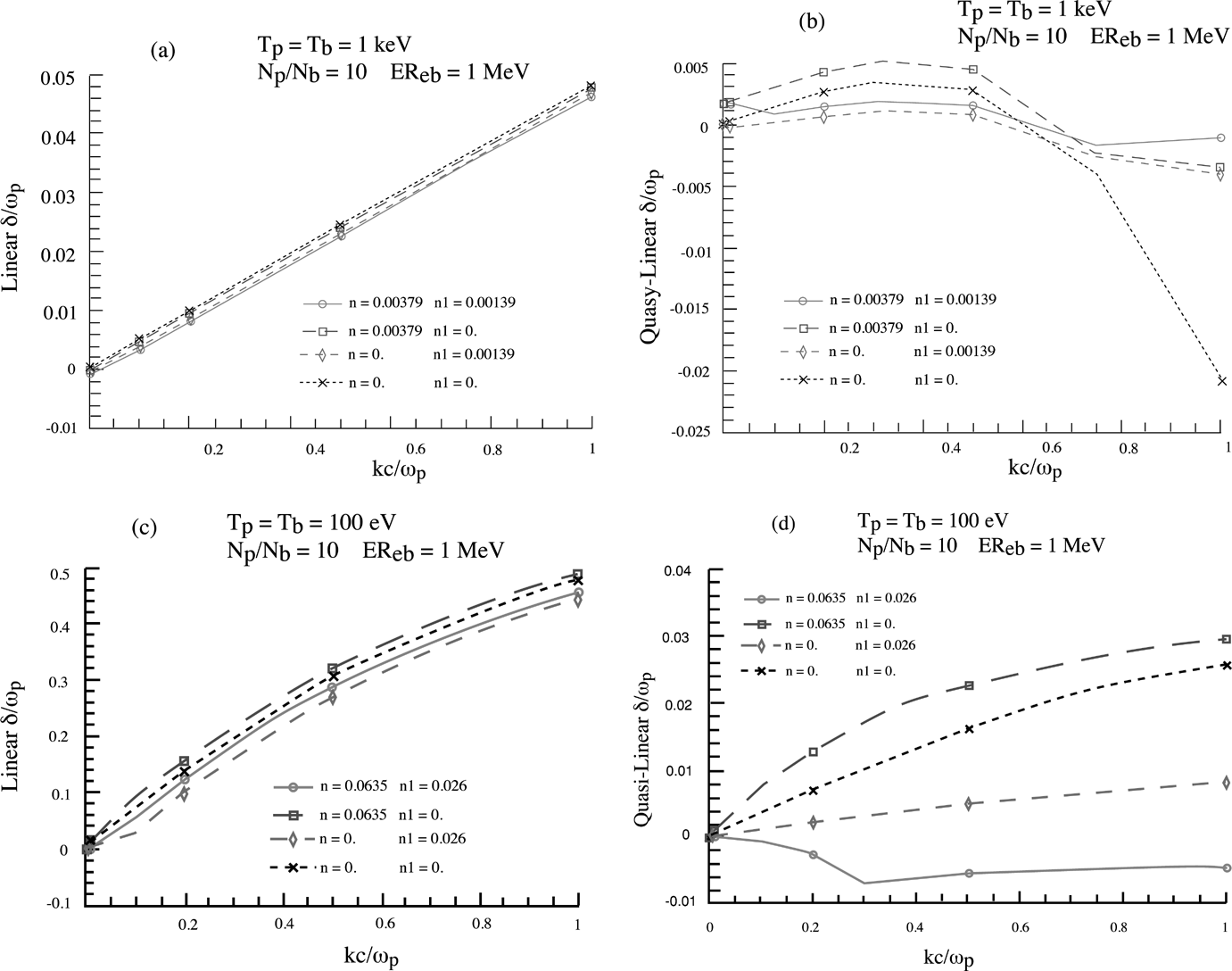
Figure 0

Linear (a) and quasi-linear (b) electromagnetic growth rates for 1 MeV REB with nb = 1022 e-cm−3 and Tb = 1 keV impinging an electron target with np = 10 nb and Tp = 1 keV. Curves are parametrized w.r.t to normalized collision frequencies n (target) and n1 (beam). (c) and (d): same plots for cooler beam and target temperature with Tb = Tp = 100 eV.

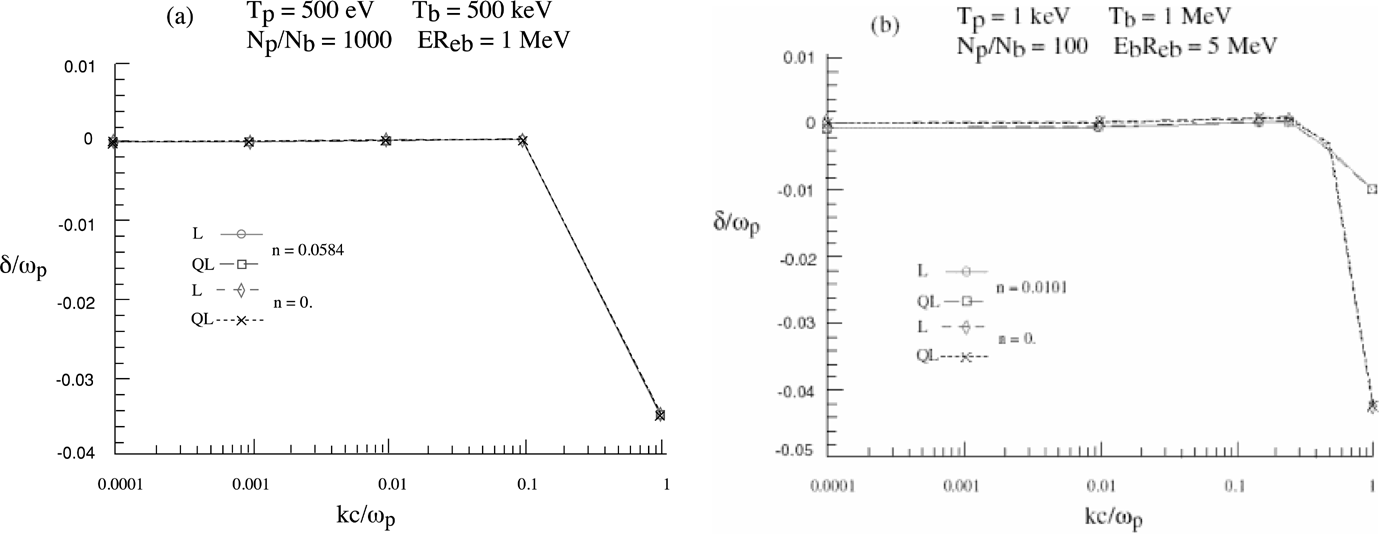
Figure 1

Same captions as in Figs. 1 for higher target densities and beam temperature with negligible intrabeam scattering. (a) np = 1025 e-cm−3, Tp = 0,5 keV with Tb = 500 keV. (b) np = 1024 e-cm−3, Tp = 1 keV with Tb = 1 MeV and 5 MeV REB.