1. INTRODUCTION
High power neodymium lasers available today with a peak power of
hundreds of gigawatts find their use in laser plasma interaction
studies, laser material processing, nonlinear studies, X-ray lasers,
laser-induced ablation of material, and so forth. Usually lasers
required to conduct such studies consist of a master oscillator
delivering a low energy (few millijoules) laser pulse of a duration
ranging from nanosecond to picosecond, which is followed by set of
amplifiers and Faraday isolator to boost the laser energy from the
laser oscillator to the desired level. The laser chain that involves a
commercial oscillator and a series of amplifiers in master oscillator
power amplifier (MOPA) configuration offers high-energy stability,
reliability, and design flexibility (Koechner,
1992). However for stable operation, these oscillators are
operated at a frequency ranging from 1 to 10 Hz. The high repetition
rate operation of the oscillator takes care of thermal lensing in YAG
rods, whereas external amplifiers and a Faraday isolator are operated
in the single-shot mode. The typical commercial oscillator operating at
10 Hz in the laser chain delivers an output consisting of some
prepulses and the main laser pulse with a contrast ratio of ∼50:1.
The experiments involving laser-induced ablation of material or
laser–plasma interaction require a single, high contrast
(>1000:1), clean, high-power laser pulse. In this article, we
present the design details of a 2 J/200 ps laser chain that
involves a modified commercial oscillator for single-shot operation by
pulsed biasing of the cavity dumping photodiode, external pulse
selector based on a single Pockel's cell used in push–pull
configuration between two crossed polarizers, two silicate glass
amplifiers, and a Faraday isolator.
The equation of state (EOS) of a material under high-pressure
conditions is important to several branches of physics, in particular,
astrophysics, materials science, and inertial confinement fusion
research (Lindl, 1995; Celliers et al., 2000). EOS data (Chidambaram, 1996; Sikka et
al., 1997) is also important for simulation of fission and
fusion devices. Several techniques are available for generating shock
pressures ranging from 1 Mbar to hundreds of megabars. Diamond anvil
cell and two-stage gas gun can produce shock pressures up to 5 and 10
Mbar, respectively (Nellis et al.,
1988; Mitchell et al., 1991).
Shock pressures greater than 10 Mbar can be obtained in underground
nuclear tests that are difficult to perform (Trunin
et al., 1969). High-power pulsed lasers offer new ways
of producing shock pressures of more than a few tens of megabars (Trainor et al., 1979; Veeser et al., 1979; Ng
et al., 1985). A high-power laser pulse focused on a
thin foil target (a few microns) kept in vacuum with irradiation
intensity ≥1014 W/cm2 creates a
high-density, high-temperature ablating plasma at the front surface
that launches a dynamic shock inside the material. The shock wave
propagates in the target material and, on unloading at the rear
surface, generates a visible optical emission. The shock transit time
in the material measured with a high-speed streak camera gives the
shock velocity. Recent laser-driven shock wave experiments have
demonstrated high-quality planar shock waves induced by thermal
radiation from a hohlraum cavity with measured pressures as high as 750
Mbar (Cauble et al., 1993; Lower et al., 1994). However, in all
published work on laser-driven shock wave experiments, the pressure has
been determined indirectly from the measurement of the shock velocity
with the use of known EOS of a reference material. Direct measurement
of the EOS data requires simultaneous measurement of particle velocity
and shock velocity, as carried out in nuclear explosion shock wave
experiments. The indirect-drive method using thermal X rays in
laser-heated cavities has demonstrated the feasibility of simultaneous
measurements of shock velocity and particle velocity (Hammel et al., 1993). However, very
recently the absolute EOS points have also been obtained using direct
irradiation (Benuzzi et al., 2002).
An intermediate method known as the “impedance matching
technique” (Koenig et al.,
1995) involves measurement of shock velocity in two materials in
stepped target configurations. The technique is used for measuring the
EOS of one of the materials, using the known EOS of the other material
as reference. In this article, we present the laser-induced shock
velocity measurements in a thin foil single layer (Al) and layered
targets (Al-Au and Al-Cu) using a 2-J/200-ps laser pulse. We use a
high-speed S-20 photo cathode streak camera for shock luminosity
detection. The equations of state of Al, Au, and Cu obtained using
impedance matching techniques are in agreement with the reported
results of SESAME. In Section 1, we report the development of a
2-J/200-ps laser chain of high contrast ratio (>5000:1) that
involves a modified commercial laser oscillator. In Section 2, we
present the experimental results of equation-of-state measurements of
Al, Au, and Cu using impedance matching techniques. Section 3 has the
error analysis and Section 4 has the results and discussion on shock
velocity measurements.
2. LASER SYSTEM
The commercial laser used is a cavity-dumped active–passive
mode-locked system consisting of a Nd:YAG picosecond oscillator and an
amplifier as shown schematically in Figure 1.
The oscillator is based on the novel resonator design utilizing
intracavity spatial filtering and large mode volume that gives a
high-energy laser pulse output along with a smooth beam profile. This
oscillator operates at a 10-Hz repetition rate to compensate for the
thermal lensing in the YAG rod and produces a high energy stability of
∼5%. The cavity dumping in the oscillator is achieved by a
high-speed photodiode–Pockel's cell combination where the
photodiode senses the intensity buildup inside the cavity and generates
a trigger pulse for the high-speed Marx circuit driving the
Pockel's cell when a predetermined intensity level is reached. The
selected output pulse, ejected via a polarizer (P1), is fed to the
Nd:YAG amplifier to give a laser pulse of energy of 70 mJ in a 40-ps
duration at 1.064 μm. The energy and pulse duration are increased
to 180 mJ and 200 ps, respectively, by using a suitable intracavity
etalon. This laser system can be operated in an internal as well as an
external mode. The schematic diagram of the 2-J/200-ps laser chain
that consists of a modified commercial laser oscillator to be operated
in a single shot, external pulse selector (EPS), two silicate Nd:Glass
amplifiers (φ = 20 mm) and Faraday isolator is shown in Figure 2. In this chain, the oscillator is operated
at 10 Hz to maintain the energy stability whereas the EPS, amplifiers,
and Faraday isolator are operated in the single-shot mode. A
centralized control unit controls the charge and fire sequence of
various stages. The heart of the unit is an Intel 8085-based
microcomputer that has serial I/O ports for interfacing data entry
terminal and switches like Charge (Chg), Trigger (Trg), and Dump (Navathe et al., 1996). This microcomputer
controls different power supplies at various stages in the laser chain
through independent control modules. The external operation of the
picosecond laser at 10 Hz is achieved by sending +5 V TTL from the
trigger generator in the control unit to the oscillator power supply.
When an amplified laser pulse in single shot mode is required, the
“Chg” command from the control unit is given that initiate
charging of the capacitor banks of the amplifiers and Faraday isolator.
Once the voltage reaches a set level, “Trg” is initiated
that enables the trigger generator designed for pulsed biasing of the
cavity dumping photodiode (HP 5082-4207) in the commercial oscillator
(Fig. 2) and discharges the capacitor banks
of amplifiers and the Faraday isolator. The trigger generator generates
a pulse in monoshot mode with an amplitude of ∼140 V and a duration
of ∼80 ms that biases the diode to select one pulse from the train
of laser pulses at 10 Hz. This electronic selection of the laser pulse
offers reliability as compared to a mechanical shutter based on stepper
motor or coil control, which is susceptible to electromagnetic
noise.
Schematic of picosecond Nd:YAG commercial oscillator.
Schematic of 2-J/200-ps laser chain.
The selected single pulse output from the oscillator consists of some
mode-locked train of prepulses (separated by a round trip time of
∼8 ns) followed by the main laser pulse with a contrast ratio of
∼50:1. This is due to the finite extinction of the polarizer used
inside the oscillator. Such a pulse is detrimental to conducting
experiments that involve laser ablation of material in thin foils as
the prepulses would puncture the foil before the main pulse arrives. An
EPS consisting of a single Pockel's cell between two crossed
polarizers is designed and incorporated in the laser chain to increase
the contrast ratio to >5000:1. The Pockel's cell is driven by
two step pulses of +3.5 kV and −3.5 kV switching in ∼7 ns.
The high-voltage generators of the step pulses are designed in a Marx
bank configuration where the high-voltage capacitors are charged in
parallel and then discharged in series through the avalanche
transistors 2N5551. Each transistor is carefully selected for the same
breakdown voltage (350 V) and fall time (∼2 ns) with a jitter of
±100 ps. The two high-voltage step pulses are applied to the
electrodes of the Pockel's cell in a floating electrode
push–pull configuration to obtain
Vλ/2 operation. This configuration is
specially used for cavity-dumped, mode-locked oscillators (Koechner, 1992). The trigger pulse for the Marx
circuits is derived from the cavity-dumping photodiode of the
oscillator by putting an additional pulse forming circuit in parallel
with the existing one. This helps in getting a trigger signal well
ahead of the main pulse (40 ns) and in synchronization of the laser
pulse with the selector. The synchronization is achieved by putting
extra passive delay using coaxial cable in the trigger signal to the
Marx circuit of the cavity-dumping Pockel's cell and variable
passive delay in the trigger signal to the external pulse selector
drivers. The energy of the selected main pulse after the EPS is
∼100 mJ. This pulse is fed to two silicate Nd:Glass amplifiers of
gain ∼4.5 to achieve laser energy of ∼2 J in 200 ps.
3. EQUATION-OF-STATE STUDIES
An intense laser beam when focussed on a thin solid target kept in
vacuum with initial density ρ0 (solid density) creates
hot ablating plasma at the surface of the target. Laser energy is
absorbed up to the critical density surface where the plasma frequency
is equal to the laser frequency. Plasma ablation in the early phase of
laser pulse duration launches a compression wave in the target material
due to momentum recoil. The successive compression waves generated by
the laser pulse after the first compression in the initial phase travel
inside the compressed material at a higher velocity than the first and
develop a steady shock wave at approximately the peak of the laser
pulse. However, the laser pulse required for shock generation should be
free from prepulses. The shock wave propagates in the target with a
shock velocity us and imparts a particle
velocity up to the material behind the
shock front. After the laser pulse is over, the ablation pressure and
velocity begin to drop rapidly. At this time, a rarefaction wave sets
in and propagates from the ablation front into the shock-compressed
target. The ablation-driven shock wave propagates in the target
material and heats the compressed material, thereby generating a
visible optical emission when the shock unloads at the rear surface of
the target.
The experimental setup to detect laser-induced shock in metals is
shown in Figure 2. A good spatial beam
quality (TEM00) and high-contrast laser pulse of an energy
of 2 J and a duration of 200 ps from the developed laser chain is used
as a driver. There were no measurable hot spots in the laser beam
(measured with a beam profiler), which is due to an intracavity spatial
filter and apodizer used inside the oscillator, giving TEM00
output, and also due to the fact that only two amplifiers were used in
the laser chain. The optical surfaces in the laser chain were kept
meticulously clean to avoid any diffraction-caused spatial intensity
variations in the laser beam intensity profile. The intense picosecond
laser pulse is focused to a spot diameter of ∼100 μm by an
aspheric lens (focal length ∼400 mm and diameter ∼50 mm) on a
planar foil target kept inside a vacuum chamber (pressure
∼10−3 mbar). The high intensity
(∼1014 W/cm2) created on the front
surface of the foil produces ablation of material and launches a shock
wave in the medium, generating thermal radiation on unloading at the
rear surface. The hot emitting rear surface behaves like a black body
and its luminescence emissivity signal is collected and focused by a
lens on the entrance slit (width ∼100 μm, length ∼6 mm) of
a high-speed visible (S-20) streak camera (Rai
et al., 1995). A CCD camera captures the streak image,
which is acquired on a personal computer (PC) through a frame grabber
card for final image processing. The streak camera that is used for
detection of the shock breakout signal has temporal and spatial
resolutions of ∼5 ps and 100 μm, respectively. A fiducial
signal that serves as a marker for the arrival of the laser pulse on
the front surface of the foil is also recorded, along with
shock-induced luminescence by the streak camera each time the laser is
fired on the target foil. This has been achieved by picking up 4% laser
reflections from a glass plate (wedge angle ∼0.5°), placed
before the focusing lens, and converting it to visible radiation by a
second harmonic crystal 20 mm long and then sending it along an optical
fiber cable at the vertical slit input of the streak camera. In the
slit, the fiber tip rests below the laser focal spot where radiations
from the target are expected to reach. The streak camera is triggered
from a photodiode that is also used to trigger the Pockel's cell
inside the laser cavity for cavity dumping. To synchronize the streak
operation with the arrival of the main laser pulse, the laser pulse is
optically delayed by means of a pair of fully reflective mirrors
(M1 and M2) before it is focused on to a target.
The arrival of the laser pulse at the streak input without any target
material in the beam path is synchronized with the arrival of the
fiducial signal by introducing an optical delay loop consisting of four
fully reflecting mirrors (M3, M4,
M5, and M6) between the focusing lens and the
beam sampling glass plate (for fiducial arrangement). The synchronized
fiducial and main laser pulse recorded is shown in Figure 3.
CCD image of the synchronized fiducial and main laser pulse.
Planar Al foil targets of different thicknesses were made from a
standard aluminum foil (density ∼2.7 gm/cm3) of 12
μm thickness by a controlled chemical etching process. Purified
NaOH pellets (assay content ∼97%) were dissolved in water and the
solution was used to etch the foil. Each foil was first carefully fixed
on a Plexiglass frame in such a manner as to make the surface of the
foil free. The assembly was then immersed in the etching bath and held
vertically during the operation. By controlling the etching time, a
foil thickness down to 3.2 μm was achieved. The foil thickness was
measured by weighing the foil sample of a definite dimension (2.5
× 2.5 cm2) on a microbalance. Target dimensions were
chosen such that they satisfy the following criteria:
a. The laser focal spot diameter was small
enough to produce the required high intensity, yet it was much larger
than the target thickness to minimize two-dimensional (2D) effects, and
hence, to ensure a planar shock (Trainor et
al., 1979).
b. The width of the target was much larger than the diameter of
the laser focal spot to avoid weakening of the shock strength by an edge
rarefaction wave. This is also required to avoid the ablation plasma
flow around the foil edges, which otherwise disturbs the luminosity
record.
c. After termination of the driving laser pulse, a rarefaction
wave propagates at a speed higher than the shock wave and eventually
overtakes and attenuates it. Therefore, to ensure steady shock
propagation (i.e., to avoid shock decay), the target thickness was
chosen such that it satisfies the condition: d ≤
2usτ, where d is target
thickness, us is the shock velocity in the
material, and τ is the laser pulse length. Typical shock velocity
in Al is ∼106 cm/s and τ = 200 ps (FWHM of
pulse) (Gu et al., 1996).
d. The thickness of the target was kept larger than the range of
suprathermal electrons and hard X rays to avoid preheat reaching ahead
of the shock wave on the rear side of the target.
We have used planar Al foils (typically 3 to 4 mm wide) of thickness
3.2 μm, 4.6 μm, 5 μm, 6 μm, 7 μm, and 8 μm. The
shock breakout times for different targets were recorded at the same
absorbed laser intensity. This was ensured by selecting only those data
points from the large number of shock transit time data taken for each
thickness of the foil used where the intensity was very nearly the
same. The absorbed laser intensity data was estimated in a separate
experiment by measuring the spatially integrated reflection over the
entire front half space of the target surface at various incident laser
intensities using an Ulbricht integrating sphere (H.C. Pant, unpubl.).
A typical experimental observation of shock emergence seen as strong
luminosity as recorded by the streak camera is shown in Figure 4. The shock transit times (t) were
calculated with respect to the peak of the fiducial signal using the
image-processing software PROMISE (Vora et
al., 1996) and it was found to be linear up to a foil
thickness (d) of ∼7 μm. The average shock velocity is
found to be ∼2.09 × 106 cm/s for an absorbed
laser intensity of ∼5 × 1013 W/cm2
as shown in Figure 5 by taking the inverse of
the slope of the best linear fit to the data in
t–d plane. Using a similar method, the shock
velocity of ∼1.78 × 106 cm/s is found at an
absorbed laser intensity of ∼3 × 1013
W/cm2.
Streak record of the fiducial and shock luminosity signals in
5-μm Al target.
Shock transit time versus thickness of Al foil for shock velocity
calculation.
The particle velocities corresponding to the experimentally measured
shock velocities were calculated from the
us and up
relationship (Marsh, 1980) using
where us and
up are shock and particle velocities in
units of 106 cm/s. The shock pressures were calculated
from the standard Rankine–Hugoniot relationship:
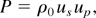
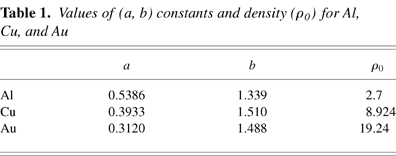
where ρ0 (gm/cm3) is the solid density.
The values of a, b, and ρ0 for Al, Cu,
and Au used are given in Table 1.
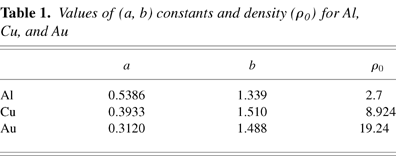
Values of (a, b) constants and density (ρ0) for Al,
Cu, and Au
One dimensional radiation hydro code MULTI simulations (Ramis et al., 1988; Pant et al., 2002) revealed that steady
shock wave propagation through Al foil requires a minimum thickness of
∼3.4 μm to achieve stability as shown in the Figure 6, which represents the simulated pressure
profile (using MULTI) at different times when the laser pulse first
strikes the target surface and at different distances from the front
surface. Because the series of shock waves launched in the material
during the initial rising portion of the laser pulse is in the
accelerating phase and attains steadiness only after the peak of the
laser pulse (200 ps from the base of the laser pulse as shown in Fig. 6), the fact that the shock transit time is
measured from the peak of the laser pulse is obvious. This also shows
that a steady shock reaches the target rear surface. The thickness of
the foil target should be taken such that the shock wave is able to
traverse it before the laser pulse is over. A 5-μm Al foil procured
from a standard manufacturer (thickness variation ∼±0.1
μm) is thus used as a reference material to find the EOS of Au and
Cu using the impedance matching technique. Au layers of ∼1.75
μm and ∼1.5 μm thick were deposited on the rear surface of
Al foil employing chemical and vacuum deposition techniques,
respectively. A pressure of 10−5 bar was maintained in
the vacuum deposition system. Similarly ∼1-μm copper was
deposited on the Al foil using vacuum deposition techniques. Suitable
changes were made in the process to obtain good adhesion and to keep
variation in the deposited thickness within ±0.05 μm.
Simulated pressure profiles showing steady shock propagation using
MULTI 1-D hydrocode.
The shock transit time was determined for two-layer targets of 5
μm Al + 1.75 μm Au and 5 μm Al + 1.50 μm Au at the same
absorbed laser intensities of 5 × 1013
W/cm2 and 3 × 1013
W/cm2, respectively, used for reference material Al.
Here also, only those data points were selected, from the large number
of shots taken for determining the shock transit time in layered
targets, where the laser intensity was very nearly equal. As the shock
transit time for the reference material Al was known, the shock transit
time for the Au layers was calculated. The measured shock velocity in
pure Al and layered Al + Au targets along with the calculated particle
velocity and shock pressure using Eq. (1) and Eq. (2) are shown in
Table 2. Typical streak records of the pure
Al target along with the layered Al + Au target are shown in Figure 7a and Figure 7b,
respectively. The shock luminosity signals recorded in the two targets
clearly show the relative shock transit time delay. The shock transit
time was also determined in a similar way for a 5-μm Al + 1-μm
Cu layered target for absorbed laser intensities of 4.4 ×
1013 W/cm2 and 3.9 × 1013
W/cm2. The measured shock velocity in pure Al and
layered Al + Cu targets along with the calculated particle velocity and
shock pressure are shown in Table 3.
EOS data for Al-Au target
EOS data for Al-Cu target
a: Streak record of the shock luminosity signal in Al target. b:
Streak record of the shock luminosity signal in Al-Au layered
target.
In the experiment, the target consists of Al foil as the reference
material and Au (or Cu) as a test material deposited on the rear
surface. Because the density of Au is higher compared to that of Al,
its Hugoniot curve, defined as the locus of all the possible states in
which a material can exist after shock wave compression, is located
above that of Al. When a shock arrives at the interface between the two
materials, a shock wave is partly transmitted through Au and partly
reflected in Al. We deduce the shock velocities
us using the time difference in the shock
luminosity signal recorded by the streak camera. As shown in Figure 8, red and magenta lines are the Rayleigh
lines (with slope values equal to the shock impedance
ρ0us) that represent the
final state of adiabatically compressed material (Al) attained with
respect to an initial state on a pressure Hugoniot curve (black). The
two slope values ρ0us for
Al in the P–up plane
intersect the Hugoniot curve at the points (1.16,6.58)1 and
(0.93,4.46)2 at an absorbed laser intensity of ∼5
× 1013 W/cm2 and ∼3 ×
1013 W/cm2, respectively. The reflected
Hugoniot for Al at the two absorbed laser intensities (light and dark
blue dotted curves) could be represented as H1
(2up(Al) −
up), where H1
(up) is the shock Hugoniot for Al and
up(Al) is the particle velocity induced by
a shock wave in Al. The intersection of the Rayleigh lines for Au
(green and gray) with the reflected Hugoniot curves for Al gives the
EOS point of Au on the pressure Hugoniot curve (orange). The final
state determined this way, as shown in Figure
8, gives pressure and the particle velocity in Au as 13.47 Mbar
and 0.59 × 106 cm/s and 9.01 Mbar and 0.47 ×
106 cm/s at absorbed laser intensities of 5 ×
1013 W/cm2 and 3 × 1013
W/cm2, respectively. Figure 9
shows the final state of Cu with respect to the initial state on a
pressure Hugoniot curve that also lies above that of Al. The pressure
and particle velocity in Cu is found to be 10.38 Mbar and 0.76 ×
106 cm/s and 8.90 Mbar and 0.70 × 106
cm/s at absorbed laser intensities of 4.4 × 1013
W/cm2 and 3.9 × 1013
W/cm2, respectively.
Graph representing final state of Au on pressure Hugoniot using an
impedance matching technique.
Graph representing the final state of Cu on pressure Hugoniot using
an impedance matching technique.
4. ERRORS ANALYSIS
The laser-induced shock generation in thin metal targets for EOS
studies aims at minimizing the possible errors that are introduced in
the shock velocity, particle velocity, and pressure. The impedance
matching technique analysis involves: (1) Evaluation of theEOS
(P, ρ, E, us,
up) point in the reference material from
the measured shock velocity, (2) construction of reflected pressure
Hugoniot curve at the reference point of the shock reflected in the
unknown material, (3) intersection of the reflected Hugoniot with the
Rayleigh line of unknown material that gives the common EOS point in
the P–up plan. Thus at the
intersection point of the reflected Hugoniot and the Rayleigh lines the
error in pressure is quadrature and is given by
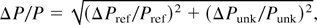
where ΔPref/Pref is
the error in the reference material (Al) and
ΔPunk/Punk is the error
in the unknown material used (Cu). Because P =
ρ0usupαρ0us2
on a pressure Hugoniot curve and
Pαρ0us on a
Rayleigh line, Eq. (3) leads to
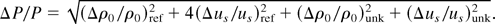
Ignoring the density errors in reference and unknown material (Rothman et al., 2002), Eq. (4) leads to
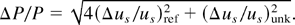
The fractional errors are assumed to be independent but they are
equal for each step. The possible errors that contribute to the shock
velocity are errors due to the foil thickness variation, transit time,
and streak camera calibration. Thus, from Eq. (5), the variation in
shock velocity can be written as
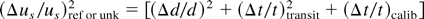
where Δd/d is the thickness variation
error, (Δt/t)transit is the
transit time error, and
(Δt/t)calib is the error in streak
camera calibration.
The variation in the coating thickness for vacuum deposition is given
by
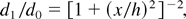
where d0 is the deposited thickness at the center
and d1 is deposited thickness at the target
boundary with dimensions of 25.4 mm × 25.4 mm. In the coating
unit x = 12.7 mm from the center of the target whereas the
height of the target from the source was h = 100 mm. Thus the
deposited thickness by vacuum deposition has a variation of
∼±0.05 μm for Au as well as Cu. The thickness variation
of the Au deposited by the chemical process is also assumed to be the
same as vacuum deposition because the deposition area is very small.
The streak camera used in our experiment has a temporal resolution of
∼5 ps for the streak rate of 15.62 mm/ns. Therefore, for a
streak camera calibration error
(Δt/t)calib ∼ 0.3% and a
transit time CCD pixel value of ∼3.2 ps, the error in pressure for
Al (reference) is calculated to be ∼ ± 5% from the
relation
whereas the errors in pressure for Au and Cu as per Eq. (5) are
∼±7% and ±9%, respectively. The calculated errors in
shock velocity, particle velocity, and pressure for Al, Au, and Cu are
shown in Table 2 and Table
3.
5. RESULTS AND DISCUSSION
We have compared the experimental data for shock velocity in Al, Au,
and Cu with the SESAME data (Bennet et al.,
1978; Batani et al., 1996;
Benuzzi et al., 1996a,
1996b; Evans et al., 1996a, 1996b;
Jones et al., 1966; Altshuler et al., 1962; Mitchell et al., 1991; Trunin
et al., 1969; Kormer et al.,
1962). Figures 10 and 11 show the experimental data points plotted on the
SESAME curve of Au and Cu, respectively, along with the results of other
laboratories. The experimentally obtained a and b
constants as per Eq. (1) are in close agreement with the LASL data (Marsh, 1980). The published data gives (a,
b) = (0.312,1.51) for Au and (0.3933,1.51) for Cu, which
compares well with the experimental values of (a,
b)Au = (0.312,1.495) and (a,
b)Cu = (0.3933,1.509). The experimental pressure
multiplication factor (m = PAu or
Cu/PAl) of 2.01 (for Au) and 1.66 (for
Cu) at the interface of the layered targets is in close agreement with
the theoretical formulations. The simulation result for the two layered
targets also predicts similar results of pressure enhancement (Pant et al., 2002). Our shock velocity
measurements and Hugoniot EOS points are in fairly good agreement
within the shock transit time error of ±3% and ±7% for
reference and unknown materials, respectively. Thus, our shock pressure
values determined are correct within an error of ±5% for
reference material and ±7% to ±9% for the unknown
material as per the error analysis. The low values of errors obtained
in the present experiment are due to the use of a high-resolution
streak camera that resulted in low transit time error. Further, the
optimized streak velocity ∼15.62 mm/ns used in the experiment
has resulted in a low CCD pixel value error and has helped in reducing
the possible errors, which is not possible otherwise in experiments
that use step targets (Koenig et al.,
1995). The small area of the reference material used for
deposition of the test materials has also helped in reducing the errors
as per Eq. (7). However, the overall errors in pressure can be
minimized within ±3% to ±5% by using random phase plates
or phase zone plates (Gu et al.,
1996) in a direct-drive approach where the uniform laser
illumination generates a planar shock or indirect drive approach (Rothman et al., 2002). In the present
experiment, no attempt was made to achieve a flat shock profile.
However, special care was taken in removing prepulses present in the
laser beam. The sharp rising shock luminosity signal shows that the
preheating effects are negligible in our case (Benuzzi et al., 1998). This fact was also
corroborated from the simulated temperature profiles using the MULTI
1-D code as shown in Figure 12. According to
the simulation results, the temperature of the rear surface of the
target remains at only 0.2 eV, which is nearly insignificant relative
to the shock luminosity signal appearing from the rear side due to the
∼5 eV temperature. The optimum foil thickness of 5 μm for Al
has also helped in minimizing the preheat effects due to hard X rays
(Honrubia et al., 1998). This has
been explained on the basis of various opacity models suggesting that
for a thin foil target (Al at 5.0 μm or so), the suprathermal X-ray
component (0.8–1.5 keV) will not have ample time to heat the rear
surface of the foil and the shock signal will pass long before the
arrival of such X-ray photons. Typically, such hard X-ray components
takes about 1–2 ns to diffuse to the rear surface. Further, the
laser intensities are well within the limits where hot electrons
generation may not lead to the preheat effect.
EOS Hugoniot data points (Au) of present experiment and other
laboratories on SESAME curve.
EOS Hugoniot data points (Cu) of present experiment and other
laboratories on SESAME curve.
Simulated temperature profiles representing negligible preheat using
MULTI 1-D hydrocode.
6. CONCLUSIONS
The commercial mode-locked laser has been modified for single shot
operation to produce a laser pulse of high contrast. The external pulse
slicer designed helps in increasing the contrast ratio to >5000:1.
We have shown the feasibility of using a simple laser driver system
(Nd:YAG, 2 J, 1.06 μm, 200 ps) for conducting shock-wave
experiments for EOS measurements of gold and copper in a thin layered
target at moderate pressures in the 8–13 Mbar range. A fast
streak camera system coupled with a fiber-based fiducial signal marker
records the shock breakout times. The results are quite consistent with
theoretical as well as experimental data published by other
laboratories. An impedance matching technique was successfully used to
find the pressure in gold and copper in multilayered Al + Au and Al +
Cu targets within errors of ±10%. The laser-driven shock
velocities and particle velocities obtained for gold and copper are in
excellent agreement with the reported results of other laboratories
including SESAME data. The pressure amplification factors of 2 and 1.66
have been derived in Al + Au and Al + Cu layered targets, respectively,
as per the theoretical calculations and MULTI simulations.
ACKNOWLEDGMENTS
We are extremely grateful to Dr. D.D. Bhawalkar for his constant
support and guidance in the present work. We are also indebted to Dr.
S.K. Sikka for fruitful discussions and many helpful suggestions. Dr.
S.N. Vyas and Mr. R.K. Khare and his colleagues are acknowledged for
their help in target fabrication and characterization. Members of high
power glass laser and electronics group deserve our special thanks for
their technical help and support during the experiment.
