Hostname: page-component-745bb68f8f-s22k5 Total loading time: 0 Render date: 2025-02-06T14:09:24.153Z Has data issue: false hasContentIssue false
  • English
  • Français

Enhancement of laser induced shock pressure in multilayer solid targets

Published online by Cambridge University Press:  06 March 2006

H.C. PANT ,
M. SHUKLA ,
H.D. PANDEY ,
YOGESH KASHYAP ,
P.S. SARKAR ,
A. SINHA ,
V.K. SENECHAM  and
B.K. GODWAL
H.C. PANT
Affiliation:
High Pressure Physics Division, Bhabha Atomic Research Centre, Mumbai, India
M. SHUKLA
Affiliation:
High Pressure Physics Division, Bhabha Atomic Research Centre, Mumbai, India
H.D. PANDEY
Affiliation:
Centre for energy studies, Indian Institute of Technology, Hauz Khas, New Delhi, India
YOGESH KASHYAP
Affiliation:
High Pressure Physics Division, Bhabha Atomic Research Centre, Mumbai, India
P.S. SARKAR
Affiliation:
High Pressure Physics Division, Bhabha Atomic Research Centre, Mumbai, India
A. SINHA
Affiliation:
High Pressure Physics Division, Bhabha Atomic Research Centre, Mumbai, India
V.K. SENECHAM
Affiliation:
Center for Advanced Technology, Indore, India
B.K. GODWAL
Affiliation:
High Pressure Physics Division, Bhabha Atomic Research Centre, Mumbai, India
Rights & Permissions [Opens in a new window]

Abstract

The impedance mismatch technique was used for shock pressure amplification in two layered planar foil targets. Numerical simulation results using one-dimensional (1D) radiation hydrocode MULTI in two layer target consisting of polyethylene (CH2)n-aluminium (Al) and polyethylene (CH2)n-gold (Au), show a pressure enhancement of 12 and 18 Mbar, respectively (or a pressure jump of 1.64 and 2.54, respectively), from initial pressure of 7 Mbar in the reference material (polyethylene) using laser intensity of 5 × 1013 Watts/cm2 at 1.064 μm. The simulation data was also corroborated by experiments in our laboratory. Results of laser driven shock wave experiments for pressure enhancement studies in CH2-Al and CH2-Au targets are also presented. A Nd:YAG laser chain (2 J, 1.064 μm wavelength, 200 ps pulse duration FWHM) is used for generating shocks in the planar CH2 foils of thickness varying from 4 to10 μm, and in two layered CH2-Al (or CH2-Au) targets with 8 μm CH2 and 1.5 μm Al or Au .

Keywords

Equation of state (EOS). Pressure amplificationShock pressure
Type
Research Article
Information
Laser and Particle Beams , Volume 24 , Issue 1 , March 2006 , pp. 169 - 174
Copyright
© 2006 Cambridge University Press

1. INTRODUCTION

Intense laser and ion beam induced shocks have found use in the field of high pressure and high energy density physics studies viz. equation of state (EOS) measurements, phase transformations, laboratory simulation of high energy density astrophysical phenomenon, inertial confinement fusion etc. (Lindl, 1995; Celliers et al., 2000; Remington et al., 2000; Temporal et al., 2005; Trusso et al., 2005; Hoffmann et al., 2005; Phillippe et al., 2004). Laser driven shock wave experiments conducted using direct/indirect laser irradiation of solid matter have proven that laser driven shocks are most viable tool for generating shock wave pressure as large as 750 Mbar (Cauble et al., 1993). To achieve higher shock pressures, one must use high laser intensities (>1014 Watts/cm2). However, at higher laser intensities, collective laser absorption processes dominate generating hot electrons and hard X-rays, leading to preheat of the target under investigation. This causes difficulty in creating high target compression. To mitigate these effects, the absorbed laser intensity, I (in Watts/cm2) and the wavelength, λ (in μm) product (Iλ2) is desired to be ≤ 1014, where the laser plasma interaction remains in the collisional absorption regime. At the same time, it is desirable to achieve shock pressures of tens of Mbar for various applications. Therefore, it is really interesting to fully exploit the technique of laser driven shock wave generation, which can be easily performed in a laboratory, with the help of pulsed moderate intensity (<1014 Watts/cm2) laser systems, and a high speed streak camera. Of course, one has to take due precautions in such experiments, to check and keep the X-ray preheating at a bare minimum, and also ensure planar and spatially uniform shocks in the material under test, so that the quantitative EOS related measurements are made with much better accuracy. With the advent of laser beam profile smoothing techniques, direct drive method can now be used to study solid matter at extreme pressure (10 to100 Mbar) with relatively better accuracy of shock wave velocity (1 to 2%), and pressure (3 to 4%) of importance to several astrophysical phenomena and also for inertial fusion energy research (Rothman et al., 2002).

Impedance mismatch techniques in the past were used to measure the EOS of various solid materials (Batani et al., 1996, 2002a, 2002b; Benuzzi et al., 1996; Hall et al., 2002; Koenig et al., 1995; Pant et al., 2002; Shukla et al., 2003). This technique consists of measuring the shock velocity simultaneously in two different materials. This makes possible to achieve a relative determination of one EOS point of one material (material under test) by taking the EOS of another material as a reference. An interesting point worth noting is that apart from providing an easy means of measuring EOS of a test material, impedance mismatch method also provides a very simple means to enhance the shock pressure at the interface of the two materials with negligible pre-heat (since the laser intensity can be kept well below thresholds of the collective processes to achieve high compression), provided that the shock impedance of the test material is higher than that of the reference material. We propose use of multilayered targets (two or more layers) for high pressure generation up to 30 Mbar using this technique. From laser irradiation side, the shock impedance of the layers is kept in ascending order. The impedance mismatch at the interface of the layers causes pressure jump, when a shock launched from the low impedance layer reaches the interface. The pressure increase depends on the shock impedance mismatch of the layers.

In this paper, we present simulation and experimental studies of pressure enhancement in two layered targets, irradiated by a pulsed Nd:Glass (λ = 1.064 μm) laser of intensity (∼5 × 1013 Watts/cm2). The targets used for the study were (CH2)n-Al and (CH2)n-Au planar foils. In the first part of the paper, we will describe the numerical simulations to estimate the shock pressure enhancement at the interface of the (CH2)n-Al and (CH2)n-Au layers when the laser induced ablative shock is launched from the (CH2)n side. Our 1D radiation hydrocode MULTI simulations shows that using a low density plastic (CH2)n layer on Al or Au substrate, produce a shock pressure enhancement of ∼1.64 and ∼2.54, respectively, at the interface (Ramis et al., 1988). The second part of the paper describes the experimental details of the pressure enhancement studies performed using (CH2)n-Al and (CH2)n-Au two layered targets consisting of 8 μm of CH2 and 1.5 μm Al or Au. The experimental data obtained shows a close agreement with the simulation results.

2. NUMERICAL SIMULATION

In laser induced shock experiments aimed at measurement of EOS, the shock pressure (Mbar) generated due to laser ablation can be obtained from the scaling law as (Lindl, 1995)

where IL is the laser intensity (Watts/cm2), λ is the laser wavelength, A and Z are the atomic mass and atomic number, respectively, of the target material. It is essential to ensure that the planar shock wave front propagate in a steady state condition through the reference and the test material. This requires a proper choice of the target thickness. In case of very thin targets, the shock breakout occurs much earlier in the laser pulse peak time, leading to an unsteady shock front (still in acceleration phase). On the other hand, rarefaction wave from the laser irradiation side can interact in case the target is too thick. Thus, for a steady state shock front propagation, the target should justify the condition (Gu et al., 1996)

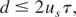
where d is the target thickness, us are the shock velocity and τ are the laser pulse duration (FWHM). Further, the laser focal spot diameter should be larger than the target thickness in order to avoid two-dimensional (2D) effects and thus to ensure a planar shock front (Trainor et al., 1979).

A proper radiation hydrodynamic simulation serves as an important tool in predicting proper target thickness that will ensure a steady state shock wave propagation condition. In our case, multilayered targets were studied in detail using 1D radiation hydrocode MULTI. This code uses multi group method of radiation transport coupled with Lagrangian hydrodynamics based on a fully implicit numerical scheme. Material properties like EOS, Planck and Rosseland opacities are used in a tabular form and are generated externally.

The simulations performed initially for a single layer of (CH2)n target (954 mg/cc) for absorbed laser irradiation of 5 × 1013 Watts/cm2 (λ = 1.064 μm, pulse duration (FWHM) = 500 ps) with sine2 pulse shape, suggested that the (CH2)n thickness must be thicker than 18 μm in order for the shock front reaching the target rear surface in a stationary condition. This also agrees well with the criteria that the target thickness should be less than 2usτ. Simulations also indicate that the shock front reaches a steady state in a time equal to the laser pulse rise time. In our case, this is 500 ps. The shock velocity deduced from the pressure profiles in plastic is found to be 3.5 × 106 cm/s and gives a maximum thickness of 34 μm for steady shock propagation. This velocity is in close agreement with the LASL data (Marsh, 1980). The final pressure in (CH2)n is observed to be ∼7 Mbar. Simulations were also performed for two layer (CH2)n-Al and (CH2)n-Au targets. These targets were chosen to provide a large density mismatch at the (CH2)n-Al (or Au) interface and inhibit generation of significant X-rays using low Z ablator. Figure 1 and Figure 2 show simulated time and space resolved shock pressure profiles in two layer (CH2)n-Al and (CH2)n-Au targets with the laser incident from (CH2)n surface. The thickness of (CH2)n, Al and Au is taken to be ∼22 μm, 7 μm, and 5 μm, respectively. A pressure multiplication of ∼ 1.64 is observed in (CH2)n-Al target as compared to ∼2.54 in (CH2)n-Au target. This matches well with the ideal gas formula viz.

here, P1 and P2 are the pressures in the first and the second material of density ρ1 and ρ2, respectively. The final pressure in two layer (CH2)n-Al and (CH2)n-Au targets is observed to be ∼12 Mbar and ∼18 Mbar, respectively, for an initial pressure of ∼7 Mbar in (CH2)n.

Simulated pressure profiles in (CH2)n-Al.

Simulated pressure profiles in (CH2)n-Au.

3. EXPERIMENTAL STUDIES

Experiments to observe pressure enhancement were conducted using a 2 J/200ps (FWHM), Nd:Glass laser (λ = 1.064 μm) (Pant et al., 2002; Shukla et al., 2003). The absorbed laser intensity was varied from 3 × 1013 Watts/cm2 to 5 × 1013 Watts/cm2. Eq. (2) suggests the use of total target thickness of ∼12 μm for steady state shock propagation for laser pulse duration of 200 ps. Simulation results also predict a μm minimum target thickness of ∼3 μm for any significant increase in rear side temperature (observed rear side temperature 0.1 eV) due to X-ray preheat. Thus, the target thickness of (CH2)n was chosen to be 8 μm. This target was primarily coated with a 100 A thick layer of Al on both the sides to avoid any possible interference of the front plasma luminosity with shock luminosity at the target rear surface. Similarly, layered targets consisting of ∼8 μm (CH2)n and ∼1.5 μm Al (or Au) were used to observe pressure enhancement using impedance mismatch technique.

The total target thickness was chosen so as to satisfy the steady state shock propagation condition as per Eq. (2). These targets were also pre-deposited with a layer of 100 A of Al on the front side (laser facing) of (CH2)n before depositing Al or Au on the rear side in order to avoid premature plasma formation at the (CH2)n-Al or Au rear side interface. Shock luminosity signal at the rear surface of the target kept under vacuum (10−3 torr), was recorded with a high speed S-20 photo cathode streak camera. The camera had a temporal resolution of 5 ps. A time fiducial signal, generated by converting 4% of the laser energy into green harmonic radiation was recorded simultaneously along with the shock luminosity signal with the help of an optical fiber. Figure 3 shows a typical shock luminosity signal recorded using a polyethylene target, along with the fiducial signal. The shock transit time for a given target thickness was determined from the peak of the laser signal. Shock velocity in polythene (used as a reference material) was determined using a set of thin foils of varying thickness from 4 to12 μm for a given laser intensity. The particle velocity and shock pressure was calculated using Rankine-Hugoniot relations (Zel'dovich & Raizer, 1976):

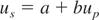
and

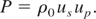
Where us, up, ρ0, and P is the shock velocity, particle velocity, density of the material and pressure, respectively, and (a, b) are the constants. The a, b, and ρ0 for CH2, Al and Au are as follows:

Shock pressure in polyethylene target was found to be ∼5 Mbar and 7 Mbar at absorbed laser intensities of 3 × 1013 Watts/cm2 and 5 ×1013 Watts/cm2, respectively, as given in Table 1. The shock pressure at the interface ((CH2)n-Al and (CH2)n-Au) was determined using impedance mismatch technique by drawing Hugoniot and reflected Hugoniot curves as shown in Figure 4a and Figure 4b and Figure 5a and Figure 5b for absorbed laser intensities of 3 × 1013 Watts/cm2 and 5 × 1013 Watts/cm2, respectively. The intersection of the reflected Hugoniot of the laser facing (CH2)n (reference material) with the Hugoniot of the Al or Au (unknown material) gives the final state and the pressure at the interface. Final pressure in Al was observed to be 8.01 Mbar and 10.92 Mbar at absorbed laser intensities of 3 × 1013 Watts/cm2 and 5 × 1013 Watts/cm2, respectively, with a pressure amplification of ∼1.58 as given in Table 1. Similarly, the final pressure in case of Au is observed to be 13.51 Mbar and 18.73 Mbar at absorbed laser intensities of 3 × 1013 Watts/cm2 and 5 × 1013 Watts/cm2, respectively, with a pressure amplification of ∼2.7.

Shock Luminosity signal in 8μm (CH2)n target.

Observed shock transit time, shock velocity, and shock pressure in two layered targets

(a) Hugoniot curves for (CH2)n-Al target. (b) Hugoniot curves for (CH2)n-Al target.

(a) Hugoniot curves for (CH2)n-Au target. (b) Hugoniot curves for (CH2)n-Au target.

4. RESULTS AND DISCUSSION

The simulation results performed using radiation hydrocode MULTI for two layered targets, show a pressure enhancement of ∼1.64 and ∼2.54, respectively, at the interface(s) of (CH2)n-Al. and (CH2)n-Au two layered targets due to impedance mismatch. This data is in close agreement with the experimentally observed values of ∼1.58 and ∼2.7 for (CH2)n-Al. and (CH2)n-Au targets, respectively. The simulation and the experimental results are also in close agreement with the LASL data (Marsh, 1980). Interestingly, the observed pressure enhancement also matches well with that predicted by the ideal gas formula as per Eq. (3).

5. CONCLUSION

In conclusion, we may say that that high shock pressures in the range of 10 to 20 Mbar can be generated in two layered targets using very modest laser intensity, employing impedance mismatch technique. Selection of target material layers in increasing order of shock impedance and an optimized thickness of layers target thickness for steady state shock propagation leads to generation of high pressure. This pressure enhancement can be further increased by using low density polymer foam in place of normal polythene. Low density polymer as an ablator and low impedance layer has a few more advantages such as high ablation efficiency, low X-ray yield and mitigation of spatial laser intensity effects on the shock front profile. The initial pressure in the reference material can also be increased using laser light at 0.53 μm or lower, since in this case the absorption of laser is dominated by collisional process and also produces initial high ablation pressure.

ACKNOWLEDGMENTS

The helpful discussions in carrying out the simulation studies with Dr. R. Ramis are acknowledged. We also thank Dr. Tara Desai for providing the two layered targets used in our experiment. Authors H.C. Pant and H.D. Pandey express their thanks to the Department of Science and Technology, India, for a grant to this work.

References

REFERENCES

Batani, D., Bossi, S., Benuzzi, A., Koenig, M., Faral, B., Boudenne, J.M., Grandjouan, Nocolas, Atzeni, S. & Temporal, M. (1996). Optical smoothing for shock-wave generation: Application to the measurement of equation of state. Laser Part. Beams 14, 211–221.Google Scholar
Batani, D., Balducci, D., Bretta, D., Bernarddinello, A., Lower, Th., Koenig, M., Benuzzi, A., Faral, B. & Hall, T. (2002a). Equation of state data for gold in the pressure range < 10 TPa. Phys. Rev. B 61, 9287–9294.Google Scholar
Batani, D., Desai, T., Lucchini, G., Lower, T.H. & Hall, T. (2002b). Pressure amplification in thermal x-ray irradiated foam layered gold targets. Laser Part. Beams 20, 165–169.Google Scholar
Benuzzi, A., Lower, Th., Koenig, M., Faral, B., Batani, D., Beretta, D., Danson, C. & Pepler, D. (1996). Indirect and direct laser driven shock waves and applications to copper equation of state measurement in 10–40 Mbar pressure range. Phys. Rev. E 54, 2162–2165.Google Scholar
Cauble, R., Phillion, D.W., Hoover, T.J., Holmes, N.C., Kilkenny, J.D. & Lee, R.W. (1993). Demonstration of 0.75 Gbar planar shocks in x-ray driven foils. Phys. Rev. Lett. 70, 2102–2104.Google Scholar
Celliers, P.M., Collins, G.W., Da Silva, L.B., Gold, D.M., Cauble, R., Wallace, R.J., Foord, M.E. & Hammel, B.A. (2000). Shock induced transformations of liquid deuterium into a metallic fluid. Phys. Rev. Lett. 84, 5564–5567.Google Scholar
Gu, Y., Fu, S., Jano, W.V., Yu, S., Ni, Y. & Wang, S. (1996). Equation of state studies at SILP by laser driven shock waves. Laser Part. Beams 14, 157–169.Google Scholar
Hall, T., Batani, D., Nazarov, W., Koenig, M. & Benuzzi, A. (2002). Recent advances in laser-plasma experiments using foams. Laser Part. Beams 20, 303–316.Google Scholar
Hoffmann, D.H.H., Blazevic, A., Ni, P., Rosmej, O., Roth, M., Tahir, N.A., Tauschwitz, A., Udrea, S., Varentsov, D., Weyrich, K. & Maron, Y. (2005). Present and future perspectives for high energy density physics with intense heavy ion and laser beams. Laser Part. Beams 23, 47–53.Google Scholar
Koenig, M., Faral, B., Boudenno, J.M., Batani, D., Bennuzi, A., Bossi, S., Remond, C., Perrine, J.P., Temporal, M. & Atzeni, S. (1995). Relative consistency of equations of stsate by laser driven shocks. Phys. Rev. Lett. 74, 2260–2263.Google Scholar
Lindl, J. (1995). Development of indirect-drive approach to inertial confinement fusion and target physics basis for ignition and gain. Phys. Plasmas 2, 3933–4024.Google Scholar
Marsh, S.P. (ed.) (1980). LASL Shock Hugoniot Data. Berkeley: University of California Press.
Pant, H.C., Shukla, M., Senecha, V.K., Bandypadhyay, S., Rai, V.N., Khare, P., Bhatt, R.K., Godwal, B.K. & Gupta, N.K. (2002). Equation of state studies using laser-driven shock wave propagation through layered targets. Current Science 82, 149–157.Google Scholar
Phillippe, F., Canaud, B., Fortin, X., Garaude, F. & Jourdren, H. (2004). Effects of microstructure on shock propagation in foams. Laser Part. Beams 22, 171–174.Google Scholar
Ramis, R., Schmalz, R. & Meyer-Ter-Ven, J. (1988). MULTI—A computer code for one dimensional muti group radiation hydrodynamics. Comp. Phys. Comm. 49, 475–500.Google Scholar
Remington, B.A., Drake, R.P., Takabe, H. & Arnett, D. (2000). A review of astrophysics experiments on intense lasers. Phys. Plasmas 7, 1641–1652.Google Scholar
Rothman, S.D., Evans, A.M., Horsfield, C.J., Graham, P. & Thomas, B.R. (2002). Impedance mismatch equation of state using indirectly laser driven mutimagabar shocks. Phys. Plasmas 9, 1721–1733.Google Scholar
Shukla, M., Pant, H.C., Senecha, V.K., Khare, P., Veram, A.K., Rao, R.S., Gupta, N.K. & Godwal, B.K. (2003). Equation-of-state study of copper using laser induced shocks near 10 Mbar pressure and revalidation of theoretical modeling. Current Science 85, 802–808.Google Scholar
Shukla, M., Upadhyay, A., Senecha, V.K., Khare, P., Bandyopadhyay, S., Rai, V.N., Navathe, C.P., Pant, H.C., Khan, M. & Godwal, B.K. (2003). Equation of state studies using a 10 Hz Nd:YAG laser oscillator. Laser Part. Beams 21, 615–626.Google Scholar
Temporal, M., Lopez-Cela, JJ., Piriz, A.R., Grandjouan, N., Tahir, N.A. & Hoffmann, D.H.H. (2005). Compression of a cylindrical hydrogen sample driven by an intense co-axial heavy ion beam. Laser Part. Beams 23, 137–142.Google Scholar
Trainor, R.J., Shaner, J.W., Auerbach, J.M. & Holmes, N.C. (1979). Ultra high pressure laser driven shock wave experiments in aluminum. Phys. Rev. Lett. 42, 1154–1157.Google Scholar
Trusso, S., Barletta, E., Barreca, F., Fazio, E. & Neri, F. (2005). Time resolved imaging studies of the plasma produced by laser ablation of silicon in O2/Ar atmosphere. Laser Part. Beams 23, 149–153.Google Scholar
Zel'dovich, Ya. B. & Raizer, Yu. P. (1976). Physics of shock waves and high temperature hydrodynamic phenomena. New York: Academic Press.
Figure 0

Simulated pressure profiles in (CH2)n-Al.

Figure 1

Simulated pressure profiles in (CH2)n-Au.

Figure 2

Shock Luminosity signal in 8μm (CH2)n target.

Figure 3

Observed shock transit time, shock velocity, and shock pressure in two layered targets

Figure 4

(a) Hugoniot curves for (CH2)n-Al target. (b) Hugoniot curves for (CH2)n-Al target.

Figure 5

(a) Hugoniot curves for (CH2)n-Au target. (b) Hugoniot curves for (CH2)n-Au target.