1. INTRODUCTION
Significant progress has been made to develop intense lasers for various applications (Borghesi et al., Reference Borghesi, Kar, Romagnani, Toncian, Antici, Audebert, Brambrink, Ceccherini, Cecchetti, Fuchs, Galimberti, Gizzi, Grismayer, Lyseikina, Jung, Macchi, Mora, Osterholtz, Schiavi and Willi2007; Bourdier et al., Reference Bourdier, Patin and Lefebvre2007; Kumar et al., Reference Kumar, Gupta and Sharma2006; Patin et al., Reference Patin, Lefebvre, Bourdier and D'Humieres2006; Sakai et al., Reference Sakai, Miyazaki, Kawata, Hasumi and Kikuchi2006; Sherlock et al., Reference Sherlock, Bell and Rozmus2006). One outstanding example is inertial fusion energy by lasers. Today, peta watt (PW) lasers are already in operation (Danson et al., Reference Danson, Brummitt, Clarke, Collier, Fell, Frackiewicz, Hawkes, Hernandez–Gomez, Holligan, Hutchinson, Kidd, Lester, Musgrave, Neely, Neville, Norreys, Pepler, Reason, Shaikh, Winstone, Wyatt and Wyborn2005), and installing exa watt (EW) lasers are under consideration in Europe. When lasers with a total power of more than 10 EW are developed, a power density of up to 1028 W/cm2 will be available. Such strong fields associated with such power density will accelerate ions and electrons. High-energy particles accelerated in intense laser fields have been demonstrated with present-day laser technology and there are schemes under discussion to improve the affect even further (Ledingham et al., Reference Ledingham, Mckenna and Singal2003; Lifshitz et al., Reference Lifschitz, Faure, Glinec, Malka and Mora2006; Flippo et al., Reference Flippo, Hegelich, Albright, Yin, Gautier, Letzring, Schollmeier, Schreiber, Schulze and Fernandez2007; Winterberg, Reference Winterberg2006). Moreover, the possibility to generate a very intense laser field will facilitate unique new applications (Li & Imaskai, Reference Li and Imasaki2005; Chyla, Reference Chyla2006).
In this paper, we discuss the effect of 10 EW laser beam focused onto a 10−7 cm2 area, which is close to the diffraction limit. The electric field in this case is E L (V/m) = 2.7 × 1016, which is strong enough to distort the coulomb barrier of nuclei around 100 to 1000 fm from the nucleus core center. This distorted field promotes a tunneling effect, which enhances fusion reaction rates even in low temperature plasma.
2. BASIC CONCEPT
At first, an intense laser with high dense target plasma is used. We can produce cluster channels with an injection. After this, we irradiate them with an appropriate first laser pulse before the main pulse, and produce plasma. The shape of the preformed plasma is more than a few tens of centimeters in length with millimeters in radius. For the fusion energy conversion, and to keep plasma confinement in a short duration between two laser pulses, mirror magnetic field may be applied to this plasma.
EW laser, main pulse, is focused, and is injected into the plasma as a Gaussian beam. EW laser propagates through it. During this, intense field of 10 EW laser is applied along the center of laser path. This field distorts the coulomb barrier in each cycle of laser peak, which promotes the tunneling. The tunneled nuclei form a cloud of probability de Broglie wave of nucleon during effective period of a laser.
The cloud expands with the group velocity, vg, of tunneled nucleon. This expands during the laser pulse in an oscillating field. When the cloud of tunneled nuclei meets each other, they immediately make a compound nucleus, and make fusion reaction. This is induced during the laser pulse.
Electric field with laser and without laser in nuclei for a simple case is roughly indicated in Figure 1. At the center of nuclei, the nucleons are trapped in nuclear potential with de Broglie wave motion. This radius is about 5 fm and the nucleon hits the inner wall. In normal cases, penetrability has very small possibility, but EW laser makes it enlarger. In this case, the nucleon wave with exponential decay penetrates Coulomb barrier, and thereafter make a cloud of possibility outside the Coulomb barrier as shown in Figure 1.
Fig. 1. Normal Coulomb barrier and laser effect around nuclear core.
Basic assumptions in this model are noted as follows: (1) Plasma is formed in a magnet field with density up to 1021/cm3. Plasma density is uniform and charge is neutral. (2) Gaussian laser beam of 2 μm in diameter with annular shape for a long and tight focusing. (3) Nucleons are trapped in nuclear potential and hit the inner wall of coulomb burrier with nucleon kinetic energy of up to 100 eV. (4) After tunneling, nucleons diffuse away with de Broglie wave group velocity in the oscillating laser field. (5) After the laser pulse, reaction is stopped. This cloud may exist after the laser pulse, but such nuclei are diffused away so we limit the nuclear fusion reaction within laser pulse. (6) A compound nuclei is formed instantaneously when the cloud meets each other. (7) Wave length of Laser for this is 1.06 µm. Only the electric field is required. In this sense, the longer wave length is favorable, but the laser technology today for solid-state laser of 1 µm wavelength is well developed for laser fusion. So the solid-state laser is the first candidate for EW laser.
When intense laser of EW level is applied, the field of a dashed line is formed as shown in Figure 1. At the period of laser intensity peak, coulomb barrier is distorted. Such field can be calculated as follows. Here, A is the laser field of the applied laser, and can be written as,
Here, I indicate the power density of the intense laser. With a simple model, the potential induced by the laser field can be written as ϕL = −Er, where the field can be simply given as E = A sin(ωt). Then, we have the total laser potential as
The first term is the coulomb field and the second term is the laser field. Let us consider two extreme cases for the Coulomb barrier. At the laser peak, we can derive the following two equations from Eq. (2). One is
And at the other is
In Eq. (3), one can decrease the barrier. Let us focus on U 1. Providing Z 1 = Z 2 = 1, we can calculate, and figure out U 1 in Figure 2.
Fig. 2. (Color online) Calculated coulomb barrier with laser field of hydrogen nuclei.
We assume that the charge of nucleon is concentrated at the center to simplify the calculation, because the most important region for fusion reaction is the field between 100 fm to 1000 fm from the center. Under this assumption, Figure 2 indicates the calculated results of fields with and without laser. The field is distorted and makes effective tunneling and fusion reaction enhancement by the laser when laser intensity exceeds more than 1024 W/cm2.
T, the penetrability, is calculated as follow assuming a simple uniform rectangular potential case (Balantekin & Takigawa, Reference Balantekin and Takigawa1998). Then, the penetration rate for a simple potential barrier is expressed as
where
Here m = 938.28 MeV/c2 is for proton, and ħ = 6.58217 × 10−16 × 10−6 MeV × s. Finally, probability of the formation of cloud by tunneling can be written as F = ΦT. Here 
is a collision time of nucleon to inner wall of nuclear potential. Setting nuclear potential radius at 5 fm in the usual case, one can estimate 
to be 100 to 1000 with nucleon velocity corresponding to 10 eV to 200 eV (Fig. 3). In an actual case, E in Eq. (6) corresponds to this relation of energy level and kinetic energy of nuclei, and should be determined by experiments. But for this simple calculation, we used parameters to evaluate as shown in Figure 3.
Fig. 3. (Color online) Laser intensity and calculated penetration rate T for F.
The region of F is much smaller than one, this is valid. But when F is approaching to one, saturation with depletion of nuclei and so on will be provoked. In this region, the penetrability Fs can be roughly written as
For the calculation as shown in Figure 3, this effect is included.
For laser irradiation, nucleon energy cannot be fixed. This may be changed by the main pulse and pre-pulse of laser, so we give typical cases as shown in Figure 3.
In a normal case without laser, this reaction rate in cm3/s is as small as 10−20 for the Gamov peak of low temperature (Ichimaru, Reference Ichimaru1993).
There are clouds around the nuclei and they meet each other in some probability. Thereafter, they make compound nuclei immediately and fusion reaction is induced. The reaction rate R in this scheme is written as
In this relation, F 1 and F 2 are probabilities of laser tunneling nuclei for n1 and n2 · n1 and n2 are number density of reacting plasma of two species like deuterium and tritium, respectively. Here, we introduce r, the radius of the cloud diffusion area of tunneled nucleon wave, estimated from effective period of laser pulse of 10 fs. This is given by the group velocity of tunneled nucleon wave and is estimated to be 107 cm/s. So r is 10−7 cm in our case. v is a relative velocity of nuclei each other by the thermal motion or differential accelerated particle velocity by laser. Here we use a typical velocity of 2 × 108 cm/s. In an actual case, we can control the particle relative velocity much higher than usual thermal velocity when we use a pre-pulse or double pulse of laser.
Then, fusion energy ɛf from this simple model is written
Here, Q is the energy from one event of nuclear reaction, n1 and n2 are number density as is noted. V0 is a volume of region in length l with radius rL of laser focusing area, and τL is a time duration of laser pulse as 10 fm. 
is a burning rate of the fuel and can be written as ϕ = 1/(1 + R 
Ln) when R τLn is much smaller than 1. Here, we take n lower than cut-off density to propagate the laser beam for several tens cm of focused region. So the density of plasma we choose is slightly lower than cut-off density, as shown in Table 1.
Table 1. Typical characteristics and parameters for this scheme
Then, a net gain of the energy G from fusion reaction for commercial reactor is
ɛL is the laser energy in 
L and can be written as ɛL = π rL2I 
L · η c is efficiency of conversion from fusion energy to electric energy: ηL is efficiency of laser total system.
A typical fusion device in this schema is shown in Figure 4 (Imasaki & Li, Reference Imasaki and Li2007). Laser is one beam with annular shape of Gaussian mode to obtain a tight focusing. Outer radius of this beam is about 1 m. The energy from laser fusion is covered by graphite blanket and MHD electrode set at both ends of linear magnetic confinement machine. This magnetic field makes plasma to hold in a short period and make charged particles escape away from loss-cone for energy recover.
Fig. 4. Laser fusion devices with beam propagation in this schema.
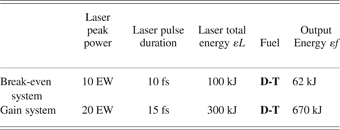
This schema is also applicable to use fuel as B or Li. These reactions are neutron free and they are applicable for commercial case for further future. The energy from the fusion reaction is recovered in direct electric converter. Parameters are summarized in Table 2.
Table 2. Total system output for break-even and gain system
3. CONCLUSION
In this article, a feasibility of new approach of laser fusion of break-even and gain systems is addressed. Deuterium and tritium reaction is assumed to used but Li or B related reactions are expected in a same way for actual commercial reactor, however higher power is required. These reactions are neutron free and can be expected a very clean energy source. In addition, long sustainability for their rich abundance is expected. Stimulated Raman and Brillion scattering are not considered because the laser pulse is much shorter than the plasma wave frequency.
There are many issues to be solved as a relation of Coulomb burrier shape of three dimensions, saturation mechanisms, nucleon kinetic energy and so on. These are summarized as follows: (1) Saturation region on F; (2) Theory for heavy nuclei of α-decay with penetration is used for light nuclei tunneling; (3) Cloud behavior in a laser pulse and after; (4) Compound nucleus formation processes; (5) Laser efficiency and repetition; (6) Relative velocity of each particle. These items are under investigation.
