I. Introduction
The U.S. brewing industry shows two paradoxical features in its macro, or mass-production, segment. First, industry concentration has risen steadily since the end of Prohibition The number of independent macro brewers reached a peak in 1935 at 766 firms and since then has continuously declined to about 20 firms today. This is reflected in the rise in the four-firm concentration ratio (CR4) and the Herfindahl-Hirschman index (HHI), two common measures of industry concentration.Footnote 1Figure 1 documents this increase for the period 1947–2009.Footnote 2 Second, in spite of rising concentration, profits have remained low, and previous studies have failed to detect the presence of market power.Footnote 3 A firm has market power when it can profitably maintain price above marginal cost.
Figure 1 Beer Industry Concentration (Four-Firm Concentration Ratio and Herfindahl-Hirschman Index), 1947–2009
This appears to be a paradox because many static models of oligopoly suggest that profits and market power will rise with a fall in the number of competitors, which is inconsistent with the trend in brewing. Nevertheless, not all models predict this outcome. For example, price equals marginal cost in the Bertrand model when products are homogeneous goods and there are two or more competitors. Furthermore, Tremblay and Tremblay (2011a) and Tremblay et al. (Reference Tremblay and Tremblay2011) demonstrate that price can equal marginal cost even in a monopoly setting when the incumbent firm competes in output and there exist one or more potential entrants that compete in price.
In the brewing industry, Tremblay and Tremblay (Reference Tremblay and Tremblay2005) speculate that firm profits remained low from the 1960s through the late 1990s because brewers were forced into a generalized war of attrition (Bulow & Klemperer, Reference Bulow and Klemperer1999). In such a war, N firms compete in a market that will profitably support N* firms in the long run. If N>N*, some firms must exit from the market for it to reach long-run equilibrium. As documented in Tremblay and Tremblay (Reference Tremblay and Tremblay2005), two events caused N* to rise in brewing. In the 1950s and 1960s, the advent of television gave a marketing advantage to large national producers, which were the only firms with enough resources to profitably advertise on television.Footnote 4 In addition, increased mechanization beginning in the 1970s reduced the cost of large-scale production. These changes gave larger beer producers a marketing and production advantage.
Table 1 shows how the market share of the national beer producersFootnote 5 grew over time and how changes in marketing and production economies affected optimal firm size. It lists estimates of the minimum efficient scale (MES) needed to take advantage of all scale economies in marketing and production for various years. MES-Output measures annual minimum efficient scale in millions of (31-gallon) barrels. MES-MS measures the market share needed to reach MES-Output. N* measures the number of firms needed to produce industry output if each firm produces at MES. This is called the efficient or cost-minimizing industry structure (Baumol et al., Reference Baumol, Panzar and Willig1982). As the table shows, over time MES grew and N* fell.
Table 1 The Market Share of the National Brewers, Minimum Efficient Scale (MES), the Number of Brewers (N), and the Cost-Minimizing Number of Competitors (N*) in the U.S. Brewing Industry
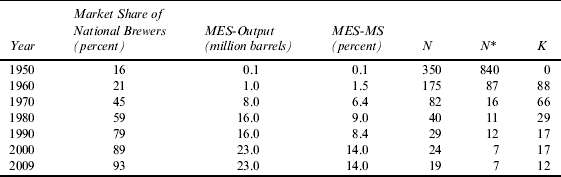
Notes: MES-Output measures minimum efficient scale measured in millions of (31-gallon) barrels. MES-MS represents the market share needed to reach minimum efficient scale. N is the number of macro brewers. N* represents the cost-minimizing industry structure (i.e., the number of firms that the industry can support if all firms produce at minimum efficient scale). MES-MS ≡ (Industry Output)/MES. N* ≡ 100/MES-MS; rounding errors explain the discrepancy in calculations. K = N – N* when (N – N*) >0 and 0 otherwise.
Sources: Steinberg (Reference Steinberg1980), the Statistical Abstract of the United States, Tremblay et al. (2005), and Tremblay and Tremblay (Reference Tremblay and Tremblay2005).
The intensity of the war is reflected in the number of firms that must exit the industry for the efficient structure to be reached in the long run. It is defined as K = N – N* when (N – N*) >0 and 0 otherwise. The value of K was largest in 1960s and 1970s, a period known as the “beer wars.” This is aptly described in Newsweek (September 4, 1978, 60):
After generations of stuffy, family-dominated management, when brewers competed against each other with camaraderie and forbearance, they are now frankly at war. Marketing and advertising, not the art of brewing, are the weapons. Brewers both large and small are racing to locate new consumers and invent new products to suit their taste. Two giants of the industry, Anheuser-Busch of St. Louis and Miller Brewing Company of Milwaukee, are the main contenders.
This description is remarkably accurate, as the facts show that the war was fought with advertising, the introduction of new brands, and tough price competition. Figure 2 plots the advertising intensity of the major brewers, measured as advertising spending per barrel. It shows that advertising spending was quite high from the mid-1950s through the late 1960s, a period in which television advertising became a prominent tool of the national brewers. In 1950, only 9 percent of households had a television set, a proportion that increased to 87 percent by 1960 and 95 percent by 1970.Footnote 6 Advertising spending rose once again in the 1980s, a period in which the Coors Brewing Company made large investments in advertising in order to expand into new regions of the country and thereby become a national brewer.Footnote 7
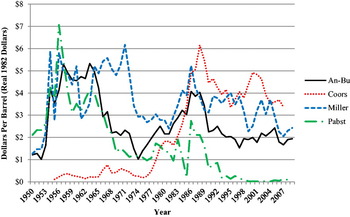
Figure 2 Advertising Per Barrel of Leading U.S. Brewers, 1950–2009
Brewers also fought for market share by introducing new brands. Table 2 lists the number of brands offered by the leading brewers. In 1950, most brewers offered a single flagship brand. The Anheuser-Busch Brewing Company is the lone exception, as it had continuously produced a flagship brand, Budweiser, and a super-premium brand, Michelob, since the end of Prohibition. Brand proliferation became apparent by the late 1970s, and by 1990 the major brewers each offered 9 or more different brands of beer.
Table 2 Major Domestic Beer Brands of the Anheuser-Busch, Coors, Miller, and Pabst Brewing Companies
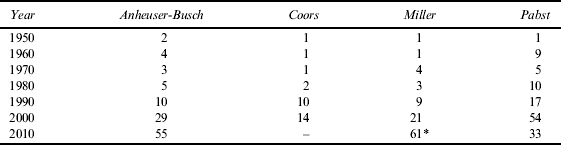
* This reflects the brands for both Miller and Coors, as the companies formed a joint venture in 2008 to form MillerCoors.
Sources: Tremblay and Tremblay (Reference Tremblay and Tremblay2005) for 1950–2000 and company web pages for 2010.
Iwasaki et al. (Reference Iwasaki, Seldon and Tremblay2008) formally tested for the effect of the war of attrition on concentration and price competition. They found that advertising and rising MES contributed to increases in industry concentration. In spite of rising concentration, they found that the war reduced price-cost margins during the 1960s through the early 1990s. Unfortunately, their work does not shed light on the extent to which market power has changed since the late 1990s.
One might have expected the intensity of the war to diminish by the 2000s for several reasons. First, little room is left for consolidation. In 2002, Miller was purchased by South African Breweries to form SABMiller. In 2008, Anheuser–Busch was purchased by Belgium's InBev to form Anheuser–Busch InBev, and Coors and SABMiller established a joint venture called MillerCoors. Second, Pabst gave up the production of beer in 2001, contracting with Miller to produce all its beer. Finally, the remaining macro brewers have retreated to niche markets, competing more with the micro than the macro brewers.
The purpose of this paper is to determine whether the degree of competition has fallen in the final stages of industry consolidation. Two methods are used. The first is the new empirical industrial organization technique, which uses regression analysis to estimate the markup of price over marginal cost. The second is a new technique developed by Boone (Reference Boone2008), which compares the variable profits of efficient firms with less efficient firms over different regimes of competition. The main advantage of Boone's technique is that it avoids measurement problems associated with accounting data. The evidence shows that competition has decreased from the late 1990s to 2008 but not enough to substantially increase market power.
II. Estimation of the Degree of Competition
In this section, we review the two methods that are used to estimate the degree of competition in brewing. The first is called the new empirical industrial organization technique.Footnote 8 The empirical model derives from a general first-order condition of profit maximization. To illustrate, assume a market with N firms, where firm i's inverse demand is p i(q 1, q 2, q 3, … , q N), p i is firm i's price, and q i is firm i's output. The firm's long-run total cost function is C(q i, w), where w is a vector in input prices; marginal cost is MC = ∂C/∂q i. Solving the firm's first-order condition for price produces an equation called an optimal price equation (supply relation or markup equation):
$$p_i = \displaystyle{{\partial \rm c} \over {\partial {q_i}}} - \theta \displaystyle{{\partial {\,p_i}} \over {\partial {q_i}}} q_i, $$where θ is a behavioral parameter of market power. We will see subsequently that choosing different values of θ will produce different oligopoly equilibria.
This specification is related to the Lerner (Reference Lerner1934) index of market power (
${\cal L}$). To illustrate, assume that firms produce homogeneous goods, such that p i=p and ∂p i/∂q i = ∂p/∂Q, where Q is industry output. Under these conditions, Equation (1) can be rearranged as
$${\cal L} = \displaystyle{{\,p - MC} \over p} = - \theta \displaystyle{{\partial p} \over {\partial Q}} \displaystyle{Q \over p}\displaystyle{{q_i} \over Q} = \displaystyle{{ms_i \theta} \over \eta} = \displaystyle{\theta \over {N{\cdot}\eta}} $$where ms i is the market share of firm i, which equals 1/N when the market is in equilibrium because of symmetry. When price equals marginal cost, market power is nonexistent and ${\cal L}$ = 0; ${\cal L}$ increases with market power. This specification describes a variety of possible cooperative and noncooperative equilibria.
• In a competitive or Bertrand equilibrium with homogeneous goods, p = MC, which implies that θ = 0 and
${\cal L}$ = 0.• For a monopolist, θ=N = 1 and
${\cal L}$ = 1/η.• In the Cournot equilibrium, θ = 1 and
${\cal L}$=ms i/η = 1/(N·η). Notice that when N = 1, ${\cal L}$ = 1/η, which is the simple monopoly outcome.• In a perfect cartel, θ=N and
${\cal L}$ = 1/η.
If the market outcome ranges from competitive to cartel, then 0 ⩽ θ ⩽ N and 0 ⩽ ${\cal L}$ ⩽1/η. One can think of θ as an indicator of the “toughness of competition,” as described by Sutton (Reference Sutton1991).
In its empirical form, Equation (1) is transformed into the following equation.
$$p =\, \lt\! MC\! \gt + \lambda q_i, $$where <MC> is an empirical specification of the marginal cost function and λ=θ(∂p/∂Q) is a market power parameter to be estimated. With appropriate data, Equation (3) is either estimated with firm demand as a system of equations or as a single equation using an instrumental variables technique given that firm output is an endogenous variable. The Lerner index is calculated from parameter estimates and mean values of the data.
The second method that we use to estimate the degree of competition in brewing was developed by Boone (Reference Boone2008). The main advantages of his method are that it requires relatively little data and it avoids the use of accounting cost and profit data, which are poor proxies for their economic counterparts.Footnote 9 In order to use Boone's method, firms must not be equally efficient. This is a reasonable assumption in brewing, in which some firms have rather antiquated equipment, are unable to advertise nationally, and may not be scale efficient. With dissimilar levels of efficiency, Boone shows that an increase in competition punishes inefficient firms more harshly than efficient firms. In other words, increasingly tougher competition causes the least efficient firms to exit first.
To test for a change in industry competitiveness, one must derive what Boone calls an index of relative profit differences (RPD). RPD compares the variable profits of different firms within an industry. Let π iv(Ei, θ) equal firm i's variable profit, which is a function of its efficiency level (Ei) and the behavioral parameter (θ). Variable profit equals total revenue minus total variable cost. To illustrate this idea, consider a market with three firms, where firm 1 is most efficient and firm 3 is least efficient (E1 > E2 > E3). Recall that θ ranges from 0 (competitive) to N (cartel), where the degree of competition increases as θ falls. With this notation,
$$RPD \equiv \displaystyle{{\pi _1^v - \pi _3^v} \over {\pi _2^v - \pi _3^v}}. $$Under the conditions of the model, an increase in competition will lead to an increase in RPD, ∂RPD/∂θ <0. In other words, an increase in competition harms the least efficient firms the most, such that (π 1v−π 3v) increases relative to (π 2v−π 3v). Thus, if RPD rises (falls) over time, we can conclude that competition has increased (decreased) and market power has fallen (risen).
Boone's index has several desirable qualities. First, by using variable profits, it circumvents the measurement problems associated with accounting profits.Footnote 10 Second, data are needed for no more than three firms in the industry. The only difficulty is that firms must be ranked in terms of their relative efficiency. One approach is to use data envelopment analysis to characterize a firm's technology and relative inefficiency, as suggested in Färe et al. (Reference Färe, Grosskopf and Lovell1985, Reference Färe, Grosskopf and Lovell2008). Boone suggests a simple alternative in which the firm with lowest average variable costs is most efficient.
In brewing, previous studies can be used to rank the relative efficiency of firms. In terms of scale efficiency, Tremblay and Tremblay (Reference Tremblay and Tremblay2005) found that only the industry leader and national brewer, Anheuser-Busch, has been consistently scale efficient. Miller, another national brewer, has been scale efficient since the late 1970s. The third largest brewer, Coors, was marginally scale efficient by the early 1990s when it became a small national brewer. None of the smaller regional brewers were scale efficient. In terms of marketing efficiency, the advent of television gave the large national brewers an advantage. This is confirmed by Färe et al. (Reference Färe, Grosskopf, Seldon and Tremblay2004), who found that Anheuser-Busch was the most efficient, while the smallest regional brewers and failing firms were the least efficient. Taken as a whole, this implies that the rank order from most to least efficient firms is: Anheuser-Busch, Miller, Coors, and local brewers.
III. Data and Empirical Results
The data set used in our regression analysis consists of annual observations from 1977 to 2008 for eleven U.S. brewing companies. These include all macro brewers that were publicly owned: Anheuser-Busch, Coors, Falstaff, Genesee, Heileman, Miller, Olympia, Pabst, Pittsburg, Schlitz, and Stroh. Firm variables include price, marginal cost, output, total revenue, and variable profit (total profit minus total variable cost). All firm data derive from the annual trade publication Beer Industry Update.
The industry data used in the study include the measures of industry concentration (HHI and CR4) and a measure of the intensity of the beer wars (WAR). The concentration indices are updated from Tremblay and Tremblay (Reference Tremblay and Tremblay2005). WAR is defined as N*/N. With this definition, the intensity of the war of attrition increases as WAR decreases.Footnote 11 The number for firms (N) is updated from Tremblay and Tremblay (Reference Tremblay and Tremblay2005). The efficient number of firms (N*) equals Q/MES, where industry production (Q) is obtained from Beer Industry Update. An estimate of minimum efficient scale (MES) is derived from Tremblay and Tremblay (Reference Tremblay and Tremblay2005).
Given that output is an exogenous variable, we also use two market demand variables that serve as instruments in the optimal price equation. These are per-capita disposable income (in 1982 dollars) and a demographics variable, the proportion of the population that ranges in age from 18 to 44.Footnote 12 Demand studies show that this is the primary beer-drinking age group (see Tremblay and Tremblay, Reference Tremblay and Tremblay2005). Table 3 displays the descriptive statistics of the firm, industry, and demand variables.
Table 3 Descriptive Statistics of Firm and Industry Data, U.S. Brewing Industry, 1977–2008
Summary statistics are for the minimum (Min), mean, maximum (Max), and standard deviation (Std. Dev.).
We first investigate the relative profit differences (RPD). The data allow us to investigate RPD for only two trios of macro producers: for Anheuser-Busch, Miller, and Genesee (A-M-G) and for Anheuser-Busch, Coors, and Genesee (A-C-G).Footnote 13 Unfortunately, this provides estimates only from 1978 to 1999. To obtain estimates through 2006, we also include a hybrid brewer, the Boston Beer Company.Footnote 14 Because of its relatively small size, we rank Boston as less efficient than Anheuser-Busch, and because of its rapid growth rate, we rank Boston as more efficient that Miller and Coors.Footnote 15 This provides two additional trios of firms to be used to calculate RPD: Anheuser-Busch, Boston, and Miller (A-BB-M) and Anheuser-Busch, Boston, and Coors (A-BB-C). Recall that an increase in RPD implies an increase in competition. Mean estimates of RPD for four sets of firms are plotted in Figure 3, where the values were normalized to equal 100 in 1991 (the first year that Boston data are available). Consistent with the findings of Iwasaki et al. (Reference Iwasaki, Seldon and Tremblay2008), the results show that the beer industry became more competitive during the beer wars that lasted through the mid-1980s, and the degree of competition remained relatively constant during the 1990s. Although RPD fluctuated in the early 2000s, its value reached historically high levels by the mid-2000s. This suggests that competition did not diminish substantially from the late 1990s through 2006.
Figure 3 Mean Relative Profit Difference (RPD) Note: This plots the mean RPD for the following triads of firms: Anheuser Busch, Miller, and Genesee; Anheuser Busch, Coors, and Genesee; Anheuser Busch, Boston Beer, and Miller; Anheuser Busch, Boston Beer, and Coors
Next, we use regression analysis to estimate the optimal price equation (Equation 1). Data limitations require that we use average cost as a proxy for marginal cost. This is a reasonable assumption for the national producers because they are large and able to reach MES. To control for cost and other possible differences between national and regional brewers, we include a dummy variable, DN, which equals 1 for national producers and 0 otherwise.
Given our uncertainty concerning whether market power remained constant over our sample period, we consider several specifications. As a starting point, we consider the simple model where market power is constant. This model is given by
$$p_i = MC_i + \beta _0 D_N + \lambda q_i, $$where β 0 and λ are parameters to be estimated. Notice that the parameter on MCi equals 1. In this specification, firms have market power when λ >0.
This model is unlikely to be valid in brewing, however, given previous evidence that there has been a war of attrition in brewing. One hypothesis is that market power changes over time and is a function of WAR: λ=β 1+β 2WAR. In this case, the model becomes
$$p_i = MC_i + \beta _0 D_N + \beta _1 q_i + \beta _2 WAR \cdot q_i. $$As we have defined WAR, a reduction in the intensity of the war implies that ∂pi/∂WAR=β 2q i >0. That is, market power increases with the WAR variable.
Sutton (Reference Sutton1991) and Tremblay and Tremblay (Reference Tremblay and Tremblay2005) argue that three periods or regimes in brewing relate to market power. In the first period, 1977–1986, the war was so intense that market power was zero.Footnote 16 Market power then rose progressively into the second period (1987–1996) and the third period (1997–2008). If this is true, the following model is appropriate.
$$p_i = MC_i + \beta _0 D_N + \beta _3 q_{87 - 96} + \beta _4 q_{97 - 08}. $$In this specification, q 87 − 96 ≡ D87 − 96 · q i, q 97 − 08 ≡ D97 − 08 · q i, D87 − 96, = 1 from 1987 through 1996 (0 otherwise), and D97 − 08 = 1 from 1997 through 2008 (0 otherwise). If market power rose from one period to another, then β 4 > β 3 > 0.
In the final specification, we modify Equation (7) to control for the effect of the war on market power during these later regimes. In this case,
$$\eqalign{\,p_i = & MC_i + \beta _0 D_N + \beta _3 q_{87 - 96} + \beta _4 q_{97 - 08} \cr & \plus \beta _5 WAR \cdot q_{87 - 96} + \beta _6 WAR \cdot q_{97 - 08}.} $$This model allows us to determine how market power changes over time and is affected by the WAR variable. If market power rises over time, then β 4>β 3 > 0 and β 6>β 5 > 0.
Each specification is estimated, with and without DN, using an instrumental variables estimation technique. As discussed above, the instruments are per-capita disposable income and the proportion of the population age 18 to 44. Given our use of pooled data, we use a clustering method that allows the standard error of the regression to vary by clusters (i.e., firms). Following Cameron, Gelbach, & Miller (Reference Cameron, Gelbach and Miller2008), we obtained standard errors using bootstrapping with repeated resampling and replacement within each cluster for 1,000 trials. The specifications that were estimated are labeled Models 1 through 8 (M1–M8) in Table 4. In each model, the Wald χ 2 statistic is sufficiently high, implying that the parameters of the model are jointly significant. The MC parameter is close to 1, and in most specifications, the national dummy variable is positive and significant, which is consistent with the fact that most national brands sell for higher prices than do regional brands.
Table 4 Parameter Estimates and Standard Errors of the Optimal Price Equation
Standard errors are in parentheses. The sample size is 174.
a Significant at 1 percent.
b Significant at 5 percent.
c Significant at 10 percent.
Regarding the issue of market power, we are particularly interested in two hypotheses. The first is the hypothesis that a decrease in WAR (i.e., an increase in the intensity of the war) reduces market power. This hypothesis is confirmed in models M3 and M4, as the parameter on the interaction variable between output and WAR is positive and significant.
Second, we are interested in determining whether market power increased progressively from 1987–1996 to 1996–2008. In the absence of the WAR variable, Models M5 and M6 are consistent with this hypothesis. In both models, the parameter estimate on q 97 − 08 is greater than the parameter estimate on q 87 − 96, although the difference between parameters is insignificant. We obtain a similar result when we include the WAR variable in Models M7 and M8. The parameter estimates on q 97 − 08 exceeds that of q 87 − 96, and parameter estimates on q 97 − 08·War exceed that of q 87 − 96·War. Furthermore, we fail to reject the joint hypothesis that the parameters differ between q 97 − 08 and q 87 − 96 and differ between q 97 − 08·War and q 87 − 96·War (at the 99 percent significance level for each model).
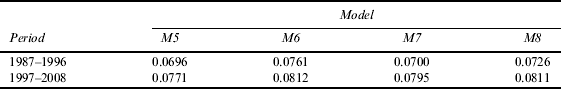
To further investigate how market power has changed over time, we estimate the Lerner index for the periods 1987–1996 and 1997–2008 from Models M5 through M8 (see Table 5). Consistent with Tremblay and Tremblay (Reference Tremblay and Tremblay2005), the results show that the Lerner index is relatively low. The results also show that there has been a small increase in the Lerner index from the 1987–1996 period to the 1997–2008 period. The increase is never significantly different from zero, however, with p-values equaling 46 percent for M5, 64 percent for M6, 36 percent for M7, and 39 percent for M8. In total, the results suggest that even though the war of attrition came to a close, there is no evidence of a substantial or significant increase in market power in the U.S. brewing industry.
Table 5 Lerner Index Estimates
IV. Concluding Remarks
Industry concentration has risen dramatically in the post–World War II era in the macro segment of the U.S. brewing industry. Previous studies show that profits and market power remained low during the 1970s and 1980s because firms were forced to compete in a war of attrition. Today, macro beer production is dominated by just two companies, Anheuser-Busch and Miller-Coors. This raises concerns that their market power may rise. The purpose of this paper is to estimate market power and determine whether it rose from the late 1990s through 2008.
Two methods are used to estimate the degree of competition in brewing. The first is the traditional new empirical industrial organization technique, which we modify to allow market power to vary over time. The second is a technique developed by Boone (Reference Boone2008), which uses data on variable profits to determine whether competition decreased over time. The results confirm that the war was intense through the 1990s. Regression results using the new empirical industrial organization approach indicate that although market power rose somewhat in the 1997–2008 period, it still remains low. This suggests that the degree of competition in brewing remains high even though there are only a few remaining major beer producers.
