INTRODUCTION
People rely heavily on arithmetic to process numbers and solve simple calculation problems in daily life. Many studies have investigated how the human brain processes simple arithmetic (Butterworth, Zorzi, Gierlli, & Jonckheere, Reference Butterworth, Zorzi, Girelli and Jonckheere2001; Campbell & Epp, Reference Campbell and Epp2004; Campbell & Metcalfe, Reference Campbell and Metcalfe2008; Dehaene & Cohen, Reference Dehaene and Cohen1995, Reference Dehaene and Cohen1997). For example, Campbell and Epp (Reference Campbell and Epp2004) suggested that solving a simple arithmetic problem (e.g., 8+5) involves three stages: encoding the arithmetic problem, retrieving or calculating the answer, and reporting the answer. Therefore, there are at least two ways to solve arithmetic problems: the retrieval of arithmetic facts and calculating quantity. Therein, arithmetic facts knowledge, such as memory retrieval of basic table facts (e.g., 2+4=6; 3×4=12), is essential (Megías & Macizo, Reference Megías and Macizo2015). The modality or format of arithmetic presentations could affect the encoding of problem and reporting of answers during arithmetic (Dehaene & Cohen, Reference Dehaene and Cohen1995; McCloskey, Reference McCloskey1992). However, whether retrieval of arithmetic facts or substantial computation depends on the modality of external input is controversial.
According to the modality-dependent processing viewpoint, arithmetic fact retrieval is dependent on the modality of presentation (e.g., visual or auditory). From this viewpoint, multiple-code models (e.g., encoding-complex model) indicate that the modality of presentation is encoded to create multimodal concepts with a specific format (Campbell & Clark, Reference Campbell and Clark1988; Campbell, Reference Campbell1994). Supportive evidence showed that patients with left ventral temporo-occipital infarctions are impaired in addition calculations, but only when the operands are presented visually, not when they are presented auditorily (Cohen & Dehaene, Reference Cohen and Dehaene1995; McNeil & Warrington, Reference McNeil and Warrington1994). The pure alexic patient could not verbally produce the correct answers in multiplication tasks, demonstrating that multiplication processing was affected by verbally reading (Cohen & Dehaene, Reference Cohen and Dehaene2000).
According to the modality-independent processing viewpoint, surface modality does not affect mental arithmetic fact retrieval. Specifically, the abstract code model proposes that arithmetic facts are addressed and retrieved by the comprehension system into abstract codes, which are then used for the retrieval of arithmetic facts or for the calculation of an answer (McCloskey, Reference McCloskey1992; McCloskey & Macaruso, Reference McCloskey and Macaruso1995). For the application, arithmetic operations are represented in symbolic formats abstracted from their surface form (e.g., visual or auditory). Consistent with this view, brain-injured patients show specific deficits regardless of presentation format. For both written and oral presentation and response modes, patient M.W.’s performance pattern consistently showed difficulty in retrieving multiplication facts (McCloskey, Carmazza, & Basili, Reference McCloskey, Caramazza and Basili1985).
The inconsistent results concerning arithmetic (e.g., addition and multiplication) may be due to different arithmetic learning experiences and strategies used. That is, schoolchildren are usually taught to use procedural strategies for simple addition and subtraction; however, they are taught to use verbal memory strategy to memorize multiplication facts (Dehaene & Cohen, Reference Dehaene and Cohen1997; Roussel, Fayol, & Baarrouillet, Reference Roussel, Fayol and Barrouillet2002; Zhou & Dong, Reference Zhou and Dong2003). These differential strategies during the acquisition of arithmetic facts may play an important role in shaping their mental representations (Siegler & Shipley, Reference Siegler and Shipley1995).
Interestingly, Chinese individuals typically use special learning strategies for mental arithmetic that are different from Western countries. Previous studies showed that a substantial number of American and Canadian adults (up to 29%) solve single-digit addition and multiplication problems via procedural strategies (Campbell & Xue, Reference Campbell and Xue2001; Lefevre & Liu, Reference Lefevre and Liu1997). In China, the differential use of these two strategies for different arithmetic operations is especially clear. For addition and subtraction, varieties of procedures (e.g., transforming 4+7=3+7+1=11) are used; however, for multiplication, Chinese children are instructed to exclusively use the rote verbal strategy (Zhou & Dong, Reference Zhou and Dong2003).
Generally, Chinese children start to memorize the multiplication table by rote verbal strategy during the second semester of the first grade or the first semester of the second grade. Since then, they use the strategy of multiplication table recitation to resolve multiplication problem (Zhou, Chen, Zang, et al., Reference Zhou, Chen, Zang, Dong, Chen, Qiao and Gong2007). The learning experience could shape memory of arithmetic facts. Previous studies have shown that the multiplication is typically represented with verbal code, but the procedure-based arithmetic is typically represented with visuospatial code (e.g., Dehaene & Cohen, Reference Dehaene and Cohen1995; Zhou, Chen, Zang, et al., Reference Zhou, Chen, Zang, Dong, Chen, Qiao and Gong2007). The auditory presenting of arithmetic problems might have more advantage to the multiplication problems due to the verbal memory. Therefore, Chinese participants’ acquisition strategy preferences provide a well-testable model to examine the effect of perceptual modality on different arithmetic operations.
We investigated whether mental arithmetic retrieval depends on surface modality and whether there is a dissociation between addition and multiplication in a stimulus-dependent manner. Although a previous study demonstrated the influence of surface form on addition calculation depending on the type of the input stimulation (i.e., Arabic digit, number word, and dot notation) (Sciama, Semenza, & Butterworth, Reference Sciama, Semenza and Butterworth1999), how the surface modality or knowledge format (i.e., visual and auditory presentation) affects mental arithmetic is still unclear.
This study reports examined one patient (Y.Q.) with 11 years of formal education in China. When he was 45 years old, he suffered a cerebral infarction in the left frontotemporal lobe and basal ganglia. Before the assessments of cognitive abilities for the current investigation, clinical neuropsychological examination showed that the patient had prevalent loss of arithmetic [zero score in Mini Mental State Examination (MMSE)]. His family reported that he could understand other persons’ spoken language. To comprehensively examine the patient’s cognitive ability, we investigated general cognitive, language, and numerical processing. Previous studies suggested that mental addition and multiplication depended on differential learning and practice experiences.
For example, multiplication facts typically are based on verbal-motor areas, including supplementary motor area, precentral gyrus (i.e., primary motor area) in Chinese adults (Zhou, Chen, Zang, et al., Reference Zhou, Chen, Zang, Dong, Chen, Qiao and Gong2007). Meanwhile, the verbal memory of multiplication facts is highly modularized. For example, Mainland Chinese participants memorized only the smaller-operand-first multiplication problems (Zhou, Chen, Zhang, et al., Reference Zhou, Chen, Zhang, Chen, Zhou and Dong2007). So, we used a typical auditory input for multiplication, which is maybe more targeted to the verbal memory of multiplication. We expected that multiplication processing would be more affected by the auditory form.
METHODS
Ethical Consideration
The ethics board of Beijing Normal University approved the study protocol and all participants provided written informed consent.
PARTICIPANTS
Patient
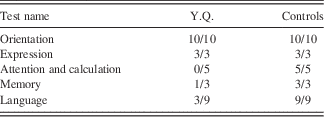
Y.Q. was a 45-year-old right-handed man with 11 years of education, who previously worked in the transportation department of the Chinese Railways. In January 2012, his right limbs showed movement disability without obvious cause, and he talked in a confused and irrational manner. When he walked, his right leg sloped to the right. The next morning, he displayed hyperspasmia once and could not recognize his family. Magnetic resonance imaging (MRI) examination of the brain indicated that he had an arteriosclerotic cerebral infarction in the left frontotemporal lobe and basal ganglia, but no lesions in the parietal lobes (Figure 1). The patient exhibited incoherent consciousness disorder lasting for 1 month. Then, he regained coherent consciousness; however, he could not distinguish between his own right and left finger. In daily life, the patient could not go shopping independently and complained about calculation difficulties. However, he could understand other persons’ spoken language. In addition, he had a 3-year medical history of hypertension, hyperlipemia, and fatty liver hepatitis. According to the MMSE, the patient was impaired in attention, calculation, memory, and language (all ps<.05); however, he showed normal scores for orientation and expression on the MMSE (Folstein, Folstein, & McHugh, Reference Folstein, Folstein and Mchugh1975) (Table 1).

Fig. 1 T2-weighted MRI images for patient Y.Q. The right side of each image refers to the left hemisphere. The patient had severe lesions in the left frontotemporal lobe and basal ganglia due to cerebral infarction. No obvious lesions were found in the parietal lobes.
Table 1 Mini-Mental State Examination in patient Y.Q. and controls

Note. When the number of years of education >6, a cutoff of 24 was chosen to indicate normal cognitive function.
Controls
Control participants were six healthy volunteers (three men, three women) who were matched with the patient in age and education years. Exclusion criteria included a history of neurological disorder, head injury, or alcohol abuse. Their mean age was 46.3 years (SD=1.6), and they had an average of 10.7 years (range from 10 to 11 years; SD=0.52) of education. There were no significant differences between controls and the patient in age and education years (ps>.48). The ethics board of Beijing Normal University approved the study protocol and each of the participants provided written informed consent.
Assessments of Cognitive Abilities
General cognitive tests, language processing tests, and numerical processing tests were used to assess cognitive abilities. To examine the influence of presentation modality of arithmetic problem on mental arithmetic, the experiments of addition and multiplication were designed. All the tasks were programmed using Web-based applications available at www.dweipsy.com/lattice (Wei et al., Reference Wei, Lu, Zhao, Chen, Dong and Zhou2012). The first completed responses were scored in each test when the participants offered several responses (see the Supplementary Material for details of cognitive tests).
RESULTS
For all neuropsychological tests, the participants’ first complete responses were scored, and the correct scores were analyzed. To examine the potential differences in performance between patient Y.Q. and controls across all tests, we used a χ2 test, a modified t test, and a program developed by Crawford, Garthwaite, and Porter (Reference Crawford, Garthwaite and Porter2010), which refers to the patient’s scores relative to a control sample. The modified t test also provides a point estimate of the effect size for the difference between the case and controls (z-cc) with an accompanying 95% confidence interval. For smaller-operand-first and larger-operand-first problems, a χ2 test was used to examine the patient’s performance of addition and multiplication in the visual and auditory condition.
General Cognitive Processing
The scores of general cognitive processing for the patient and controls are displayed in Table 2. To compare the scores, t tests were used. For Raven’s Progressive Matrices, mental rotation, forward digit span, backward digit span, and choice reaction time, the differences between controls and patient Y.Q. were not significant (all ps>.1).
Table 2 Scores on the general cognitive tasks in patient Y.Q. and controls

Note. The numerator refers to the number of correct trials; the denominator refers to the total number of trials for each task.
Language Processing
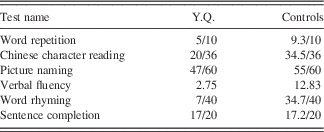
The language processing scores for the patient and controls are displayed in Table 3. To compare the scores, t tests were used. For word repetition, the patient showed lower scores than did the controls, t(5)=3.87, p<.05, z-cc=−4.18 (−6.80 to −1.55). Patient Y.Q. showed significantly lower performance than did controls in Chinese character reading, t(5)=11.04, p<.01, z-cc=−11.89 (−19.08 to −4.79); picture naming, t(5)=2.65, p<.05, z-cc=−2.90 (−4.80 to −0.97); verbal fluency, t(5)=4.96, p<.01, z-cc=−5.36 (−8.67 to −2.07); and word rhyming, t(5)=6.01, p<.01, z-cc=−6.49 (−10.46 to −2.55). There were no significant differences between patient Y.Q. and controls in sentence completion (ps>.05).
Table 3 Scores on the language processing tasks
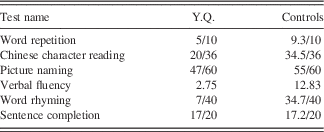
Note. The numerator refers to the number of correct trials; the denominator refers to the total number of trials for each task.
Numerical Processing
The scores of numerical processing for the patient and controls are displayed in Table 4. Because the control group achieved full scores in numerical processing except for the numerical magnitude comparison task, the t test precondition was not met. Therefore, χ2 tests were used to compare the scores. For dot counting, abstract counting, 1-digit and 2-digit Arabic numeral reading, 1-digit Chinese number word reading, 1-digit and 2-digit Arabic number dictating, numerical magnitude comparison, comparison of two dot arrays, and number series completion, there were no significant differences between patient Y.Q. and controls (all ps>.05). The patient had lower scores than did the controls for backward verbal number sequence, χ2(1)=5.55, p<.05; 3-digit Arabic numeral reading, χ2(1)=16.64, p<.001; 4-digit Arabic numeral reading, χ2(1)=16.64, p<.001; 2-digit Chinese number word reading, χ2(1)=5.17, p<.05; simple division, χ2(1)=26.59, p<.001; simple addition, t(5)=11.65, p<.001, z-cc=−12.58 (−20.19 to −5.08); simple subtraction, t(5)=5.91, p<.01, z-cc=−6.38 (−10.29 to −2.50); and simple multiplication, t(5)=12.41, p<.001, z-cc=−13.40 (−21.51 to −5.42).
Table 4 Scores on the numerical processing tasks

Note. The numerator refers to the number of correct trials; the denominator refers to the total number of trials for each task.
Addition and Multiplication in Visual and Auditory Condition
The patient’s performance in addition and multiplication of the visual and auditory condition is shown in Figure 2 and Appendices 1 and 2. For smaller-operand-first and tie addition and larger-operand-first and tie addition, there were no significant differences between visual and auditory conditions, χ2(1)=0.05, p=.82; χ2(1)=0.55, p=.46, respectively. For smaller-operand-first and tie multiplication and larger-operand-first and tie multiplication, patient Y.Q. showed higher scores in the auditory than visual condition, χ2(1)=28.28, p<.001; χ2(1)=35.50, p<.001, respectively.

Fig. 2 The patient’s percentage correct responses for single-digit multiplication and addition problems. The problems included smaller-operand-first (e.g., 3+7, 3×7), larger-operand-first (e.g., 7+3, 7×3), and tie problems.
DISCUSSION
The present study aimed to investigate the influence of presentation modality of arithmetic problem on mental arithmetic for a patient with infarction of the left anterior hemisphere (i.e., left frontotemporal lobe and basal ganglia). The results showed that the patient was impaired in the addition task, regardless of visual or auditory presentation; however, he showed preserved multiplication in the auditory condition. This suggests that mental multiplication could be characterized as modality-dependent processing, which was accessed through auditory input.
Compared to controls, Y.Q. had generally impaired abilities in the numerical and arithmetic tasks. Although parietal lobes contribute to simple calculation (multiplication or addition) (Della Puppa et al., Reference Della Puppa, De Pellegrin, d’Avella, Gioffrè, Munari, Saladini and Semenza2013; Semenza, Salillas, De Pallegrin, & Della Puppa, Reference Semenza, Salillas, De Pallegrin and Della Puppa2016), the frontal cortex is also closely related to the demands imposed by arithmetic fact retrieval (Jost, Khader, Burke, Bien, & Rösler, Reference Jost, Khader, Burke, Bien and Rösler2011). Neuropsychological studies have suggested that anterior parts of the left hemisphere including the frontal cortex (Fasotti, Elilng, & Bremer, Reference Fasotti, Eling and Bremer1992; Lucchelli & De Renzi, Reference Lucchelli and De Renzi1993; Tohgi et al., Reference Tohgi, Saitoh, Takahashi, Takahashi, Utsugisawa, Yonezawa and Sasaki1995) and basal ganglia (Dehaene & Cohen, Reference Dehaene and Cohen1997) play an important role in mathematical and language processing.
First, patients with frontal lobe lesion show deficits in numerical processing (Cappelletti, Butterworth, & Kopelman, Reference Cappelletti, Butterworth and Kopelman2012; Fasotti et al., Reference Fasotti, Eling and Bremer1992; Lucchelli & De Renzi, Reference Lucchelli and De Renzi1993). For example, five frontal lesion patients showed impaired performance in number reading, single-digit arithmetical operations (i.e., addition, subtraction, multiplication, and division problems), multi-digit calculation, and reading (Cappelletti et al., Reference Cappelletti, Butterworth and Kopelman2012). Furthermore, some researchers have also suggested that the frontal lobes are involved in two components of the calculation system: retrieval of basic arithmetical facts and execution of calculation procedures (Sokol, McCloskey, Cohen, and Aliminosa, Reference Sokol, McCloskey, Cohen and Aliminosa1991).
Second, deficits in arithmetic have been reported after left subcortical lesions that affect the basal ganglia (Benke, Delazer, Bartha, & Auer, Reference Benke, Delazer, Bartha and Auer2003; Dehaene & Cohen, Reference Dehaene and Cohen1997; Delazer et al., Reference Delazer, Domahs, Lochy, Karner, Benke and Poewe2004; Hittmair-Delazer, Semenza, & Denes, Reference Hittmair-Delazer, Semenza and Denes1994). Two patients affected by basal ganglia lesions showed impairment in a timed mental arithmetic task and writing complex problems (Benke et al., Reference Benke, Delazer, Bartha and Auer2003). It has also been suggested that cortico-subcortical loops involving the basal ganglia are part of a functional circuit mediating language-based retrieval of simple addition and multiplication facts (Dehaene & Cohen, Reference Dehaene and Cohen1997).
Building on the extensive evidence on the role of the left anterior hemisphere in most numerical and language abilities, the relatively preserved auditory multiplication ability in Y.Q. was rather interesting. We infer that the left frontotemporal lobe and basal ganglia lesions impaired the ability to execute the calculation procedure that was important to learn addition facts (Sokol et al., Reference Sokol, McCloskey, Cohen and Aliminosa1991); however, the intact linguistic motor areas sustained the verbal memory for the multiplication table (Zhou, Chen, Zang, et al., Reference Zhou, Chen, Zang, Dong, Chen, Qiao and Gong2007). Studies have demonstrated that schoolchildren are taught to use procedural strategies for simple addition such as counting and transformation (e.g., 6+7=6+6+1, 9+7=9+1+6), while they are taught to use a verbal memory strategy to memorize multiplication facts (Dehaene & Cohen, Reference Dehaene and Cohen1997; Roussel et al., Reference Roussel, Fayol and Barrouillet2002; Zhou, Chen, Zang, et al., Reference Zhou, Chen, Zang, Dong, Chen, Qiao and Gong2007).
With the verbal memory strategy, people repeatedly recite multiplication tables that could be stored as verbal memory. Moreover, functional MRI studies showed that multiplication activated more the precentral gyrus, supplementary motor areas, and posterior and anterior superior temporal gyrus relative to addition (Lee, Reference Lee2000; Zhou, Chen, Zang, et al., Reference Zhou, Chen, Zang, Dong, Chen, Qiao and Gong2007). This confirmed that learning multiplication relies heavily on verbal memory and is related to linguistic motor areas.
There was the dissociation between addition and multiplication in auditory modality but not in visual modality. Previous studies have shown lots of dissociations among simple arithmetic. The main finding is the dissociation between procedure-based arithmetic (e.g., addition) and verbal-based arithmetic (e.g., multiplication). The patients with verbal processing deficits typically had problems in multiplication but not in the procedure-based arithmetic (e.g., Cohen & Dehaene, Reference Cohen and Dehaene2000; Dehaene & Cohen, Reference Dehaene and Cohen1997; Delazer & Benke, Reference Delazer and Benke1997; Hittmair-Delazer et al., Reference Hittmair-Delazer, Semenza and Denes1994; Puvanendran, Dowker, & Demeyere, Reference Puvanendran, Dowker and Demeyere2016). For example, Broca’s aphasia had preserved performance in simple addition, but can retrieve no multiplication facts (Hittmair-Delazer et al., Reference Hittmair-Delazer, Semenza and Denes1994). The pure alexic patient, although unable to read correctly the operands of visually presented problems, could still produce verbally the exact result of the very same problems in subtraction, addition, and division tasks rather than multiplication task (Cohen & Dehaene, Reference Cohen and Dehaene2000). To the contrary, the patients with normal verbal processing could have regular multiplication performance but have impaired performance in the procedure-based arithmetic. For example, a Gerstmann’s syndrome apraxia patient cannot do simple addition and subtraction, but had preserved language and multiplication abilities (Delazer & Benke, Reference Delazer and Benke1997).
Furthermore, Cohen and Dehaene (Reference Cohen and Dehaene2000) also demonstrate a dissociation between multiplication and addition in auditory and visual presentation. The pattern was that the alexic patient impaired only in multiplication on visual Arabic input, but showed preserved performance in addition, subtraction, multiplication and division on orally input. The auditory input in the study could be transformed into visual code for addition, subtraction, and division, which were intact in visual modality. But the multiplication processing was more associated with verbally reading that could not realize the transformation. Therefore, the pattern of dissociation is different from the present results. In the present study, we found the dissociation between addition and multiplication in auditory modality but not in visual modality. We infer that the difference may be because the differential learning or practice strategies between Chinese and Western countries. Chinese individuals typically use the rote verbal strategy for multiplication. Auditory input could easily activate the verbal code to improve problem solving.
In the present study, the patient had a relatively preserved multiplication ability in auditory presentation. The auditory input format directly induced this verbal memory strategy for multiplication. Besides the contributing factor of rote verbal strategy, there may be other factor contributed to the pattern of dissociation. Even though the patient was impaired in language production and most numerical processing abilities compared to controls, he showed preserved verbal processing in basic numerical abilities (e.g., counting, Arabic number reading, number word reading, and Arabic number dictating) and semantic memory.
One of the factors might be the patient’s prevalent loss of simple arithmetic in Arabic digit input. The injuries in frontotemporal lobe and basal ganglia led a prevalent loss of simple arithmetic in Arabic digit input. The loss might be a type of production deficit, which might be equivalent to his overall language production deficits (including word rhyming, Chinese character reading, word repetition, verbal fluency, and naming of characters with low frequency). The auditory input could be helpful for the retrieval of the verbal-based multiplication because the auditory input could directly activate the verbal code and thus mitigate the production load.
However, the poor performance of addition in the visual modality could not be improved in auditory modality. For example, the patient in Cohen and Dehaene’s study (Reference Cohen and Dehaene2000) had nearly perfect performance in addition, subtraction and division on visual input, and also had similar performance on auditory input. It indicated that performances of addition, subtraction and division in visual modality were not affected by auditory modality. Therefore, the current findings support the notion of modality-dependent processing in multiplication. The learning strategy of multiplication table recitation could shape the verbal memory of multiplication.
ACKNOWLEDGMENTS
There are no conflicts of interest to declare. This research was supported by the Beijing Excellent Talents Program (2014000021469G227), the Cultivation Plan Fund for the Capital Institute of Pediatrics (PY-15-02), the Beijing Municipal Administration of Hospitals’ Youth Program (QML20151202) to Dazhi Cheng, the Capital Health Research and Development of Special (2016-2-2103) to Qian Chen, grants from the Natural Science Foundation of China (grant numbers 31271187 and 31221003) to Xinlin Zhou, and grants from Beijing National Science Foundation (7154227) and National Natural Science Foundation of China (81503480) to Rui Xu.
Supplementary material
To view supplementary material for this article, please visit https://doi.org/10.1017/S1355617717000479
APPENDIX 1
Patient Y.Q.’s Single-Digit Multiplication Performance for Visual and Auditory Presentation

APPENDIX 2
Patient Y.Q.’s Single-Digit Addition Performance for Visual and Auditory Presentation