Introduction
Spina bifida (SB) is a common congenital neurodevelopmental disorder that affects the development of the spine and brain and is associated with a modal cognitive and academic phenotype (Dennis, Landry, Barnes, & Fletcher, Reference Dennis, Landry, Barnes and Fletcher2006); in academics this includes better-developed word reading than mathematical skills (Ayr, Yeates, & Enrile, Reference Ayr, Yeates and Enrile2005; Barnes & Dennis, Reference Barnes and Dennis1992; Barnes et al., Reference Barnes, Pengelly, Dennis, Wilkinson, Rogers and Faulkner2002, Reference Barnes, Wilkinson, Khemani, Boudesquie, Dennis and Fletcher2006; Fletcher et al., Reference Fletcher, Dennis, Northrup, Barnes, Hannay, Landry, Francis and Glidden2004, Reference Fletcher, Copeland, Frederick, Blaser, Kramer, Northrup and Dennis2005). Because SB is associated with a high rate of math disability and the condition is diagnosed before or at birth, SB affords an opportunity to investigate early math abilities and their cognitive predictors in this disorder.
Approximately 50% of children and adolescents with SB who are not intellectually disabled have math difficulties, and over half of these children have specific math difficulties (or MD); that is, difficulties in math that are not accompanied by problems in reading (or RD) (Fletcher et al., Reference Fletcher, Copeland, Frederick, Blaser, Kramer, Northrup and Dennis2005). Cognitive studies of single- and multi-digit arithmetic in school-age children with SB show that the difficulties of those with MD or MD+RD (Ayr et al., Reference Ayr, Yeates and Enrile2005; Barnes et al., Reference Barnes, Pengelly, Dennis, Wilkinson, Rogers and Faulkner2002, Reference Barnes, Wilkinson, Khemani, Boudesquie, Dennis and Fletcher2006) are remarkably similar to those of children with no neurodevelopmental disorder who have MD and MD+RD (e.g., Hanich, Jordan, Kaplan, & Dick, Reference Hanich, Jordan, Kaplan and Dick2001; Raghubar et al., Reference Raghubar, Cirino, Barnes, Ewing-Cobbs, Fletcher and Fuchs2009). In adults with SB, math skills are stronger predictors of level of independence than IQ or literacy levels (Dennis & Barnes, Reference Dennis and Barnes2002; Hetherington, Dennis, Barnes, Drake, & Gentili, Reference Hetherington, Dennis, Barnes, Drake and Gentili2006). Although the nature of mathematical difficulties in school age children and adults with SB is becoming better understood, little is known about early developing math abilities in SB and the neurocognitive underpinnings of their early mathematical performance.
Correlates of Early Mathematical Development
Visual–spatial processes and early math
Visual–spatial abilities (encoding and mental manipulation of spatial information) and visual–spatial memory (ability to remember spatial locations or spatial sequences) may be particularly important in mathematical performance in young children and in new mathematical learning in older children (review in Raghubar, Barnes, & Hecht, Reference Raghubar, Barnes and Hecht2010). Based on longitudinal studies, both preschool visual–spatial abilities (Assel, Landry, Swank, Smith, & Steelman, Reference Assel, Landry, Swank, Smith and Steelman2003) and visual–spatial working memory (Bull, Espy, & Wiebe, Reference Bull, Espy and Wiebe2008) predict math achievement in the early grades. It has been suggested that preschoolers use mental models, which are nonverbal representations of the mathematical situation, to accomplish some mathematical tasks, particularly those involving transformations on quantity (Mix, Huttenlocher, & Levine, Reference Mix, Huttenlocher and Levine2002). An association between visual–spatial memory and quantity transformation tasks, such as object-based arithmetic and inversion, has been found for preschoolers (Rasmussen, Ho, & Bisanz, Reference Rasmussen, Ho and Bisanz2003), consistent with the idea that visual mental models are used to solve these problems (Bisanz, Sherman, Rasmussen, & Ho, Reference Bisanz, Sherman, Rasmussen, Ho and Campbell2005).
SB is associated with deficits in some aspects of visual–spatial processing (e.g., Dennis, Fletcher, Rogers, Hetherington, & Francis, Reference Dennis, Fletcher, Rogers, Hetherington and Francis2002; Dennis et al., Reference Dennis, Landry, Barnes and Fletcher2006). However, visual–spatial abilities are only weakly related to single- and multi-digit arithmetic in school-age children with and without SB (Ayr et al., Reference Ayr, Yeates and Enrile2005; Barnes et al., Reference Barnes, Pengelly, Dennis, Wilkinson, Rogers and Faulkner2002, Reference Barnes, Wilkinson, Khemani, Boudesquie, Dennis and Fletcher2006), although they are related to other domains of mathematics, including geometry and estimation (Barnes et al., Reference Barnes, Pengelly, Dennis, Wilkinson, Rogers and Faulkner2002). Given that visual spatial abilities may be used in the development of mental models, which in turn predict performance on informal mathematical tasks, the relation of visual spatial abilities to early math in SB is of considerable interest and has not been studied.
Language-based abilities and early math skills
Language-based abilities such as word knowledge, verbal working memory, and phonological skills predict performance on some mathematical tasks in both preschoolers and school-age children (Durand, Hulme, Larkin, & Snowling, Reference Durand, Hulme, Larkin and Snowling2005; LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010; review in Raghubar et al., Reference Raghubar, Barnes and Hecht2010). Language is thought to be important for mapping number words and symbols, representing exact number (Dehaene, Piazza, Pinel, & Cohen, Reference Dehaene, Piazza, Pinel, Cohen and Campbell2005), and using verbal strategies to solve some types of mathematical problems (Jordan, Kaplan, Olah, & Locuniak, Reference Jordan, Kaplan, Olah and Locuniak2006). Phonological processes, specifically phonological awareness and phonological working memory, are related to learning the number–word sequence in children from kindergarten to third grade (Krajewski & Schneider, Reference Krajewski and Schneider2009), predict growth in early math achievement (Hecht, Torgesen, Wagner, & Rashotte, Reference Hecht, Torgesen, Wagner and Rashotte2001), are related to math disabilities (Simmons & Singleton, Reference Simmons and Singleton2008; Swanson & Jerman, Reference Swanson and Jerman2006), and mediate individual differences in direct retrieval of answers to small addition problems (De Smedt, Taylor, Archibald, & Ansari, Reference De Smedt, Taylor, Archibald and Ansari2009). In school age children with SB, phonological ability is only weakly related to arithmetic skills (Barnes et al., Reference Barnes, Wilkinson, Khemani, Boudesquie, Dennis and Fletcher2006). However, little is known about the relation between language-based abilities such as vocabulary knowledge and phonological processes and early mathematical skills in either typically developing preschool children or in preschoolers with SB.
Fine motor abilities and early math
Finger gnosis has been linked to achievement in math in the early school grades (Noel, Reference Noel2005). Such findings have been used to argue for common neural representations of fingers and numbers because of their functional developmental connections through the use of fingers to count and calculate (e.g., Butterworth, Reference Butterworth1999). Fine motor skills involved in finger counting and pointing may also help young children compensate for limited working memory capacity by avoiding having to internally store a mental representation of each counted object (Alibali & DiRusso, Reference Alibali and DiRusso1999). Finger agility has also been linked to an ability to use fingers to perform counting and calculation procedures (Penner-Wilger et al., Reference Penner-Wilger, Fast, LeFevre, Smith-Chant, Skwarchuk, Kamawar, Bisanz, McNamara and Trafton2007). Older children with SB use their fingers more often than typically developing children when solving single digit arithmetic problems (Barnes et al., Reference Barnes, Wilkinson, Khemani, Boudesquie, Dennis and Fletcher2006). The influence of fine motor skills on math in preschoolers with SB may be of particular importance because the brain anomalies associated with SB affect fine motor skills such as finger function (Dennis et al., Reference Dennis, Landry, Barnes and Fletcher2006; Friedrich, Lovejoy, Shaffer, Shurtleff, & Beilke, Reference Friedrich, Lovejoy, Shaffer, Shurtleff and Beilke1991; Lomax-Bream, Barnes, Copeland, Taylor, & Landry, Reference Lomax-Bream, Barnes, Copeland, Taylor and Landry2007; Wills, Reference Wills1993).
Mathematical performance may draw on language-based, visual–spatial, and finger representations to greater or lesser extents depending on the cognitive characteristics of the child, the age of the child, and whether particular mathematical problems can be solved using different strategies (Ansari & Dhital, Reference Ansari and Dhital2006; Bull, Reference Bull, Berch and Mazzocco2007; LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010). Whether preschoolers with SB and typically developing preschoolers differ in the neurocognitive abilities they bring to bear in performing informal mathematical tasks is unknown.
The Present Study
This study has two aims: (1) to compare the performance of children with SB and their typically developing peers at 36 months and 60 months of age on measures of informal mathematical abilities that have been found to predict later math achievement in longitudinal studies (Jordan, Kaplan, Locuniak, & Ramineni, Reference Jordan, Kaplan, Locuniak and Ramineni2007; Jordan et al., Reference Jordan, Kaplan, Olah and Locuniak2006); and (2) to determine what neurocognitive abilities are related to different mathematical skills at each age in children with SB and in age peers.
Based on theories of mathematical development in which informal mathematical knowledge acquired by preschoolers forms the foundation for the acquisition of formal mathematical abilities at school-age (Ginsburg, Klein, & Starkey, Reference Ginsburg, Klein, Starkey, Damon, Sigel and Renninger1998), we hypothesize that difficulties in mathematics might be present as early as 36 and 60 months of age, such that preschoolers with SB will score lower than their typically developing (TD) peers on both standardized and experimental tests of informal mathematical knowledge. We also hypothesize that by 60 months of age, in comparison to TD peers, children with SB will demonstrate deficits on those informal math skills thought to draw more heavily on visual–spatial abilities (e.g., object-based arithmetic) and show less difficulty on math skills thought to be more strongly related to verbal ability (e.g., oral counting). Given differences in the neurocognitive profiles of children with SB and TD children, we ask whether the patterns of relations between math performance and abilities in domains other than mathematics (e.g., visual–spatial, language, fine motor) might differ between groups.
Method
Participants
This study is part of a longitudinal project on cognitive, motor, and social development in children with SB in Toronto, Ontario, and Houston, Texas. Starting in 1997, children with SB were identified by treating neurosurgeons and pediatricians and recruited into the study and examined several times between 6 and 60 months of age. Detailed inclusion and exclusion criteria for the larger study are in Lomax-Bream et al. (Reference Lomax-Bream, Barnes, Copeland, Taylor and Landry2007). In the current study, children from this larger group were excluded if: (1) they did not have complete correlates data (i.e., language, visual–spatial, fine motor variables) within an assessment point; (2) their performance on more than one mathematical task at each assessment point was unreliable; and (3) they had global intellectual impairment (i.e., their scores on both Vocabulary and Pattern Analysis subtests of the Stanford-Binet-IV [SB-IV: Thorndike, Hagen, & Sattler, Reference Thorndike, Hagen and Sattler1989] were more than 2 standard deviations (SD) below the population mean at 36 months, and their scores on both Picture Vocabulary and Spatial Relations subtests from the Woodcock Johnson Tests of Cognitive Abilities-Revised [WJ-R: Woodcock & Johnson, Reference Woodcock and Johnson1989] were more than 2 SD below the population mean at 60 months) (Table 1). This resulted in the loss of two children with SB at 36 months of age and at 60 months of age. Differences in numbers of participants on tasks within an assessment most often reflect lack of reliability for a task as coded by assessors (e.g., child fatigue, behavior). Differences in numbers between assessments were most often due to the inability of the family to attend the second assessment. At 60 months, SB-IV Quantitative was added after testing had begun, accounting for the lower sample size for this task.
Table 1 Descriptive information on spina bifida and control groups

Note. *Denotes a significant group difference. Values are standard scores except for Purdue Pegs which was number of errors at 36 months and pegs placed at 60 months. SB-IV = Stanford-Binet Intelligence Scales, Fourth Edition; WJ-R = Woodcock-Johnson Test of Cognitive Abilities—Revised; CTOPP = Comprehensive Test of Phonological Processing; Phonological Awareness Composite = CTOPP Sound Matching and CTOPP Elision raw scores.
The distribution of participants was relatively equal between the two sites. Most of the children had hydrocephalus treated with a diversionary shunt; nine children had arrested hydrocephalus and no shunt. The majority had lower spinal lesions below L1 (87% and 89% depending on the sample at each time point).
Table 1 shows the distributions of gender, ethnicity, and socioeconomic status (SES) as assessed with the Hollingshead (Reference Hollingshead1975) four-factor scale. The sociodemographics of the Texas and Ontario sites differed because the Texas site included more children of Hispanic origin. The TD group had a higher SES than the group with SB [(t(95) = 12.46, p < .001 at 36 months; t(90) = 3.25, p < .01 and at 60 months], mainly reflecting the greater number of economically disadvantaged Hispanic children with SB in Texas. Consequently, SES was used as a covariate in analyses involving group comparisons. Groups differed in gender with more female participants in the group with SB (χ2(1) = 5.84; p < .05 at 36 months; χ2(1) = 9.08; p < .01 at 60 months). Gender was not used as a covariate as it was not associated with math outcomes.
Measures and Procedures
Participants were assessed in a single session lasting between 1.5 and 3 hours depending on the assessment. Most were assessed at facilities associated with the project and some were assessed in their homes. Consent was obtained from parents in accordance with the institutional review boards at the University of Texas Health Science Center at Houston and the Toronto Hospital for Sick Children.
Preschool math measures at 36 months
The Test of Early Mathematics Ability-2 or TEMA-2 (Ginsburg & Baroody, Reference Ginsburg and Baroody1990) measures informal mathematics skills. The first several items measure counting small and larger sets of objects, showing number using fingers, quantity comparison including understanding of “more,” and understanding of cardinality. The test yields an age-standardized math quotient (internal consistency coefficient = .95 at 3 years of age). Because 36 months is the entry level for the test and to provide a larger range of scores at this age, we also used an experimental score derived by giving children credit for each correct answer they provided on the test (e.g., some items contain more than one question, but require that all be answered correctly to score 1 point),
Preschool math measures at 60 months
Counting principles
In this measure of counting knowledge (Briars & Siegler, Reference Briars and Siegler1984; Gelman & Galistell, Reference Gelman and Galistell1978), a hand puppet pointed to and counted colored dots on a page (12 dots for half of the trials, and 16 for the other half). Children were instructed to tell the puppet whether or not he/she was counting correctly. This measure assessed knowledge of one-to-one correspondence (one counting tag is applied to each object), stable order (number tags must be applied in an invariant order), and cardinality (the last number counted refers to the total quantity) principles.
The puppet counted correctly on 8 trials and incorrectly on 16 trials. One-to-one correspondence was violated on 4 trials (e.g., puppet skipped counting a dot); stable order on 6 trials (e.g., reversing two numbers in a count); and cardinality on 6 trials (e.g., puppet gives an incorrect answer when he is asked “how many things he counted”). Because we did not have different hypotheses corresponding to each principle, correct detection of counting errors was collapsed across principles, consistent with procedures used in other studies (e.g., LeFevre et al., Reference LeFevre, Smith-Chant, Fast, Skwarchuk, Sargla, Arnup and Kamawar2006). Unconventional counts (e.g., puppet counted all blue then all red dots) were administered, but not analyzed, given the findings of LeFevre et al. (Reference LeFevre, Smith-Chant, Fast, Skwarchuk, Sargla, Arnup and Kamawar2006) and Kamawar et al. (Reference Kamawar, LeFevre, Bisanz, Fast, Skwarchuk, Smith-Chant and Penner-Wilger2010) suggesting a curvilinear relation between age and the acceptance of these as correct counts that is moderated by individual differences in numeration abilities.
Oral counting
Procedural counting was measured by asking children to count as high as they could (Miller, Smith, Zhu, & Zhang, Reference Miller, Smith, Zhu and Zhang1995). The score was the highest number counted without any errors, with correct counts above 100 capped at 100.
Object-based arithmetic
In this task (based on Jordan, Huttenlocher, & Levine, Reference Jordan, Huttenlocher and Levine1992), the child watched the examiner place poker chips (from the examiner's box of 10 chips) on the examiner's mat. Then a screen was placed in front of the array to occlude the array from the child, and the child watched while the examiner added chips all at one time to the array (addition) or removed chips all at one time from behind the screen (subtraction). Children could not see the current quantity behind the screen but clearly saw the quantity that was added or removed. Children were then asked to use their chips (from their box of 10 chips) to match the quantity that was hidden after the transformation. Explicit quantity information was used in the instructions (e.g., “How many do I have under here now? Show me on your mat. Make yours like mine”). There were 12 trials (half addition and half subtraction). Five problems involved small size sets in the subitizable range (subitizing refers to the ability to discern exact quantity without counting up to 3 or 4; in this study, problems with quantities in the subitizable range involved a sum of 3 or less for addition and a subtrahend of 3 or less for subtraction; 1+1; 2−1; 2+1; 3−1; 3−2) and seven problems involved quantities of 4 or greater (sum or subtrahend 4 or greater; 1+3; 2+2, 4−1, 4−3, 4−2, 1+4, 2+3). Two demonstration items involving matching of one and two chips without covering the display were given followed by a matching phase (see Jordan, Huttenlocher, & Levine, Reference Jordan, Huttenlocher and Levine1994) in which the examiner placed between one and five disks on her mat, covered the display and asked the participant to make the same number on his/her mat. The task was discontinued for children who obtained less than two correct on matching, resulting in the loss of one participant from each group.
Stanford-Binet IV Quantitative (Thorndike et al., Reference Thorndike, Hagen and Sattler1989)
For 5-year-olds, items measure quantitative concepts including matching on the basis of number using blocks, counting using blocks, and addition using blocks and pictures.
Language-Based, Visual–Spatial, and Fine Motor Predictors
At 36 months, measures of visual–spatial ability (Pattern Analysis from the SB-IV), vocabulary knowledge (Vocabulary from the SB-IV), and fine motor skill (Purdue Pegboard, both hands) were used as predictors of mathematical performance. At 60 months, visual–spatial ability was measured using the Spatial Relations subtest of the WJ-R, vocabulary was assessed with WJ-R Picture Vocabulary, phonological awareness was assessed using a composite score combining the Elision and Sound Matching subtests from the Comprehensive Test of Phonological Processing (Wagner, Torgesen, & Rashotte, Reference Wagner, Torgesen and Rashotte1999), and fine motor skill was measured using Purdue Pegboard.
Results
36-Month Math Group Comparisons
At 36 months, analysis of covariance (ANCOVA) controlling for SES showed that the group with SB was lower than the TD group on the TEMA-2 Math Quotient, F(1,98) = 10.57; p < .01; η2 = .10, and on the TEMA-2 experimental score, F(1,98) = 4.10; p < .05; η2 = .04 (Table 2).
Table 2 Math outcomes at 36 and 60 months of age for spina bifida and control groups

Note. *Denotes a significant group difference after covarying for the effect of SES; SB-IV = Stanford-Binet Intelligence Scales, Fourth Edition.
60-Month Math Group Comparisons
In ANCOVAs covarying for SES, the group with SB scored lower than the TD group on Oral Counting, F(1,95) = 8.08; p < .01; η2 = .08; Large Set Object-Based Arithmetic F(1,90) = 6.44; p < .05; η2 = .07); Counting Principles, F(1,87) = 9.81; p < .01; η2 = .10; and SB-IV Quantitative, F(1,79) = 4.36; p < .05; η2 = .05. No differences were found on Small Set Object-Based Arithmetic, F(1,93) < 1 (Table 2).
Correlations among the non-math predictors at 36 months and 60 months are in Tables 3a and 3b, respectively.
Table 3a Correlations among 36-month predictors

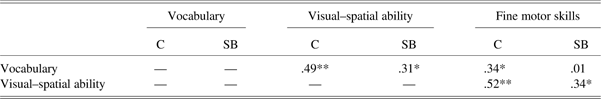
Note. * = p < .05; ** = p < .01; Fine motor = Purdue Pegs; Visual–spatial ability = SB-IV Pattern Analysis; Vocabulary = SB-IV Vocabulary.
Table 3b Correlations among 60-month predictors

Note. * = p < .05; ** = p < .01; Fine motor = Purdue Pegs; Visual–spatial ability = WJ-R Spatial Relations; Phonological Awareness = Composite of CTOPP Sound Matching and CTOPP Elision; Vocabulary = WJ-R Picture Vocabulary.
Non-Math Correlates of 36-Month Math Outcomes
Correlations among the non-math predictor variables (language, visual–spatial, and fine motor) and the TEMA-2 at 36 months are in Table 4a. Tests comparing the size of the correlations between groups revealed no significant findings. Hierarchical regressions were conducted to examine the predictors of math outcomes and to determine whether these varied as a function of group. The regressions included group in the first block, all of the non-math predictors in the second block, and the interaction between group and each of these predictors in the third block. The overall model accounted for 46% of the variance in TEMA-2 scores. The non-math correlates accounted for additional unique variance above that accounted for by group membership, F(3,85) = 15.97; p < .001; R2 change = .31, with vocabulary, visual–spatial ability, and fine motor skills, emerging as significant predictors. The interaction terms of group with each of the correlates did not account for additional variance (Table 5).
Table 4a Correlation matrix among predictors and outcomes at 36 months of age
Note. * = p < .05, ** = p < .01; Fine motor = Purdue Pegs; Visual–spatial ability = SB-IV Pattern Analysis; Vocabulary = SB-IV Vocabulary.
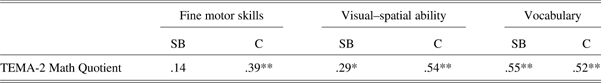
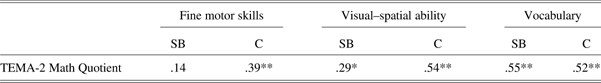
Table 4b Correlation matrix among predictors and outcomes at 60 months of age

Note. * = p < .05, ** = p < .01; Fine motor = Purdue Pegs; Visual–spatial ability = WJ-R Spatial Relations; Phonological Awareness = Composite of CTOPP Sound Matching and CTOPP Elision; Vocabulary = WJ-R Picture Vocabulary.
Table 5 Hierarchical regression for TEMA-2 at 36-months of age

Note. * = p < .05; ** = p < .01.
Non-Math Correlates of 60-Month Math Outcomes
The correlations among math measures and the non-math predictors at 60 months are in Table 4b. The size of the correlations between groups did not differ. Visual–spatial, phonological, vocabulary, and fine motor abilities were included in all models in the second block, with group and the interaction of group and each predictor in the first and third blocks, respectively (Table 6).
Table 6 Hierarchical regressions for math outcomes at 60-months of age

Note. * = p < .05; ** = p < .01; PA = Phonological Awareness.
Oral counting
The model accounted for 47% of the variance in oral counting. Non-math abilities accounted for unique variance, F(4,82) = 9.81; p < .001; R2 change = .28, with significant contributions from both phonological awareness and visual–spatial ability. The interaction terms did not account for unique variance.
Counting principles
This model accounted for 42% of variance in error detection. Non-math abilities accounted for unique variance, F(4,74) = 5.96; p < .001; R2 change = .20, with phonological awareness emerging as the only significant predictor. The interaction terms did not contribute to the model.
Quantitative
The model accounted for 32% of the variance in Quantitative scores. Non-math abilities accounted for additional unique variance, F(4,66) = 6.06; p < .001; R2 change = .25, with a significant contribution of visual–spatial ability. The interaction terms did not account for additional variance.
Small set arithmetic
In this model, only the non-math predictors were significant, F(4,77) = 3.79; p < .01; R2 = .16, specifically, fine motor skill.
Large set arithmetic
This model accounted for 42% of the variance in large set arithmetic with non-math abilities accounting for significant additional variance, F(4,75) = 10.36; p < .001; R2 change = .34. Visual–spatial ability and fine motor skills emerged as significant predictors. The interaction terms did not account for additional unique variance.
Predicting 60-month math outcomes from 36 month math and 60 month non-math abilities
We were interested in whether language-based, visual–spatial and fine motor abilities from 60 months predicted unique variance in 60-month math outcomes after controlling for the autoregressive effects of mathematical skills at 36 months (TEMA-2) and whether such effects varied as a function of group. In longitudinal academic research when cognitive variables are significant after the inclusion of the autoregressor (measure of academic skill from an earlier time point), this is taken as evidence of their importance for the performance of that skill (de Jong & van der Leij, Reference de Jong and van der Leij2002). Here, the skill of interest is mathematical knowledge and so TEMA-2 performance at 36 months was used as the autoregressor. The question is whether visual–spatial, language-based, and fine motor skills are important for mathematical performance at 5 years of age after accounting for the child's level of mathematical knowledge from an earlier developmental time-point.
For all analyses group membership was in the first block, raw scores from the TEMA-2 experimental measure from 36 months were entered in the second block, the 60-month non-math abilities were entered in the third block, and the interaction term of group with each of the relevant abilities was entered in the fourth block (Table 7).
Table 7 Hierarchical regressions of math outcomes at 60-months of age controlling for the autoregressive effects of math skill at 36-months of age

Note. * = p < .05; ** = p < .01; PA = Phonological Awareness.
Oral counting
Non-math abilities accounted for significant variance, F(4,64) = 2.86; p < .05; R2 change = .11, after accounting for 36-month math performance, with significant contributions from both phonological awareness and visual–spatial abilities. The inclusion of the interaction terms did not add significantly to the model.
Counting principles
The TEMA-2 accounted for additional variance in error detection when added after group, but neither the predictor abilities nor the interaction terms added significantly to the model.
Quantitative
Non-math abilities accounted for additional variance after accounting for 36-month math performance, F(4,49) = 2.93; p < .05; R2 change = .16, with visual–spatial abilities emerging as a significant predictor. The interaction terms did not account for additional unique variance.
Small set arithmetic
The TEMA-2 significantly predicted small set arithmetic performance when added after group. However, neither the non-math abilities nor the interaction terms significantly contributed to the model.
Large set arithmetic
Non-math abilities accounted for unique variance, F(4,59) = 6.30; p < .001; R2 change = .25, after accounting for 36-month math performance with significant contributions from visual–spatial and fine motor skills. The inclusion of the interaction terms did not contribute to the model.
Discussion
SB is a disorder that is associated with a high risk of math disability by school-age (Fletcher et al., Reference Fletcher, Copeland, Frederick, Blaser, Kramer, Northrup and Dennis2005). In this study of preschoolers, we hypothesized that difficulties in mathematics would be present in 3- and 5-year-old children with SB. Support for this hypothesis was provided by the findings that at 36 months, the group with SB had less mathematical knowledge than TD peers on a standardized test of informal mathematics. At 60 months, they were less skilled on measures of counting knowledge, oral counting, large set object-based arithmetic, and a standardized test of quantitative concepts. Thus mathematical difficulties can be discerned early in development in SB, and these difficulties extend to most areas of informal mathematics as might be expected based on theories of mathematical development in which informal mathematical abilities lay the foundation for the development of formal mathematical skills at school-age (Ginsburg et al., Reference Ginsburg, Klein, Starkey, Damon, Sigel and Renninger1998).
Small set object-based arithmetic, involving the ability to copy transformations on quantities in the subitizable range, was the only math task on which preschoolers with SB did not differ from their TD peers, and although performance was high, neither group was at ceiling. It has been hypothesized that quantities in the subitizable range are handled by object files (Trick & Plyshyn, Reference Trick and Plyshyn1994), which are episodic visual representations involved in the storage and updating of information about small sets of objects as they move in time and space (Noles, Scholl, & Mitroff, Reference Noles, Scholl and Mitroff2005). Object-based representations, which involve categorical visual perception, are better developed in SB than other aspects of visual–spatial performance (Dennis et al., Reference Dennis, Fletcher, Rogers, Hetherington and Francis2002; review in Dennis & Barnes, Reference Dennis and Barnes2010). Whether object-file tracking is intact in SB and related to small set arithmetic performance is not known.
Our hypotheses that the group with SB would show relative strengths in math skills presumed to rely on language-based representations and greater difficulty on tasks thought to rely on visual–spatial representations were not supported. Although children with SB had difficulty on large set object-based arithmetic, which is related to visual–spatial memory in preschoolers (Rasmussen & Bisanz, Reference Rasmussen and Bisanz2005), their performance on tasks measuring counting knowledge and procedures was equally disrupted. Counting is often characterized as drawing on verbal/phonological abilities, but the findings discussed below suggest that, in preschoolers, both language-based and visual–spatial processes may be important. Furthermore, even though verbal and phonological abilities of the group with SB were average, they were lower than those of the TD group, suggesting that the phonological representations supporting counting are of lower quality in preschoolers with SB than in their TD peers.
Because SB is associated with both assets and deficits in the cognitive correlates of math (Dennis et al., Reference Dennis, Landry, Barnes and Fletcher2006; Dennis & Barnes, Reference Dennis and Barnes2010), and because a particular mathematical task may be performed using different strategies or representational systems (Ansari & Dhital, Reference Ansari and Dhital2006; Bull, Reference Bull, Berch and Mazzocco2007), we hypothesized that the correlates of mathematical performance might differ in preschoolers with SB and their TD peers. The findings revealed similarities rather than differences between groups.
At 36 months, performance on the TEMA-2 was uniquely related to visual–spatial ability, vocabulary knowledge, and fine motor skill in both groups. The TEMA-2 assesses a range of informal mathematical skills in the preschool period (quantity comparison, counting, comprehension of mathematical language) so it is not surprising that several neurocognitive abilities predict performance on this measure as mathematical thinking does not reflect a unitary skill in either younger or older children (Ginsburg et al., Reference Ginsburg, Klein, Starkey, Damon, Sigel and Renninger1998; LeFevre, Reference LeFevre2000; LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010).
Findings for 60-month mathematical outcomes are discussed for each math task below.
Phonological awareness uniquely predicted the ability to detect violations of counting principles in both groups. This is consistent with studies of older TD children in which phonological memory seems to be important for monitoring counting errors (Geary, Hoard, & Hamson, Reference Geary, Hoard and Hamson1999). For these younger children in the current study, phonological awareness likely indexes the quality of their phonological representations, which may be important for monitoring what the puppet says.
Both phonological awareness and visual–spatial ability were uniquely predictive of oral counting even in the longitudinal analyses accounting for performance on the TEMA-2, which involves several counting items at age 3. These findings are consistent with a recent study showing that visual–spatial attention is a unique predictor of performance on both verbal and nonverbal measures of early numeracy (LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010). Although phonological codes are directly related to learning the first several words in the verbal counting string (Krajewski & Schneider, Reference Krajewski and Schneider2009), counting into higher numbers may additionally involve the mapping of verbal number symbols onto space, perhaps reflecting the emergence of the mental number line in children this age (Dehaene, Izard, Spelke, & Pica, Reference Dehaene, Izard, Spelke and Pica2008). Our findings in preschoolers are compatible with adult neuropsychological studies (Piazza, Mechelli, Price, & Butterworth, Reference Piazza, Mechelli, Price and Butterworth2006) in which counting activates not only language association areas, but also those regions of brain implicated in other mathematical tasks that do not involve counting (Piazza, Mechelli, Butterworth, & Price, Reference Piazza, Mechelli, Butterworth and Price2002). Importantly, the data suggest that the correlates of what appears to be an overtly verbal task may include visual–spatial competence during skill development (Ansari et al., Reference Ansari, Donlan, Thomas, Ewing, Peen and Karmiloff-Smith2003; LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010).
Of interest, phonological awareness emerged as the unique language-based predictor at 5 years of age for both oral counting and counting knowledge. As suggested by De Smedt et al. (Reference De Smedt, Taylor, Archibald and Ansari2009), phonological awareness, more so than measures of word meaning, tap the quality of lexical representations, which might be an important mediator of some mathematical skills such as math fact retrieval. Because counting is a developmental precursor to learning arithmetic facts, phonological representations might also be important for acquiring those counting skills that lay the foundation for arithmetic problem solving.
Visual–spatial and fine motor abilities uniquely predicted large set object-based arithmetic for both groups. Given that tests of visual–spatial ability and visual–spatial working memory largely measure similar constructs (Miyake, Friedman, Rettinger, Shah, & Hegarty, Reference Miyake, Friedman, Rettinger, Shah and Hegarty2001), our findings are consistent with studies relating visual–spatial memory to object-based arithmetic in typically developing preschoolers (LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010; Rasmussen & Bisanz, Reference Rasmussen and Bisanz2005), and with the idea that mental models are used to solve these types of problems (Bisanz et al., Reference Bisanz, Sherman, Rasmussen, Ho and Campbell2005).
The findings on the relations of fine motor skill and large set object-based arithmetic are interesting for three reasons: (1) Fine motor skill is typically not assessed in studies of the correlates of these informal math abilities so the finding that it was a unique predictor of object-based arithmetic is novel. (2) Because fine motor skill is also a unique predictor of multi-digit calculation in older TD children (Noel, Reference Noel2005) and children with SB (Barnes, Smith-Chant, Landry, Reference Barnes, Smith-Chant, Landry and Campbell2005), the findings provide evidence for cognitive continuity in object-based or nonverbal and symbolic arithmetic problem solving. (3) Because fine motor skill was specifically related to transformations on number and not to other mathematical abilities, the findings provide evidence for the view that the development of early math skills is multi-componential (LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010).
What is the nature of the relation between fingers and arithmetic? One hypothesis is that because young children use fingers to solve simple arithmetic problems, fingers and calculation come to have functional and neural connections (Butterworth, Reference Butterworth1999). It is assumed that fingers are used to represent objects during problem solving. However, in object-based arithmetic the objects are actually present so children might use their fingers to help them keep track of the hidden transformation on number. It is also possible that finger skills are only related to performance on object-based arithmetic tasks when the task requires a motor response (e.g., vs. Hodent, Bryant, & Houde, Reference Hodent, Bryant and Houde2005). Another possibility for the relation of fine motor skill and object-based arithmetic is that both may rely on the integrity of parietal lobes (Penner-Wilger & Anderson, Reference Penner-Wilger, Anderson, Love, McRae and Sloutsky2008), which are thinned in SB (Fletcher et al., Reference Fletcher, Copeland, Frederick, Blaser, Kramer, Northrup and Dennis2005). Given that finger counting habits moderate the association between space and number (Fischer, Reference Fischer2008), understanding the connections between finger skills, visual–spatial ability, and calculation in preschoolers is important.
Because the abilities that were related to small set object-based arithmetic differed from those for large set object-based arithmetic, operations on small sets may be processed differently from those involving transformation on larger quantities. However, most studies do not separately analyze small and large object-based arithmetic problems. As was argued for oral counting, these findings underscore the importance of considering that different strategies and/or representational systems may be involved in mathematical operations that, on the surface, appear more similar than different.
Recent studies show that mathematical performance in children with and without math disabilities is predicted by both domain-specific number and domain-general cognitive skills (Fuchs et al., Reference Fuchs, Geary, Compton, Fuchs, Hamlett and Bryant2010; Geary et al., Reference Geary, Bailey, Littlefield, Wood, Hoard and Nugent2009; LeFevre et al., Reference LeFevre, Fast, Skwarchuk, Smith-Chant, Bisanz, Kamawar and Penner-Wilger2010). Consequently, we thought that math performance at 5 years would be predicted by concurrent neurocognitive abilities after accounting for earlier-developing number abilities at 3 years. The combination of earlier mathematical skill and later language-based, visual–spatial, and fine motor abilities accounted for substantial proportions of variance in informal mathematical outcomes. One view is that it is the combination of early deficits in domain-general and domain-specific math abilities that best predicts later severe mathematical disability (Geary et al., Reference Geary, Bailey, Littlefield, Wood, Hoard and Nugent2009). Whether deficits in domain-specific and domain-general abilities at preschool will predict mathematical disability in school-age children with SB is a question of considerable interest in our ongoing longitudinal study.
Limitations and implications
Consistent with most studies of early mathematical performance, the current study lacks measures of strategy-use, which would be helpful in deciding whether the findings for cognitive correlates reflect differences in the underlying representational systems and strategies brought to bear in mathematical problem solving. Another limitation is that we were unable to use all the same tasks at both ages. We also did not include some cognitive correlates such as working memory that are related to mathematical achievement in younger and older children with and without SB (Blair & Razza, Reference Blair and Razza2007; Bull et al., Reference Bull, Espy and Wiebe2008; English, Barnes, Taylor, & Landry, Reference English, Barnes, Taylor and Landry2009). Finally, although the TD group was average on most standardized cognitive and academic measures, their vocabulary skills at 60 months were relatively high, suggesting a cautious approach to the interpretation of group differences on mathematical tasks.
This is the first study to test the emergence of mathematical difficulties and their correlates in very young children with SB. The findings have implications for both early identification of risk and for intervention strategies. Several of the 60-month math tasks measure early “number sense” (Berch, Reference Berch2005) and have been useful in predicting later math achievement in longitudinal studies (e.g., Jordan et al., Reference Jordan, Kaplan, Olah and Locuniak2006, Reference Jordan, Kaplan, Locuniak and Ramineni2007). Whether these measures of early number sense in preschoolers with SB also predict individual differences and growth in their later mathematical achievement could have implications for the early identification of risk in these children. Finally, similarities in the neurocognitive correlates of mathematical performance in TD children and in those with SB suggest that effective math interventions for TD children might also hold promise for individuals with SB (see Coughlin & Montague, Reference Coughlin and Montague2010).
Acknowledgments
This research was supported by grants from the National Institute of Child Health and Development, P01 HD35946, Spina Bifida: Cognitive and Neurobiological Variability and R01HD046609, Longitudinal Effects of Spina Bifida on Learning, and by funding from the Canadian Institutes of Health Research and from the CIHR Institute of Human Development, Child and Youth Health. We thank Stephanie Lane, Michelle Ladd, Monica Gomez, Francis Leal, and Laura Lomax-Bream for their assistance. The authors report no financial, professional, or personal conflicts of interest in the conduct of this study.