No CrossRef data available.
Published online by Cambridge University Press: 16 January 2020
Let 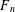 $F_{n}$ and
$F_{n}$ and 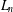 $L_{n}$ be the Fibonacci and Lucas numbers, respectively. Four corresponding zeta functions in
$L_{n}$ be the Fibonacci and Lucas numbers, respectively. Four corresponding zeta functions in  $s$ are defined by
$s$ are defined by 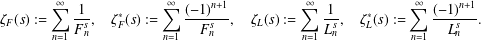 $$\begin{eqnarray}\unicode[STIX]{x1D701}_{F}(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{1}{F_{n}^{s}}},\quad \unicode[STIX]{x1D701}_{F}^{\ast }(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{(-1)^{n+1}}{F_{n}^{s}}},\quad \unicode[STIX]{x1D701}_{L}(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{1}{L_{n}^{s}}},\quad \unicode[STIX]{x1D701}_{L}^{\ast }(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{(-1)^{n+1}}{L_{n}^{s}}}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D701}_{F}(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{1}{F_{n}^{s}}},\quad \unicode[STIX]{x1D701}_{F}^{\ast }(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{(-1)^{n+1}}{F_{n}^{s}}},\quad \unicode[STIX]{x1D701}_{L}(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{1}{L_{n}^{s}}},\quad \unicode[STIX]{x1D701}_{L}^{\ast }(s):=\mathop{\sum }_{n=1}^{\infty }{\displaystyle \frac{(-1)^{n+1}}{L_{n}^{s}}}.\end{eqnarray}$$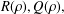 $R(\unicode[STIX]{x1D70C}),Q(\unicode[STIX]{x1D70C}),$ and
$R(\unicode[STIX]{x1D70C}),Q(\unicode[STIX]{x1D70C}),$ and  $P(\unicode[STIX]{x1D70C})$ for any algebraic number
$P(\unicode[STIX]{x1D70C})$ for any algebraic number  $\unicode[STIX]{x1D70C}$ with
$\unicode[STIX]{x1D70C}$ with  $0<\unicode[STIX]{x1D70C}<1$, the algebraic independence or dependence of various sets of these numbers is already known for positive even integers
$0<\unicode[STIX]{x1D70C}<1$, the algebraic independence or dependence of various sets of these numbers is already known for positive even integers  $s$. In this paper, we investigate linear forms in the above zeta functions and determine the dimension of linear spaces spanned by such linear forms. In particular, it is established that for any positive integer
$s$. In this paper, we investigate linear forms in the above zeta functions and determine the dimension of linear spaces spanned by such linear forms. In particular, it is established that for any positive integer  $m$ the solutions of
$m$ the solutions of  $$\begin{eqnarray}\mathop{\sum }_{s=1}^{m}(t_{s}\unicode[STIX]{x1D701}_{F}(2s)+u_{s}\unicode[STIX]{x1D701}_{F}^{\ast }(2s)+v_{s}\unicode[STIX]{x1D701}_{L}(2s)+w_{s}\unicode[STIX]{x1D701}_{L}^{\ast }(2s))=0\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{s=1}^{m}(t_{s}\unicode[STIX]{x1D701}_{F}(2s)+u_{s}\unicode[STIX]{x1D701}_{F}^{\ast }(2s)+v_{s}\unicode[STIX]{x1D701}_{L}(2s)+w_{s}\unicode[STIX]{x1D701}_{L}^{\ast }(2s))=0\end{eqnarray}$$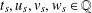 $t_{s},u_{s},v_{s},w_{s}\in \mathbb{Q}$
$t_{s},u_{s},v_{s},w_{s}\in \mathbb{Q}$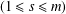 $(1\leq s\leq m)$ form a
$(1\leq s\leq m)$ form a  $\mathbb{Q}$-vector space of dimension
$\mathbb{Q}$-vector space of dimension  $m$. This proves a conjecture from the Ph.D. thesis of Stein, who, in 2012, was inspired by the relation
$m$. This proves a conjecture from the Ph.D. thesis of Stein, who, in 2012, was inspired by the relation  $-2\unicode[STIX]{x1D701}_{F}(2)+\unicode[STIX]{x1D701}_{F}^{\ast }(2)+5\unicode[STIX]{x1D701}_{L}^{\ast }(2)=0$. All the results are also true for zeta functions in
$-2\unicode[STIX]{x1D701}_{F}(2)+\unicode[STIX]{x1D701}_{F}^{\ast }(2)+5\unicode[STIX]{x1D701}_{L}^{\ast }(2)=0$. All the results are also true for zeta functions in 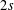 $2s$, where the Fibonacci and Lucas numbers are replaced by numbers from sequences satisfying a second-order recurrence formula.
$2s$, where the Fibonacci and Lucas numbers are replaced by numbers from sequences satisfying a second-order recurrence formula.
Communicated by M. Coons