No CrossRef data available.
Published online by Cambridge University Press: 30 May 2018
Let  $G$ be a semisimple complex algebraic group with Lie algebra
$G$ be a semisimple complex algebraic group with Lie algebra 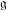 $\mathfrak{g}$. For a nilpotent
$\mathfrak{g}$. For a nilpotent 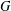 $G$-orbit
$G$-orbit 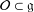 ${\mathcal{O}}\subset \mathfrak{g}$, let
${\mathcal{O}}\subset \mathfrak{g}$, let 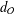 $d_{{\mathcal{O}}}$ denote the maximal dimension of a subspace
$d_{{\mathcal{O}}}$ denote the maximal dimension of a subspace 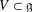 $V\subset \mathfrak{g}$ that is contained in the closure of
$V\subset \mathfrak{g}$ that is contained in the closure of 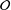 ${\mathcal{O}}$. In this note, we prove that
${\mathcal{O}}$. In this note, we prove that  $d_{{\mathcal{O}}}\leq {\textstyle \frac{1}{2}}\dim {\mathcal{O}}$ and this upper bound is attained if and only if
$d_{{\mathcal{O}}}\leq {\textstyle \frac{1}{2}}\dim {\mathcal{O}}$ and this upper bound is attained if and only if 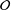 ${\mathcal{O}}$ is a Richardson orbit. Furthermore, if
${\mathcal{O}}$ is a Richardson orbit. Furthermore, if 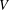 $V$ is
$V$ is 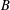 $B$-stable and
$B$-stable and 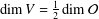 $\dim V={\textstyle \frac{1}{2}}\dim {\mathcal{O}}$, then
$\dim V={\textstyle \frac{1}{2}}\dim {\mathcal{O}}$, then 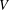 $V$ is the nilradical of a polarisation of
$V$ is the nilradical of a polarisation of 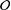 ${\mathcal{O}}$. Every nilpotent orbit closure has a distinguished
${\mathcal{O}}$. Every nilpotent orbit closure has a distinguished  $B$-stable subspace constructed via an
$B$-stable subspace constructed via an 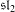 $\mathfrak{sl}_{2}$-triple, which is called the Dynkin ideal. We then characterise the nilpotent orbits
$\mathfrak{sl}_{2}$-triple, which is called the Dynkin ideal. We then characterise the nilpotent orbits 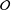 ${\mathcal{O}}$ such that the Dynkin ideal (1) has the minimal dimension among all
${\mathcal{O}}$ such that the Dynkin ideal (1) has the minimal dimension among all 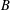 $B$-stable subspaces
$B$-stable subspaces  $\mathfrak{c}$ such that
$\mathfrak{c}$ such that 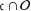 $\mathfrak{c}\cap {\mathcal{O}}$ is dense in
$\mathfrak{c}\cap {\mathcal{O}}$ is dense in  $\mathfrak{c}$, or (2) is the only
$\mathfrak{c}$, or (2) is the only  $B$-stable subspace
$B$-stable subspace  $\mathfrak{c}$ such that
$\mathfrak{c}$ such that 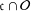 $\mathfrak{c}\cap {\mathcal{O}}$ is dense in
$\mathfrak{c}\cap {\mathcal{O}}$ is dense in  $\mathfrak{c}$.
$\mathfrak{c}$.
The research of the first author was carried out at the IITP R.A.S. at the expense of the Russian Foundation for Sciences (project no. 14-50-00150). The second author is partially supported by the DFG priority programme SPP 1388 ‘Darstellungstheorie’ and by the Graduiertenkolleg GRK 1523 ‘Quanten- und Gravitationsfelder’.