Hostname: page-component-745bb68f8f-lrblm Total loading time: 0 Render date: 2025-02-06T13:14:15.140Z Has data issue: false hasContentIssue false
  • English
  • Français

DECIDABLE ALGEBRAIC FIELDS

Published online by Cambridge University Press:  19 June 2017

MOSHE JARDEN  and
ALEXANDRA SHLAPENTOKH
MOSHE JARDEN
Affiliation:
SCHOOL OF MATHEMATICS TEL AVIV UNIVERSITY RAMAT AVIV TEL AVIV6139001, ISRAELE-mail: jarden@post.tau.ac.il
ALEXANDRA SHLAPENTOKH
Affiliation:
DEPARTMENT OF MATHEMATICS EAST CAROLINA UNIVERSITY UNIVERSITY GREENVILLE NC27858-4353, USAE-mail: shlapentokha@ecu.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We discuss the connection between decidability of a theory of a large algebraic extensions of 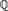${\Bbb Q}$ and the recursiveness of the field as a subset of a fixed algebraic closure. In particular, we prove that if an algebraic extension K of 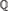${\Bbb Q}$ has a decidable existential theory, then within any fixed algebraic closure $\widetilde{\Bbb Q}$ of ${\Bbb Q}$, the field K must be conjugate over 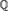${\Bbb Q}$ to a field which is recursive as a subset of the algebraic closure. We also show that for each positive integer e there are infinitely many e-tuples $\sigma \in {\text{Gal}}\left( {\Bbb Q} \right)^e $ such that the field 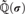$\widetilde{\Bbb Q}\left( \sigma \right)$ is primitive recursive in 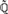$\widetilde{\Bbb Q}$ and its elementary theory is primitive recursively decidable. Moreover, 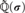$\widetilde{\Bbb Q}\left( \sigma \right)$ is PAC and 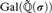${\text{Gal}}\left( {\widetilde{\Bbb Q}\left( \sigma \right)} \right)$ is isomorphic to the free profinite group on e generators.

Keywords

12E3003C0703D35decidable theoryrecursive subsets
Type
Articles
Information
The Journal of Symbolic Logic , Volume 82 , Issue 2 , June 2017 , pp. 474 - 488
Copyright
Copyright © The Association for Symbolic Logic 2017 

References

REFERENCES

Ershov, Y. L., Nice local-global fields I. Algebra and Logic, vol. 35 (1996), pp. 229–235.CrossRefGoogle Scholar
Fried, M., Haran, D., and Jarden, M., Galois stratification over Frobenius fields . Advances of Mathematics, vol. 51 (1984), pp. 1–35.Google Scholar
Fried, M. D., Haran, D., and Völklein, H., Real hilbertianity and the field of totally real numbers . Contemporary Mathematics, vol. 174 (1994), pp. 1–34.Google Scholar
Fried, M. D. and Jarden, M., Field Arithmetic, third ed., revised by Jarden, Moshe, der Mathematik, Ergebnisse (3), vol. 11, Springer, Heidelberg, 2008.Google Scholar
Halmos, P. R., Measure Theory, D. Van Nostrand Company, Princeton, 1968.Google Scholar
Huppert, B., Angewandte Linear Algebra, de Gruyter, Berlin, 1990.Google Scholar
Jarden, M., Intersection of local algebraic extensions of a Hilbertian field , Generators and Relations in Groups and Geometries (Barlotti, A., Ellers, E. W., Plaumann, P. and Strambach, K., editors), NATO ASI Series C, vol. 333, Kluwer, Dordrecht, 1991, pp. 343–405.Google Scholar
Jarden, M. and Kiehne, U., The elementary theory of algebraic fields of finite corank . Inventiones Mathematicae, vol. 30 (1975), pp. 275–294.Google Scholar
Lang, S., Algebra, third ed., Addison-Wesley, Reading, 1993.Google Scholar
Marker, D., Model Theory: An Introduction, Springer, New York, 2002.Google Scholar
Prestel, A., Pseudo real closed fields , Set Theory and Model Theory, Lecture Notes, vol. 872, Springer, Berlin-New York, 1981, pp. 127–156.Google Scholar
Prestel, A. and Roquette, P., Formally p-adic Fields, Lecture Notes in Mathematics, vol. 1050, Springer, Berlin, 1984.Google Scholar
Schmidt, F. K., Mehrfach perfekte Körper . Mathematische Annalen, vol. 108 (1933), pp. 1–25.Google Scholar
Tarski, A., A decision Method for Elementary Algebra and Geometry, The RAND Corporation, Santa Monica, 1948. Available at http://www.rand.org/content/dam/rand/pubs/reports/2008/R109.pdf.Google Scholar
Walkowiak, Y., Théorème d’irréductibilité de Hilbert effectif . Acta Arithmetica, vol. 116 (2005), pp. 343–362.Google Scholar
Weispfenning, V., Quantifier elimination and decision procedures for valued fields , Models and Sets, Lecture Notes in Mathematics, vol. 1103, Springer, Berlin, 1984, pp. 419–472.Google Scholar