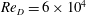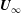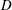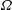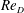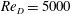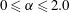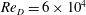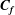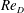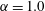1 Introduction
The drag crisis for flow over a smooth, non-rotating cylinder, where a sharp decrease of the drag coefficient occurs over a relatively narrow band of Reynolds number
![]() $Re_{D}$
, was first noted by Taylor around 1915 (Dryden, Murnaghan & Bateman Reference Dryden, Murnaghan and Bateman1932). A widely accepted interpretation of the drag crisis is centred on the onset of flow transition to turbulence that occurs initially on the separating shear layer on one side of the cylinder and which then migrates to the upstream boundary layer. This produces a robust near-wall flow that better resists the adverse pressure gradient, leading to a subsequent downstream movement of the primary-separation zone, and reduces pressure drag. A related phenomenon is the presence of a mean-flow, secondary-separation bubble which resides within the primary-separation zone for subcritical flow (Son & Hanratty Reference Son and Hanratty1969), moving upstream of the primary-separation bubble as
$Re_{D}$
, was first noted by Taylor around 1915 (Dryden, Murnaghan & Bateman Reference Dryden, Murnaghan and Bateman1932). A widely accepted interpretation of the drag crisis is centred on the onset of flow transition to turbulence that occurs initially on the separating shear layer on one side of the cylinder and which then migrates to the upstream boundary layer. This produces a robust near-wall flow that better resists the adverse pressure gradient, leading to a subsequent downstream movement of the primary-separation zone, and reduces pressure drag. A related phenomenon is the presence of a mean-flow, secondary-separation bubble which resides within the primary-separation zone for subcritical flow (Son & Hanratty Reference Son and Hanratty1969), moving upstream of the primary-separation bubble as
![]() $Re_{D}$
reaches the supercritical regime (Schewe Reference Schewe1983).
$Re_{D}$
reaches the supercritical regime (Schewe Reference Schewe1983).
Large-eddy simulation (LES) by Lehmkuhl et al. (Reference Lehmkuhl, Rodríguez, Borrell, Chiva and Oliva2014) showed a mean-flow separation bubble upstream of the primary-separation zone at supercritical
![]() $Re_{D}$
. Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) investigated the detailed near-surface flow behaviour through the drag crisis using LES. Their azimuthal skin-friction profiles at
$Re_{D}$
. Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) investigated the detailed near-surface flow behaviour through the drag crisis using LES. Their azimuthal skin-friction profiles at
![]() $Re_{D}=8.5\times 10^{5}$
capture boundary-layer transition and agree well with experiments by Achenbach (Reference Achenbach1968). Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) observed local mean-flow separation bubbles at both subcritical and supercritical
$Re_{D}=8.5\times 10^{5}$
capture boundary-layer transition and agree well with experiments by Achenbach (Reference Achenbach1968). Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) observed local mean-flow separation bubbles at both subcritical and supercritical
![]() $Re_{D}$
. They hypothesize that the drag crisis is a consequence of a global change in the overall flow state produced by the interaction of unsteady, secondary flow reattachment cells, visible as bundles of diverging surface streamlines within the large-scale separation region, with the principal separation shear layer. They further argue that boundary-layer laminar–turbulent transition is not itself the dynamical agent of the drag crisis but instead is an effect of this changed flow state. Some evidence supporting this interpretation is provided by Cheng, Pullin & Samtaney (Reference Cheng, Pullin and Samtaney2018), who find a drag crisis for flow past a grooved cylinder where well-developed boundary layers do not exist.
$Re_{D}$
. They hypothesize that the drag crisis is a consequence of a global change in the overall flow state produced by the interaction of unsteady, secondary flow reattachment cells, visible as bundles of diverging surface streamlines within the large-scale separation region, with the principal separation shear layer. They further argue that boundary-layer laminar–turbulent transition is not itself the dynamical agent of the drag crisis but instead is an effect of this changed flow state. Some evidence supporting this interpretation is provided by Cheng, Pullin & Samtaney (Reference Cheng, Pullin and Samtaney2018), who find a drag crisis for flow past a grooved cylinder where well-developed boundary layers do not exist.
When the cylinder rotates about its axis with constant angular velocity
![]() $\unicode[STIX]{x1D6FA}$
, a new parameter, the non-dimensional rotation speed
$\unicode[STIX]{x1D6FA}$
, a new parameter, the non-dimensional rotation speed
![]() $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FA}D/(2U_{\infty })$
is introduced, resulting in cylinder top–bottom flow asymmetry. Initial experimental studies (Reid Reference Reid1924; Thom Reference Thom1931) showed that the lift coefficient
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FA}D/(2U_{\infty })$
is introduced, resulting in cylinder top–bottom flow asymmetry. Initial experimental studies (Reid Reference Reid1924; Thom Reference Thom1931) showed that the lift coefficient
![]() $C_{L}$
generally increases as
$C_{L}$
generally increases as
![]() $\unicode[STIX]{x1D6FC}$
increases at fixed
$\unicode[STIX]{x1D6FC}$
increases at fixed
![]() $Re_{D}$
. A significant portion of the
$Re_{D}$
. A significant portion of the
![]() $Re_{D}{-}\unicode[STIX]{x1D6FC}$
plane was explored experimentally by Swanson (Reference Swanson1961), who found that, at fixed
$Re_{D}{-}\unicode[STIX]{x1D6FC}$
plane was explored experimentally by Swanson (Reference Swanson1961), who found that, at fixed
![]() $Re_{D}$
greater than approximately
$Re_{D}$
greater than approximately
![]() $Re_{D}=3.58\times 10^{4}$
,
$Re_{D}=3.58\times 10^{4}$
,
![]() $C_{L}$
decreased almost discontinuously and then continued to increase as
$C_{L}$
decreased almost discontinuously and then continued to increase as
![]() $\unicode[STIX]{x1D6FC}$
passed through a small range that depended on
$\unicode[STIX]{x1D6FC}$
passed through a small range that depended on
![]() $Re_{D}$
. This so-called lift crisis has been confirmed in subsequent experiments (Fletcher Reference Fletcher1972; Takayama & Aoki Reference Takayama and Aoki2005). The fluid-dynamical mechanisms active during the lift crisis are not well understood. Swanson (Reference Swanson1961) suggests that the boundary layer on the bottom, or counter-flow side of the cylinder (where the cylinder surface velocity opposes the free-stream velocity), transitions to turbulence when
$Re_{D}$
. This so-called lift crisis has been confirmed in subsequent experiments (Fletcher Reference Fletcher1972; Takayama & Aoki Reference Takayama and Aoki2005). The fluid-dynamical mechanisms active during the lift crisis are not well understood. Swanson (Reference Swanson1961) suggests that the boundary layer on the bottom, or counter-flow side of the cylinder (where the cylinder surface velocity opposes the free-stream velocity), transitions to turbulence when
![]() $C_{L}$
begins to decrease, and reaches a fully developed turbulent state when
$C_{L}$
begins to decrease, and reaches a fully developed turbulent state when
![]() $C_{L}$
is near its local minimum value. This then affects the azimuthal location of separation with subsequent changes in
$C_{L}$
is near its local minimum value. This then affects the azimuthal location of separation with subsequent changes in
![]() $C_{L}$
. While later studies, for example, Fletcher (Reference Fletcher1972), adopt this explanation, there appears to be a dearth of supporting experimental or numerical evidence. We note that the lift crisis, also referred to as the inverse Magnus effect, is also observed in other flows over rotating bluff bodies – for example, flow over a rotating sphere (Kim et al.
Reference Kim, Choi, Park and Yoo2014).
$C_{L}$
. While later studies, for example, Fletcher (Reference Fletcher1972), adopt this explanation, there appears to be a dearth of supporting experimental or numerical evidence. We note that the lift crisis, also referred to as the inverse Magnus effect, is also observed in other flows over rotating bluff bodies – for example, flow over a rotating sphere (Kim et al.
Reference Kim, Choi, Park and Yoo2014).
For the flow over a rotating cylinder, numerical simulation has been focused on low
![]() $Re_{D}$
, emphasizing flow instability or vortex shedding at different
$Re_{D}$
, emphasizing flow instability or vortex shedding at different
![]() $\unicode[STIX]{x1D6FC}$
. The direct-numerical simulation (DNS) study of Mittal & Kumar (Reference Mittal and Kumar2003) covers
$\unicode[STIX]{x1D6FC}$
. The direct-numerical simulation (DNS) study of Mittal & Kumar (Reference Mittal and Kumar2003) covers
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 5$
at
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 5$
at
![]() $Re_{D}=200$
. Vortex shedding is found to shrink with increasing
$Re_{D}=200$
. Vortex shedding is found to shrink with increasing
![]() $\unicode[STIX]{x1D6FC}$
, disappearing for
$\unicode[STIX]{x1D6FC}$
, disappearing for
![]() $\unicode[STIX]{x1D6FC}>1.91$
. Another range of one-sided vortex shedding, for
$\unicode[STIX]{x1D6FC}>1.91$
. Another range of one-sided vortex shedding, for
![]() $4.34<\unicode[STIX]{x1D6FC}<4.8$
, was also found. Similarly, DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
$4.34<\unicode[STIX]{x1D6FC}<4.8$
, was also found. Similarly, DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
![]() $Re_{D}=5000$
also finds two vortex shedding ranges. For all cases studied by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015), turbulent transition occurs in the wake flow and no lift crisis is observed, presumably because
$Re_{D}=5000$
also finds two vortex shedding ranges. For all cases studied by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015), turbulent transition occurs in the wake flow and no lift crisis is observed, presumably because
![]() $Re_{D}$
is below Swanson’s critical value
$Re_{D}$
is below Swanson’s critical value
![]() $Re_{D}=3.58\times 10^{4}$
. The main goal of the present research is to use wall-bounded LES, following Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017, Reference Cheng, Pullin and Samtaney2018), to investigate the detailed flow physics of the lift crisis at
$Re_{D}=3.58\times 10^{4}$
. The main goal of the present research is to use wall-bounded LES, following Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017, Reference Cheng, Pullin and Samtaney2018), to investigate the detailed flow physics of the lift crisis at
![]() $Re_{D}$
above this value.
$Re_{D}$
above this value.
In what follows, the numerical LES method, physical model and the parameter cases considered are described in § 2. In § 3 we consider LES of flows at
![]() $Re_{D}=6\times 10^{4}$
with varying
$Re_{D}=6\times 10^{4}$
with varying
![]() $\unicode[STIX]{x1D6FC}$
, showing some surface mean-flow properties such as pressure and skin-friction coefficients. Some LES at relatively low
$\unicode[STIX]{x1D6FC}$
, showing some surface mean-flow properties such as pressure and skin-friction coefficients. Some LES at relatively low
![]() $Re_{D}=5\times 10^{3}$
are also described as reference flows that do not exhibit the lift crisis. Integrals of wall-surface pressure and skin friction, mainly
$Re_{D}=5\times 10^{3}$
are also described as reference flows that do not exhibit the lift crisis. Integrals of wall-surface pressure and skin friction, mainly
![]() $C_{L}$
and the drag coefficient
$C_{L}$
and the drag coefficient
![]() $C_{D}$
, are compared with existing experimental data, and it is shown that the LES produces a lift crisis at
$C_{D}$
, are compared with existing experimental data, and it is shown that the LES produces a lift crisis at
![]() $Re_{D}=6\times 10^{4}$
. In §§ 4 and 5 we utilize diagnostics such as instantaneous surface skin-friction lines, and spanwise-averaged streamlines to elucidate its dynamical underpinnings. This leads to a hypothesis that the lift crisis is the result of flow reorganization characterized by the aggregation of scattered small-scale separation/reattachment cells on the leeward side of the cylinder surface, into a narrow, spanwise band, accompanied by the formation of a corresponding low-pressure spike. Discussion of the flow induced by the rotating cylinder, including incipient turbulence transition behaviour and also the relation between the present lift crisis and the drag crisis in flow over a static cylinder, is given in § 6. Concluding remarks are provided in § 7.
$Re_{D}=6\times 10^{4}$
. In §§ 4 and 5 we utilize diagnostics such as instantaneous surface skin-friction lines, and spanwise-averaged streamlines to elucidate its dynamical underpinnings. This leads to a hypothesis that the lift crisis is the result of flow reorganization characterized by the aggregation of scattered small-scale separation/reattachment cells on the leeward side of the cylinder surface, into a narrow, spanwise band, accompanied by the formation of a corresponding low-pressure spike. Discussion of the flow induced by the rotating cylinder, including incipient turbulence transition behaviour and also the relation between the present lift crisis and the drag crisis in flow over a static cylinder, is given in § 6. Concluding remarks are provided in § 7.
2 Description of LES and cases set-up
2.1 LES framework: numerical method and physical model
The governing equations for LES of incompressible viscous flow are derived by formally applying a spatial filter on the Navier–Stokes equations. These are
Here
![]() $x_{i}$
,
$x_{i}$
,
![]() $i=1,2,3$
are Cartesian coordinates with
$i=1,2,3$
are Cartesian coordinates with
![]() $\widetilde{u_{i}}$
the corresponding filtered velocity and
$\widetilde{u_{i}}$
the corresponding filtered velocity and
![]() $\widetilde{p}$
the filtered pressure.
$\widetilde{p}$
the filtered pressure.
![]() $T_{ij}=\widetilde{u_{i}u_{j}}-\widetilde{u_{i}}\widetilde{u_{j}}$
denotes the effect of unresolved scales on the resolved-scale motion, which is modelled via a sub-grid scale (SGS) model.
$T_{ij}=\widetilde{u_{i}u_{j}}-\widetilde{u_{i}}\widetilde{u_{j}}$
denotes the effect of unresolved scales on the resolved-scale motion, which is modelled via a sub-grid scale (SGS) model.
It is convenient sometimes to use
![]() $(x,y,z)$
as Cartesian coordinates with
$(x,y,z)$
as Cartesian coordinates with
![]() $(u,v,w)$
as the corresponding filtered velocity components. Two other coordinate systems are also utilized: the first is cylindrical coordinates (
$(u,v,w)$
as the corresponding filtered velocity components. Two other coordinate systems are also utilized: the first is cylindrical coordinates (
![]() $\unicode[STIX]{x1D703},y,r$
) with velocity components (
$\unicode[STIX]{x1D703},y,r$
) with velocity components (
![]() $u_{\unicode[STIX]{x1D703}},u_{y},u_{r}$
), which is useful for analysing results, while the second is general curvilinear coordinates
$u_{\unicode[STIX]{x1D703}},u_{y},u_{r}$
), which is useful for analysing results, while the second is general curvilinear coordinates
![]() $(\unicode[STIX]{x1D709},y,\unicode[STIX]{x1D702})$
, used for the implementation of the numerical method.
$(\unicode[STIX]{x1D709},y,\unicode[STIX]{x1D702})$
, used for the implementation of the numerical method.
The governing LES equations are discretized on a body-fitted computational domain
![]() $(\unicode[STIX]{x1D709},y,\unicode[STIX]{x1D702})$
which is mapped from the physical domain in
$(\unicode[STIX]{x1D709},y,\unicode[STIX]{x1D702})$
which is mapped from the physical domain in
![]() $(x,y,z)$
coordinates. Discretization employs a fourth-order-accurate, central-difference scheme for all terms, except the convective term, which uses a fourth-order energy-conservative scheme for the skew-symmetric form (Morinishi et al.
Reference Morinishi, Lund, Vasilyev and Moin1998). For time integration, a third-order Runge–Kutta scheme is used, combined with the fractional-step method. The modified Helmholtz equation for velocity and the Poisson equation for pressure are solved with a multigrid method with line-relaxed Gauss–Seidel smoothers. The code is described in detail by Zhang et al. (Reference Zhang, Cheng, Gao, Qamar and Samtaney2015), Zhang & Samtaney (Reference Zhang and Samtaney2016) for DNS of airfoil flow and by Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017, Reference Cheng, Pullin and Samtaney2018) for LES of flow past non-rotating cylinder. The present implementation is identical to that described in § 2.1 of Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017), where outer boundary conditions and use of a sponge region to control the effect of the far wake on the near-cylinder flow are described. All LES reported here use a span-diameter ratio
$(x,y,z)$
coordinates. Discretization employs a fourth-order-accurate, central-difference scheme for all terms, except the convective term, which uses a fourth-order energy-conservative scheme for the skew-symmetric form (Morinishi et al.
Reference Morinishi, Lund, Vasilyev and Moin1998). For time integration, a third-order Runge–Kutta scheme is used, combined with the fractional-step method. The modified Helmholtz equation for velocity and the Poisson equation for pressure are solved with a multigrid method with line-relaxed Gauss–Seidel smoothers. The code is described in detail by Zhang et al. (Reference Zhang, Cheng, Gao, Qamar and Samtaney2015), Zhang & Samtaney (Reference Zhang and Samtaney2016) for DNS of airfoil flow and by Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017, Reference Cheng, Pullin and Samtaney2018) for LES of flow past non-rotating cylinder. The present implementation is identical to that described in § 2.1 of Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017), where outer boundary conditions and use of a sponge region to control the effect of the far wake on the near-cylinder flow are described. All LES reported here use a span-diameter ratio
![]() $L_{y}/D=3$
and an O grid of radius of
$L_{y}/D=3$
and an O grid of radius of
![]() $L_{r}=40D$
. The O grid is a cylinder fitted with equal grid spacing in both the azimuthal and spanwise directions but with grid stretching in the radial direction, particularly near the cylinder surface. The no-slip boundary condition at a stationary cylinder surface is here replaced by
$L_{r}=40D$
. The O grid is a cylinder fitted with equal grid spacing in both the azimuthal and spanwise directions but with grid stretching in the radial direction, particularly near the cylinder surface. The no-slip boundary condition at a stationary cylinder surface is here replaced by
![]() $(u_{\unicode[STIX]{x1D703}},u_{y},u_{r})=(\unicode[STIX]{x1D6FC}D/2,0,0)$
at the cylinder surface.
$(u_{\unicode[STIX]{x1D703}},u_{y},u_{r})=(\unicode[STIX]{x1D6FC}D/2,0,0)$
at the cylinder surface.
For LES we employ the stretched-vortex SGS model (SVM) (Misra & Pullin Reference Misra and Pullin1997; Voelkl, Pullin & Chan Reference Voelkl, Pullin and Chan2000; Chung & Pullin Reference Chung and Pullin2009), where the subgrid flow is modelled by spiral vortices (Lundgren Reference Lundgren1982) stretched by the eddies comprising the local resolved-scale flow. The implementation of the SVM is described in detail in § 2.2 of Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017, Reference Cheng, Pullin and Samtaney2018) and summarized presently in appendix B. The surface skin friction is obtained using a one-sided, four-point stencil defined by their equation (2.12) after first subtracting the solid-body rotation field with azimuthal speed
![]() $\unicode[STIX]{x1D6FC}r$
. The present LES is wall-resolved, meaning that the wall-normal grid size at the wall is on the order of the local viscous wall scale
$\unicode[STIX]{x1D6FC}r$
. The present LES is wall-resolved, meaning that the wall-normal grid size at the wall is on the order of the local viscous wall scale
![]() $u_{\unicode[STIX]{x1D70F}}/\unicode[STIX]{x1D708}$
, where
$u_{\unicode[STIX]{x1D70F}}/\unicode[STIX]{x1D708}$
, where
![]() $u_{\unicode[STIX]{x1D70F}}\equiv \sqrt{|\unicode[STIX]{x1D749}_{w}|/\unicode[STIX]{x1D70C}}$
is the friction velocity with
$u_{\unicode[STIX]{x1D70F}}\equiv \sqrt{|\unicode[STIX]{x1D749}_{w}|/\unicode[STIX]{x1D70C}}$
is the friction velocity with
![]() $|\unicode[STIX]{x1D749}_{w}|$
the magnitude of the wall shear stress and
$|\unicode[STIX]{x1D749}_{w}|$
the magnitude of the wall shear stress and
![]() $\unicode[STIX]{x1D70C}$
the constant fluid density. See Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) for further details and also tables 1 and 2 of this paper.
$\unicode[STIX]{x1D70C}$
the constant fluid density. See Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) for further details and also tables 1 and 2 of this paper.
2.2 LES performed

Figure 1. Sketch of simulation geometry. (a) 3D view of the flow configuration with
![]() $(x,y,z)$
coordinates; (b) cross-section view of the cylinder with
$(x,y,z)$
coordinates; (b) cross-section view of the cylinder with
![]() $(\unicode[STIX]{x1D703},r)$
coordinates.
$(\unicode[STIX]{x1D703},r)$
coordinates.
![]() $\unicode[STIX]{x1D703}$
is measured in the clockwise direction with
$\unicode[STIX]{x1D703}$
is measured in the clockwise direction with
![]() $\unicode[STIX]{x1D703}=0$
on the most windward point of the cylinder surface.
$\unicode[STIX]{x1D703}=0$
on the most windward point of the cylinder surface.
![]() $U_{\infty }$
is the free-stream speed and
$U_{\infty }$
is the free-stream speed and
![]() $\unicode[STIX]{x1D6FA}$
the (constant) angular velocity of the cylinder. The flow can be described by two dimensionless parameters: Reynolds number
$\unicode[STIX]{x1D6FA}$
the (constant) angular velocity of the cylinder. The flow can be described by two dimensionless parameters: Reynolds number
![]() $Re_{D}=U_{\infty }D/\unicode[STIX]{x1D708}$
and velocity ratio
$Re_{D}=U_{\infty }D/\unicode[STIX]{x1D708}$
and velocity ratio
![]() $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FA}D/(2U_{\infty })$
, where
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FA}D/(2U_{\infty })$
, where
![]() $\unicode[STIX]{x1D708}$
is the kinematic viscosity.
$\unicode[STIX]{x1D708}$
is the kinematic viscosity.
Table 1. LES flows with
![]() $Re_{D}=6\times 10^{4}$
and varying
$Re_{D}=6\times 10^{4}$
and varying
![]() $\unicode[STIX]{x1D6FC}$
. For all cases, the domain size is
$\unicode[STIX]{x1D6FC}$
. For all cases, the domain size is
![]() $40D$
in the radial
$40D$
in the radial
![]() $r$
direction and
$r$
direction and
![]() $3D$
in the spanwise
$3D$
in the spanwise
![]() $y$
direction.
$y$
direction.

Table 2. LES flows with
![]() $Re_{D}=5\times 10^{3}$
and varying
$Re_{D}=5\times 10^{3}$
and varying
![]() $\unicode[STIX]{x1D6FC}$
. For all cases, the domain size is
$\unicode[STIX]{x1D6FC}$
. For all cases, the domain size is
![]() $40D$
in the radial
$40D$
in the radial
![]() $r$
direction and
$r$
direction and
![]() $3D$
in the spanwise
$3D$
in the spanwise
![]() $y$
direction.
$y$
direction.

Table 3. Summary of experimental data discussed in the present study.

The cylinder geometry is depicted in figure 1. In
![]() $(x,y,z)$
coordinates the free-stream velocity vector is in the positive
$(x,y,z)$
coordinates the free-stream velocity vector is in the positive
![]() $x$
-direction while the cylinder axis is aligned with the
$x$
-direction while the cylinder axis is aligned with the
![]() $y$
-axis. The cylinder rotates with dimensional angular velocity
$y$
-axis. The cylinder rotates with dimensional angular velocity
![]() $\unicode[STIX]{x1D6FA}$
in the sense of the right-hand screw rule. The azimuthal angle
$\unicode[STIX]{x1D6FA}$
in the sense of the right-hand screw rule. The azimuthal angle
![]() $\unicode[STIX]{x1D703}$
is as shown. Here and subsequently we refer to that part of the cylinder surface instantaneously lying in
$\unicode[STIX]{x1D703}$
is as shown. Here and subsequently we refer to that part of the cylinder surface instantaneously lying in
![]() $0\leqslant \unicode[STIX]{x1D703}\leqslant 180^{\circ }$
(
$0\leqslant \unicode[STIX]{x1D703}\leqslant 180^{\circ }$
(
![]() $z\geqslant 0$
), where the
$z\geqslant 0$
), where the
![]() $x$
-component of the cylinder surface speed is in the direction of the free stream as the ‘top’ or advancing part of the cylinder and that part instantaneously in
$x$
-component of the cylinder surface speed is in the direction of the free stream as the ‘top’ or advancing part of the cylinder and that part instantaneously in
![]() $180^{\circ }\leqslant \unicode[STIX]{x1D703}<360^{\circ }$
(
$180^{\circ }\leqslant \unicode[STIX]{x1D703}<360^{\circ }$
(
![]() $z<0$
), where the
$z<0$
), where the
![]() $x$
-component of the cylinder surface speed opposes the free stream as the ‘bottom’ or retreating portion of the cylinder surface.
$x$
-component of the cylinder surface speed opposes the free stream as the ‘bottom’ or retreating portion of the cylinder surface.
A summary of both experimental and simulation data from reference studies is shown in table 3, with
![]() $Re_{D}\leqslant 1.28\times 10^{5}$
. For
$Re_{D}\leqslant 1.28\times 10^{5}$
. For
![]() $Re_{D}=5\times 10^{3}$
, DNS results (Aljure et al.
Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) show that
$Re_{D}=5\times 10^{3}$
, DNS results (Aljure et al.
Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) show that
![]() $C_{L}$
increases monotonically in the range
$C_{L}$
increases monotonically in the range
![]() $0<\unicode[STIX]{x1D6FC}<5$
. In experiments by Swanson (Reference Swanson1961), for flows with
$0<\unicode[STIX]{x1D6FC}<5$
. In experiments by Swanson (Reference Swanson1961), for flows with
![]() $3.58\times 10^{4}<Re_{D}<4.9\times 10^{4}$
, the variation of
$3.58\times 10^{4}<Re_{D}<4.9\times 10^{4}$
, the variation of
![]() $C_{L}$
with
$C_{L}$
with
![]() $\unicode[STIX]{x1D6FC}$
at fixed
$\unicode[STIX]{x1D6FC}$
at fixed
![]() $Re_{D}$
does not exhibit a discernible crisis in the sense of a sudden change over a small range of
$Re_{D}$
does not exhibit a discernible crisis in the sense of a sudden change over a small range of
![]() $\unicode[STIX]{x1D6FC}$
. For flows with higher
$\unicode[STIX]{x1D6FC}$
. For flows with higher
![]() $Re_{D}$
,
$Re_{D}$
,
![]() $C_{L}(\unicode[STIX]{x1D6FC})$
clearly shows lift crisis behaviour (Swanson Reference Swanson1961; Takayama & Aoki Reference Takayama and Aoki2005). The experimental data of Takayama & Aoki (Reference Takayama and Aoki2005) show
$C_{L}(\unicode[STIX]{x1D6FC})$
clearly shows lift crisis behaviour (Swanson Reference Swanson1961; Takayama & Aoki Reference Takayama and Aoki2005). The experimental data of Takayama & Aoki (Reference Takayama and Aoki2005) show
![]() $C_{L}(\unicode[STIX]{x1D6FC})$
making zero crossings and becoming negative at approximately
$C_{L}(\unicode[STIX]{x1D6FC})$
making zero crossings and becoming negative at approximately
![]() $Re_{D}\approx 1.0\times 10^{5}$
while the data of Swanson (Reference Swanson1961) shows this at
$Re_{D}\approx 1.0\times 10^{5}$
while the data of Swanson (Reference Swanson1961) shows this at
![]() $Re_{D}\approx 1.28\times 10^{5}$
. Based on the above summary, we choose
$Re_{D}\approx 1.28\times 10^{5}$
. Based on the above summary, we choose
![]() $Re_{D}=5\times 10^{3}$
as a starting verification case and also to provide a reference flow behaviour with laminar boundary layers on the cylinder surface. For our main LES we choose
$Re_{D}=5\times 10^{3}$
as a starting verification case and also to provide a reference flow behaviour with laminar boundary layers on the cylinder surface. For our main LES we choose
![]() $Re_{D}=6\times 10^{4}$
, where the lift crisis has been demonstrated experimentally (Swanson Reference Swanson1961; Takayama & Aoki Reference Takayama and Aoki2005). Most of our LES utilize
$Re_{D}=6\times 10^{4}$
, where the lift crisis has been demonstrated experimentally (Swanson Reference Swanson1961; Takayama & Aoki Reference Takayama and Aoki2005). Most of our LES utilize
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
.
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
.
Numerical code verification is described in appendix A. This includes comparison with DNS results at
![]() $Re_{D}=5\times 10^{3}$
, analysis of the wall-adjacent mesh size in wall units, examination of the two-point correlation of the spanwise velocity component for evaluating the sufficiency of the chosen spanwise domain, and a mesh refinement study at
$Re_{D}=5\times 10^{3}$
, analysis of the wall-adjacent mesh size in wall units, examination of the two-point correlation of the spanwise velocity component for evaluating the sufficiency of the chosen spanwise domain, and a mesh refinement study at
![]() $Re_{D}=6\times 10^{4}$
for
$Re_{D}=6\times 10^{4}$
for
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
. Unless otherwise specified, the ‘average’ or ‘mean’ is defined as a combined spanwise and time average. The averaging procedure begins after some initial transient period, and spans a time window of
$\unicode[STIX]{x1D6FC}=0.6$
. Unless otherwise specified, the ‘average’ or ‘mean’ is defined as a combined spanwise and time average. The averaging procedure begins after some initial transient period, and spans a time window of
![]() $250$
units of
$250$
units of
![]() $D/U_{\infty }$
for
$D/U_{\infty }$
for
![]() $Re_{D}=3.9\times 10^{3}$
and approximately
$Re_{D}=3.9\times 10^{3}$
and approximately
![]() $60$
times
$60$
times
![]() $D/U_{\infty }$
for
$D/U_{\infty }$
for
![]() $Re_{D}=6\times 10^{4}$
.
$Re_{D}=6\times 10^{4}$
.
3 Cylinder-wall profiles for
 $Re_{D}=6\times 10^{4}$
and
$Re_{D}=6\times 10^{4}$
and
 $5\times 10^{3}$
$5\times 10^{3}$

Figure 2.
![]() $Re_{D}=6\times 10^{4}$
. (a,c,e,g) Pressure coefficient
$Re_{D}=6\times 10^{4}$
. (a,c,e,g) Pressure coefficient
![]() $C_{p}$
. (b,d,f,h) Azimuthal component of the skin-friction coefficient
$C_{p}$
. (b,d,f,h) Azimuthal component of the skin-friction coefficient
![]() $C_{f\unicode[STIX]{x1D703}}$
. In (a,b): ——,
$C_{f\unicode[STIX]{x1D703}}$
. In (a,b): ——,
![]() $\unicode[STIX]{x1D6FC}=0$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.2$
; – – – –,
$\unicode[STIX]{x1D6FC}=0.2$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.48$
. In (c,d): – – – –,
$\unicode[STIX]{x1D6FC}=0.48$
. In (c,d): – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.48$
, — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.48$
, — ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.52$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.52$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.56$
; ——,
$\unicode[STIX]{x1D6FC}=0.56$
; ——,
![]() $\unicode[STIX]{x1D6FC}=0.6$
. In (e,f): ——,
$\unicode[STIX]{x1D6FC}=0.6$
. In (e,f): ——,
![]() $\unicode[STIX]{x1D6FC}=0.6$
; – – – –,
$\unicode[STIX]{x1D6FC}=0.6$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.68$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.68$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.8$
; — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.8$
; — ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=1.0$
. In (g,h): — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=1.0$
. In (g,h): — ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=1.0$
; ——,
$\unicode[STIX]{x1D6FC}=1.0$
; ——,
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
3.1
 $Re_{D}=6\times 10^{4}$
: pressure coefficient
$Re_{D}=6\times 10^{4}$
: pressure coefficient
 $C_{p}$
and skin-friction coefficient
$C_{p}$
and skin-friction coefficient
 $C_{f\unicode[STIX]{x1D703}}$
$C_{f\unicode[STIX]{x1D703}}$
The LES performed are summarized in table 1. Figure 2 shows azimuthal profiles of the mean surface pressure coefficient
![]() $C_{p}=2(p-p_{\infty })/(\unicode[STIX]{x1D70C}U_{\infty }^{2})$
(panels a,c,e,g) and the
$C_{p}=2(p-p_{\infty })/(\unicode[STIX]{x1D70C}U_{\infty }^{2})$
(panels a,c,e,g) and the
![]() $\unicode[STIX]{x1D703}$
component of the mean surface vector skin-friction coefficient
$\unicode[STIX]{x1D703}$
component of the mean surface vector skin-friction coefficient
![]() $C_{f\unicode[STIX]{x1D703}}=2\unicode[STIX]{x1D70F}_{w,\unicode[STIX]{x1D703}}/(\unicode[STIX]{x1D70C}U_{\infty }^{2})$
(panels b,d,f,h) for
$C_{f\unicode[STIX]{x1D703}}=2\unicode[STIX]{x1D70F}_{w,\unicode[STIX]{x1D703}}/(\unicode[STIX]{x1D70C}U_{\infty }^{2})$
(panels b,d,f,h) for
![]() $Re_{D}=6\times 10^{4}$
over a range of
$Re_{D}=6\times 10^{4}$
over a range of
![]() $\unicode[STIX]{x1D6FC}$
chosen to capture the expected lift crisis. Here
$\unicode[STIX]{x1D6FC}$
chosen to capture the expected lift crisis. Here
![]() $p_{\infty }$
is the free-stream pressure which is used to make sure
$p_{\infty }$
is the free-stream pressure which is used to make sure
![]() $C_{p}=1$
at the front stagnation point. In figure 2(a),
$C_{p}=1$
at the front stagnation point. In figure 2(a),
![]() $C_{p}$
at
$C_{p}$
at
![]() $\unicode[STIX]{x1D6FC}=0$
shows symmetric behaviour for the top and bottom parts of the cylinder. As
$\unicode[STIX]{x1D6FC}=0$
shows symmetric behaviour for the top and bottom parts of the cylinder. As
![]() $\unicode[STIX]{x1D6FC}$
increases, the azimuthal symmetry about
$\unicode[STIX]{x1D6FC}$
increases, the azimuthal symmetry about
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
is broken. At
$\unicode[STIX]{x1D703}=180^{\circ }$
is broken. At
![]() $\unicode[STIX]{x1D6FC}=0.2$
, the local minimum
$\unicode[STIX]{x1D6FC}=0.2$
, the local minimum
![]() $C_{p}$
for the top portion of the cylinder decreases while the local minimum value on the bottom part of the cylinder increases. This is a natural tendency owing to momentum conservation and should exist for all positive
$C_{p}$
for the top portion of the cylinder decreases while the local minimum value on the bottom part of the cylinder increases. This is a natural tendency owing to momentum conservation and should exist for all positive
![]() $\unicode[STIX]{x1D6FC}$
cases. Similar behaviour is observed for
$\unicode[STIX]{x1D6FC}$
cases. Similar behaviour is observed for
![]() $C_{p}$
at
$C_{p}$
at
![]() $\unicode[STIX]{x1D6FC}=0.48$
where the local minimum value on the bottom side of the cylinder has disappeared.
$\unicode[STIX]{x1D6FC}=0.48$
where the local minimum value on the bottom side of the cylinder has disappeared.
Further increase in
![]() $\unicode[STIX]{x1D6FC}$
shows a quite different trend, as for example, seen in figure 2(c). For
$\unicode[STIX]{x1D6FC}$
shows a quite different trend, as for example, seen in figure 2(c). For
![]() $\unicode[STIX]{x1D6FC}=0.52$
, a plateau value for
$\unicode[STIX]{x1D6FC}=0.52$
, a plateau value for
![]() $C_{p}$
has formed which persists with increasing
$C_{p}$
has formed which persists with increasing
![]() $C_{p}$
up to
$C_{p}$
up to
![]() $\unicode[STIX]{x1D6FC}=0.56$
. At
$\unicode[STIX]{x1D6FC}=0.56$
. At
![]() $\unicode[STIX]{x1D6FC}=0.56$
the local minimum on the bottom side has reappeared with a more pronounced negative minimum value compared to the original value at
$\unicode[STIX]{x1D6FC}=0.56$
the local minimum on the bottom side has reappeared with a more pronounced negative minimum value compared to the original value at
![]() $\unicode[STIX]{x1D6FC}=0$
. As
$\unicode[STIX]{x1D6FC}=0$
. As
![]() $\unicode[STIX]{x1D6FC}$
increases further to
$\unicode[STIX]{x1D6FC}$
increases further to
![]() $\unicode[STIX]{x1D6FC}=0.6$
, there is little variation in the
$\unicode[STIX]{x1D6FC}=0.6$
, there is little variation in the
![]() $C_{p}$
profile on the top side of the cylinder, while for the bottom side, a yet deeper valley appears with an even more negative minimum
$C_{p}$
profile on the top side of the cylinder, while for the bottom side, a yet deeper valley appears with an even more negative minimum
![]() $C_{p}$
. For larger
$C_{p}$
. For larger
![]() $\unicode[STIX]{x1D6FC}=0.68,0.8,1.0$
shown in figure 2(e), the minimum
$\unicode[STIX]{x1D6FC}=0.68,0.8,1.0$
shown in figure 2(e), the minimum
![]() $C_{p}$
on the top side of the cylinder becomes more negative as
$C_{p}$
on the top side of the cylinder becomes more negative as
![]() $\unicode[STIX]{x1D6FC}$
increases while the
$\unicode[STIX]{x1D6FC}$
increases while the
![]() $C_{p}$
minimum on the bottom increases algebraically. The plateau value of
$C_{p}$
minimum on the bottom increases algebraically. The plateau value of
![]() $C_{p}\approx -0.7$
remains almost unchanged in this range of
$C_{p}\approx -0.7$
remains almost unchanged in this range of
![]() $\unicode[STIX]{x1D6FC}$
. The overall effect is that
$\unicode[STIX]{x1D6FC}$
. The overall effect is that
![]() $C_{L}$
again increases with increasing
$C_{L}$
again increases with increasing
![]() $\unicode[STIX]{x1D6FC}$
. At
$\unicode[STIX]{x1D6FC}$
. At
![]() $\unicode[STIX]{x1D6FC}=2$
in figure 2(g) the top-side minimum
$\unicode[STIX]{x1D6FC}=2$
in figure 2(g) the top-side minimum
![]() $C_{p}$
is strongly negative. A
$C_{p}$
is strongly negative. A
![]() $C_{p}$
plateau still exists but is increased in
$C_{p}$
plateau still exists but is increased in
![]() $C_{p}$
value and shifted azimuthally towards the bottom surface of the cylinder. There is no local
$C_{p}$
value and shifted azimuthally towards the bottom surface of the cylinder. There is no local
![]() $C_{p}$
minimum on the bottom side.
$C_{p}$
minimum on the bottom side.
At
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $C_{f\unicode[STIX]{x1D703}}$
is symmetric about
$C_{f\unicode[STIX]{x1D703}}$
is symmetric about
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
and a small secondary-separation bubble can be observed, as shown in figure 2(b). When the cylinder is rotating, the secondary-separation bubble on the top side disappears. For
$\unicode[STIX]{x1D703}=180^{\circ }$
and a small secondary-separation bubble can be observed, as shown in figure 2(b). When the cylinder is rotating, the secondary-separation bubble on the top side disappears. For
![]() $\unicode[STIX]{x1D6FC}>0.2$
, the whole leeward side of the cylinder has a negative
$\unicode[STIX]{x1D6FC}>0.2$
, the whole leeward side of the cylinder has a negative
![]() $C_{f\unicode[STIX]{x1D703}}$
plateau, where a negative value means that the skin-friction force corresponds to a thrust. When
$C_{f\unicode[STIX]{x1D703}}$
plateau, where a negative value means that the skin-friction force corresponds to a thrust. When
![]() $\unicode[STIX]{x1D6FC}=0.48$
, this plateau further moves to a lower level at approximately
$\unicode[STIX]{x1D6FC}=0.48$
, this plateau further moves to a lower level at approximately
![]() $C_{f\unicode[STIX]{x1D703}}=0.004$
. For
$C_{f\unicode[STIX]{x1D703}}=0.004$
. For
![]() $\unicode[STIX]{x1D6FC}=0.48{-}0.6$
in figure 2(d), the plateau
$\unicode[STIX]{x1D6FC}=0.48{-}0.6$
in figure 2(d), the plateau
![]() $C_{f\unicode[STIX]{x1D703}}$
remains almost constant. At
$C_{f\unicode[STIX]{x1D703}}$
remains almost constant. At
![]() $\unicode[STIX]{x1D6FC}=0.6$
, an abrupt hill appears at approximately
$\unicode[STIX]{x1D6FC}=0.6$
, an abrupt hill appears at approximately
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
and touches
$\unicode[STIX]{x1D703}=270^{\circ }$
and touches
![]() $C_{f\unicode[STIX]{x1D703}}=0$
. This implies the incipient appearance of a tiny mean-flow separation region. In figure 2(f), further increase of
$C_{f\unicode[STIX]{x1D703}}=0$
. This implies the incipient appearance of a tiny mean-flow separation region. In figure 2(f), further increase of
![]() $\unicode[STIX]{x1D6FC}$
shows further decrease of the plateau
$\unicode[STIX]{x1D6FC}$
shows further decrease of the plateau
![]() $C_{f\unicode[STIX]{x1D703}}$
value and also a smoothing of the hill. Still further increase to
$C_{f\unicode[STIX]{x1D703}}$
value and also a smoothing of the hill. Still further increase to
![]() $\unicode[STIX]{x1D6FC}=2.0$
, as shown in figure 2(h), results in a plateau at a much smaller
$\unicode[STIX]{x1D6FC}=2.0$
, as shown in figure 2(h), results in a plateau at a much smaller
![]() $C_{f\unicode[STIX]{x1D703}}$
.
$C_{f\unicode[STIX]{x1D703}}$
.
3.2
 $Re_{D}=5\times 10^{3}$
$Re_{D}=5\times 10^{3}$

Figure 3.
![]() $C_{p}$
and
$C_{p}$
and
![]() $C_{f\unicode[STIX]{x1D703}}$
at
$C_{f\unicode[STIX]{x1D703}}$
at
![]() $Re_{D}=5\times 10^{3}$
. (a,c)
$Re_{D}=5\times 10^{3}$
. (a,c)
![]() $C_{p}$
; (b,d)
$C_{p}$
; (b,d)
![]() $C_{f\unicode[STIX]{x1D703}}$
. In (a,b): ——,
$C_{f\unicode[STIX]{x1D703}}$
. In (a,b): ——,
![]() $\unicode[STIX]{x1D6FC}=0$
; – – – –,
$\unicode[STIX]{x1D6FC}=0$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.2$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.2$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.4$
; — — — —,
$\unicode[STIX]{x1D6FC}=0.4$
; — — — —,
![]() $\unicode[STIX]{x1D6FC}=0.6$
. In (c,d): — — — —,
$\unicode[STIX]{x1D6FC}=0.6$
. In (c,d): — — — —,
![]() $\unicode[STIX]{x1D6FC}=0.6$
;
— ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.6$
;
— ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.8$
; ——,
$\unicode[STIX]{x1D6FC}=0.8$
; ——,
![]() $\unicode[STIX]{x1D6FC}=1.0$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=1.0$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
We discuss LES results at
![]() $Re_{D}=5\times 10^{3}$
as a reference. The LES performed are summarized in table 2. For
$Re_{D}=5\times 10^{3}$
as a reference. The LES performed are summarized in table 2. For
![]() $Re_{D}=5\times 10^{3}$
, the variation of both
$Re_{D}=5\times 10^{3}$
, the variation of both
![]() $C_{p}$
and
$C_{p}$
and
![]() $C_{f\unicode[STIX]{x1D703}}$
with increasing
$C_{f\unicode[STIX]{x1D703}}$
with increasing
![]() $\unicode[STIX]{x1D6FC}$
shows an almost uniform tendency in figure 3. This includes the decreasing minimum
$\unicode[STIX]{x1D6FC}$
shows an almost uniform tendency in figure 3. This includes the decreasing minimum
![]() $C_{p}$
on the top side of the cylinder and the gradual disappearance of the minimum
$C_{p}$
on the top side of the cylinder and the gradual disappearance of the minimum
![]() $C_{p}$
on the bottom side. The plateau
$C_{p}$
on the bottom side. The plateau
![]() $C_{p}$
remains almost unchanged for
$C_{p}$
remains almost unchanged for
![]() $\unicode[STIX]{x1D6FC}<1$
. The trend of
$\unicode[STIX]{x1D6FC}<1$
. The trend of
![]() $C_{f\unicode[STIX]{x1D703}}$
variation is similarly uniform, with a monotonically decreasing plateau value.
$C_{f\unicode[STIX]{x1D703}}$
variation is similarly uniform, with a monotonically decreasing plateau value.
It is of interest to compare cases with different
![]() $Re_{D}$
at fixed
$Re_{D}$
at fixed
![]() $\unicode[STIX]{x1D6FC}$
, for example
$\unicode[STIX]{x1D6FC}$
, for example
![]() $\unicode[STIX]{x1D6FC}=2$
in figures 2(g,h) and 3(c,d), respectively. Although profiles of
$\unicode[STIX]{x1D6FC}=2$
in figures 2(g,h) and 3(c,d), respectively. Although profiles of
![]() $C_{f\unicode[STIX]{x1D703}}$
are rather different, the azimuthal profiles of pressure coefficient are quite close. Both cases have a minimum
$C_{f\unicode[STIX]{x1D703}}$
are rather different, the azimuthal profiles of pressure coefficient are quite close. Both cases have a minimum
![]() $C_{p}\approx -6.5$
near
$C_{p}\approx -6.5$
near
![]() $\unicode[STIX]{x1D703}=90^{\circ }$
, and also a peak value
$\unicode[STIX]{x1D703}=90^{\circ }$
, and also a peak value
![]() $C_{p}\approx 1$
at approximately
$C_{p}\approx 1$
at approximately
![]() $\unicode[STIX]{x1D703}=330^{\circ }$
. Since the pressure distribution on the cylinder surface contributes the dominant part of both the drag and the lift, the dependence of the drag/lift on
$\unicode[STIX]{x1D703}=330^{\circ }$
. Since the pressure distribution on the cylinder surface contributes the dominant part of both the drag and the lift, the dependence of the drag/lift on
![]() $Re_{D}$
is quite weak for high-
$Re_{D}$
is quite weak for high-
![]() $\unicode[STIX]{x1D6FC}$
cases.
$\unicode[STIX]{x1D6FC}$
cases.
3.3 Lift and drag coefficients
 $C_{L}$
,
$C_{L}$
,
 $C_{D}$
$C_{D}$
It has been seen that the
![]() $C_{p}$
profiles for
$C_{p}$
profiles for
![]() $Re_{D}=6\times 10^{4}$
in
$Re_{D}=6\times 10^{4}$
in
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
show rather different features than for
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
show rather different features than for
![]() $Re_{D}=5\times 10^{3}$
. These account for the presence of the
$Re_{D}=5\times 10^{3}$
. These account for the presence of the
![]() $C_{L}$
crisis for the higher
$C_{L}$
crisis for the higher
![]() $Re_{D}$
and for smaller differences in the
$Re_{D}$
and for smaller differences in the
![]() $C_{D}-\unicode[STIX]{x1D6FC}$
variation for the two
$C_{D}-\unicode[STIX]{x1D6FC}$
variation for the two
![]() $Re_{D}$
.
$Re_{D}$
.
3.3.1 Lift crisis of
 $C_{L}(Re_{D},\unicode[STIX]{x1D6FC})$
$C_{L}(Re_{D},\unicode[STIX]{x1D6FC})$

Figure 4. Lift coefficient
![]() $C_{L}$
. (a) full scale; (b) for
$C_{L}$
. (a) full scale; (b) for
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
. Present LES data: ▪,
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
. Present LES data: ▪,
![]() $Re_{D}=5\times 10^{3}$
; ●,
$Re_{D}=5\times 10^{3}$
; ●,
![]() $Re_{D}=6\times 10^{4}$
; ▶,
$Re_{D}=6\times 10^{4}$
; ▶,
![]() $Re_{D}=6\times 10^{4}$
with fine mesh. Reference data: ▵, DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
$Re_{D}=6\times 10^{4}$
with fine mesh. Reference data: ▵, DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
![]() $Re_{D}=5\times 10^{3}$
; ▿, experiments by Swanson (Reference Swanson1961) at
$Re_{D}=5\times 10^{3}$
; ▿, experiments by Swanson (Reference Swanson1961) at
![]() $Re_{D}=6\times 10^{4}$
; ○, experiments by Thom (Reference Thom1934) at
$Re_{D}=6\times 10^{4}$
; ○, experiments by Thom (Reference Thom1934) at
![]() $Re_{D}=5.3\times 10^{3}$
; ▫, experiments by Carstensen et al. (Reference Carstensen, Mandviwalla, Vita and Paulsen2014).
$Re_{D}=5.3\times 10^{3}$
; ▫, experiments by Carstensen et al. (Reference Carstensen, Mandviwalla, Vita and Paulsen2014).
The
![]() $C_{L}(Re_{D},\unicode[STIX]{x1D6FC})$
results for the present LES are shown in figure 4(a) over
$C_{L}(Re_{D},\unicode[STIX]{x1D6FC})$
results for the present LES are shown in figure 4(a) over
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 2$
. Here all LES results for
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 2$
. Here all LES results for
![]() $Re_{D}=5\times 10^{3}$
and
$Re_{D}=5\times 10^{3}$
and
![]() $6\times 10^{4}$
are shown. Also shown are DNS (Aljure et al.
Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
$6\times 10^{4}$
are shown. Also shown are DNS (Aljure et al.
Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
![]() $Re_{D}=5\times 10^{3}$
and experimental measurements (Thom Reference Thom1934; Swanson Reference Swanson1961; Carstensen et al.
Reference Carstensen, Mandviwalla, Vita and Paulsen2014). The present LES at
$Re_{D}=5\times 10^{3}$
and experimental measurements (Thom Reference Thom1934; Swanson Reference Swanson1961; Carstensen et al.
Reference Carstensen, Mandviwalla, Vita and Paulsen2014). The present LES at
![]() $Re_{D}=5\times 10^{3}$
generally agree with both DNS at the same
$Re_{D}=5\times 10^{3}$
generally agree with both DNS at the same
![]() $Re_{D}$
and also experiment at
$Re_{D}$
and also experiment at
![]() $Re_{D}=5.3\times 10^{3}$
by (Thom Reference Thom1934). The other two experiments, Swanson (Reference Swanson1961), which covers
$Re_{D}=5.3\times 10^{3}$
by (Thom Reference Thom1934). The other two experiments, Swanson (Reference Swanson1961), which covers
![]() $Re_{D}=3.5\times 10^{4}$
–
$Re_{D}=3.5\times 10^{4}$
–
![]() $3\times 10^{5}$
, and Carstensen et al. (Reference Carstensen, Mandviwalla, Vita and Paulsen2014), in the range
$3\times 10^{5}$
, and Carstensen et al. (Reference Carstensen, Mandviwalla, Vita and Paulsen2014), in the range
![]() $Re_{D}=3.2\times 10^{4}{-}9.9\times 10^{4}$
, agree with each other. Both show a small decrease from measurements at
$Re_{D}=3.2\times 10^{4}{-}9.9\times 10^{4}$
, agree with each other. Both show a small decrease from measurements at
![]() $Re_{D}=5\times 10^{3}$
. This decrease is observed in our LES results with
$Re_{D}=5\times 10^{3}$
. This decrease is observed in our LES results with
![]() $Re_{D}=6\times 10^{4}$
at
$Re_{D}=6\times 10^{4}$
at
![]() $\unicode[STIX]{x1D6FC}=1$
, but not at higher
$\unicode[STIX]{x1D6FC}=1$
, but not at higher
![]() $\unicode[STIX]{x1D6FC}$
.
$\unicode[STIX]{x1D6FC}$
.
A plot of
![]() $C_{L}$
versus
$C_{L}$
versus
![]() $\unicode[STIX]{x1D6FC}$
in
$\unicode[STIX]{x1D6FC}$
in
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
is shown in figure 4(b). Here LES data at
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
is shown in figure 4(b). Here LES data at
![]() $Re_{D}=6\times 10^{4}$
, with both a fine and coarse mesh, cases ‘F’ and ‘C’ respectively of table 1, are included. The former LES are computationally expensive and were performed only at
$Re_{D}=6\times 10^{4}$
, with both a fine and coarse mesh, cases ‘F’ and ‘C’ respectively of table 1, are included. The former LES are computationally expensive and were performed only at
![]() $\unicode[STIX]{x1D6FC}=0.48,0.6$
. They show good agreement with the coarser-mesh LES at these
$\unicode[STIX]{x1D6FC}=0.48,0.6$
. They show good agreement with the coarser-mesh LES at these
![]() $\unicode[STIX]{x1D6FC}$
values, indicating that the coarse-mesh LES are converged. The LES
$\unicode[STIX]{x1D6FC}$
values, indicating that the coarse-mesh LES are converged. The LES
![]() $C_{L}$
shows reasonable agreement with the data by Swanson (Reference Swanson1961) for
$C_{L}$
shows reasonable agreement with the data by Swanson (Reference Swanson1961) for
![]() $\unicode[STIX]{x1D6FC}\leqslant 0.48$
, before the beginning of the lift crisis. While the range of
$\unicode[STIX]{x1D6FC}\leqslant 0.48$
, before the beginning of the lift crisis. While the range of
![]() $\unicode[STIX]{x1D6FC}$
spanning the
$\unicode[STIX]{x1D6FC}$
spanning the
![]() $C_{L}$
dip is well captured by the LES, the magnitude of the change differs between LES and experiment. In
$C_{L}$
dip is well captured by the LES, the magnitude of the change differs between LES and experiment. In
![]() $0.48\leqslant \unicode[STIX]{x1D6FC}\leqslant 0.6$
, the experimental data (Swanson Reference Swanson1961) show a decreases
$0.48\leqslant \unicode[STIX]{x1D6FC}\leqslant 0.6$
, the experimental data (Swanson Reference Swanson1961) show a decreases
![]() $C_{L}\sim 0.37\rightarrow 0.24$
whereas LES data from both the fine and coarse-mesh LES show
$C_{L}\sim 0.37\rightarrow 0.24$
whereas LES data from both the fine and coarse-mesh LES show
![]() $C_{L}\sim 0.4\rightarrow -0.1$
. In comparison, Kim et al. (Reference Kim, Choi, Park and Yoo2014) report experimental measurements for flow past a rotating sphere showing
$C_{L}\sim 0.4\rightarrow -0.1$
. In comparison, Kim et al. (Reference Kim, Choi, Park and Yoo2014) report experimental measurements for flow past a rotating sphere showing
![]() $C_{L}\sim 0.3\rightarrow 0.05$
over the range
$C_{L}\sim 0.3\rightarrow 0.05$
over the range
![]() $\unicode[STIX]{x1D6FC}\approx 0.53{-}0.7$
at the same
$\unicode[STIX]{x1D6FC}\approx 0.53{-}0.7$
at the same
![]() $Re_{D}=6\times 10^{4}$
. For higher
$Re_{D}=6\times 10^{4}$
. For higher
![]() $Re_{D}$
, up to
$Re_{D}$
, up to
![]() $Re_{D}=1.8\times 10^{5}$
they find more negative local
$Re_{D}=1.8\times 10^{5}$
they find more negative local
![]() $C_{L}$
minima. The experimental studies by Swanson (Reference Swanson1961) and Takayama & Aoki (Reference Takayama and Aoki2005) give scant details of the measurement technique for
$C_{L}$
minima. The experimental studies by Swanson (Reference Swanson1961) and Takayama & Aoki (Reference Takayama and Aoki2005) give scant details of the measurement technique for
![]() $C_{L}$
and so assessment of the experimental accuracy is uncertain. The rotating sphere experiments by Kim et al. (Reference Kim, Choi, Park and Yoo2014) show a relatively strong lift crisis consistent with the trend shown by the present LES. We conclude that the magnitude of the lift crisis for a rotating cylinder as a function of
$C_{L}$
and so assessment of the experimental accuracy is uncertain. The rotating sphere experiments by Kim et al. (Reference Kim, Choi, Park and Yoo2014) show a relatively strong lift crisis consistent with the trend shown by the present LES. We conclude that the magnitude of the lift crisis for a rotating cylinder as a function of
![]() $(Re_{D},\unicode[STIX]{x1D6FC})$
remains an open question.
$(Re_{D},\unicode[STIX]{x1D6FC})$
remains an open question.

Figure 5. Drag coefficient
![]() $C_{D}$
. (a) Full scale; (b) for
$C_{D}$
. (a) Full scale; (b) for
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
. Symbols same as in figure 4.
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$
. Symbols same as in figure 4.
![]() $\diamondsuit$
, experiments by Takayama & Aoki (Reference Takayama and Aoki2005) at
$\diamondsuit$
, experiments by Takayama & Aoki (Reference Takayama and Aoki2005) at
![]() $Re_{D}=6\times 10^{4}$
.
$Re_{D}=6\times 10^{4}$
.
3.3.2 Drag crisis of
 $C_{D}(Re_{D},\unicode[STIX]{x1D6FC})$
$C_{D}(Re_{D},\unicode[STIX]{x1D6FC})$
The drag coefficient results
![]() $C_{D}(Re_{D},\unicode[STIX]{x1D6FC})$
are shown in figure 5. In figure 5(a), the LES at
$C_{D}(Re_{D},\unicode[STIX]{x1D6FC})$
are shown in figure 5. In figure 5(a), the LES at
![]() $Re_{D}=5\times 10^{3}$
generally agree with DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015). For this
$Re_{D}=5\times 10^{3}$
generally agree with DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015). For this
![]() $Re_{D}$
, the drag decrease is smooth and continuous while for
$Re_{D}$
, the drag decrease is smooth and continuous while for
![]() $Re_{D}=6\times 10^{4}$
,
$Re_{D}=6\times 10^{4}$
,
![]() $C_{D}$
shows a somewhat sharp decrease at approximately
$C_{D}$
shows a somewhat sharp decrease at approximately
![]() $\unicode[STIX]{x1D6FC}=0.6$
. In figure 5(b), we compare the present LES and the experimental data by Swanson (Reference Swanson1961) and Takayama & Aoki (Reference Takayama and Aoki2005) at
$\unicode[STIX]{x1D6FC}=0.6$
. In figure 5(b), we compare the present LES and the experimental data by Swanson (Reference Swanson1961) and Takayama & Aoki (Reference Takayama and Aoki2005) at
![]() $Re_{D}=6\times 10^{4}$
. The drag crisis is reasonably well captured.
$Re_{D}=6\times 10^{4}$
. The drag crisis is reasonably well captured.
3.3.3 Time histories of
 $C_{L}$
and
$C_{L}$
and
 $C_{D}$
$C_{D}$
The time histories of
![]() $C_{L}$
and
$C_{L}$
and
![]() $C_{D}$
for rotating-cylinder flow are known to show regular shedding and a closed curve in a phase diagram of
$C_{D}$
for rotating-cylinder flow are known to show regular shedding and a closed curve in a phase diagram of
![]() $C_{L}$
versus
$C_{L}$
versus
![]() $C_{D}$
, as shown by Mittal & Kumar (Reference Mittal and Kumar2003) for
$C_{D}$
, as shown by Mittal & Kumar (Reference Mittal and Kumar2003) for
![]() $Re_{D}=200$
. For high-Reynolds-number flow, strong three-dimensional (3D) flow effects together with turbulent transition in the wake flow are expected to produce a more complex
$Re_{D}=200$
. For high-Reynolds-number flow, strong three-dimensional (3D) flow effects together with turbulent transition in the wake flow are expected to produce a more complex
![]() $C_{L}-C_{D}$
evolution.
$C_{L}-C_{D}$
evolution.

Figure 6.
![]() $C_{L}$
and
$C_{L}$
and
![]() $C_{D}$
for
$C_{D}$
for
![]() $Re_{D}=5\times 10^{3}$
, cases:
$Re_{D}=5\times 10^{3}$
, cases:
![]() $\unicode[STIX]{x1D6FC}=0,1.0,2.0$
. (a) Time histories of
$\unicode[STIX]{x1D6FC}=0,1.0,2.0$
. (a) Time histories of
![]() $C_{L}(T)$
, where
$C_{L}(T)$
, where
![]() $T=tU_{\infty }/D$
is dimensionless physical time. (b)
$T=tU_{\infty }/D$
is dimensionless physical time. (b)
![]() $C_{L}{-}C_{D}$
phase diagram.
$C_{L}{-}C_{D}$
phase diagram.

Figure 7.
![]() $C_{L}$
and
$C_{L}$
and
![]() $C_{D}$
for
$C_{D}$
for
![]() $Re_{D}=6\times 10^{4}$
,
$Re_{D}=6\times 10^{4}$
,
![]() $\unicode[STIX]{x1D6FC}=0,1.0,2.0$
. (a) Time histories of
$\unicode[STIX]{x1D6FC}=0,1.0,2.0$
. (a) Time histories of
![]() $C_{L}$
. (b)
$C_{L}$
. (b)
![]() $C_{L}{-}C_{D}$
phase diagram.
$C_{L}{-}C_{D}$
phase diagram.
In figure 6, LES results for
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $1.0$
and
$1.0$
and
![]() $2.0$
at
$2.0$
at
![]() $Re_{D}=5\times 10^{3}$
are shown, including the
$Re_{D}=5\times 10^{3}$
are shown, including the
![]() $C_{L}$
evolution with time
$C_{L}$
evolution with time
![]() $T$
and also the
$T$
and also the
![]() $C_{L}-C_{D}$
phase diagram. Results at
$C_{L}-C_{D}$
phase diagram. Results at
![]() $\unicode[STIX]{x1D6FC}=1.0$
show a slight increase of the fluctuation magnitude in
$\unicode[STIX]{x1D6FC}=1.0$
show a slight increase of the fluctuation magnitude in
![]() $C_{L}(t)$
compared with
$C_{L}(t)$
compared with
![]() $\unicode[STIX]{x1D6FC}=0$
, in agreement with the trend of DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015). At
$\unicode[STIX]{x1D6FC}=0$
, in agreement with the trend of DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015). At
![]() $\unicode[STIX]{x1D6FC}=2.0$
, although the vortex shedding should vanish owing to the magnification of the asymmetry,
$\unicode[STIX]{x1D6FC}=2.0$
, although the vortex shedding should vanish owing to the magnification of the asymmetry,
![]() $C_{L}$
still shows robust oscillatory behaviour owing to turbulence in the far-wake flow. This can also be observed in the
$C_{L}$
still shows robust oscillatory behaviour owing to turbulence in the far-wake flow. This can also be observed in the
![]() $C_{L}-C_{D}$
phase diagram, which shows reasonably regular structures corresponding to shedding for
$C_{L}-C_{D}$
phase diagram, which shows reasonably regular structures corresponding to shedding for
![]() $\unicode[STIX]{x1D6FC}=0$
and
$\unicode[STIX]{x1D6FC}=0$
and
![]() $1.0$
, but a rather chaotic trajectory for
$1.0$
, but a rather chaotic trajectory for
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
In figure 7, results at
![]() $Re_{D}=6\times 10^{4}$
are shown for
$Re_{D}=6\times 10^{4}$
are shown for
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $1.0$
and
$1.0$
and
![]() $2.0$
. For this higher
$2.0$
. For this higher
![]() $Re_{D}$
flow,
$Re_{D}$
flow,
![]() $\unicode[STIX]{x1D6FC}=1.0$
is found to produce a much smaller fluctuation in
$\unicode[STIX]{x1D6FC}=1.0$
is found to produce a much smaller fluctuation in
![]() $C_{L}$
than for
$C_{L}$
than for
![]() $\unicode[STIX]{x1D6FC}=0$
. Also from the phase diagram,
$\unicode[STIX]{x1D6FC}=0$
. Also from the phase diagram,
![]() $\unicode[STIX]{x1D6FC}=0$
can be seen to produce regular shedding, while for
$\unicode[STIX]{x1D6FC}=0$
can be seen to produce regular shedding, while for
![]() $\unicode[STIX]{x1D6FC}=1.0$
and
$\unicode[STIX]{x1D6FC}=1.0$
and
![]() $2.0$
, strongly chaotic behaviour overwhelms the shedding and quasiperiodic behaviour is not apparent.
$2.0$
, strongly chaotic behaviour overwhelms the shedding and quasiperiodic behaviour is not apparent.

Figure 8.
![]() $C_{L}$
and
$C_{L}$
and
![]() $C_{D}$
for
$C_{D}$
for
![]() $Re_{D}=6\times 10^{4}$
across the lift crisis:
$Re_{D}=6\times 10^{4}$
across the lift crisis:
![]() $\unicode[STIX]{x1D6FC}=0.48,0.6$
. (a) Time histories of
$\unicode[STIX]{x1D6FC}=0.48,0.6$
. (a) Time histories of
![]() $C_{L}$
. (b)
$C_{L}$
. (b)
![]() $C_{L}{-}C_{D}$
phase diagram.
$C_{L}{-}C_{D}$
phase diagram.

Figure 9. Skin-friction lines for case at
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (b)
$\unicode[STIX]{x1D6FC}=0.48$
; (b)
![]() $\unicode[STIX]{x1D6FC}=0.6$
.
$\unicode[STIX]{x1D6FC}=0.6$
.
It is of interest to check the time-history behaviour around the lift crisis, for example,
![]() $\unicode[STIX]{x1D6FC}=0.48$
before the lift crisis and
$\unicode[STIX]{x1D6FC}=0.48$
before the lift crisis and
![]() $\unicode[STIX]{x1D6FC}=0.6$
just following the lift crisis. Figure 8 shows striking differences for these cases. At
$\unicode[STIX]{x1D6FC}=0.6$
just following the lift crisis. Figure 8 shows striking differences for these cases. At
![]() $\unicode[STIX]{x1D6FC}=0.48$
there is a strong fluctuation in
$\unicode[STIX]{x1D6FC}=0.48$
there is a strong fluctuation in
![]() $C_{L}$
while for
$C_{L}$
while for
![]() $\unicode[STIX]{x1D6FC}=0.6$
this is largely suppressed. This suppression of vortex shedding provides a useful signature of the onset of the lift crisis. In order to quantify the fluctuation behaviour of the lift/drag coefficients we compute the root-mean-square
$\unicode[STIX]{x1D6FC}=0.6$
this is largely suppressed. This suppression of vortex shedding provides a useful signature of the onset of the lift crisis. In order to quantify the fluctuation behaviour of the lift/drag coefficients we compute the root-mean-square
![]() $C_{Lrms}$
and
$C_{Lrms}$
and
![]() $C_{Drms}$
, of the timewise variation of
$C_{Drms}$
, of the timewise variation of
![]() $C_{L}(t)$
and
$C_{L}(t)$
and
![]() $C_{D}(t)$
, respectively. Results for
$C_{D}(t)$
, respectively. Results for
![]() $Re_{D}=5\times 10^{3}$
are shown in appendix A for comparison with the DNS of Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015). Results at
$Re_{D}=5\times 10^{3}$
are shown in appendix A for comparison with the DNS of Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015). Results at
![]() $Re_{D}=6\times 10^{4}$
are listed in table 4. It can be seen that, after the lift crisis, both
$Re_{D}=6\times 10^{4}$
are listed in table 4. It can be seen that, after the lift crisis, both
![]() $C_{Lrms}$
and
$C_{Lrms}$
and
![]() $C_{Drms}$
decrease to relatively low values while
$C_{Drms}$
decrease to relatively low values while
![]() $C_{Lrms}$
remains nearly constant for
$C_{Lrms}$
remains nearly constant for
![]() $\unicode[STIX]{x1D6FC}\geqslant 0.6$
.
$\unicode[STIX]{x1D6FC}\geqslant 0.6$
.
Table 4. LES calculation of lift coefficient fluctuation
![]() $C_{Lrms}$
, and drag coefficient fluctuation
$C_{Lrms}$
, and drag coefficient fluctuation
![]() $C_{Drms}$
:
$C_{Drms}$
:
![]() $Re_{D}=6\times 10^{4}$
.
$Re_{D}=6\times 10^{4}$
.
4 Skin-friction portraits and mean-flow streamlines
4.1 Flow reorganization: the instantaneous
 $\boldsymbol{C}_{\boldsymbol{f}}$
vector field
$\boldsymbol{C}_{\boldsymbol{f}}$
vector field

Figure 10. Skin-friction lines for case at
![]() $Re_{D}=6\times 10^{4}$
and
$Re_{D}=6\times 10^{4}$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
. This shows a close-up view of the band of small-scale separation/reattachment cells. ▫, typical repelling points (local flow reattachment). ○, typical attracting points (local flow separation).
$\unicode[STIX]{x1D6FC}=0.6$
. This shows a close-up view of the band of small-scale separation/reattachment cells. ▫, typical repelling points (local flow reattachment). ○, typical attracting points (local flow separation).
Following Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017), we discuss separation and reattachment events as flow near or surrounding critical points of the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
vector field on the cylinder surface. Two representative
$\boldsymbol{C}_{\boldsymbol{f}}$
vector field on the cylinder surface. Two representative
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
surface fields for
$\boldsymbol{C}_{\boldsymbol{f}}$
surface fields for
![]() $Re_{D}=6\times 10^{4}$
are shown in figure 9 for
$Re_{D}=6\times 10^{4}$
are shown in figure 9 for
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
. With
$\unicode[STIX]{x1D6FC}=0.6$
. With
![]() $\unicode[STIX]{x1D6FC}=0.48$
, the flow attachment line, indicated by diverging
$\unicode[STIX]{x1D6FC}=0.48$
, the flow attachment line, indicated by diverging
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
trajectories, is seen at approximately
$\boldsymbol{C}_{\boldsymbol{f}}$
trajectories, is seen at approximately
![]() $\unicode[STIX]{x1D703}=5^{\circ }$
. On the top side of the cylinder, the attached flow shows large-scale separation at approximately
$\unicode[STIX]{x1D703}=5^{\circ }$
. On the top side of the cylinder, the attached flow shows large-scale separation at approximately
![]() $\unicode[STIX]{x1D703}=96^{\circ }$
while on the bottom side, the attached flow does not show a focused separation line. According to Chong et al. (Reference Chong, Soria, Perry, Chacin, Cantwell and Na1998), prior to the main flow separation, there generally exists fluctuating small-scale flow reversals scattered over a finite portion of the body surface, corresponding to multiple, scattered critical points in the
$\unicode[STIX]{x1D703}=96^{\circ }$
while on the bottom side, the attached flow does not show a focused separation line. According to Chong et al. (Reference Chong, Soria, Perry, Chacin, Cantwell and Na1998), prior to the main flow separation, there generally exists fluctuating small-scale flow reversals scattered over a finite portion of the body surface, corresponding to multiple, scattered critical points in the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
field. For
$\boldsymbol{C}_{\boldsymbol{f}}$
field. For
![]() $\unicode[STIX]{x1D6FC}=0.48$
, for example at
$\unicode[STIX]{x1D6FC}=0.48$
, for example at
![]() $y/D=1.0$
, the flow shows critical points at approximately
$y/D=1.0$
, the flow shows critical points at approximately
![]() $\unicode[STIX]{x1D703}=264^{\circ }$
while at
$\unicode[STIX]{x1D703}=264^{\circ }$
while at
![]() $y/D=2.5$
, these are present at approximately
$y/D=2.5$
, these are present at approximately
![]() $\unicode[STIX]{x1D703}=216^{\circ }$
. This indicates that the flow is locally strongly 3D and unsteady, with the consequence that structured separation is difficult to recognize.
$\unicode[STIX]{x1D703}=216^{\circ }$
. This indicates that the flow is locally strongly 3D and unsteady, with the consequence that structured separation is difficult to recognize.

Figure 11.
![]() $C_{L}$
versus time for cases at
$C_{L}$
versus time for cases at
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (b)
$\unicode[STIX]{x1D6FC}=0.48$
; (b)
![]() $\unicode[STIX]{x1D6FC}=0.6$
. Points labelled A, B, C and D are used for comparison of flow behaviour in the shedding process. Points labelled C
$\unicode[STIX]{x1D6FC}=0.6$
. Points labelled A, B, C and D are used for comparison of flow behaviour in the shedding process. Points labelled C
![]() $^{\prime }$
and D
$^{\prime }$
and D
![]() $^{\prime }$
at
$^{\prime }$
at
![]() $\unicode[STIX]{x1D6FC}=0.48$
are useful intermediate points.
$\unicode[STIX]{x1D6FC}=0.48$
are useful intermediate points.

Figure 12. Instantaneous skin-friction lines for
![]() $\unicode[STIX]{x1D6FC}=0.48$
at
$\unicode[STIX]{x1D6FC}=0.48$
at
![]() $Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
$Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
![]() $C_{L}$
for each instant, see figure 11(a).
$C_{L}$
for each instant, see figure 11(a).
In contrast, with
![]() $\unicode[STIX]{x1D6FC}=0.6$
, the
$\unicode[STIX]{x1D6FC}=0.6$
, the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
surface field shows a somewhat different appearance. The attachment stagnation line moves to approximately
$\boldsymbol{C}_{\boldsymbol{f}}$
surface field shows a somewhat different appearance. The attachment stagnation line moves to approximately
![]() $\unicode[STIX]{x1D703}=12^{\circ }$
, while the separation line on the top side of the cylinder is still at around
$\unicode[STIX]{x1D703}=12^{\circ }$
, while the separation line on the top side of the cylinder is still at around
![]() $\unicode[STIX]{x1D703}=96^{\circ }$
. Near
$\unicode[STIX]{x1D703}=96^{\circ }$
. Near
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
the flow on the bottom side of the cylinder forms small-scale reversal flow cells concentrated in a zone
$\unicode[STIX]{x1D703}=270^{\circ }$
the flow on the bottom side of the cylinder forms small-scale reversal flow cells concentrated in a zone
![]() $\unicode[STIX]{x1D703}\approx 250^{\circ }{-}280^{\circ }$
and extending across the entire span. A close-up view in
$\unicode[STIX]{x1D703}\approx 250^{\circ }{-}280^{\circ }$
and extending across the entire span. A close-up view in
![]() $0<y/D<0.4$
is shown in figure 10. Localized flow reversals correspond to bundles of skin-friction lines appearing as separatrices connecting critical points of the
$0<y/D<0.4$
is shown in figure 10. Localized flow reversals correspond to bundles of skin-friction lines appearing as separatrices connecting critical points of the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
field. Example critical points are labelled as ‘repelling’ (square symbol), where skin-friction lines diverge indicating localized reattaching flow, and as ‘attracting’ (circular symbol), where skin-friction converge implying local flow separation. A large array of roughly randomly distributed critical points exists in this region at the time instant displayed. We refer to this as an aggregation zone of small-scale, flow separation/reattachment cells. Its formation is interpreted as strong evidence of structured but distributed localized flow separation/reattachment.
$\boldsymbol{C}_{\boldsymbol{f}}$
field. Example critical points are labelled as ‘repelling’ (square symbol), where skin-friction lines diverge indicating localized reattaching flow, and as ‘attracting’ (circular symbol), where skin-friction converge implying local flow separation. A large array of roughly randomly distributed critical points exists in this region at the time instant displayed. We refer to this as an aggregation zone of small-scale, flow separation/reattachment cells. Its formation is interpreted as strong evidence of structured but distributed localized flow separation/reattachment.
4.2 Sequential
 $\boldsymbol{C}_{\boldsymbol{f}}(\unicode[STIX]{x1D703},y)$
portraits
$\boldsymbol{C}_{\boldsymbol{f}}(\unicode[STIX]{x1D703},y)$
portraits
In figure 11 we show the instantaneous lift coefficient
![]() $\boldsymbol{C}_{\boldsymbol{f}}(\unicode[STIX]{x1D703},y)$
at several different times during a cycle for
$\boldsymbol{C}_{\boldsymbol{f}}(\unicode[STIX]{x1D703},y)$
at several different times during a cycle for
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $0.6$
at
$0.6$
at
![]() $Re_{D}=6\times 10^{4}$
. In each panel we mark four dots which represent times at which the instantaneous
$Re_{D}=6\times 10^{4}$
. In each panel we mark four dots which represent times at which the instantaneous
![]() $C_{f}$
fields are plotted. For the four points, point A is close to
$C_{f}$
fields are plotted. For the four points, point A is close to
![]() $C_{L}=0$
, point B corresponds to minimum
$C_{L}=0$
, point B corresponds to minimum
![]() $C_{L}$
, point C is near the midvalue between the valley and peak and point D is around the
$C_{L}$
, point C is near the midvalue between the valley and peak and point D is around the
![]() $C_{L}$
peak. For
$C_{L}$
peak. For
![]() $\unicode[STIX]{x1D6FC}=0.48$
, two other instants labelled as C
$\unicode[STIX]{x1D6FC}=0.48$
, two other instants labelled as C
![]() $^{\prime }$
and D
$^{\prime }$
and D
![]() $^{\prime }$
are shown, which will be discussed subsequently.
$^{\prime }$
are shown, which will be discussed subsequently.
4.2.1
 $\unicode[STIX]{x1D6FC}=0.48$
$\unicode[STIX]{x1D6FC}=0.48$

Figure 13. Examples of ‘wash flow’ in instantaneous skin-friction lines for
![]() $\unicode[STIX]{x1D6FC}=0.48$
at
$\unicode[STIX]{x1D6FC}=0.48$
at
![]() $Re_{D}=6\times 10^{4}$
. (a) Close-up at instant C; (b) close-up at instant D.
$Re_{D}=6\times 10^{4}$
. (a) Close-up at instant C; (b) close-up at instant D.

Figure 14. Streamlines of instantaneous spanwise-averaged fields of
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
(defined in (4.1)) for
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
(defined in (4.1)) for
![]() $\unicode[STIX]{x1D6FC}=0.48$
at
$\unicode[STIX]{x1D6FC}=0.48$
at
![]() $Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
$Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
![]() $C_{L}$
for each instant, see figure 11(a).
$C_{L}$
for each instant, see figure 11(a).

Figure 15. Streamlines of instantaneous spanwise-averaged fields of
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
(defined in (4.1)) for
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
(defined in (4.1)) for
![]() $\unicode[STIX]{x1D6FC}=0.48$
at
$\unicode[STIX]{x1D6FC}=0.48$
at
![]() $Re_{D}=6\times 10^{4}$
. (a) instant C
$Re_{D}=6\times 10^{4}$
. (a) instant C
![]() $^{\prime }$
; (b) instant D
$^{\prime }$
; (b) instant D
![]() $^{\prime }$
.
$^{\prime }$
.

Figure 16. Instantaneous skin-friction lines for
![]() $\unicode[STIX]{x1D6FC}=0.6$
at
$\unicode[STIX]{x1D6FC}=0.6$
at
![]() $Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
$Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
![]() $C_{L}$
for each instant, see figure 11(b).
$C_{L}$
for each instant, see figure 11(b).
For
![]() $\unicode[STIX]{x1D6FC}=0.48$
, we emphasize again that the flow on the bottom cylinder surface does not clearly show a structured pattern in the range
$\unicode[STIX]{x1D6FC}=0.48$
, we emphasize again that the flow on the bottom cylinder surface does not clearly show a structured pattern in the range
![]() $\unicode[STIX]{x1D703}=200^{\circ }{-}270^{\circ }$
due to its strong flow variation in the spanwise direction. In figure 12, at instant B the whole skin-friction field looks chaotic with no obvious unidirectional flow apparent at approximately
$\unicode[STIX]{x1D703}=200^{\circ }{-}270^{\circ }$
due to its strong flow variation in the spanwise direction. In figure 12, at instant B the whole skin-friction field looks chaotic with no obvious unidirectional flow apparent at approximately
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
, while for instant C a clockwise wash flow is evident at approximately
$\unicode[STIX]{x1D703}=180^{\circ }$
, while for instant C a clockwise wash flow is evident at approximately
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
. Among the four plots, the instant D, where
$\unicode[STIX]{x1D703}=180^{\circ }$
. Among the four plots, the instant D, where
![]() $C_{L}$
shows a maximum, shows strong anticlockwise wash flow towards the top side of the cylinder at around
$C_{L}$
shows a maximum, shows strong anticlockwise wash flow towards the top side of the cylinder at around
![]() $180^{\circ }$
. The nomenclature ‘wash flow’ is presently used to denote a sloshing and reversing but largely attached surface flow. To clarify, in figure 13, we show close-up views at instants C and D for
$180^{\circ }$
. The nomenclature ‘wash flow’ is presently used to denote a sloshing and reversing but largely attached surface flow. To clarify, in figure 13, we show close-up views at instants C and D for
![]() $1.5<y/D<2.0$
. At around
$1.5<y/D<2.0$
. At around
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
, footprints of attached clockwise directional attached flow are clearly visible at C, while at instant D, anticlockwise flow is evident. The existence of wash flow, which implies visible vortex shedding, is a feature of the flow at
$\unicode[STIX]{x1D703}=180^{\circ }$
, footprints of attached clockwise directional attached flow are clearly visible at C, while at instant D, anticlockwise flow is evident. The existence of wash flow, which implies visible vortex shedding, is a feature of the flow at
![]() $\unicode[STIX]{x1D6FC}=0.48$
.
$\unicode[STIX]{x1D6FC}=0.48$
.

Figure 17. Streamlines of instantaneous spanwise-averaged fields of
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
(defined in equation (4.1) for
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
(defined in equation (4.1) for
![]() $\unicode[STIX]{x1D6FC}=0.6$
at
$\unicode[STIX]{x1D6FC}=0.6$
at
![]() $Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
$Re_{D}=6\times 10^{4}$
. (a) Instant A; (b) instant B; (c) instant C; (d) instant D. For the corresponding
![]() $C_{L}$
for each instant, see figure 11(b).
$C_{L}$
for each instant, see figure 11(b).

Figure 18. Instantaneous, spanwise-averaged spanwise vorticity for
![]() $Re_{D}=6\times 10^{4}$
at instant of ‘C’ corresponding to figures 14(c) and 17(c). (a)
$Re_{D}=6\times 10^{4}$
at instant of ‘C’ corresponding to figures 14(c) and 17(c). (a)
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (b)
$\unicode[STIX]{x1D6FC}=0.48$
; (b)
![]() $\unicode[STIX]{x1D6FC}=0.6$
. The arrow near the colour bar indicates the ambient vorticity
$\unicode[STIX]{x1D6FC}=0.6$
. The arrow near the colour bar indicates the ambient vorticity
![]() $\unicode[STIX]{x1D714}_{\infty }=4\unicode[STIX]{x1D6FC}U_{\infty }/D$
:
$\unicode[STIX]{x1D714}_{\infty }=4\unicode[STIX]{x1D6FC}U_{\infty }/D$
:
![]() $\unicode[STIX]{x1D714}_{\infty }=1.92$
for (a),
$\unicode[STIX]{x1D714}_{\infty }=1.92$
for (a),
![]() $\unicode[STIX]{x1D714}_{\infty }=2.4$
for (b).
$\unicode[STIX]{x1D714}_{\infty }=2.4$
for (b).

Figure 19. An instantaneous spanwise vorticity field for
![]() $\unicode[STIX]{x1D6FC}=0.6$
at several spanwise slices.
$\unicode[STIX]{x1D6FC}=0.6$
at several spanwise slices.
The directional change of the wash flow provides evidence of some quasiperiodic flow behaviour. To explore this we implement a spanwise average for the four instantaneous flow fields, and plot their streamlines in figure 14. If
![]() $\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
represents the full velocity field, we define spanwise-averaged, instantaneous streamlines of the modified field
$\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
represents the full velocity field, we define spanwise-averaged, instantaneous streamlines of the modified field
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}=(u_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}},u_{\unicode[STIX]{x1D6FC},y},u_{\unicode[STIX]{x1D6FC},r})$
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}=(u_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}},u_{\unicode[STIX]{x1D6FC},y},u_{\unicode[STIX]{x1D6FC},r})$
This is used because
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)=0$
on the cylinder surface, and, as a consequence, the
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)=0$
on the cylinder surface, and, as a consequence, the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
portraits are in fact the limiting streamlines of
$\boldsymbol{C}_{\boldsymbol{f}}$
portraits are in fact the limiting streamlines of
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r\rightarrow D/2,t)$
on the cylinder surface, and not of
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r\rightarrow D/2,t)$
on the cylinder surface, and not of
![]() $\boldsymbol{u}(\unicode[STIX]{x1D703},y,r\rightarrow D/2,t)$
. In fact this can be formulated as a general principle for bodies that are heaving and rotating with respect to a fixed laboratory frame of reference. For instant A, the streamlines clearly show two flow regions. On the top of the cylinder, the flow attaches at approximately
$\boldsymbol{u}(\unicode[STIX]{x1D703},y,r\rightarrow D/2,t)$
. In fact this can be formulated as a general principle for bodies that are heaving and rotating with respect to a fixed laboratory frame of reference. For instant A, the streamlines clearly show two flow regions. On the top of the cylinder, the flow attaches at approximately
![]() $\unicode[STIX]{x1D703}=10^{\circ }$
and separates at approximately
$\unicode[STIX]{x1D703}=10^{\circ }$
and separates at approximately
![]() $\unicode[STIX]{x1D703}=100^{\circ }$
. On the cylinder bottom, the flow is also attached at approximately
$\unicode[STIX]{x1D703}=100^{\circ }$
. On the cylinder bottom, the flow is also attached at approximately
![]() $10^{\circ }$
and separates at approximately
$10^{\circ }$
and separates at approximately
![]() $100^{\circ }$
. For instant B a small separation bubble appears in the range of
$100^{\circ }$
. For instant B a small separation bubble appears in the range of
![]() $210^{\circ }<\unicode[STIX]{x1D703}<230^{\circ }$
, which corresponds to strongly 3D skin-friction lines. For instant C, this anticlockwise separation bubble increases, reflecting the strong clockwise directional wash flow evident in the skin-friction lines plot. For instant D, the near-wall flow on the bottom side of the cylinder is again fully attached, while downstream there is a clockwise-rotating vortex.
$210^{\circ }<\unicode[STIX]{x1D703}<230^{\circ }$
, which corresponds to strongly 3D skin-friction lines. For instant C, this anticlockwise separation bubble increases, reflecting the strong clockwise directional wash flow evident in the skin-friction lines plot. For instant D, the near-wall flow on the bottom side of the cylinder is again fully attached, while downstream there is a clockwise-rotating vortex.
To expose detailed differences between instants C and D, we show further streamline patterns in figure 15. Figure 15(a), which corresponds to instant C
![]() $^{\prime }$
, shows a clockwise vortex at approximately
$^{\prime }$
, shows a clockwise vortex at approximately
![]() $\unicode[STIX]{x1D703}=150^{\circ }$
, located off the wall and within the flow, induced by the strong shear layer. With further development, in figure 15(b) which corresponds to instant D
$\unicode[STIX]{x1D703}=150^{\circ }$
, located off the wall and within the flow, induced by the strong shear layer. With further development, in figure 15(b) which corresponds to instant D
![]() $^{\prime }$
, we find that the wall-attached separation bubble shrinks while the new clockwise vortex increases in extent. This flow behaviour is further followed by instant D (figure 14
d), where the separation bubble disappears. This process provides a full sequence consisting of the appearance/disappearance of a pair of vortices, one anticlockwise and attached to the wall, and the other clockwise and off the wall.
$^{\prime }$
, we find that the wall-attached separation bubble shrinks while the new clockwise vortex increases in extent. This flow behaviour is further followed by instant D (figure 14
d), where the separation bubble disappears. This process provides a full sequence consisting of the appearance/disappearance of a pair of vortices, one anticlockwise and attached to the wall, and the other clockwise and off the wall.

Figure 20. Skin-friction lines for
![]() $Re_{D}=6\times 10^{4}$
and
$Re_{D}=6\times 10^{4}$
and
![]() $\unicode[STIX]{x1D6FC}=1.0$
. (a) Instant with minimal
$\unicode[STIX]{x1D6FC}=1.0$
. (a) Instant with minimal
![]() $C_{L}$
; (b) instant with maximum
$C_{L}$
; (b) instant with maximum
![]() $C_{L}$
.
$C_{L}$
.

Figure 21. Skin-friction lines for
![]() $Re_{D}=6\times 10^{4}$
and
$Re_{D}=6\times 10^{4}$
and
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
4.2.2
 $\unicode[STIX]{x1D6FC}=0.6$
$\unicode[STIX]{x1D6FC}=0.6$
The four snapshot fields in figure 16 for
![]() $\unicode[STIX]{x1D6FC}=0.6$
look more ordered in the sense of a broad spanwise coherent region of anticlockwise near-surface flow. For the flow on the bottom side of the cylinder, all fields show a spanwise band of small-scale separation/reattachment cells restricted to the range
$\unicode[STIX]{x1D6FC}=0.6$
look more ordered in the sense of a broad spanwise coherent region of anticlockwise near-surface flow. For the flow on the bottom side of the cylinder, all fields show a spanwise band of small-scale separation/reattachment cells restricted to the range
![]() $\unicode[STIX]{x1D703}=250^{\circ }{-}280^{\circ }$
. For instants A, C and D, this small-scale, flow-reversal region extends approximately
$\unicode[STIX]{x1D703}=250^{\circ }{-}280^{\circ }$
. For instants A, C and D, this small-scale, flow-reversal region extends approximately
![]() $30^{\circ }$
and, at
$30^{\circ }$
and, at
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
, unidirectional wash flow can be seen. For instant B, which corresponds to the
$\unicode[STIX]{x1D703}=180^{\circ }$
, unidirectional wash flow can be seen. For instant B, which corresponds to the
![]() $C_{L}$
minimum, this region extends across a large range, reaching beyond
$C_{L}$
minimum, this region extends across a large range, reaching beyond
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
. For all time instants, the centre of this small-scale separation/reattachment zone corresponds to the
$\unicode[STIX]{x1D703}=180^{\circ }$
. For all time instants, the centre of this small-scale separation/reattachment zone corresponds to the
![]() $\unicode[STIX]{x1D703}$
-location of the pressure minimum of figure 2(c,e), and is thus associated with the sudden drop in
$\unicode[STIX]{x1D703}$
-location of the pressure minimum of figure 2(c,e), and is thus associated with the sudden drop in
![]() $C_{L}$
, or the lift crisis. No obvious wash flow can be found in any cases. The streamlines for the spanwise-averaged, instantaneous fields are plotted in figure 17. In each of the four cases, the flow on the bottom side of the cylinder does not show any coherent separation bubble phenomena. This indicates that the small-scale reversal flow field in the band
$C_{L}$
, or the lift crisis. No obvious wash flow can be found in any cases. The streamlines for the spanwise-averaged, instantaneous fields are plotted in figure 17. In each of the four cases, the flow on the bottom side of the cylinder does not show any coherent separation bubble phenomena. This indicates that the small-scale reversal flow field in the band
![]() $\unicode[STIX]{x1D703}=240^{\circ }{-}280^{\circ }$
does not produce strong, large-scale vortex shedding.
$\unicode[STIX]{x1D703}=240^{\circ }{-}280^{\circ }$
does not produce strong, large-scale vortex shedding.
The flow differences between
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
can also be seen in figure 18, which shows colour-coded instantaneous but spanwise-averaged vorticity fields with spanwise-averaged streamlines imposed at instant C. Clear vortex formation can be observed for
$\unicode[STIX]{x1D6FC}=0.6$
can also be seen in figure 18, which shows colour-coded instantaneous but spanwise-averaged vorticity fields with spanwise-averaged streamlines imposed at instant C. Clear vortex formation can be observed for
![]() $\unicode[STIX]{x1D6FC}=0.48$
, while little large-scale coherent vortex shedding is observed for
$\unicode[STIX]{x1D6FC}=0.48$
, while little large-scale coherent vortex shedding is observed for
![]() $\unicode[STIX]{x1D6FC}=0.6$
. For
$\unicode[STIX]{x1D6FC}=0.6$
. For
![]() $\unicode[STIX]{x1D6FC}=0.6$
, a high vorticity region forms around the bottom of the cylinder. This is a signature of local dynamic recirculation flow, which is part of the separation phenomenon. A 3D view of this vorticity distribution is provided in figure 19, which clearly shows the near-wall thin layer containing strong vorticity.
$\unicode[STIX]{x1D6FC}=0.6$
, a high vorticity region forms around the bottom of the cylinder. This is a signature of local dynamic recirculation flow, which is part of the separation phenomenon. A 3D view of this vorticity distribution is provided in figure 19, which clearly shows the near-wall thin layer containing strong vorticity.
4.2.3
 $\unicode[STIX]{x1D6FC}=1$
,
$\unicode[STIX]{x1D6FC}=1$
,
 $\unicode[STIX]{x1D6FC}=2$
$\unicode[STIX]{x1D6FC}=2$
Since we hypothesize that the flow reorganization is characterized by the aggregation of the small-scale separation/reattachment events, it is prudent to check the behaviour of the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
field for higher-
$\boldsymbol{C}_{\boldsymbol{f}}$
field for higher-
![]() $\unicode[STIX]{x1D6FC}$
cases. In figure 20, we show two plots of the skin-friction lines at
$\unicode[STIX]{x1D6FC}$
cases. In figure 20, we show two plots of the skin-friction lines at
![]() $Re_{D}=6\times 10^{4}$
for
$Re_{D}=6\times 10^{4}$
for
![]() $\unicode[STIX]{x1D6FC}=1$
corresponding to minimum and maximum
$\unicode[STIX]{x1D6FC}=1$
corresponding to minimum and maximum
![]() $C_{L}$
during a cycle. Little difference can be seen between the plots. A notable feature is the shrinking of the azimuthal extent of the small-scale separation/reattachment cell zone, which for
$C_{L}$
during a cycle. Little difference can be seen between the plots. A notable feature is the shrinking of the azimuthal extent of the small-scale separation/reattachment cell zone, which for
![]() $\unicode[STIX]{x1D6FC}=0.6$
typically spans a range of approximately
$\unicode[STIX]{x1D6FC}=0.6$
typically spans a range of approximately
![]() $30^{\circ }$
, and approximately
$30^{\circ }$
, and approximately
![]() $15^{\circ }$
for
$15^{\circ }$
for
![]() $\unicode[STIX]{x1D6FC}=1$
. At
$\unicode[STIX]{x1D6FC}=1$
. At
![]() $\unicode[STIX]{x1D6FC}=2$
in figure 21, small-scale reversal flows can barely be seen at around
$\unicode[STIX]{x1D6FC}=2$
in figure 21, small-scale reversal flows can barely be seen at around
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
and most of the skin-friction lines are continuous on the entire surface without critical points, except on the reattachment line at approximately
$\unicode[STIX]{x1D703}=270^{\circ }$
and most of the skin-friction lines are continuous on the entire surface without critical points, except on the reattachment line at approximately
![]() $15^{\circ }$
and on the separation line at approximately
$15^{\circ }$
and on the separation line at approximately
![]() $115^{\circ }$
.
$115^{\circ }$
.
4.3 Instantaneous pressure field
The changes in skin-friction portraits between
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
suggest examination of the corresponding pressure distribution on the cylinder surface. In figure 22, we show colour-coded maps of the surface pressure for
$\unicode[STIX]{x1D6FC}=0.6$
suggest examination of the corresponding pressure distribution on the cylinder surface. In figure 22, we show colour-coded maps of the surface pressure for
![]() $\unicode[STIX]{x1D6FC}=0.48$
,
$\unicode[STIX]{x1D6FC}=0.48$
,
![]() $0.6$
and
$0.6$
and
![]() $1.0$
. These show substantial changes in the location of the low-pressure region on the cylinder bottom as
$1.0$
. These show substantial changes in the location of the low-pressure region on the cylinder bottom as
![]() $\unicode[STIX]{x1D6FC}$
increases at fixed
$\unicode[STIX]{x1D6FC}$
increases at fixed
![]() $Re_{D}$
. A low-pressure region appears at approximately
$Re_{D}$
. A low-pressure region appears at approximately
![]() $\unicode[STIX]{x1D703}=200^{\circ }$
for
$\unicode[STIX]{x1D703}=200^{\circ }$
for
![]() $\unicode[STIX]{x1D6FC}=0.48$
, shifting to around
$\unicode[STIX]{x1D6FC}=0.48$
, shifting to around
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
for
$\unicode[STIX]{x1D703}=270^{\circ }$
for
![]() $\unicode[STIX]{x1D6FC}=0.6$
. This clearly shows the flow separation behaviour across the lift crisis. For
$\unicode[STIX]{x1D6FC}=0.6$
. This clearly shows the flow separation behaviour across the lift crisis. For
![]() $\unicode[STIX]{x1D6FC}=1.0$
, the relative low-pressure region on the bottom part of the cylinder still resides around
$\unicode[STIX]{x1D6FC}=1.0$
, the relative low-pressure region on the bottom part of the cylinder still resides around
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
but is much weaker than for
$\unicode[STIX]{x1D703}=270^{\circ }$
but is much weaker than for
![]() $\unicode[STIX]{x1D6FC}=0.6$
. A large low-pressure region can be seen on the top side of the cylinder. This is a common feature at all
$\unicode[STIX]{x1D6FC}=0.6$
. A large low-pressure region can be seen on the top side of the cylinder. This is a common feature at all
![]() $Re_{D}$
with increasing
$Re_{D}$
with increasing
![]() $\unicode[STIX]{x1D6FC}$
.
$\unicode[STIX]{x1D6FC}$
.
A close-up view in figure 23 illustrates the relation between instantaneous pressure and identifiable features of the surface skin-friction portrait. The converging separatrices are indicative of locally separating flow, and can be seen to correspond to local, instantaneous minimal pressure, while the diverging bundles of skin-friction lines, associated with flow reattachment, tend to accumulate on patches of local maximum pressure. According to this correspondence between small-scale separation/reattachment zones, as seen in the surface skin-friction portrait, and local minima/maxima in pressure, it is reasonable to conclude that the formation of small-scale structures, perhaps in the form of near-wall recirculation eddies, results in local separation/reattachment behaviour. This is also apparent in the thin, near-wall region with concentrated vorticity, as shown in figure 19.

Figure 22. Instantaneous pressure field on the cylinder surface for
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (b)
$\unicode[STIX]{x1D6FC}=0.48$
; (b)
![]() $\unicode[STIX]{x1D6FC}=0.6$
; (c)
$\unicode[STIX]{x1D6FC}=0.6$
; (c)
![]() $\unicode[STIX]{x1D6FC}=1.0$
.
$\unicode[STIX]{x1D6FC}=1.0$
.

Figure 23. Instantaneous pressure field and skin-friction lines for
![]() $Re_{D}=6\times 10^{4}$
and
$Re_{D}=6\times 10^{4}$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
.
$\unicode[STIX]{x1D6FC}=0.6$
.
4.4 Mean flow

Figure 24. Streamlines of the mean velocity for
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
field for
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
field for
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (b)
$\unicode[STIX]{x1D6FC}=0.48$
; (b)
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
field
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
field
![]() $\unicode[STIX]{x1D6FC}=0.6$
; (c)
$\unicode[STIX]{x1D6FC}=0.6$
; (c)
![]() $\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
for
$\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
for
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (d)
$\unicode[STIX]{x1D6FC}=0.48$
; (d)
![]() $\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
field with
$\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
field with
![]() $\unicode[STIX]{x1D6FC}=0.6$
.
$\unicode[STIX]{x1D6FC}=0.6$
.
In the above comparison of surface skin-friction images for cases before (
![]() $\unicode[STIX]{x1D6FC}=0.48$
) and after (
$\unicode[STIX]{x1D6FC}=0.48$
) and after (
![]() $\unicode[STIX]{x1D6FC}=0.6$
) the lift crisis, we examine features of the instantaneous, spanwise-averaged flow field in order to examine local flow detail, revealing complex vortex movement for
$\unicode[STIX]{x1D6FC}=0.6$
) the lift crisis, we examine features of the instantaneous, spanwise-averaged flow field in order to examine local flow detail, revealing complex vortex movement for
![]() $\unicode[STIX]{x1D6FC}=0.48$
but relatively smooth flow for
$\unicode[STIX]{x1D6FC}=0.48$
but relatively smooth flow for
![]() $\unicode[STIX]{x1D6FC}=0.6$
and above. Here we give a short description of streamlines of the mean flow at
$\unicode[STIX]{x1D6FC}=0.6$
and above. Here we give a short description of streamlines of the mean flow at
![]() $Re_{D}=6\times 10^{4}$
, defined as a time–spanwise-averaged flow. In figure 24(a,b), we show the streamlines of the mean
$Re_{D}=6\times 10^{4}$
, defined as a time–spanwise-averaged flow. In figure 24(a,b), we show the streamlines of the mean
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
velocity field where the two cases shows similar streamlines, all attached on the wall except for the windward attachment at around
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(\unicode[STIX]{x1D703},y,r,t)$
velocity field where the two cases shows similar streamlines, all attached on the wall except for the windward attachment at around
![]() $15^{\circ }$
and separation at approximately
$15^{\circ }$
and separation at approximately
![]() $\unicode[STIX]{x1D703}=96^{\circ }$
. In figure 24(c,d), we plot the streamlines of the mean
$\unicode[STIX]{x1D703}=96^{\circ }$
. In figure 24(c,d), we plot the streamlines of the mean
![]() $\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
field. The clockwise-rotating vortex bubbles in the plots should not be considered as separation bubbles. Because the cylinder is rotating, the near-wall flow always follows the wall movement and thus the clockwise vortex, which has a near-wall reversed direction, cannot reside on the surface. The flow is also characterized by an anticlockwise wall-attached bubble and an off-wall vortex. However, since we are observing the structure in the laboratory-frame velocity field,
$\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
field. The clockwise-rotating vortex bubbles in the plots should not be considered as separation bubbles. Because the cylinder is rotating, the near-wall flow always follows the wall movement and thus the clockwise vortex, which has a near-wall reversed direction, cannot reside on the surface. The flow is also characterized by an anticlockwise wall-attached bubble and an off-wall vortex. However, since we are observing the structure in the laboratory-frame velocity field,
![]() $\unicode[STIX]{x1D6FC}=0.48$
shows a smaller vortex size which extends to only
$\unicode[STIX]{x1D6FC}=0.48$
shows a smaller vortex size which extends to only
![]() $x/D\approx 0.8$
while
$x/D\approx 0.8$
while
![]() $\unicode[STIX]{x1D6FC}=0.6$
shows a larger scale vortex which extends to
$\unicode[STIX]{x1D6FC}=0.6$
shows a larger scale vortex which extends to
![]() $x/D\approx 1.3$
. A further detail is that, for
$x/D\approx 1.3$
. A further detail is that, for
![]() $\unicode[STIX]{x1D6FC}=0.6$
, at around
$\unicode[STIX]{x1D6FC}=0.6$
, at around
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
, the
$\unicode[STIX]{x1D703}=270^{\circ }$
, the
![]() $\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
flow shows a small bubble, which although still part of the wall-attached bubble, has its own low-pressure region. This is exactly the flow signature of the low-
$\boldsymbol{u}(\unicode[STIX]{x1D703},y,r,t)$
flow shows a small bubble, which although still part of the wall-attached bubble, has its own low-pressure region. This is exactly the flow signature of the low-
![]() $C_{p}$
region, and in fact corresponds to the aggregation of the small-scale separation/reattachment cells on the wall discussed earlier.
$C_{p}$
region, and in fact corresponds to the aggregation of the small-scale separation/reattachment cells on the wall discussed earlier.
5 Statistical diagnostics of skin-friction vector field
 $C_{f}$
$C_{f}$
5.1 PDF of skin-friction coefficient
 $C_{f\unicode[STIX]{x1D703}}$
$C_{f\unicode[STIX]{x1D703}}$
To quantify the statistical features of the
![]() $C_{f\unicode[STIX]{x1D703}}$
profile, we sort
$C_{f\unicode[STIX]{x1D703}}$
profile, we sort
![]() $C_{f\unicode[STIX]{x1D703}}(y,t)$
over a long time frame and so construct the joint probability distribution function (PDF)
$C_{f\unicode[STIX]{x1D703}}(y,t)$
over a long time frame and so construct the joint probability distribution function (PDF)
![]() $P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
, normalized such that
$P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
, normalized such that
Scatter plots depicting
![]() $P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
at
$P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
at
![]() $Re_{D}=6\times 10^{4}$
, with
$Re_{D}=6\times 10^{4}$
, with
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
, are shown in figure 25. Monotonic grey-scale density is used, with darker colour denoting larger (positive) values of
$\unicode[STIX]{x1D6FC}=0.6$
, are shown in figure 25. Monotonic grey-scale density is used, with darker colour denoting larger (positive) values of
![]() $P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
. Comparing
$P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
. Comparing
![]() $P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
for the two cases at
$P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
for the two cases at
![]() $\unicode[STIX]{x1D703}=60^{\circ }$
, where the peak value of the mean-flow
$\unicode[STIX]{x1D703}=60^{\circ }$
, where the peak value of the mean-flow
![]() $C_{f\unicode[STIX]{x1D703}}(\unicode[STIX]{x1D703})$
is observed, it can be seen that the dark region for
$C_{f\unicode[STIX]{x1D703}}(\unicode[STIX]{x1D703})$
is observed, it can be seen that the dark region for
![]() $\unicode[STIX]{x1D6FC}=0.6$
is more concentrated, which implies weaker shedding compared to
$\unicode[STIX]{x1D6FC}=0.6$
is more concentrated, which implies weaker shedding compared to
![]() $\unicode[STIX]{x1D6FC}=0.48$
. On the bottom side of the cylinder a similar tendency can be observed at approximately
$\unicode[STIX]{x1D6FC}=0.48$
. On the bottom side of the cylinder a similar tendency can be observed at approximately
![]() $\unicode[STIX]{x1D703}=320^{\circ }$
.
$\unicode[STIX]{x1D703}=320^{\circ }$
.

Figure 25. PDF of the skin-friction coefficient
![]() $C_{f\unicode[STIX]{x1D703}}$
for
$C_{f\unicode[STIX]{x1D703}}$
for
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (b)
$\unicode[STIX]{x1D6FC}=0.48$
; (b)
![]() $\unicode[STIX]{x1D6FC}=0.6$
; (c) 3D view for
$\unicode[STIX]{x1D6FC}=0.6$
; (c) 3D view for
![]() $\unicode[STIX]{x1D6FC}=0.48$
; (d) 3D view for
$\unicode[STIX]{x1D6FC}=0.48$
; (d) 3D view for
![]() $\unicode[STIX]{x1D6FC}=0.6$
.
$\unicode[STIX]{x1D6FC}=0.6$
.
Substantial differences are apparent in the plateau region – the range roughly approximately
![]() $110^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
. For
$110^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
. For
![]() $\unicode[STIX]{x1D6FC}=0.48$
,
$\unicode[STIX]{x1D6FC}=0.48$
,
![]() $P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
shows broad tails in the
$P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
shows broad tails in the
![]() $C_{f\unicode[STIX]{x1D703}}$
variable from
$C_{f\unicode[STIX]{x1D703}}$
variable from
![]() $\unicode[STIX]{x1D703}=150^{\circ }$
to
$\unicode[STIX]{x1D703}=150^{\circ }$
to
![]() $210^{\circ }$
. This can be attributed to the washing flow, which changes direction gradually and slowly between up-wash and down-wash. For
$210^{\circ }$
. This can be attributed to the washing flow, which changes direction gradually and slowly between up-wash and down-wash. For
![]() $\unicode[STIX]{x1D6FC}=0.6$
, a widely scattered region is found in the range
$\unicode[STIX]{x1D6FC}=0.6$
, a widely scattered region is found in the range
![]() $240^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, which covers the bottom of the cylinder. Here
$240^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, which covers the bottom of the cylinder. Here
![]() $P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
is broad in the
$P(C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703})$
is broad in the
![]() $C_{f\unicode[STIX]{x1D703}}$
variable, with little dark concentration. From the previous description of the skin-friction lines, we conclude that this region is not related to the presence of washing flow. Instead, it is the result of the aggregation of small-scale separation/reattachment cells, which wrap up the critical points, resulting in chaotic local flow. For the purpose of clarity, 3D views of the two PDFs are shown in figure 25(c,d), respectively.
$C_{f\unicode[STIX]{x1D703}}$
variable, with little dark concentration. From the previous description of the skin-friction lines, we conclude that this region is not related to the presence of washing flow. Instead, it is the result of the aggregation of small-scale separation/reattachment cells, which wrap up the critical points, resulting in chaotic local flow. For the purpose of clarity, 3D views of the two PDFs are shown in figure 25(c,d), respectively.
5.2 The
 $\unicode[STIX]{x1D6FE}$
parameter
$\unicode[STIX]{x1D6FE}$
parameter

Figure 26. Sketch of the configuration of two flow regions: region I and region II. Attachment point (A) and separation point (S) are denoted.
We define the parameter
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
as
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
as
At fixed
![]() $\unicode[STIX]{x1D703}$
,
$\unicode[STIX]{x1D703}$
,
![]() $0\leqslant \unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})\leqslant 1$
is a measure of that total fraction of the
$0\leqslant \unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})\leqslant 1$
is a measure of that total fraction of the
![]() $C_{f\unicode[STIX]{x1D703}}$
time–spanwise ensemble that are positive, i.e.
$C_{f\unicode[STIX]{x1D703}}$
time–spanwise ensemble that are positive, i.e.
![]() $C_{f\unicode[STIX]{x1D703}}>0$
. This will now be shown to be a useful statistical metric for locally separated flow.
$C_{f\unicode[STIX]{x1D703}}>0$
. This will now be shown to be a useful statistical metric for locally separated flow.
Table 5. Flow features of the two regions.

The streamlines plots of
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(r,\unicode[STIX]{x1D703},y,t)$
clearly show one attachment and one primary-separation line, which broadly divides the flow into two parts. In figure 26, these are denoted regions I and II, respectively. In the sketch, the near-wall flow in region I attaches and flows in a clockwise direction, finally separating at the denoted separation point. Hence at a given
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(r,\unicode[STIX]{x1D703},y,t)$
clearly show one attachment and one primary-separation line, which broadly divides the flow into two parts. In figure 26, these are denoted regions I and II, respectively. In the sketch, the near-wall flow in region I attaches and flows in a clockwise direction, finally separating at the denoted separation point. Hence at a given
![]() $\unicode[STIX]{x1D703}$
in region I, if the flow is always attached, then
$\unicode[STIX]{x1D703}$
in region I, if the flow is always attached, then
![]() $\unicode[STIX]{x1D6FE}=1$
. Periods of statistical reversed flow at fixed
$\unicode[STIX]{x1D6FE}=1$
. Periods of statistical reversed flow at fixed
![]() $\unicode[STIX]{x1D703}$
will appear as excursions with
$\unicode[STIX]{x1D703}$
will appear as excursions with
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})<1$
. In contrast, in region II, statistically persistent attached flow at fixed
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})<1$
. In contrast, in region II, statistically persistent attached flow at fixed
![]() $\unicode[STIX]{x1D703}$
will have
$\unicode[STIX]{x1D703}$
will have
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})=0$
, while reversed or backflow, that is clockwise flow, will show
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})=0$
, while reversed or backflow, that is clockwise flow, will show
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})>0$
. The value
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})>0$
. The value
![]() $\unicode[STIX]{x1D6FE}=0.5$
is a nominal threshold (Simpson Reference Simpson1989): in region II,
$\unicode[STIX]{x1D6FE}=0.5$
is a nominal threshold (Simpson Reference Simpson1989): in region II,
![]() $\unicode[STIX]{x1D6FE}=0.5$
corresponds to instantaneous back flow 50 % of the time. Simpson (Reference Simpson1989) defines this as transitory separation. A schematic of the flow features in regions I and II is shown in table 5.
$\unicode[STIX]{x1D6FE}=0.5$
corresponds to instantaneous back flow 50 % of the time. Simpson (Reference Simpson1989) defines this as transitory separation. A schematic of the flow features in regions I and II is shown in table 5.

Figure 27.
![]() $\unicode[STIX]{x1D6FE}$
for
$\unicode[STIX]{x1D6FE}$
for
![]() $Re_{D}=6\times 10^{4}$
. In (a): ——,
$Re_{D}=6\times 10^{4}$
. In (a): ——,
![]() $\unicode[STIX]{x1D6FC}=0$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.2$
, – – – –,
$\unicode[STIX]{x1D6FC}=0.2$
, – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.48$
. In (b): – – – –,
$\unicode[STIX]{x1D6FC}=0.48$
. In (b): – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.48$
;
— ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.48$
;
— ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.52$
, — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.52$
, — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.56$
, ——,
$\unicode[STIX]{x1D6FC}=0.56$
, ——,
![]() $\unicode[STIX]{x1D6FC}=0.6$
. In (c): ——,
$\unicode[STIX]{x1D6FC}=0.6$
. In (c): ——,
![]() $\unicode[STIX]{x1D6FC}=0.6$
; – – – –,
$\unicode[STIX]{x1D6FC}=0.6$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.68$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.68$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.8$
;
— ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.8$
;
— ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=1.0$
. In (d): — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=1.0$
. In (d): — ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=1.0$
; – – – –,
$\unicode[STIX]{x1D6FC}=1.0$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
In figure 27, we show
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
for all cases at
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
for all cases at
![]() $Re_{D}=6\times 10^{4}$
. Figure 27(a) shows
$Re_{D}=6\times 10^{4}$
. Figure 27(a) shows
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
with
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
with
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $0.2$
and
$0.2$
and
![]() $0.48$
. For
$0.48$
. For
![]() $\unicode[STIX]{x1D6FC}=0$
, the distribution is essentially symmetric about
$\unicode[STIX]{x1D6FC}=0$
, the distribution is essentially symmetric about
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
. For this case,
$\unicode[STIX]{x1D703}=180^{\circ }$
. For this case,
![]() $\unicode[STIX]{x1D6FE}\approx 0.5$
on the leeward side of the cylinder, indicating transitory separation. Fluctuations about
$\unicode[STIX]{x1D6FE}\approx 0.5$
on the leeward side of the cylinder, indicating transitory separation. Fluctuations about
![]() $\unicode[STIX]{x1D6FE}\approx 0.5$
occur because primary separation is strongly unsteady during the shedding process. As
$\unicode[STIX]{x1D6FE}\approx 0.5$
occur because primary separation is strongly unsteady during the shedding process. As
![]() $\unicode[STIX]{x1D6FC}$
increases, the flow is no longer symmetric. On the bottom side of the cylinder the flow tends to be attached. For
$\unicode[STIX]{x1D6FC}$
increases, the flow is no longer symmetric. On the bottom side of the cylinder the flow tends to be attached. For
![]() $\unicode[STIX]{x1D6FC}=0.48$
, the flow in region II is generally attached, with a peak region at around
$\unicode[STIX]{x1D6FC}=0.48$
, the flow in region II is generally attached, with a peak region at around
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
, which is clearly associated with up-wash and down-wash flow.
$\unicode[STIX]{x1D703}=180^{\circ }$
, which is clearly associated with up-wash and down-wash flow.
In figure 27(b), four cases with
![]() $\unicode[STIX]{x1D6FC}=0.48$
,
$\unicode[STIX]{x1D6FC}=0.48$
,
![]() $0.52$
,
$0.52$
,
![]() $0.56$
and
$0.56$
and
![]() $0.6$
show a different tendency. With increasing
$0.6$
show a different tendency. With increasing
![]() $\unicode[STIX]{x1D6FC}$
, the peak at around
$\unicode[STIX]{x1D6FC}$
, the peak at around
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
weakens, while another peak emerges at approximately
$\unicode[STIX]{x1D703}=180^{\circ }$
weakens, while another peak emerges at approximately
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
. For
$\unicode[STIX]{x1D703}=270^{\circ }$
. For
![]() $\unicode[STIX]{x1D6FC}=0.6$
, that part of region II where
$\unicode[STIX]{x1D6FC}=0.6$
, that part of region II where
![]() $\unicode[STIX]{x1D6FE}\sim 0$
is focused for the most part in the zone
$\unicode[STIX]{x1D6FE}\sim 0$
is focused for the most part in the zone
![]() $250^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, with a relatively sharp peak where
$250^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, with a relatively sharp peak where
![]() $\unicode[STIX]{x1D6FE}$
is close to
$\unicode[STIX]{x1D6FE}$
is close to
![]() $0.5$
. This corresponds to the previously discussed aggregation zone of small-scale separation/reattachment cells, and indicates small-scale but localized transitory separation. With further increase of
$0.5$
. This corresponds to the previously discussed aggregation zone of small-scale separation/reattachment cells, and indicates small-scale but localized transitory separation. With further increase of
![]() $\unicode[STIX]{x1D6FC}$
the
$\unicode[STIX]{x1D6FC}$
the
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
profile does not show new features. The local peak value in region II gradually falls with increasing
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
profile does not show new features. The local peak value in region II gradually falls with increasing
![]() $\unicode[STIX]{x1D6FC}$
.
$\unicode[STIX]{x1D6FC}$
.

Figure 28.
![]() $\unicode[STIX]{x1D6FE}$
for
$\unicode[STIX]{x1D6FE}$
for
![]() $Re_{D}=5\times 10^{3}$
. In (a): ——,
$Re_{D}=5\times 10^{3}$
. In (a): ——,
![]() $\unicode[STIX]{x1D6FC}=0$
; – – – –,
$\unicode[STIX]{x1D6FC}=0$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.2$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.2$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.4$
; — — — —,
$\unicode[STIX]{x1D6FC}=0.4$
; — — — —,
![]() $\unicode[STIX]{x1D6FC}=0.6$
,
— ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.6$
,
— ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.8$
. In (b): — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.8$
. In (b): — ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=0.8$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=0.8$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=1.0$
, – – – –,
$\unicode[STIX]{x1D6FC}=1.0$
, – – – –,
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
It is of interest to consider the
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
plot for the lower
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
plot for the lower
![]() $Re_{D}$
flows. In figure 28 for
$Re_{D}$
flows. In figure 28 for
![]() $Re_{D}=5\times 10^{3}$
, the peak at approximately
$Re_{D}=5\times 10^{3}$
, the peak at approximately
![]() $\unicode[STIX]{x1D703}=270^{\circ }$
found at
$\unicode[STIX]{x1D703}=270^{\circ }$
found at
![]() $Re_{D}=6\times 10^{4}$
at finite
$Re_{D}=6\times 10^{4}$
at finite
![]() $\unicode[STIX]{x1D6FC}$
is not observed. Another interesting difference is the behaviour of
$\unicode[STIX]{x1D6FC}$
is not observed. Another interesting difference is the behaviour of
![]() $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
near primary separation for
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D703})$
near primary separation for
![]() $\unicode[STIX]{x1D6FC}=0$
. For
$\unicode[STIX]{x1D6FC}=0$
. For
![]() $Re_{D}=6\times 10^{4}$
,
$Re_{D}=6\times 10^{4}$
,
![]() $\unicode[STIX]{x1D6FE}$
only reaches
$\unicode[STIX]{x1D6FE}$
only reaches
![]() $\unicode[STIX]{x1D6FE}=0.5$
, which corresponds to 50 % of back flow, implying strong shedding and corresponding movement of the primary-separation line. At
$\unicode[STIX]{x1D6FE}=0.5$
, which corresponds to 50 % of back flow, implying strong shedding and corresponding movement of the primary-separation line. At
![]() $Re_{D}=5\times 10^{3}$
,
$Re_{D}=5\times 10^{3}$
,
![]() $\unicode[STIX]{x1D6FE}$
extends to 100 % of back flow, reaches
$\unicode[STIX]{x1D6FE}$
extends to 100 % of back flow, reaches
![]() $\unicode[STIX]{x1D6FE}=0$
for the flow on the top side of the cylinder, and
$\unicode[STIX]{x1D6FE}=0$
for the flow on the top side of the cylinder, and
![]() $\unicode[STIX]{x1D6FE}=1$
for the flow on the bottom side of the cylinder. This indicates that
$\unicode[STIX]{x1D6FE}=1$
for the flow on the bottom side of the cylinder. This indicates that
![]() $Re_{D}=5\times 10^{3}$
has weak shedding and that the primary-separation lines show little movement.
$Re_{D}=5\times 10^{3}$
has weak shedding and that the primary-separation lines show little movement.
6 Discussion
6.1 Wall-parallel velocity profiles: laminar or turbulent?

Figure 29. Wall-parallel mean-velocity profiles for
![]() $Re_{D}=6\times 10^{4}$
with
$Re_{D}=6\times 10^{4}$
with
![]() $\unicode[STIX]{x1D6FC}=1.0$
. (a) — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=1.0$
. (a) — ⋅ ⋅ —,
![]() $315^{\circ }$
; — ⋅ —,
$315^{\circ }$
; — ⋅ —,
![]() $247.5^{\circ }$
; – – – –,
$247.5^{\circ }$
; – – – –,
![]() $225^{\circ }$
. (b) – – – –,
$225^{\circ }$
. (b) – – – –,
![]() $225^{\circ }$
; ——,
$225^{\circ }$
; ——,
![]() $202.5$
; — ⋅ —,
$202.5$
; — ⋅ —,
![]() $135^{\circ }$
;
— ⋅ ⋅ —,
$135^{\circ }$
;
— ⋅ ⋅ —,
![]() $112.5^{\circ }$
. Two dashed reference lines: linear relationship (
$112.5^{\circ }$
. Two dashed reference lines: linear relationship (
![]() $U_{\unicode[STIX]{x1D703}}^{+}=r^{+}$
) and log relationship (
$U_{\unicode[STIX]{x1D703}}^{+}=r^{+}$
) and log relationship (
![]() $U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
) with
$U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
) with
![]() $k=0.378$
and
$k=0.378$
and
![]() $B=4.08$
.
$B=4.08$
.
For flow over a non-rotating cylinder, near-wall laminar–turbulence transition first occurs on one side of the body within the upper portion of a ‘prior’ separation bubble for
![]() $Re_{D}>3.5\times 10^{5}$
. In the sense of increasing
$Re_{D}>3.5\times 10^{5}$
. In the sense of increasing
![]() $Re_{D}$
, this just follows the drag crisis, continuing to
$Re_{D}$
, this just follows the drag crisis, continuing to
![]() $Re_{D}\approx 10^{6}$
before the beginning of the trans-critical flow regime (Lehmkuhl et al.
Reference Lehmkuhl, Rodríguez, Borrell, Chiva and Oliva2014; Cheng et al.
Reference Cheng, Pullin, Samtaney, Zhang and Gao2017). For higher
$Re_{D}\approx 10^{6}$
before the beginning of the trans-critical flow regime (Lehmkuhl et al.
Reference Lehmkuhl, Rodríguez, Borrell, Chiva and Oliva2014; Cheng et al.
Reference Cheng, Pullin, Samtaney, Zhang and Gao2017). For higher
![]() $Re_{D}$
, the transition zone migrates to the cylinder surface as the prior separation bubble disappears (Roshko Reference Roshko1961). For the flow over a rotating cylinder, turbulence transition is difficult to characterize within the
$Re_{D}$
, the transition zone migrates to the cylinder surface as the prior separation bubble disappears (Roshko Reference Roshko1961). For the flow over a rotating cylinder, turbulence transition is difficult to characterize within the
![]() $(Re_{D}{-}\unicode[STIX]{x1D6FC})$
plane. The experimental data of Aldoss & Abou-Arab (Reference Aldoss and Abou-Arab1990) shows boundary-layer transition at
$(Re_{D}{-}\unicode[STIX]{x1D6FC})$
plane. The experimental data of Aldoss & Abou-Arab (Reference Aldoss and Abou-Arab1990) shows boundary-layer transition at
![]() $\unicode[STIX]{x1D6FC}=1.29$
for
$\unicode[STIX]{x1D6FC}=1.29$
for
![]() $Re_{D}=4.42\times 10^{4}$
while Peller (Reference Peller1986) observes turbulence transition at
$Re_{D}=4.42\times 10^{4}$
while Peller (Reference Peller1986) observes turbulence transition at
![]() $\unicode[STIX]{x1D6FC}=1.0$
for
$\unicode[STIX]{x1D6FC}=1.0$
for
![]() $Re_{D}=4.8\times 10^{4}$
. It is therefore of interest to investigate turbulence transition in the present LES in order to clarify its role in the lift crisis.
$Re_{D}=4.8\times 10^{4}$
. It is therefore of interest to investigate turbulence transition in the present LES in order to clarify its role in the lift crisis.
6.1.1 Turbulent boundary profiles at
 $Re_{D}=6\times 10^{4}$
$Re_{D}=6\times 10^{4}$
We begin with
![]() $Re_{D}=6\times 10^{4}$
with
$Re_{D}=6\times 10^{4}$
with
![]() $\unicode[STIX]{x1D6FC}=1.0$
which, according to the reference experiments, might be expected to show turbulence transition on the cylinder surface. Figure 29 shows the wall-parallel, mean-velocity boundary-layer profiles on the bottom side of the cylinder where turbulent transition is expected to first occur. In this plot
$\unicode[STIX]{x1D6FC}=1.0$
which, according to the reference experiments, might be expected to show turbulence transition on the cylinder surface. Figure 29 shows the wall-parallel, mean-velocity boundary-layer profiles on the bottom side of the cylinder where turbulent transition is expected to first occur. In this plot
![]() $U_{\unicode[STIX]{x1D703}}^{+}\equiv U_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}/u_{\unicode[STIX]{x1D70F}}$
, with
$U_{\unicode[STIX]{x1D703}}^{+}\equiv U_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}/u_{\unicode[STIX]{x1D70F}}$
, with
![]() $U_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}(\unicode[STIX]{x1D703},r)$
the spanwise–time average of
$U_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}(\unicode[STIX]{x1D703},r)$
the spanwise–time average of
![]() $u_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}(\unicode[STIX]{x1D703},y,r,t)$
(see (4.1)) and
$u_{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D703}}(\unicode[STIX]{x1D703},y,r,t)$
(see (4.1)) and
![]() $r^{+}\equiv (r-D/2)u_{\unicode[STIX]{x1D70F}}/\unicode[STIX]{x1D708}$
. Six
$r^{+}\equiv (r-D/2)u_{\unicode[STIX]{x1D70F}}/\unicode[STIX]{x1D708}$
. Six
![]() $\unicode[STIX]{x1D703}$
locations are chosen along the direction of flow:
$\unicode[STIX]{x1D703}$
locations are chosen along the direction of flow:
![]() $\unicode[STIX]{x1D703}=315^{\circ }$
,
$\unicode[STIX]{x1D703}=315^{\circ }$
,
![]() $247.5^{\circ }$
,
$247.5^{\circ }$
,
![]() $225^{\circ }$
,
$225^{\circ }$
,
![]() $202.5^{\circ }$
,
$202.5^{\circ }$
,
![]() $135^{\circ }$
,
$135^{\circ }$
,
![]() $112.5^{\circ }$
. Two reference lines are also plotted in figure 29: one is a linear relationship while the other is a log relationship
$112.5^{\circ }$
. Two reference lines are also plotted in figure 29: one is a linear relationship while the other is a log relationship
![]() $U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
with
$U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
with
![]() $k=0.378$
and
$k=0.378$
and
![]() $B=4.08$
. Up to
$B=4.08$
. Up to
![]() $\unicode[STIX]{x1D703}=247.5^{\circ }$
, the velocity profile is still laminar, but at
$\unicode[STIX]{x1D703}=247.5^{\circ }$
, the velocity profile is still laminar, but at
![]() $\unicode[STIX]{x1D703}=225^{\circ }$
, a clear log-layer can be seen. This log-layer persists up to
$\unicode[STIX]{x1D703}=225^{\circ }$
, a clear log-layer can be seen. This log-layer persists up to
![]() $\unicode[STIX]{x1D703}=202.5^{\circ }$
. Further downstream the velocity profile becomes gradually lifted owing to an adverse pressure gradient, tending to show separation behaviour at
$\unicode[STIX]{x1D703}=202.5^{\circ }$
. Further downstream the velocity profile becomes gradually lifted owing to an adverse pressure gradient, tending to show separation behaviour at
![]() $\unicode[STIX]{x1D703}=112.5^{\circ }$
.
$\unicode[STIX]{x1D703}=112.5^{\circ }$
.

Figure 30. Wall-parallel mean-velocity profiles for
![]() $Re_{D}=6\times 10^{4}$
with
$Re_{D}=6\times 10^{4}$
with
![]() $\unicode[STIX]{x1D6FC}=0.48$
. (a) — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.48$
. (a) — ⋅ ⋅ —,
![]() $315^{\circ }$
; — ⋅ —,
$315^{\circ }$
; — ⋅ —,
![]() $247.5^{\circ }$
; – – – –,
$247.5^{\circ }$
; – – – –,
![]() $225^{\circ }$
. (b) – – – –,
$225^{\circ }$
. (b) – – – –,
![]() $225^{\circ }$
——,
$225^{\circ }$
——,
![]() $160^{\circ }$
; — ⋅ —,
$160^{\circ }$
; — ⋅ —,
![]() $135^{\circ }$
;
$135^{\circ }$
;
![]() $\cdots \cdots$
,
$\cdots \cdots$
,
![]() $112.5^{\circ }$
. Two dashed reference lines: linear relationship (
$112.5^{\circ }$
. Two dashed reference lines: linear relationship (
![]() $U_{\unicode[STIX]{x1D703}}^{+}=r^{+}$
) and log relationship (
$U_{\unicode[STIX]{x1D703}}^{+}=r^{+}$
) and log relationship (
![]() $U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
) with
$U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
) with
![]() $k=0.378$
and
$k=0.378$
and
![]() $B=4.08$
.
$B=4.08$
.

Figure 31. Wall-parallel mean-velocity profiles for
![]() $Re_{D}=6\times 10^{4}$
with
$Re_{D}=6\times 10^{4}$
with
![]() $\unicode[STIX]{x1D6FC}=0.6$
. (a) — ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0.6$
. (a) — ⋅ ⋅ —,
![]() $315^{\circ }$
, — ⋅ —,
$315^{\circ }$
, — ⋅ —,
![]() $247.5^{\circ }$
; – – – –,
$247.5^{\circ }$
; – – – –,
![]() $225^{\circ }$
. (b) – – – –,
$225^{\circ }$
. (b) – – – –,
![]() $225^{\circ }$
——,
$225^{\circ }$
——,
![]() $160^{\circ }$
; — ⋅ —,
$160^{\circ }$
; — ⋅ —,
![]() $135^{\circ }$
;
$135^{\circ }$
;
![]() $\cdots \cdots$
,
$\cdots \cdots$
,
![]() $112.5^{\circ }$
. Two dashed reference lines: linear relationship (
$112.5^{\circ }$
. Two dashed reference lines: linear relationship (
![]() $U_{\unicode[STIX]{x1D703}}^{+}=r^{+}$
) and log relationship (
$U_{\unicode[STIX]{x1D703}}^{+}=r^{+}$
) and log relationship (
![]() $U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
) with
$U_{\unicode[STIX]{x1D703}}^{+}=\ln (r^{+})/k+B$
) with
![]() $k=0.378$
and
$k=0.378$
and
![]() $B=4.08$
.
$B=4.08$
.
Velocity profiles for
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
at
$\unicode[STIX]{x1D6FC}=0.6$
at
![]() $Re_{D}=6\times 10^{4}$
, are shown in figures 30 and 31, respectively, for six
$Re_{D}=6\times 10^{4}$
, are shown in figures 30 and 31, respectively, for six
![]() $\unicode[STIX]{x1D703}$
locations. For both flows the evolution of the velocity profiles in the near-wall flow direction is initially somewhat similar to the
$\unicode[STIX]{x1D703}$
locations. For both flows the evolution of the velocity profiles in the near-wall flow direction is initially somewhat similar to the
![]() $\unicode[STIX]{x1D6FC}=1$
flow. Both cases show weak evidence of a log-layer present at
$\unicode[STIX]{x1D6FC}=1$
flow. Both cases show weak evidence of a log-layer present at
![]() $160^{\circ }$
, but this is not persistent as for the case
$160^{\circ }$
, but this is not persistent as for the case
![]() $\unicode[STIX]{x1D6FC}=1$
. We can conclude that a fully developed turbulent boundary layer is not found for either
$\unicode[STIX]{x1D6FC}=1$
. We can conclude that a fully developed turbulent boundary layer is not found for either
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
. In particular, no significant differences in near-wall turbulence characteristics are observed for these two flows.
$\unicode[STIX]{x1D6FC}=0.6$
. In particular, no significant differences in near-wall turbulence characteristics are observed for these two flows.
6.2 The lift crisis
Although no quantification exists of critical-point area density in the recirculation region for flow over a stationary cylinder, an intuitively reasonable hypothesis is that the mean distance between critical points, if statistically measurable, should decrease with increasing
![]() $Re_{D}$
. For high-
$Re_{D}$
. For high-
![]() $Re_{D}$
flow over the stationary cylinder, the surface skin-friction images of Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) at
$Re_{D}$
flow over the stationary cylinder, the surface skin-friction images of Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) at
![]() $Re_{D}=10^{4},10^{5}$
show some indication of an aggregation small-scale separation/reattachment cells, and hence critical points with increasing
$Re_{D}=10^{4},10^{5}$
show some indication of an aggregation small-scale separation/reattachment cells, and hence critical points with increasing
![]() $Re_{D}$
, within two zones, one on each side of the cylinder. Between these zones, their figure 26 shows a strong washing flow which is interpreted as the agent of a dip in the mean-flow pressure distribution at around
$Re_{D}$
, within two zones, one on each side of the cylinder. Between these zones, their figure 26 shows a strong washing flow which is interpreted as the agent of a dip in the mean-flow pressure distribution at around
![]() $\unicode[STIX]{x1D703}=180^{\circ }$
. Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) suggest that the critical-point aggregation zones form the fluctuating, area-distributed separation region of an intermediate-scale, mean-flow structure called a secondary-separation bubble, one of which exists on each side of the cylinder at subcritical
$\unicode[STIX]{x1D703}=180^{\circ }$
. Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) suggest that the critical-point aggregation zones form the fluctuating, area-distributed separation region of an intermediate-scale, mean-flow structure called a secondary-separation bubble, one of which exists on each side of the cylinder at subcritical
![]() $Re_{D}$
. As
$Re_{D}$
. As
![]() $Re_{D}$
increases this secondary-separation bubble together with its reattachment, surface-streamline bundles moves upstream, dynamically interacting with the primary-separation shear layer. Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) argue that this interaction becomes sufficiently strong to produce an almost discontinuous (in
$Re_{D}$
increases this secondary-separation bubble together with its reattachment, surface-streamline bundles moves upstream, dynamically interacting with the primary-separation shear layer. Cheng et al. (Reference Cheng, Pullin, Samtaney, Zhang and Gao2017) argue that this interaction becomes sufficiently strong to produce an almost discontinuous (in
![]() $Re_{D}$
) transition of the global flow structure characterized by a strong rearward shift in the primary-separation line. This is the drag crisis. It is accompanied by vanishing of the secondary-separation bubble and the appearance of a prior bubble upstream of primary-flow separation.
$Re_{D}$
) transition of the global flow structure characterized by a strong rearward shift in the primary-separation line. This is the drag crisis. It is accompanied by vanishing of the secondary-separation bubble and the appearance of a prior bubble upstream of primary-flow separation.
For the rotating-cylinder flow, for example at
![]() $\unicode[STIX]{x1D6FC}=0.6$
, a phenomenon associated with the small-scale separation/reattachment cells can also be observed, but with different effects. When the aggregation of small-scale separation/reattachment cells forms near the bottom side of the cylinder in
$\unicode[STIX]{x1D6FC}=0.6$
, a phenomenon associated with the small-scale separation/reattachment cells can also be observed, but with different effects. When the aggregation of small-scale separation/reattachment cells forms near the bottom side of the cylinder in
![]() $240^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, the attached flow, after dynamical interaction with these separation/reattachment cells, behaves like an adverse pressure gradient flow and forms a low-pressure region. In the rotating-cylinder flow, since there is no primary separation for the flow on the bottom side of the cylinder, this low-pressure region then leads to a suddenly reduced
$240^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, the attached flow, after dynamical interaction with these separation/reattachment cells, behaves like an adverse pressure gradient flow and forms a low-pressure region. In the rotating-cylinder flow, since there is no primary separation for the flow on the bottom side of the cylinder, this low-pressure region then leads to a suddenly reduced
![]() $C_{L}$
, which is the lift crisis. The crisis is essentially conditioned by the rapid aggregation of wide-area patches of critical points into the relatively narrow band over a quite small range of
$C_{L}$
, which is the lift crisis. The crisis is essentially conditioned by the rapid aggregation of wide-area patches of critical points into the relatively narrow band over a quite small range of
![]() $Re_{D}$
.
$Re_{D}$
.
We have argued that, at
![]() $Re_{D}=6\times 10^{4}$
, the lift crisis itself is a result of flow reorganization on the under and leeward parts of the cylinder surface where an aggregation region of small-scale separation/reattachment cells forms in the range
$Re_{D}=6\times 10^{4}$
, the lift crisis itself is a result of flow reorganization on the under and leeward parts of the cylinder surface where an aggregation region of small-scale separation/reattachment cells forms in the range
![]() $\unicode[STIX]{x1D6FC}=0.48{-}0.6$
. The aggregation zone forms an ordered dividing line separating upstream, almost two-dimensional oncoming flow, from downstream small-scale reversal flows. For even higher
$\unicode[STIX]{x1D6FC}=0.48{-}0.6$
. The aggregation zone forms an ordered dividing line separating upstream, almost two-dimensional oncoming flow, from downstream small-scale reversal flows. For even higher
![]() $Re_{D}$
, especially at those values where boundary-layer turbulent transition already exists with
$Re_{D}$
, especially at those values where boundary-layer turbulent transition already exists with
![]() $\unicode[STIX]{x1D6FC}=0$
, the flow could be more complex. For
$\unicode[STIX]{x1D6FC}=0$
, the flow could be more complex. For
![]() $Re_{D}$
around the drag crisis range in the static cylinder case, the cylinder rotation at even small
$Re_{D}$
around the drag crisis range in the static cylinder case, the cylinder rotation at even small
![]() $\unicode[STIX]{x1D6FC}$
results in the coalescence of the prior separation bubble and the primary separation, which is directly followed by the flow reorganization phenomenon discussed previously. For even higher
$\unicode[STIX]{x1D6FC}$
results in the coalescence of the prior separation bubble and the primary separation, which is directly followed by the flow reorganization phenomenon discussed previously. For even higher
![]() $Re_{D}$
in the supercritical regime, turbulence transition still takes place on the prior separation bubble and, at some finite
$Re_{D}$
in the supercritical regime, turbulence transition still takes place on the prior separation bubble and, at some finite
![]() $\unicode[STIX]{x1D6FC}$
, is absorbed by the primary separation.
$\unicode[STIX]{x1D6FC}$
, is absorbed by the primary separation.
7 Conclusion
The present study uses wall-resolved large-eddy simulation (LES) in order to provide insight into our understanding of the lift crisis in the flow over a cylinder rotating with constant angular velocity. Here the stretched-spiral vortex SGS model is embedded in a fourth-order curvilinear coordinate code. Cases implemented include an LES set at a lower
![]() $Re_{D}$
at
$Re_{D}$
at
![]() $5\times 10^{3}$
, used both for code verification and also to demonstrate flow behaviour in the absence of a lift crisis, while the LES set at the higher
$5\times 10^{3}$
, used both for code verification and also to demonstrate flow behaviour in the absence of a lift crisis, while the LES set at the higher
![]() $Re_{D}=6\times 10^{4}$
,
$Re_{D}=6\times 10^{4}$
,
![]() $0\leqslant \unicode[STIX]{x1D6FC}\leqslant 2$
provides the basis for the present study. Profiles of both the pressure coefficient
$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 2$
provides the basis for the present study. Profiles of both the pressure coefficient
![]() $C_{p}$
and the skin-friction coefficient
$C_{p}$
and the skin-friction coefficient
![]() $C_{f\unicode[STIX]{x1D703}}$
clearly illustrate the presence of a lift crisis from
$C_{f\unicode[STIX]{x1D703}}$
clearly illustrate the presence of a lift crisis from
![]() $\unicode[STIX]{x1D6FC}=0.48$
to
$\unicode[STIX]{x1D6FC}=0.48$
to
![]() $\unicode[STIX]{x1D6FC}=0.6$
, where
$\unicode[STIX]{x1D6FC}=0.6$
, where
![]() $\unicode[STIX]{x1D6FC}$
is the non-dimensional rotation speed. This range agrees well with experimental data.
$\unicode[STIX]{x1D6FC}$
is the non-dimensional rotation speed. This range agrees well with experimental data.
Detailed flow studies are presented for
![]() $Re_{D}=6\times 10^{4}$
with
$Re_{D}=6\times 10^{4}$
with
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
. Instantaneous surface skin-friction portraits indicate that the movement of near-wall, small-scale reversal flows with increasing
$\unicode[STIX]{x1D6FC}=0.6$
. Instantaneous surface skin-friction portraits indicate that the movement of near-wall, small-scale reversal flows with increasing
![]() $\unicode[STIX]{x1D6FC}$
through the lift crisis is a critical flow phenomenon. At
$\unicode[STIX]{x1D6FC}$
through the lift crisis is a critical flow phenomenon. At
![]() $\unicode[STIX]{x1D6FC}=0.48$
, the area distribution of small-scale separation/reattachment cells on the cylinder under surface is divided into two loosely scattered groups separated by regions of attached, sloshing flow, presently termed ‘washing flow’. The overall under-surface flow is chaotic, with fluctuating, local separation, and small-scale vortex shedding. At
$\unicode[STIX]{x1D6FC}=0.48$
, the area distribution of small-scale separation/reattachment cells on the cylinder under surface is divided into two loosely scattered groups separated by regions of attached, sloshing flow, presently termed ‘washing flow’. The overall under-surface flow is chaotic, with fluctuating, local separation, and small-scale vortex shedding. At
![]() $\unicode[STIX]{x1D6FC}=0.6$
, a flow reorganization has occurred where the small-scale separation/reattachment cells become aggregated within a region of around
$\unicode[STIX]{x1D6FC}=0.6$
, a flow reorganization has occurred where the small-scale separation/reattachment cells become aggregated within a region of around
![]() $240^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, with
$240^{\circ }<\unicode[STIX]{x1D703}<280^{\circ }$
, with
![]() $\unicode[STIX]{x1D703}$
measured in the direction of cylinder rotation from the windward geometrical centreline of the cylinder surface. It is apparent from the skin-friction portraits that this zone is coherent in the spanwise direction, consisting of broadly unidirectional, mainly attached flow, as opposed to washing flow.
$\unicode[STIX]{x1D703}$
measured in the direction of cylinder rotation from the windward geometrical centreline of the cylinder surface. It is apparent from the skin-friction portraits that this zone is coherent in the spanwise direction, consisting of broadly unidirectional, mainly attached flow, as opposed to washing flow.
This flow scenario is supported by streamlines of the instantaneous, but spanwise-averaged flow field, the latter constructed by subtraction of the solid-body rotation field so that surface skin-friction lines are the wall limit of instantaneous streamlines. Streamlines of the spanwise-averaged velocity field at
![]() $\unicode[STIX]{x1D6FC}=0.48$
clearly show the pinch-off of vortices, including a wall-attached separation bubble, which rotates in an anticlockwise direction and is a generator of the wash flow, and an off-wall clockwise vortex. In contrast, for
$\unicode[STIX]{x1D6FC}=0.48$
clearly show the pinch-off of vortices, including a wall-attached separation bubble, which rotates in an anticlockwise direction and is a generator of the wash flow, and an off-wall clockwise vortex. In contrast, for
![]() $\unicode[STIX]{x1D6FC}=0.6$
, no obvious vortex is generated, which is consistent with the largely attached surface flow and disappearance of the wash flow. This suggests the hypothesis that the aggregation of the small-scale reversal flows, identified on the plot of skin-friction lines as small-scale separation/reattachment cells, is the dominant signature of flow reorganization associated with the onset of the lift crisis.
$\unicode[STIX]{x1D6FC}=0.6$
, no obvious vortex is generated, which is consistent with the largely attached surface flow and disappearance of the wash flow. This suggests the hypothesis that the aggregation of the small-scale reversal flows, identified on the plot of skin-friction lines as small-scale separation/reattachment cells, is the dominant signature of flow reorganization associated with the onset of the lift crisis.
We further construct a joint probability distribution of
![]() $C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703}$
on the cylinder surface from which we define a parameter
$C_{f\unicode[STIX]{x1D703}},\unicode[STIX]{x1D703}$
on the cylinder surface from which we define a parameter
![]() $\unicode[STIX]{x1D6FE}$
which can be interpreted as a statistical measure of the extent of surface separation/reattachment. The azimuthal distribution of
$\unicode[STIX]{x1D6FE}$
which can be interpreted as a statistical measure of the extent of surface separation/reattachment. The azimuthal distribution of
![]() $\unicode[STIX]{x1D6FE}$
clearly shows a region of incipient transitory separation around the bottom (retreating) of the cylinder for cases after the lift crisis, while this phenomenon is not observed for cases before the lift crisis.
$\unicode[STIX]{x1D6FE}$
clearly shows a region of incipient transitory separation around the bottom (retreating) of the cylinder for cases after the lift crisis, while this phenomenon is not observed for cases before the lift crisis.
In order to explore the issue of boundary-layer laminar–turbulent transition, we have studied the wall-parallel velocity profiles for three flow cases at
![]() $Re_{D}=6\times 10^{4}$
. While profiles with
$Re_{D}=6\times 10^{4}$
. While profiles with
![]() $\unicode[STIX]{x1D6FC}=1$
clearly show log-law behaviour characteristic of a turbulent boundary layer, for
$\unicode[STIX]{x1D6FC}=1$
clearly show log-law behaviour characteristic of a turbulent boundary layer, for
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
, log-law type velocity profiles cannot be clearly found. This is taken to indicate that boundary-layer turbulent transition does not play a role in the lift crisis, at least for
$\unicode[STIX]{x1D6FC}=0.6$
, log-law type velocity profiles cannot be clearly found. This is taken to indicate that boundary-layer turbulent transition does not play a role in the lift crisis, at least for
![]() $Re_{D}=6\times 10^{4}$
.
$Re_{D}=6\times 10^{4}$
.
In summary, visualization of the
![]() $\boldsymbol{C}_{\boldsymbol{f}}$
portraits through a lift cycle together with instantaneous but spanwise-averaged streamline plots of the modified velocity field
$\boldsymbol{C}_{\boldsymbol{f}}$
portraits through a lift cycle together with instantaneous but spanwise-averaged streamline plots of the modified velocity field
![]() $\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(r,\unicode[STIX]{x1D703},y,t)$
suggests that, at
$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}(r,\unicode[STIX]{x1D703},y,t)$
suggests that, at
![]() $Re_{D}=6\times 10^{4}$
, the flow on the leeward-bottom surface of the cylinder undergoes a dramatic reorganization. This consists of a transition of patches of scattered, small-scale separation/reattachment cells at
$Re_{D}=6\times 10^{4}$
, the flow on the leeward-bottom surface of the cylinder undergoes a dramatic reorganization. This consists of a transition of patches of scattered, small-scale separation/reattachment cells at
![]() $\unicode[STIX]{x1D6FC}=0.48$
, separated by regions of wash-type flow and accompanied by separation events sufficiently strong to form both wall-attached and fully separated vortices, to a more ordered state at
$\unicode[STIX]{x1D6FC}=0.48$
, separated by regions of wash-type flow and accompanied by separation events sufficiently strong to form both wall-attached and fully separated vortices, to a more ordered state at
![]() $\unicode[STIX]{x1D6FC}=0.6$
. Here the small-scale separation/reattachment events aggregate into a relatively narrow zone producing both small-scale incoherent separation accompanied by a deep pressure minimum. Hence the lift crisis. The detailed dynamical mechanism of this transition is probably conditioned by the presence of enhanced rotation on the near-wall boundary-layer dynamics.
$\unicode[STIX]{x1D6FC}=0.6$
. Here the small-scale separation/reattachment events aggregate into a relatively narrow zone producing both small-scale incoherent separation accompanied by a deep pressure minimum. Hence the lift crisis. The detailed dynamical mechanism of this transition is probably conditioned by the presence of enhanced rotation on the near-wall boundary-layer dynamics.
Acknowledgements
This work was partially supported by the KAUST baseline research funds of R.S. The Cray XC40, Shaheen, at KAUST was utilized for all the reported LES.
Appendix A. Numerical verification

Figure 32. Numerical verification at
![]() $Re_{D}=5\times 10^{3}$
. (a) Pressure coefficient
$Re_{D}=5\times 10^{3}$
. (a) Pressure coefficient
![]() $C_{p}$
; (b) skin-friction coefficient
$C_{p}$
; (b) skin-friction coefficient
![]() $C_{f\unicode[STIX]{x1D703}}$
. Symbols for DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015): ▫,
$C_{f\unicode[STIX]{x1D703}}$
. Symbols for DNS by Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015): ▫,
![]() $\unicode[STIX]{x1D6FC}=0$
; ▵,
$\unicode[STIX]{x1D6FC}=0$
; ▵,
![]() $\unicode[STIX]{x1D6FC}=1.0$
; ▿,
$\unicode[STIX]{x1D6FC}=1.0$
; ▿,
![]() $\unicode[STIX]{x1D6FC}=2.0$
. Lines for present LES: ——,
$\unicode[STIX]{x1D6FC}=2.0$
. Lines for present LES: ——,
![]() $\unicode[STIX]{x1D6FC}=0$
; – – – –,
$\unicode[STIX]{x1D6FC}=0$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=1.0$
; — ⋅ —,
$\unicode[STIX]{x1D6FC}=1.0$
; — ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
For verification purposes, we consider the reference DNS of Aljure et al. (Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) at
![]() $Re_{D}=5\times 10^{3}$
, which utilized an unstructured mesh consisting of approximately
$Re_{D}=5\times 10^{3}$
, which utilized an unstructured mesh consisting of approximately
![]() $40\times 10^{6}$
cells with
$40\times 10^{6}$
cells with
![]() $320$
cells in the spanwise direction. For the present LES, the total mesh count is approximately
$320$
cells in the spanwise direction. For the present LES, the total mesh count is approximately
![]() $4\times 10^{6}$
with
$4\times 10^{6}$
with
![]() $64$
spanwise cells. We first compare, in figure 32, the present LES profiles for both
$64$
spanwise cells. We first compare, in figure 32, the present LES profiles for both
![]() $C_{p}$
and
$C_{p}$
and
![]() $C_{f\unicode[STIX]{x1D703}}$
with the reference DNS results at
$C_{f\unicode[STIX]{x1D703}}$
with the reference DNS results at
![]() $Re_{D}=5\times 10^{3}$
,
$Re_{D}=5\times 10^{3}$
,
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $1.0$
and
$1.0$
and
![]() $2.0$
. This is considered satisfactory. In table 6, we list the lift and drag coefficients and also the lift coefficient fluctuations for both DNS and LES. The drag coefficient fluctuation
$2.0$
. This is considered satisfactory. In table 6, we list the lift and drag coefficients and also the lift coefficient fluctuations for both DNS and LES. The drag coefficient fluctuation
![]() $C_{Drms}$
for LES is also shown.
$C_{Drms}$
for LES is also shown.
Table 6. Comparison of DNS data (Aljure et al.
Reference Aljure, Rodríguez, Lehmkuhl, Pérez-Segarra and Oliva2015) and LES results: lift coefficient
![]() $C_{L}$
, drag coefficient
$C_{L}$
, drag coefficient
![]() $C_{D}$
, lift coefficient fluctuation
$C_{D}$
, lift coefficient fluctuation
![]() $C_{Lrms}$
and drag coefficient fluctuation
$C_{Lrms}$
and drag coefficient fluctuation
![]() $C_{Drms}$
.
$C_{Drms}$
.


Figure 33. Wall-adjacent cell size in wall units:
![]() $\unicode[STIX]{x0394}r^{+}$
. (a)
$\unicode[STIX]{x0394}r^{+}$
. (a)
![]() $Re_{D}=5\times 10^{3}$
; (b)
$Re_{D}=5\times 10^{3}$
; (b)
![]() $Re_{D}=6\times 10^{4}$
. – – – –,
$Re_{D}=6\times 10^{4}$
. – – – –,
![]() $\unicode[STIX]{x1D6FC}=0$
;
— ⋅ ⋅ —,
$\unicode[STIX]{x1D6FC}=0$
;
— ⋅ ⋅ —,
![]() $\unicode[STIX]{x1D6FC}=1.0$
; ——,
$\unicode[STIX]{x1D6FC}=1.0$
; ——,
![]() $\unicode[STIX]{x1D6FC}=2.0$
.
$\unicode[STIX]{x1D6FC}=2.0$
.
A reasonable verification metric for wall-bounded LES is the wall-normal scale of the near-wall mesh in inner viscous units, defined as
![]() $\unicode[STIX]{x0394}r^{+}=u_{\unicode[STIX]{x1D70F}}\unicode[STIX]{x0394}r/\unicode[STIX]{x1D708}$
. Figure 33(a,b) show
$\unicode[STIX]{x0394}r^{+}=u_{\unicode[STIX]{x1D70F}}\unicode[STIX]{x0394}r/\unicode[STIX]{x1D708}$
. Figure 33(a,b) show
![]() $\unicode[STIX]{x0394}r^{+}(\unicode[STIX]{x1D703})$
at
$\unicode[STIX]{x0394}r^{+}(\unicode[STIX]{x1D703})$
at
![]() $Re_{D}=5\times 10^{3}$
and
$Re_{D}=5\times 10^{3}$
and
![]() $Re_{D}=6\times 10^{4}$
, respectively, for
$Re_{D}=6\times 10^{4}$
, respectively, for
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $1.0$
and
$1.0$
and
![]() $2.0$
. For all cases the maximum
$2.0$
. For all cases the maximum
![]() $\unicode[STIX]{x0394}r^{+}$
are
$\unicode[STIX]{x0394}r^{+}$
are
![]() $O(1)$
or smaller. For
$O(1)$
or smaller. For
![]() $Re_{D}=6\times 10^{4}$
with
$Re_{D}=6\times 10^{4}$
with
![]() $\unicode[STIX]{x1D6FC}=1$
,
$\unicode[STIX]{x1D6FC}=1$
,
![]() $\unicode[STIX]{x0394}r^{+}\approx 0.5$
on the back side of the cylinder, which corresponds to the observed turbulent boundary-layer region.
$\unicode[STIX]{x0394}r^{+}\approx 0.5$
on the back side of the cylinder, which corresponds to the observed turbulent boundary-layer region.
For the spanwise domain size, a common test is a posteriori spanwise two-point correlation analysis. This has been documented in many wall-bounded flows, such as airfoil (Zhang & Samtaney Reference Zhang and Samtaney2016) and cylinder flows at high
![]() $Re_{D}$
(Lehmkuhl et al.
Reference Lehmkuhl, Rodríguez, Borrell, Chiva and Oliva2014). Here we compute the two-point correlation for the spanwise velocity component
$Re_{D}$
(Lehmkuhl et al.
Reference Lehmkuhl, Rodríguez, Borrell, Chiva and Oliva2014). Here we compute the two-point correlation for the spanwise velocity component
![]() $v$
, defined as
$v$
, defined as
where the overline denotes a time average. In figure 34, we plot
![]() $R_{vv}$
for several cases at
$R_{vv}$
for several cases at
![]() $(x,z)=(2D,0)$
, including
$(x,z)=(2D,0)$
, including
![]() $\unicode[STIX]{x1D6FC}=0$
,
$\unicode[STIX]{x1D6FC}=0$
,
![]() $1.0$
at
$1.0$
at
![]() $Re_{D}=5\times 10^{3}$
in figure 34(a) and
$Re_{D}=5\times 10^{3}$
in figure 34(a) and
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $0.6$
at
$0.6$
at
![]() $Re_{D}=6\times 10^{4}$
in figure 34(b).
$Re_{D}=6\times 10^{4}$
in figure 34(b).

Figure 34. Spanwise two-point correlation of spanwise velocity:
![]() $R_{vv}$
. (a)
$R_{vv}$
. (a)
![]() $Re_{D}=5\times 10^{3}$
. ——,
$Re_{D}=5\times 10^{3}$
. ——,
![]() $\unicode[STIX]{x1D6FC}=0$
; – – – –,
$\unicode[STIX]{x1D6FC}=0$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=1$
. (b)
$\unicode[STIX]{x1D6FC}=1$
. (b)
![]() $Re_{D}=6\times 10^{4}$
. ——,
$Re_{D}=6\times 10^{4}$
. ——,
![]() $\unicode[STIX]{x1D6FC}=0.48$
; – – – –,
$\unicode[STIX]{x1D6FC}=0.48$
; – – – –,
![]() $\unicode[STIX]{x1D6FC}=0.6$
.
$\unicode[STIX]{x1D6FC}=0.6$
.

Figure 35. Mesh verification case of
![]() $\unicode[STIX]{x1D6FC}=0.48$
at
$\unicode[STIX]{x1D6FC}=0.48$
at
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $C_{p}$
; (b)
$C_{p}$
; (b)
![]() $C_{f\unicode[STIX]{x1D703}}$
. – – – –, coarse mesh; ——, fine mesh.
$C_{f\unicode[STIX]{x1D703}}$
. – – – –, coarse mesh; ——, fine mesh.

Figure 36. Mesh verification case of
![]() $\unicode[STIX]{x1D6FC}=0.6$
at
$\unicode[STIX]{x1D6FC}=0.6$
at
![]() $Re_{D}=6\times 10^{4}$
. (a)
$Re_{D}=6\times 10^{4}$
. (a)
![]() $C_{p}$
; (b)
$C_{p}$
; (b)
![]() $C_{f\unicode[STIX]{x1D703}}$
. – – – –, coarse mesh; ——, fine mesh.
$C_{f\unicode[STIX]{x1D703}}$
. – – – –, coarse mesh; ——, fine mesh.
Since the main theme of present LES is investigation of the lift crisis phenomenon, mesh-convergence verification is mainly carried out for two cases,
![]() $\unicode[STIX]{x1D6FC}=0.48$
and
$\unicode[STIX]{x1D6FC}=0.48$
and
![]() $\unicode[STIX]{x1D6FC}=0.6$
. In figures 35 and 36, we compare both
$\unicode[STIX]{x1D6FC}=0.6$
. In figures 35 and 36, we compare both
![]() $C_{p}$
and
$C_{p}$
and
![]() $C_{f\unicode[STIX]{x1D703}}$
for LES using both a coarser mesh with
$C_{f\unicode[STIX]{x1D703}}$
for LES using both a coarser mesh with
![]() $(N_{\unicode[STIX]{x1D703}},N_{y},N_{r})=(2048,192,256)$
and a finer mesh with
$(N_{\unicode[STIX]{x1D703}},N_{y},N_{r})=(2048,192,256)$
and a finer mesh with
![]() $(N_{\unicode[STIX]{x1D703}},N_{y},N_{r})=(4096,384,512)$
. For the two
$(N_{\unicode[STIX]{x1D703}},N_{y},N_{r})=(4096,384,512)$
. For the two
![]() $\unicode[STIX]{x1D6FC}$
cases, mesh refinement does not show substantial differences, indicating that our coarser-mesh results are converged.
$\unicode[STIX]{x1D6FC}$
cases, mesh refinement does not show substantial differences, indicating that our coarser-mesh results are converged.
Appendix B. Subgrid scale model
For wall-resolved LES we utilize the stretched-vortex (SV) SGS model (Misra & Pullin Reference Misra and Pullin1997; Voelkl et al.
Reference Voelkl, Pullin and Chan2000; Chung & Pullin Reference Chung and Pullin2009), where the subgrid flow is represented by tube/sheet-like structures that are modelled as spiral vortices (Lundgren Reference Lundgren1982) stretched by the eddies comprising the local resolved-scale flow. Specifically, in a numerical cell there exists an SGS vortex with direction vector
![]() $\boldsymbol{e}^{v}$
, resulting in the subgrid stress
$\boldsymbol{e}^{v}$
, resulting in the subgrid stress
where
![]() $K$
is the subgrid kinetic energy. This is computed from an integral of the SGS energy spectrum as
$K$
is the subgrid kinetic energy. This is computed from an integral of the SGS energy spectrum as
Here
![]() $k_{c}=\unicode[STIX]{x03C0}/\unicode[STIX]{x1D6E5}_{c}$
is the cutoff wavenumber,
$k_{c}=\unicode[STIX]{x03C0}/\unicode[STIX]{x1D6E5}_{c}$
is the cutoff wavenumber,
![]() $\tilde{a}=\boldsymbol{e}_{i}^{v}\boldsymbol{e}_{j}^{v}\widetilde{\unicode[STIX]{x1D61A}}_{ij}$
is the stretching along the subgrid vortex with
$\tilde{a}=\boldsymbol{e}_{i}^{v}\boldsymbol{e}_{j}^{v}\widetilde{\unicode[STIX]{x1D61A}}_{ij}$
is the stretching along the subgrid vortex with
![]() $\widetilde{\unicode[STIX]{x1D61A}}_{ij}$
the local resolved-scale, rate-of-strain tensor. In the present implementation, the
$\widetilde{\unicode[STIX]{x1D61A}}_{ij}$
the local resolved-scale, rate-of-strain tensor. In the present implementation, the
![]() $\boldsymbol{e}_{j}^{v}$
are aligned with the principal extensional eigenvector of
$\boldsymbol{e}_{j}^{v}$
are aligned with the principal extensional eigenvector of
![]() $\widetilde{\unicode[STIX]{x1D61A}}_{ij}$
. The grouped constant
$\widetilde{\unicode[STIX]{x1D61A}}_{ij}$
. The grouped constant
![]() ${\mathcal{K}}_{0}^{\prime }$
is calculated from the resolved-scale velocity using a matching procedure as
${\mathcal{K}}_{0}^{\prime }$
is calculated from the resolved-scale velocity using a matching procedure as
![]() ${\mathcal{K}}_{0}^{\prime }=\langle F_{2}\rangle /\langle Q(\unicode[STIX]{x1D705}_{c},d)\rangle$
, where
${\mathcal{K}}_{0}^{\prime }=\langle F_{2}\rangle /\langle Q(\unicode[STIX]{x1D705}_{c},d)\rangle$
, where
![]() $\langle \cdots \rangle$
denotes an averaging strategy, computed as the arithmetic mean of
$\langle \cdots \rangle$
denotes an averaging strategy, computed as the arithmetic mean of
![]() $26$
neighbouring points and
$26$
neighbouring points and
![]() $\unicode[STIX]{x1D705}_{c}=k_{c}(2\unicode[STIX]{x1D708}/3|\tilde{a}|)^{1/2}$
(Chung & Pullin Reference Chung and Pullin2009). The quantity
$\unicode[STIX]{x1D705}_{c}=k_{c}(2\unicode[STIX]{x1D708}/3|\tilde{a}|)^{1/2}$
(Chung & Pullin Reference Chung and Pullin2009). The quantity
![]() $F_{2}$
is a second-order velocity difference of the resolved-scale velocity field between two points,
$F_{2}$
is a second-order velocity difference of the resolved-scale velocity field between two points,
![]() $Q(\unicode[STIX]{x1D705}_{c},d)$
is calculated using an asymptotic approximation to an integral with
$Q(\unicode[STIX]{x1D705}_{c},d)$
is calculated using an asymptotic approximation to an integral with
![]() $d=r/\unicode[STIX]{x1D6E5}_{c}$
, where
$d=r/\unicode[STIX]{x1D6E5}_{c}$
, where
![]() $r$
is the distance of the cell centre to the neighbouring point. The model implementation is dynamic with no adjustable parameters. For details, see Misra & Pullin (Reference Misra and Pullin1997), Voelkl et al. (Reference Voelkl, Pullin and Chan2000), Chung & Pullin (Reference Chung and Pullin2009).
$r$
is the distance of the cell centre to the neighbouring point. The model implementation is dynamic with no adjustable parameters. For details, see Misra & Pullin (Reference Misra and Pullin1997), Voelkl et al. (Reference Voelkl, Pullin and Chan2000), Chung & Pullin (Reference Chung and Pullin2009).