3.1. Green function for an open water channel confined by two semi-infinite ice sheets

To solve the boundary value problem for the disturbed velocity potential, we may first seek the corresponding Green function $G(p,q)$ which is defined as the velocity potential at field point $p(x,y,z)$ owing to a source at point $q(\xi ,\eta ,\zeta )$. Here, $G$ should satisfy the following governing equation

(3.1)\begin{equation} \nabla^2G + \frac{\partial^2 G}{\partial z^2} ={-}4{\rm \pi} \delta(x-\xi)\delta(y-\eta)\delta(z-\zeta), \end{equation}

throughout the fluid, and the same boundary conditions in (2.4), (2.8), (2.9), (2.13) and the radiation condition. Here, $\delta (x)$ is the Dirac delta function.

To derive $G$, we use the Fourier transform

(3.2)\begin{equation} \tilde{G} = \frac{1}{2{\rm \pi}} \int_{-\infty}^{+\infty} G \,\mathrm{e}^{-\mathrm{i}\alpha x} \, \mathrm{d} x. \end{equation}

It should be mentioned that $G$ is an oscillatory function as $|x| \to +\infty$. One way to perform Fourier transform for this kind of function is to introduce a small negative imaginary part in the radian frequency $\omega$ (Lighthill Reference Lighthill1978). In the inverse Fourier transform, the imaginary part will tend to zero. The integration path at the singularities are deflected and the radiation condition is then satisfied automatically. This procedure is used by Li, Wu & Ji (Reference Li, Wu and Ji2018b). Alternatively, we do not introduce the imaginary part in $\omega$. Once $\tilde {G}$ is derived and its inverse transform is performed, the integration path at the singularities will be decided by the radiation condition, as can be seen later. The governing equation (3.1) for $G$ then becomes, after Fourier transform,

(3.3)\begin{equation} -\alpha^2\tilde{G} + \frac{\partial^2 \tilde{G}}{\partial y^2} + \frac{\partial^2 \tilde{G}}{\partial z^2} ={-}2\mathrm{e}^{-\mathrm{i}\alpha\xi} \delta(y-\eta)\delta(z-\zeta). \end{equation}

Similar to (3.3), Fourier transform is applied to the boundary conditions in (2.4), (2.8) and (2.13), which provides

(3.4)\begin{gather} -\omega^2\tilde{G}+g\frac{\partial \tilde{G}}{\partial z} = 0 \quad (|y|\leqslant b-0 \ \text{and} \ z=0), \end{gather}

(3.5)\begin{gather}\left[ L\left( \alpha^2 - \frac{\partial^2}{\partial y^2} \right)^2 + \rho_w g - m_i\omega^2 \right]\frac{\partial \tilde{G}}{\partial z} - \rho_w \omega^2 \tilde{G} = 0 \quad (|y|\geqslant b+0 \ \text{and} \ z=0), \end{gather}

and

(3.6)\begin{equation} \frac{\partial \tilde{G}}{\partial z} = 0 \quad (z={-}H). \end{equation}

In the channel with $|y|\leqslant b-0$, we may write $\tilde {G}$ in the vertical direction into the eigenfunction expansion as

(3.7)\begin{equation} \tilde{G}\equiv\tilde{G}_f = \sum_{m=0}^{\infty} \,\mathrm{e}^{-\mathrm{i}\alpha\xi}f_m(y)Z_m(z), \end{equation}

where the subscript $f$ implies that the field point is in the water channel, and

(3.8)\begin{equation} Z_m(z) = \frac{\cosh[k_m(z+H)]}{\cosh(k_mH)}, \end{equation}

with $k_m$ as the roots of the dispersion equation for a free surface, or

(3.9)\begin{equation} K_1(\omega,k) \equiv gk\tanh(kH)-\omega^2=0, \end{equation}

Here, $k_0$ is the purely positive real root and $k_m$ $(m=1,\ldots ,\infty )$ are an infinite number of purely negative imaginary roots. It should be noted that the eigenfunction expansion of $\tilde {G}$ in (3.7) has already satisfied the boundary conditions in (3.4) and (3.6). Without loss of generality, we may assume that the source is in the channel or $|\eta | < b$. Substituting (3.7) into (3.3), we have

(3.10)\begin{equation} \sum_{m=0}^{\infty} Z_m(z) [f_m''(y) + \beta_m^2 \,f_m(y)] ={-}2\delta(y-\eta)\delta(z-\zeta), \end{equation}

where $\beta _m^2=k_m^2-\alpha ^2$, and the prime denotes derivative with respect to $y$. Using the orthogonality of the vertical mode $Z_m(z)$, we obtain

(3.11)\begin{equation} f_m''(y) + \beta_m^2 \,f_m(y) ={-}\frac{2}{P_m} \delta(y-\eta) Z_m(\zeta), \end{equation}

where

(3.12)\begin{equation} \int_{{-}H}^{0} Z_m(z)Z_m(z) \, \mathrm{d}z = P_m, \end{equation}

with

(3.13)\begin{equation} P_m = \frac{2k_mH + \sinh(2k_mH)}{4k_m\cosh^2(k_mH)}. \end{equation}

A particular solution of (3.11) can be written as

(3.14)\begin{equation} f_m(y) = \frac{1}{\mathrm{i}\beta_mP_m} \exp({-\mathrm{i}\beta_m|y-\eta|})Z_m(\zeta). \end{equation}

Here, we have assumed $\textrm {Im}(\beta _m) \leqslant 0$ when it is a complex number and $\beta _m>0$ when it is a purely real number. Substituting (3.14) into (3.7), we can write the general solution as

(3.15)\begin{equation} \tilde{G}_f = \tilde{F} + \sum_{m=0}^{\infty} (a_m \varPsi_m^a + b_m \varPsi_m^b), \end{equation}

for $|y| \leqslant b-0$, where

(3.16)\begin{equation} \tilde{F} = \sum_{m=0}^{\infty} \frac{1}{\mathrm{i}\beta_mP_m} \exp({-\mathrm{i}\alpha\xi}) \exp({-\mathrm{i}\beta_m|y-\eta|}) Z_m(\zeta)Z_m(z), \end{equation}

and

(3.17)\begin{gather} \varPsi_m^a = \exp({-\mathrm{i}\alpha\xi})\exp({-\mathrm{i}\beta_m(b-y)})Z_m(z), \end{gather}

(3.18)\begin{gather}\varPsi_m^b = \exp({-\mathrm{i}\alpha\xi})\exp({-\mathrm{i}\beta_m(b+y)})Z_m(z). \end{gather}

The summation in (3.15) is the general solution of (3.3) when its right-hand side is zero. As shown in Appendix A, the first term on the right-hand side of (3.15) is in fact the Fourier transform of the Green function for full free surface without an ice sheet, which is the same as that in Wehausen & Laitone (Reference Wehausen and Laitone1960).

In the ice covered waters, because $y \ne \eta$, equation (3.3) can be further written as

(3.19)\begin{equation} -\alpha^2\tilde{G} + \frac{\partial^2 \tilde{G}}{\partial y^2} + \frac{\partial^2 \tilde{G}}{\partial z^2} = 0. \end{equation}

Then by following the procedure in Li, Wu & Ren (Reference Li, Wu and Ren2020b), we have

(3.20)\begin{equation} \tilde{G}\equiv\tilde{G}_\pm{=} \sum_{m={-}2}^{\infty} c_m^\pm \varPsi_m^\pm, \end{equation}

where the subscript $+$ and $-$ in $\tilde {G}$ are for $y \geqslant b+0$ and $y \leqslant -b-0$, respectively,

(3.21)\begin{equation} \varPsi_m^\pm{=} \exp({-\mathrm{i}\alpha\xi}) \exp({\mp\mathrm{i}\gamma_m(y\mp b)})Q_m(z), \end{equation}

and

(3.22)\begin{equation} Q_m(z) = \frac{\cosh[\kappa_m(z+H)]}{\cosh(\kappa_m H)}, \end{equation}

with $\kappa _m$ being the roots of the dispersion equation for ice sheet or

(3.23)\begin{equation} K_2(\omega,k) \equiv (Lk^4 + \rho_w g - m_i\omega^2)k\tanh(kH)-\rho_w\omega^2 = 0. \end{equation}

Here, $\kappa _{-1}$ and $\kappa _{-2}$ are two complex roots with negative imaginary parts and symmetric about the imaginary axis, $\kappa _0$ is the purely positive real root and $\kappa _m$ $(m=1,\ldots ,\infty )$ are an infinite number of purely negative imaginary roots. In (3.21), $\gamma _m^2 = \kappa _m^2 - \alpha ^2$ and ${\textrm {Im}(\gamma _m) \leqslant 0}$ when it is a complex number and $\gamma _m>0$ when it is a purely real number, which is based on the requirement of the radiation condition. It should be noted that $\tilde {G}$ in (3.20) has already satisfied the boundary conditions in (3.5) and (3.6).

There are four sets of unknown coefficients in (3.15) and (3.20), i.e. $a_m$, $b_m$, $c_m^+$ and $c_m^-$. These can be determined through the continuous conditions at the interfaces $y = \pm b$ or

(3.24)\begin{equation} \left. \tilde{G}_\pm \right|_{y={\pm} b} = \left. \tilde{G}_f \right|_{y={\pm} b}, \end{equation}

and

(3.25)\begin{equation} \left. \frac{\partial \tilde{G}_\pm}{\partial y} \right|_{y={\pm} b} = \left. \frac{\partial \tilde{G}_f}{\partial y} \right|_{y={\pm} b}, \end{equation}

together with the ice edge conditions. We may apply the Fourier transform (3.2) to the free ice edge conditions (2.9), which provides

(3.26a,b)\begin{equation} \tilde{\mathcal{B}} \left( \frac{\partial \tilde{G}}{\partial z} \right) = 0 \quad \text{and} \quad \tilde{\mathcal{S}} \left( \frac{\partial \tilde{G}}{\partial z} \right) = 0, \end{equation}

with

(3.27)\begin{gather} \tilde{\mathcal{B}} = \frac{\partial^2}{\partial y^2} - \nu \alpha^2, \end{gather}

(3.28)\begin{gather}\tilde{\mathcal{S}} = \frac{\partial}{\partial y} \left[ \frac{\partial^2}{\partial y^2} - (2-\nu)\alpha^2 \right]. \end{gather}

To impose these conditions, we may adopt Green's second theorem over the boundary $\varGamma _+$ of the domain with $y\geqslant b+0$, which provides

(3.29)\begin{equation} \oint_{\varGamma_+} \left( \tilde{G}_+ \frac{\partial \varPsi_m^+}{\partial n} - \frac{\partial \tilde{G}_+}{\partial n} \varPsi_m^+ \right) \mathrm{d}l = 0. \end{equation}

Here, it should be noted that $\tilde {G}_+$ and $\varPsi _m^+$ should satisfy the same boundary conditions on the flat seabed, ice sheet and the vertical surface at the far field $y=+\infty$. Removing the zero terms, we have

(3.30)\begin{equation} -\int_{{-}H}^{0} \left( \tilde{G}_+ \frac{\partial \varPsi_m^+}{\partial y} - \frac{\partial \tilde{G}_+}{\partial y} \varPsi_m^+ \right)_{y={+}b} \, \mathrm{d}z + \int_{{+}b}^{+\infty} \left( \tilde{G}_+ \frac{\partial \varPsi_m^+}{\partial z} - \frac{\partial \tilde{G}_+}{\partial z} \varPsi_m^+ \right)_{z=0} \, \mathrm{d} y =0. \end{equation}

Equation (3.5) provides

(3.31)\begin{equation} \tilde{G}_+{=} \frac{L}{\rho_w \omega^2}\left( \alpha^4 - 2\alpha^2\frac{\partial^2}{\partial y^2} + \frac{\partial ^4}{\partial y^4} \right)\frac{\partial \tilde{G}_+}{\partial z} +\frac{\rho_w g - m_i\omega^2}{\rho_w \omega^2}\frac{\partial \tilde{G}_+}{\partial z}, \end{equation}

which is also satisfied by $\psi _m^+$. Substituting (3.31) into (3.30), and using integration by parts over the ice sheet surface, we can obtain

(3.32)\begin{align} & \int_{{-}H}^{0} \left( \tilde{G}_+ \frac{\partial \varPsi_m^+}{\partial y} - \frac{\partial \tilde{G}_+}{\partial y} \varPsi_m^+ \right)_{y={+}b} \, \mathrm{d}z + \frac{L}{\rho_w\omega^2} \left[ 2\alpha^2\left( \frac{\partial \tilde{G}_+}{\partial z}\frac{\partial^2 \varPsi_m^+}{\partial y \partial z} - \frac{\partial^2 \tilde{G}_+}{\partial y \partial z}\frac{\partial \varPsi_m^+}{\partial z} \right) \right. \nonumber\\ &\quad \left. + \left( \frac{\partial^4 \tilde{G}_+}{\partial y^3 \partial z}\frac{\partial \varPsi_m^+}{\partial z} - \frac{\partial \tilde{G}_+}{\partial z}\frac{\partial^4 \varPsi_m^+}{\partial y^3\partial z} \right) - \left( \frac{\partial^3 \tilde{G}_+}{\partial y^2 \partial z}\frac{\partial^2 \varPsi_m^+}{\partial y \partial z} - \frac{\partial^2 \tilde{G}_+}{\partial y \partial z}\frac{\partial^3 \varPsi_m^+}{\partial y^2\partial z} \right) \right]_{y={+}b,z=0} =0. \end{align}

Similarly, we have at $y=-b$

(3.33)\begin{align} & \int_{{-}H}^{0} \left( \tilde{G}_- \frac{\partial \varPsi_m^-}{\partial y} - \frac{\partial \tilde{G}_-}{\partial y} \varPsi_m^- \right)_{y={-}b} \, \mathrm{d}z + \frac{L}{\rho_w\omega^2} \left[ 2\alpha^2\left( \frac{\partial \tilde{G}_-}{\partial z}\frac{\partial^2 \varPsi_m^-}{\partial y \partial z} - \frac{\partial^2 \tilde{G}_-}{\partial y \partial z}\frac{\partial \varPsi_m^-}{\partial z} \right) \right. \nonumber\\ &\quad\left. + \left( \frac{\partial^4 \tilde{G}_-}{\partial y^3 \partial z}\frac{\partial \varPsi_m^-}{\partial z} - \frac{\partial \tilde{G}_-}{\partial z}\frac{\partial^4 \varPsi_m^-}{\partial y^3\partial z} \right) - \left( \frac{\partial^3 \tilde{G}_-}{\partial y^2 \partial z}\frac{\partial^2 \varPsi_m^-}{\partial y \partial z} - \frac{\partial^2 \tilde{G}_-}{\partial y \partial z}\frac{\partial^3 \varPsi_m^-}{\partial y^2\partial z} \right) \right]_{y={-}b,z=0} =0. \end{align}

Invoking the free ice edge condition (3.26a,b), we have

(3.34a,b)\begin{equation} \frac{\partial^3 \tilde{G}_\pm}{\partial y^2 \partial z} = \nu \alpha^2 \frac{\partial \tilde{G}_\pm}{\partial z} \quad \text{and} \quad \frac{\partial^4 \tilde{G}_\pm}{\partial y^3 \partial z} = (2-\nu) \alpha^2 \frac{\partial^2\tilde{G}_\pm}{\partial y \partial z} \end{equation}

at $y = \pm b$ and $z = 0$. Substituting this equation into (3.32) and (3.33), we obtain

(3.35)\begin{align} & \int_{{-}H}^{0} \left( \tilde{G}_f \frac{\partial \varPsi_m^\pm}{\partial y} - \frac{\partial \tilde{G}_\pm}{\partial y} \varPsi_m^\pm \right)_{y={\pm} b} \, \mathrm{d}z + \frac{L}{\rho_w\omega^2} \left\{ \alpha^2\left[ (2-\nu)\frac{\partial \tilde{G}_\pm}{\partial z}\frac{\partial^2 \varPsi_m^\pm}{\partial y \partial z}\right. \right. \nonumber\\ &\quad \left. -\nu\frac{\partial^2 \tilde{G}_\pm}{\partial y \partial z}\frac{\partial \varPsi_m^\pm}{\partial z} \right] \left. + \frac{\partial^2 \tilde{G}_\pm}{\partial y \partial z} \frac{\partial^3\varPsi_m^\pm}{\partial y^2 \partial z} - \frac{\partial \tilde{G}_\pm}{\partial z} \frac{\partial^4 \varPsi_m^\pm}{\partial y^3 \partial z} \right\}_{y={\pm} b, z=0} =0, \end{align}

where the continuity condition (3.24) has been used. It should be noted that the free ice edge condition has been imposed in (3.35) through replacing the corresponding terms on the ice edge in (3.32) and (3.33). There is no need to have further actions to impose this condition. The way to satisfy the ice edge condition here is similar to that of Ren et al. (Reference Ren, Wu and Thomas2016). To impose the continuity condition (3.25), we multiply both sides of (3.15) with $Z_m(z)$ and then integrate with respect to $z$. This gives

(3.36)\begin{align} \int_{{-}H}^{0} \frac{\partial \tilde{G}_+}{\partial y} Z_m(z) \, \mathrm{d}z &= \int_{{-}H}^{0} \frac{\partial \tilde{G}_f}{\partial y} Z_m(z) \, \mathrm{d}z \nonumber\\ &= \mathrm{i}\beta_m P_m (a_m - b_m \exp({-2\mathrm{i}\beta_m b})) \nonumber\\ &\quad - \exp({-\mathrm{i}\alpha \xi}) \exp({-\mathrm{i}\beta_m(b-\eta)}) Z_m(\zeta), \end{align}

(3.37)\begin{align} \int_{{-}H}^{0} \frac{\partial \tilde{G}_-}{\partial y} Z_m(z) \, \mathrm{d}z &= \int_{{-}H}^{0} \frac{\partial \tilde{G}_f}{\partial y} Z_m(z) \, \mathrm{d}z \nonumber\\ &= \mathrm{i}\beta_m P_m (a_m \exp({-2\mathrm{i}\beta_m b}) - b_m) \nonumber\\ &\quad + \exp({-\mathrm{i}\alpha \xi})\exp({-\mathrm{i}\beta_m(b+\eta)}) Z_m(\zeta), \end{align}

for $y=+b$ and $y=-b$, respectively, in which (3.12) has been used. Substituting (3.15) and (3.20) into (3.35) and (3.36), we have at $y=+b$

(3.38)\begin{align} & \gamma_m \sum_{\tilde{m}=0}^{\infty} (a_{\tilde{m}} + b_{\tilde{m}} \exp({-2\mathrm{i}\beta_{\tilde{m}}b})) V_{m,{\tilde{m}}} - c_m^+ \gamma_m U_m -\frac{LT_m}{\rho_w \omega^2} \sum_{\tilde{m}={-}2}^{\infty} c_{\tilde{m}}^+ T_{\tilde{m}} [\nu\alpha^2(\gamma_{\tilde{m}}+\gamma_m) \nonumber\\ &\qquad - 2\alpha^2\gamma_m + \gamma_m^2(\gamma_{\tilde{m}}-\gamma_m) - \gamma_{\tilde{m}} (\kappa_m^2 + \kappa_{\tilde{m}}^2)]\nonumber\\ &\quad =\mathrm{i}\gamma_m\sum_{\tilde{m}=0}^{\infty}\frac{1}{\beta_{\tilde{m}}P_{\tilde{m}}} \exp({-\mathrm{i}\beta_{\tilde{m}}(b-\eta)})Z_{\tilde{m}}(\zeta)V_{m,{\tilde{m}}}, \end{align}

(3.39)\begin{align} &\beta_m P_m ( b_m \exp({-2\mathrm{i}\beta_m b}) - a_m ) - \sum_{\tilde{m}={-}2}^{\infty} c_{\tilde{m}}^+ \gamma_{\tilde{m}}V_{{\tilde{m}},m} = \mathrm{i} \exp({-\mathrm{i}\beta_m(b-\eta)})Z_m(\zeta), \end{align}

where

(3.40)\begin{gather} T_m = \kappa_m \tanh(\kappa_m H), \end{gather}

(3.41)\begin{gather}U_m = \frac{2\kappa_m H + \sinh(2\kappa_m H)}{4\kappa_m\cosh^2(\kappa_m H)} + \frac{2LT_m^2\kappa_m^2}{\rho_w\omega^2}, \end{gather}

(3.42)\begin{gather}V_{m,{\tilde{m}}}=\int_{{-}H}^{0} Q_m Z_{\tilde{m}} \, \mathrm{d}z = \frac{T_m - k_{\tilde{m}}\tanh(k_{{\tilde{m}}H})}{\kappa_m^2 - k_{\tilde{m}}^2}. \end{gather}

It should be noted that in (3.38) the following relationship has been used

(3.43)\begin{equation} \int_{{-}H}^{0} Q_m Q_{\tilde{m}} \, \mathrm{d}z = \delta_{m,\tilde{m}}U_m - \frac{LT_mT_{\tilde{m}}}{\rho_w\omega^2} (\kappa_m^2 + \kappa_{\tilde{m}}^2), \end{equation}

where $\delta _{m,\tilde {m}}$ is the Kronecker delta function. Similarly, we have at $y=-b$

(3.44)\begin{align} & \gamma_m \sum_{\tilde{m}=0}^{\infty} (a_{\tilde{m}} \exp({-2\mathrm{i}\beta_{\tilde{m}}b}) + b_{\tilde{m}} ) V_{m,{\tilde{m}}} - c_m^- \gamma_m U_m -\frac{LT_m}{\rho_w \omega^2} \sum_{\tilde{m}={-}2}^{\infty} c_{\tilde{m}}^- T_{\tilde{m}} [\nu\alpha^2(\gamma_{\tilde{m}}+\gamma_m) \nonumber\\ &\qquad - 2\alpha^2\gamma_m + \gamma_m^2(\gamma_{\tilde{m}}-\gamma_m) - \gamma_{\tilde{m}} (\kappa_m^2 + \kappa_{\tilde{m}}^2)]\nonumber\\ &\quad =\mathrm{i}\gamma_m\sum_{\tilde{m}=0}^{\infty}\frac{1}{\beta_{\tilde{m}}P_{\tilde{m}}} \exp({-\mathrm{i}\beta_{\tilde{m}}(b+\eta)})Z_{\tilde{m}}(\zeta)V_{m,{\tilde{m}}}, \end{align}

(3.45)\begin{align} &\beta_m P_m ( a_m \exp({-2\mathrm{i}\beta_m b}) - b_m ) - \sum_{\tilde{m}={-}2}^{\infty} c_{\tilde{m}}^- \gamma_{\tilde{m}}V_{{\tilde{m}},m} = \mathrm{i} \exp({-\mathrm{i}\beta_m(b+\eta)})Z_m(\zeta). \end{align}

From (3.39) and (3.45), we can obtain

(3.46)\begin{align} a_m &= \frac{1}{\beta_mP_m(\exp({-4\mathrm{i}\beta_m b}) - 1)} \sum_{\tilde{m}={-}2}^{\infty} ( c_{\tilde{m}}^+{+} c_{\tilde{m}}^- \exp({-2\mathrm{i}\beta_m b})) \gamma_{\tilde{m}} V_{\tilde{m},m} \nonumber\\ &\quad + \mathrm{i}Z_m(\zeta) \frac{\exp({-\mathrm{i}\beta_m (3b+\eta)}) + \exp({-\mathrm{i}\beta_m (b-\eta)})}{\beta_mP_m(\exp({-4\mathrm{i}\beta_m b}) - 1)}, \end{align}

(3.47)\begin{align} b_m &= \frac{1}{\beta_mP_m(\exp({-4\mathrm{i}\beta_m b}) - 1)} \sum_{\tilde{m}={-}2}^{\infty} ( c_{\tilde{m}}^+ \exp({-2\mathrm{i}\beta_m b}) + c_{\tilde{m}}^-) \gamma_{\tilde{m}} V_{\tilde{m},m} \nonumber\\ &\quad + \mathrm{i}Z_m(\zeta) \frac{\exp({-\mathrm{i}\beta_m (b+\eta)}) + \exp({-\mathrm{i}\beta_m (3b-\eta)})}{\beta_mP_m(\exp({-4\mathrm{i}\beta_m b}) - 1)}. \end{align}

Substituting these two equations into (3.38), we have

(3.48)\begin{align} & -c_m^+ \gamma_mU_m + \gamma_m \sum_{\tilde{m}=0}^{\infty} \frac{V_{m,\tilde{m}}}{\beta_{\tilde{m}} P_{\tilde{m}}(\exp({-4\mathrm{i}\beta_{\tilde{m}}b}) - 1)}\nonumber\\ &\qquad \times \sum_{n={-}2}^{\infty} [ c_n^+ (1 + \exp({-4\mathrm{i}\beta_{\tilde{m}}b})) + 2c_n^- \exp({-2\mathrm{i}\beta_{\tilde{m}}b}) ] \gamma_n V_{n,\tilde{m}} \nonumber\\ &\qquad - \frac{LT_m}{\rho_w \omega^2} \sum_{\tilde{m}={-}2}^{\infty} c_{\tilde{m}}^+T_{\tilde{m}} [ \nu\alpha^2 (\gamma_{\tilde{m}} + \gamma_m) - 2\alpha^2\gamma_m + \gamma_m^2 (\gamma_{\tilde{m}} - \gamma_m) - \gamma_{\tilde{m}} (\kappa_m^2 + \kappa_{\tilde{m}}^2) ] \nonumber\\ &\quad ={-}2\mathrm{i}\gamma_m \sum_{\tilde{m}=0}^{\infty} \frac{\exp({-\mathrm{i} \beta_{\tilde{m}} (b-\eta)}) + \exp({-\mathrm{i}\beta_{\tilde{m}} (3b+\eta)})}{\beta_{\tilde{m}} P_{\tilde{m}}(\exp({-4\mathrm{i}\beta_{\tilde{m}} b}) - 1)} Z_{\tilde{m}}(\zeta) V_{m,\tilde{m}}. \end{align}

Similarly, we have for (3.44)

(3.49)\begin{align} & -c_m^- \gamma_mU_m + \gamma_m \sum_{\tilde{m}=0}^{\infty} \frac{V_{m,\tilde{m}}}{\beta_{\tilde{m}} P_{\tilde{m}}(\exp({-4\mathrm{i}\beta_{\tilde{m}}b}) - 1)}\nonumber\\ &\qquad \times \sum_{n={-}2}^{\infty} [ 2c_n^+ \exp({-2\mathrm{i}\beta_{\tilde{m}}b}) + c_n^- (1 + \exp({-4\mathrm{i}\beta_{\tilde{m}}b})) ] \gamma_n V_{n,\tilde{m}} \nonumber\\ &\qquad - \frac{LT_m}{\rho_w \omega^2} \sum_{\tilde{m}={-}2}^{\infty} c_{\tilde{m}}^-T_{\tilde{m}} [ \nu\alpha^2 (\gamma_{\tilde{m}} + \gamma_m) - 2\alpha^2\gamma_m + \gamma_m^2 (\gamma_{\tilde{m}} - \gamma_m) - \gamma_{\tilde{m}} (\kappa_m^2 + \kappa_{\tilde{m}}^2) ] \nonumber\\ &\quad ={-}2\mathrm{i}\gamma_m \sum_{\tilde{m}=0}^{\infty} \frac{\exp({-\mathrm{i}\beta_{\tilde{m}} (b+\eta)}) + \exp({-\mathrm{i}\beta_{\tilde{m}} (3b-\eta)})}{\beta_{\tilde{m}}P_{\tilde{m}}(\exp({-4\mathrm{i}\beta_{\tilde{m}} b}) - 1)} Z_{\tilde{m}}(\zeta) V_{m,\tilde{m}}. \end{align}

This shows $c_m^+$ and $c_m^-$ can be solved first independently from (3.48) and (3.49), after which $a_m$ and $b_m$ can be obtained directly from (3.46) and (3.47).

In practical computations, (3.48) and (3.49) can be solved through truncating the infinite summation at a finite number $m=M$. Here, it should be noted that the matrix coefficients for the unknowns only depend on the value of $\alpha$ and are independent of the source position. Therefore, the inverse does not have to be recalculated at different source position. In addition, from (3.46) and (3.47), we can see that the truncation for $a_m$ and $b_m$ can be made at a value different from $M$.

The Green function $G$ can be found through applying the inverse Fourier transform to $\tilde {G}$, or

(3.50)\begin{equation} G = \int_{-\infty}^{+\infty} \tilde{G} \,\mathrm{e}^{+\mathrm{i}\alpha x} \, \mathrm{d} \alpha. \end{equation}

Substituting (3.15) and (3.20) into this equation, and using the symmetry property of $\tilde {G}$, we have

(3.51)\begin{align} G = \left\{\begin{array}{ll} \displaystyle 2\sum_{m={-}2}^{\infty} Q_m(z) \int_{0}^{+\infty} c_m^+ \exp({-\mathrm{i}\gamma_m(y-b)}) \cos[\alpha(x-\xi)] \, \mathrm{d}\alpha, & y\geqslant b+0 \\ \displaystyle F+2\sum_{m=0}^{\infty} Z_m(z) \int_{0}^{+\infty} (a_m \exp({-\mathrm{i}\beta_m(b-y)} ) & \\ \quad + b_m \, \exp({-\mathrm{i}\beta_m(b+y)})) \cos[\alpha(x-\xi)] \, \mathrm{d}\alpha, & |y| \leqslant b-0 \\ \displaystyle 2\sum_{m={-}2}^{\infty} Q_m(z) \int_{0}^{+\infty} c_m^- \exp({+\mathrm{i}\gamma_m(y+b)}) \cos[\alpha(x-\xi)] \, \mathrm{d}\alpha, & y\leqslant -b-0. \end{array} \right. \end{align}

where

(3.52)\begin{equation} F = \sum_{m=0}^{\infty} \frac{1}{\mathrm{i}P_m} Z_m(\zeta)Z_m(z)I_m, \end{equation}

with

(3.53)\begin{equation} I_m = \int_{-\infty}^{+\infty} \frac{1}{\beta_m} \exp({+\mathrm{i}\alpha(x-\xi)}) \exp({-\mathrm{i}\beta_m|y-\eta|}) \, \mathrm{d}\alpha = {\rm \pi}H_0^{(2)}(k_mR). \end{equation}

Here, $F$ is identical to the Green function for a free surface in (A16), and (A11) and (A14) have been used in (3.53). It should be noted that when the ice thickness $h=0$, the Green function in (3.51) will become that for a free surface, as shown in Appendix A. As shown in Appendix B, we also have that the Green function is symmetric regarding the source and field points or

(3.54)\begin{equation} G(p,q) - G(q,p)=0. \end{equation}

When $\alpha \to k_0$ or $\beta _0^2 = k_0^2 - \alpha ^2 \to 0$, (3.38) and (3.44) show that there is a singularity on the right-hand side. However, it is of square root order, or $1/(k_0^2 - \alpha ^2)^{1/2}$ in the inverse Fourier transform, which numerically can be computed through the Gauss–Chebyshev procedure (Abramowitz & Stegun Reference Abramowitz and Stegun1965). Special care should be paid to the integrals over the domain $\kappa _0 < \alpha < k_0$ when $k_0>\kappa _0$. In such a case, there are non-decaying wave modes at $x=\pm \infty$ at discrete wave numbers of $\kappa _0 < \alpha _1 < \ldots < \alpha _N < k_0$, which correspond to the trapped modes in Porter (Reference Porter2018). This means that there may be a number of poles at $\alpha _j$ $(\,j=1,\ldots ,N)$ of the integrand in (3.51). To satisfy the radiation condition, which states that the waves should propagate away from the source, the integral route in (3.51) from $0$ to $+\infty$ should pass over these poles. Then, through applying the Fourier integrals in Wehausen & Laitone (Reference Wehausen and Laitone1960), we can obtain the asymptotic expression of (3.51) at $|x-\xi | \to +\infty$, or

(3.55)\begin{align} G_\infty \equiv & \lim_{|x-\xi| \to +\infty} G ={-}2\mathrm{i}{\rm \pi} \sum_{j=1}^{N} \exp({-\mathrm{i}\alpha_j |x-\xi|}) \nonumber\\ &\quad \times \left\{ \begin{array}{ll} \displaystyle\sum_{m={-}2}^{\infty} Q_m(z) \lim_{\alpha \to \alpha_j} [(\alpha - \alpha_j)c_m^+\exp({-\mathrm{i}\gamma_{m,j}(y-b)})], & y\geqslant b+0 \\ \displaystyle \sum_{m=0}^{\infty} Z_m(z) \lim_{\alpha \to \alpha_j} \{(\alpha - \alpha_j)[a_m \exp({-\mathrm{i}\beta_{m,j}(b-y)}) & \\ \quad + b_m \exp({-\mathrm{i}\beta_{m,j}(b+y)})]\}, & |y| \leqslant b-0 \\ \displaystyle \sum_{m={-}2}^{\infty} Q_m(z) \lim_{\alpha \to \alpha_j} [(\alpha - \alpha_j)c_m^- \exp({+\mathrm{i}\gamma_{m,j}(y+b)})], & y\leqslant -b-0. \end{array} \right. \end{align}

For $|y| \leqslant b-0$, the wave component of $\alpha _j$ exists inside the channel. However, for $|y| \geqslant b + 0$ below the ice sheet, $\gamma _{m,j}$, corresponding to $\alpha _j$, always have $\textrm {Im}{(\gamma _{m,j})}<0$, which indicates that the wave of $\alpha _j$ will decay exponentially with $y$. It should also be noted that $F$ in (3.51) for $|y| \leqslant b-0$ corresponds to the cylindrical wave at the far field, the amplitude of which decreases with $1/\sqrt {R}$. Therefore, this term has been dropped in (3.55) because it will diminish as $|x-\xi | \to +\infty$.

To search for $\alpha _j$ numerically, we may use the fact that the singularities of $a_m$, $b_m$, $c_m^+$ and $c_m^-$ are of the form of $1/(\alpha -\alpha _j)$. When (3.46)– (3.49) are solved at different $\alpha$, sufficiently small step $\Delta \alpha$ is used. When the results become very large at both $\alpha$ and $\alpha + \Delta \alpha$, and their signs are different, one of the $\alpha _j$ will exist within this step. The accuracy of $\alpha _j$ can be refined by using the further smaller steps within the region.

The calculation of the limit in (3.55) needs some special attention. In theory, the limit can be obtained through L'Hospital's rule. However, the integrand and its singularities here are not explicitly given and they are obtained from the numerical procedure described previously. Thus, the following method is used to calculate the limit. We may consider a function $f(\alpha )$, which has a singularity in the form $f(\alpha ) \to g(\alpha )/(\alpha - \alpha _j)$ as $\alpha \to \alpha _j$. Then, $g(\alpha _j) = \lim _{\alpha \to \alpha _j} (\alpha - \alpha _j) f(\alpha )$ can be found numerically by

(3.56)\begin{align} g(\alpha_j) & = \frac{g(\alpha_j + \Delta\alpha) + g(\alpha_j - \Delta\alpha)}{2} + O[g''(\alpha_j)(\Delta\alpha)^2] \nonumber\\ & \approx \Delta\alpha \frac{f(\alpha_j + \Delta\alpha) - f(\alpha_j - \Delta\alpha)}{2}. \end{align}

3.2. Solution to the disturbed velocity potential for a body in the ice channel

As shown in Appendix B, we have the boundary integral equation for the disturbed velocity potential $\phi$ as follow

(3.57)\begin{equation} \ell \phi(p) = \int_{S_B} \left[ G(p,q) \frac{\partial \phi(q)}{\partial n_q} - \frac{\partial G(p,q)}{\partial n_q} \phi(q) \right] \, \mathrm{d}s_q, \end{equation}

where only the integral over the mean wetted body surface $S_B$ is needed, and $\ell$ is the solid angle at point $p$. As noted by Lee, Newman & Zhu (Reference Lee, Newman and Zhu1996) in the free surface problem, for floating bodies there could exist a discrete spectrum of irregular frequencies, at which the solution to the boundary integral equation is non-unique. Similar irregular frequencies also exist in (3.57). To remove the irregular frequencies, we follow the procedure described in Lee et al. (Reference Lee, Newman and Zhu1996) and rewrite (3.57) equivalently as

(3.58)\begin{equation} \ell \phi(p) + \int_{S_B+S_E} \frac{\partial G(p,q)}{\partial n_q} \phi(q) \,\mathrm{d}s_q = \int_{S_B} G(p,q) \frac{\partial \phi(q)}{\partial n_q} \, \mathrm{d}s_q \quad \text{for} \ p \in S_B, \end{equation}

and

(3.59)\begin{equation} -4{\rm \pi} \phi(p) + \int_{S_B+S_E} \frac{\partial G(p,q)}{\partial n_q} \phi(q) \, \mathrm{d}s_q = \int_{S_B} G(p,q) \frac{\partial \phi(q)}{\partial n_q} \, \mathrm{d}s_q \quad \text{for} \ p \in S_E, \end{equation}

where $S_E$ is the extended surface interior the body at $z=0$. This has been found to remove the irregular frequencies effectively.

For the radiation problem, $\phi = \phi _j$ and $\partial \phi _j / \partial n = n_j$ can be used directly in the integral equations (3.58) and (3.59). However, for the diffraction problem, it has two components. The incident wave will be diffracted by both the channel and the body. Thus, similar to Li et al. (Reference Li, Wu and Ji2018b), we write the total diffracted potential as

(3.60)\begin{equation} \phi_D = \phi_D^1 + \phi_D^2, \end{equation}

where $\phi _D^1$ is the diffracted potential of the flexural gravity incident potential $\phi _I$ by the water channel, and $\phi _D^2$ is that by the body to $\varphi = \phi _I + \phi _D^1$. It should be mentioned that $\varphi$ satisfies the ice edge condition (2.9). Here, the incident potential $\phi _I$ can be written as

(3.61)\begin{equation} \phi_I = \varphi_I(y,z) \,\mathrm{e}^{-\mathrm{i}\kappa_x x}, \end{equation}

with

(3.62)\begin{equation} \varphi_I = A \,\mathrm{e}^{-\mathrm{i}\kappa_y y} Q_0(z), \end{equation}

where $A=\mathrm {i}\omega /[\kappa _0\tanh (\kappa _0H)]$, $\kappa _x = \kappa _0 \cos \beta$ and $\kappa _y = \kappa _0\sin \beta$. Correspondingly, the potential $\varphi$ can be written as

(3.63)\begin{equation} \varphi = \bar{\varphi}(y,z) \,\mathrm{e}^{-\mathrm{i}\kappa_x x}. \end{equation}

Here $\bar {\varphi }$ or $\phi _D^1$ can be obtained virtually in the same way as $\tilde {G}$. The main difference is that $\alpha$ should be replaced by $\kappa _x$ and terms on the right-hand sides due to $\tilde {F}$ in (3.46)– (3.49) should be replaced by the contribution due to $\phi _I$. Because $\varphi =\phi _I + \phi _D^1$ satisfies the conditions at the ice sheet edge, then $\phi _D^2$ should also satisfy these conditions. Thus, we can apply the integral equation (3.57) to $\phi _D^2$ by imposing the boundary condition $\partial \phi _D^2 / \partial n = -\partial \varphi / \partial n$ on the body surface.

After the velocity potentials have been found, the pressure at any point in fluid can be computed through the linearized Bernoulli equation. Then the hydrodynamic forces on the body can be obtained through integrating the pressure over its surface. Based on the decomposition of the velocity potential in (2.1), we may divide the total hydrodynamic loads into two parts, i.e. the radiation force due to the forced oscillatory motions and the wave exciting force due to the scattering potential (Newman Reference Newman1977). For the radiation potential, we have

(3.64)\begin{equation} \mu_{jk} - \mathrm{i}\frac{\lambda_{jk}}{\omega} = \rho_w \int_{S_B} \phi_kn_j \, \mathrm{d}s, \end{equation}

where $\mu _{jk}$ and $\lambda _{jk}$ are the added mass and damping coefficient, respectively. For the scattering potential, we have

(3.65)\begin{equation} f_{E,j} ={-}\mathrm{i} \omega \rho_w \int_{S_B} \phi_0 n_j \, \mathrm{d}s, \end{equation}

where $f_{E,j}$ is the wave exciting force.

4.1. Wave induced by a source submerged in the ice channel

We first consider the wave induced by a source submerged in the channel confined by two semi-infinite ice sheets, with the ice sheet thickness $h=1\ \mathrm {m}$ taken as the characteristic length scale and the half channel width fixed to be $b=50\ \mathrm {m}$. This is to shed some lights on some features of the free surface and the ice sheet deflection pattern. Numerical calculations are carried out through truncating the infinite summations in (3.51) to a finite number, or keeping only the first $M_G + 1$ terms. The wave elevation is computed based on the kinematic boundary condition, which gives

(4.2)\begin{equation} w = \frac{1}{\mathrm{i}\omega} \left. \frac{\partial G}{\partial z} \right|_{z=0}. \end{equation}

The values of $\alpha _j$ are obtained through the procedure described towards the end of § 3.1. It should be noted that $\alpha _j$ do not depend on the location of the source. However, each $\alpha _j$ corresponds to a wave in the channel either symmetric or antisymmetric about $y=0$ (Porter Reference Porter2018). When the source is located at the centre of the channel, only symmetric waves will be triggered. Thus, to capture all $\alpha _j$, corresponding to both symmetric and antisymmetric modes, they are computed through the case with the source located at $(0,b/2,-H/100)$. To ensure the accuracy of $\alpha _j$, as well as the accuracies of integration and the approximation of (3.56), the step $\Delta \alpha$ is chosen from the lowest value among $0.0001$, $(\alpha _j - \alpha _{j-1})/50$ and $(\alpha _{j+1} - \alpha _j)/50$ when $\alpha$ is between $\alpha _{j-1}$ and $\alpha _{j+1}$, where $\alpha _0 = \kappa _0$ for $j=1$ and $\alpha _{N+1}=k_0$ for $j=N$ with $N$ as the number of the singularities. Searching for $\alpha _j$ is done numerically through the Gauss elimination with partial pivoting for the matrix equation. When the solution of the unknown in the last line jumps from a large positive (negative) number $\mathcal {R}$ to a large negative (position) number $-\mathcal {R}$ within a small $\Delta \alpha$, it is assumed that $\alpha _j$ is within $\Delta \alpha$. Here we have used $\mathcal {R}=10^{10}$ and $\Delta \alpha = 10^{-16}$. Figure 2 provides the first symmetric trapped mode $\alpha$ at a large water depth $H/h=100$ against $K=\omega ^2/g$. The infinite summations are truncated at $M_G=100$. As a comparison, the result in Porter (Reference Porter2018) for infinite water depth is also provided, and the same parameters of ice sheet and fluid as well as the characteristic length scale are taken. It can be observed from this figure that the values of $\alpha$ for $H/h=100$ are close to the values in Porter (Reference Porter2018) for infinite water depth. It should be noted that in the present formulation, the expansion in the vertical direction is, in fact, a cosine series. When $H$ is very large, the terms required in the expansion to ensure convergence will increase rapidly. In particular, the series expansion cannot be used when $H=+\infty$. Instead, an integral form should be used to replace the series, similar to that Fourier series should be replaced by Fourier transform. Thus, the present work cannot be used for $H=+\infty$ directly. In fact, when $H$ is very large, the series expansion will require a very large number of terms to ensure accuracy and the method become very inefficient. Therefore, larger water depth is not attempted. A more efficient method in such a case would be to use the integral form in the vertical direction, for example as in Li et al. (Reference Li, Wu and Ji2018b).

Figure 2. The first symmetric trapped mode $\alpha$ at a large water depth $H/h=100$ against $K=\omega ^2/g$. Open circles: $\alpha$ taken from figure 3 of Porter (Reference Porter2018) for infinite water depth. (The parameters of ice sheet and fluid as well as the characteristic length scale are taken to be the same as those in Porter Reference Porter2018).

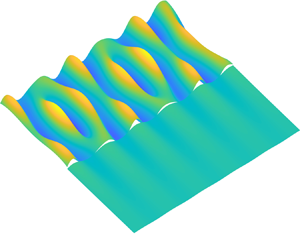
Figure 3 shows the wave elevation $w$ induced by a source with position $(\xi ,\eta ,\zeta )=(0,0,-1)$ and wave number $k_0=0.1$ (the corresponding dimensional wave radian frequency $\omega$ is $0.99 \ \mathrm {rad}\ \mathrm {s}^{-1}$). It can be observed from the figure that there is no visible difference between the results obtained by $M_G=50$ and $M_G=100$, indicating that the convergence has been achieved, and the former will be used for numerical computations of the Green function in this and following sections if it is not specifically specified.

Figure 3. The real part (Re) and imaginary part (Im) of wave elevation $w$ induced by a source at $(\xi ,\eta ,\zeta )=(0,0,-1)$: (a) $w$ varies along the longitudinal cut with $y/b=0$; (b) $w$ varies along the transverse cut with $x/b=35$ ($k_0=0.1$, $b=50$, $h=1\ \mathrm {m}$ is taken as the characteristic length scale).

In figure 4, the transverse variation of $w$ with $y$ at four different $x$ are given, with the wave number being the same as that in figure 3. Two source positions are considered, namely $(\xi ,\eta ,\zeta )=(0,0,-1)$ and $(\xi ,\eta ,\zeta )=(0,25,-1)$. For $x/b=30$, the wave elevation $w_\infty$ computed by the asymptotic formula (3.55) is also provided, and the result agrees well with that obtained by the exact formula (4.2) for both central and non-central source positions. From figure 4, it can be seen that $w$ is generally discontinuous at the ice edge between free surface and ice sheet. This is because that although the kinematic boundary conditions on free surface and ice sheet are the same, their dynamic boundary conditions are different.

Figure 4. The modulus of wave elevation $w$ induced by a source: (a) $(\xi ,\eta ,\zeta )=(0,0,-1)$; (b) $(\xi ,\eta ,\zeta )=(0,25,-1)$ ($k_0=0.1$, $b=50$, $h=1\ \mathrm {m}$ is taken as the characteristic length scale).

The discontinuity of $w$ across the ice sheet edge can be observed more clearly from $w$ at $y/b=1-0$ and $y/b=1+0$ in figure 5, which shows the wave elevation $w_\infty$ along the longitudinal cut at different $y$ computed by the asymptotic formula (3.55). It is well known that for full free surface problem (Wehausen & Laitone Reference Wehausen and Laitone1960) or ice sheet problem (Li, Wu & Shi Reference Li, Wu and Shi2018c), the Green function will decrease in the form of $1/\sqrt {R}$ with $R$ as the horizontal distance between the field and source points. However, this is not the case for the problem of an open water channel confined by two semi-infinite ice sheets. From figure 5, it can be seen that at the far field when $x$ changes, $w$ will oscillate with periodical components both in the channel and in the ice sheet, and the wave numbers of the oscillation are $\alpha _1,\ldots ,\alpha _N$. In (3.55), because $\kappa _0 < \alpha _j < k_0$ we have that all $\gamma _{m,j}$ will be complex with a negative imaginary part. This indicates that the wave components corresponding to the trapped wave modes $\alpha _j$ will decay exponentially with $y$ away from the ice sheet edge. The decay can be seen from figure 5 for wave elevation $w_\infty$ by the asymptotic formula along the longitudinal cut at different $y$, and more clearly observed from figure 6, which shows a contour plot of $w_\infty$ as a function of $x$ and $y$.

Figure 5. The wave elevation $w$ induced by a source at $(\xi ,\eta ,\zeta )=(0,0,-1)$: (a) real part (Re) of $w_\infty$; (b) imaginary part (Im) of $w_\infty$ ($k_0=0.1$, $b=50$, $h=1\ \mathrm {m}$ is taken as the characteristic length scale).

Figure 6. The (a) real part and (b) imaginary part of wave elevation $w_\infty$ induced by a source at $(\xi ,\eta ,\zeta )=(0,0,-1)$ ($k_0=0.1$, $b=50$, $h=1\ \mathrm {m}$ is taken as the characteristic length scale).

In figure 7, the wave elevation $w$ along the longitudinal cut $y/b=0$ at large $x$ is shown for four different wave numbers, namely $k_0=0.1$, $0.2$, $0.3$ and $0.4$ (the corresponding dimensional wave radian frequencies $\omega$ are $0.99$, $1.40$, $1.71$ and $1.98 \ \mathrm {rad}\ \mathrm {s}^{-1}$). The source point $q$ is located at $(0,0,-1)$, and $w$ is computed by the asymptotic formula (3.55). The corresponding trapped modes $\alpha _j$ for each $k_0$ are provided in table 1 to four decimal places, together with the corresponding amplitude $w_j$. Because $\eta =0$, only the symmetric modes are non-zero. It can be seen from table 1 that the number $N$ of $\alpha _j$ increases with $k_0$. This leads to a more oscillatory $w$, as can be observed in figure 7. From table 1, it can be also observed that at larger $k_0$, when $j$ is close to $N$, different $\alpha _j$ are close to each other and $\alpha _N$ is very close to $k_0$. In the numerical computation, it is noted that when $\alpha =\alpha _j \pm \Delta \alpha$, the magnitudes of $a_m$, $b_m$, $c_m^+$ and $c_m^-$ are no longer exceedingly large when $\Delta \alpha = O(10^{-4})$. Here, $\alpha _{j+1} - \alpha _j = O(10^{-3})$, and $\alpha _{j+1}$ does not have a major effect on $w_j$.

Figure 7. The wave elevation $w$ induced by a source at $(\xi ,\eta ,\zeta )=(0,0,-1)$: (a) real part (Re) of $w_\infty$; (b) imaginary part (Im) of $w_\infty$ ($y/b=0$, $b=50$, $h=1\,\mathrm {m}$ is taken as the characteristic length scale).

Table 1. Trapped wave modes $\alpha _j$ at different wave number $k_0$ for an ice channel with half width $b=50$. The amplitude of each wave component $w_j$ along the longitudinal cut due to $\alpha _j$ is also provided ($(\xi ,\eta ,\zeta )=(0,0,-1)$, $y/b=0$, $h=1\ \mathrm {m}$ is taken as the characteristic length scale).

The stress (strain) of the ice sheet is associated with its possible breakup when it becomes excessive. The principal strain $\varepsilon$ can be obtained by the eigenvalues of the strain tensor matrix (Timoshenko & Woinowsky Reference Timoshenko and Woinowsky1959)

(4.3)\begin{equation} \sigma ={-}\frac{h}{2} \begin{bmatrix} \dfrac{\partial^2 W}{\partial x^2} & \dfrac{\partial^2 W}{\partial x \partial y} \\ \dfrac{\partial^2 W}{\partial x \partial y} & \dfrac{\partial^2 W}{\partial y^2} \end{bmatrix}, \end{equation}

or the solution of $\text {det}[\sigma - \varepsilon I] = 0$, which provides

(4.4)\begin{equation} \varepsilon_{1,2} ={-}\frac{h}{4} \left[ \left( \frac{\partial^2 W}{\partial x^2} + \frac{\partial^2 W}{\partial y^2} \right) \pm \sqrt{ \left( \frac{\partial^2 W}{\partial x^2} + \frac{\partial^2 W}{\partial y^2} \right)^2 + 4\left( \frac{\partial^2 W}{\partial x \partial y} \right)^2} \right]. \end{equation}

Invoking (4.2), the physical deflection of the ice sheet can be written as

(4.5)\begin{equation} W(x,y,t) = \textrm{Re}(w)\cos(\omega t) - \textrm{Im}(w)\sin(\omega t). \end{equation}

Then, at each point in the ice sheet the maximum principal strain, can be found as the largest eigenvalue $\varepsilon _M$ of $\sigma$ in (4.3) as $\omega t$ varies from $0$ to $2{\rm \pi}$. Figure 8 shows the maximum principal strain $\varepsilon _M$ induced by a source with position $(\xi , \eta , \zeta )=(0, 0, -1)$. The wave numbers are taken to be the same as those in figure 7. From figure 8(a) which gives $\varepsilon _M$ along the longitudinal cut with $y/b=1+0$, we have that $\varepsilon _M$ will increase to a maximum at a distance away from the source. When $|x-\xi |$ is very large or only the waves due to the trapped wave modes have contributions to $\varepsilon _M$, it can be expected from (3.55) that $\varepsilon _M$ will oscillate with periodical components (wave numbers as $\alpha _1,\ldots ,\alpha _N$) against $|x-\xi |$. When $x=\xi$ and $y$ varies, it can be seen from figure 8 that $\varepsilon _M$ will first decrease with $y$ and it will then increase and reach its maximum rapidly. After the maximum $\varepsilon _M$ it will decrease with $y$ slowly. From (3.55), at each of these $\alpha _j$ modes $G$ decays exponentially with $y$. However, at a finite $|x-\xi |$, in addition to these modes, there are also some local modes at which $G$ decays in form of $1/\sqrt {|y|}$ which is similar to that in the case when water surface is fully covered by an ice sheet Li et al. (Reference Li, Wu and Shi2018c).

Figure 8. The maximum principal strain $\varepsilon _M$ induced by a source at $(\xi , \eta , \zeta )=(0, 0, -1)$: (a) along the longitudinal cut with $y/b=1+0$; (b) along the transverse cut with $x/b = 0$ ($b=50$, $h=1\ \mathrm {m}$ is taken as the characteristic length scale).

4.2. Wave interactions with a body floating on the ice channel

We now consider the wave interactions with a body floating on the ice channel. The body used for this case study is a barge of length $L_L$, beam $L_B$ and draught $D$. The half beam or $L_B/2=10\ \mathrm {m}$ is chosen as the characteristic length scale. Computations are carried out for $L_L/L_B=4$ and $D/L_B=0.25$. These ratios are the same as those in Newman (Reference Newman2017) for the tank problem. The rotational centre $(x_0,y_0,z_0)$ of the barge is taken at the geometry centre. Both the wave radiation and diffraction problems are solved, and the incident flexural gravity wave is assumed to be from $\beta ={\rm \pi} /4$. The barge is assumed to float at the centre of the channel or $y_0=0$. Two channel widths are considered, i.e. $b=3$ and $b=5$. To conduct numerical computations, the body surface $S_B$ is discretized into $N_B=1408$ flat panels, as shown in figure 9. The extended interior surface $S_E$ introduced to remove the irregular frequencies is discretized into $N_E=156$ flat panels. To obtain the diffracted potential $\phi _D^1$ by the channel due to $\phi _I$, the first $M_D+1$ terms are kept in its eigenfunction expansion, and $M_D=200$ is taken for calculation. It has been observed that further increase of $N_B$ and $N_E$, $M_D$ and $M_G$ will give graphically indistinguishable results in the figures given here.

Figure 9. General view of the geometry and distribution of panels on the floating barge.

The diagonal terms of the added mass and damping coefficient for $b=3$ with $y_0=0$ and for $b=5$ with $y_0=0$ and $y_0=2$, are presented in figures 10 and 11 respectively against free surface wave number $k_0$, whereas the wave exciting forces are plotted in figure 12. The hydrodynamic forces for the barge in open sea are also provided. The wave number $k_0$ varies from $0.02$ to $4$, and the increment has been chosen to be $0.02$ to capture the more detailed oscillatory features of the curves. When $k_0$ is small, it can be seen that the hydrodynamic forces in the ice channel case are all very close to those in the open sea case. When $k_0 \to 0$ or $\omega \to 0$, both the leading term of the boundary conditions on ice sheet and free surface will be $\partial \phi _j / \partial z = 0$. This indicates that as $k_0 \to 0$ the upper surface boundary condition for ice channel will tend to be the same as that for open sea. Thus the hydrodynamic forces for ice channel will be very similar to those for open sea when $k_0$ is small.

Figure 10. Added mass $\mu _{jj}$ of a barge floating on different transverse positions of the ice channel with different widths against wave number $k_0$ ($L_L/L_B=4$, $D/L_B=0.25$, $(x_0,z_0)=(0,-D/2)$, $\beta = {\rm \pi}/4$, $L_B/2=10\ \mathrm {m}$ is taken as the characteristic length scale).

Figure 11. Damping coefficient $\lambda _{jj}$ of a barge floating on different transverse positions of the ice channel with different widths against wave number $k_0$. See the caption of figure 10 for further information.

Figure 12. Wave exciting force $f_{E,j}$ on a barge floating on different transverse positions of the ice channel with different widths against wave number $k_0$. See the caption of figure 10 for further information.

As $k_0$ increases, the hydrodynamic forces for ice channel with different widths begin to depart from each other, and all of them show an oscillatory behaviour with $k_0$ and the oscillation is around the results for open sea. The problem for a barge in a channel with two solid side walls has been studied previously, and the numerical result of Newman (Reference Newman2017) revealed that at some discrete wave numbers the wave due to the barge oscillation would not propagate to infinity along the channel or the wave would be confined near the body. At these wave numbers, the added mass would be infinite and the damping coefficient would be zero. Here, only the free surface in the channel is confined by two semi-infinite ice sheets, whereas the fluid domain below the surface still tends to infinity. This means that the radiated wave will propagate not only along the channel on the free surface, but also into the domain below the ice sheet. Therefore, no zero damping case is observed. On the other hand, as in the 2-D problem (Li et al. Reference Li, Shi and Wu2017), there will be wave motions in the channel, which may resemble a ‘transverse sloshing wave’, leading to the oscillatory behaviour of the results with the wave number.

As the channel width increases or the barge floats off-centrally, it can be seen from the figures that the hydrodynamic forces become more oscillatory. This may be partly explained by the 2-D approximate solution for a body in a wide channel (Li et al. Reference Li, Shi and Wu2017). The obtained explicit formulae reveal that the oscillatory behaviours of the hydrodynamic forces will depend on two parameters, namely $b$ and $y_0$, or the oscillation has two periods $2k_0b$ and $2k_0|y_0|$, respectively. This indicates that at a larger $b$ or a larger $y_0$, the hydrodynamic forces will oscillate more quickly with $k_0$, as observed in the figures.

4.3. Wave interactions with two bodies floating on the ice channel

As discussed in § 3 and illustrated in § 4.1, a major feature of this ice channel problem is those $\alpha _j$ waves, which do not decay along the channel. This means when there are two bodies in the channel at the same time, the wave generated by one body will significantly affect the other, even when the distance between them is relatively large. Here, we shall undertake a case study of two bodies floating in the channel. Both bodies have the same geometry shape, i.e. a truncated vertical circular cylinder with radius $a$ and draught $D$. The radius $a=10\ \mathrm {m}$ is chosen as the characteristic length scale with $D/a=0.5$ and $b=5$. The rotational center is taken at the geometry centre. The incident flexural gravity wave is assumed to be from $\beta ={\rm \pi} /4$. The first cylinder is taken to be at $x_0^1=0$, whereas three positions of the second cylinder are considered, namely $x_0^2=2.5$, $10$ and $40$, respectively. Here, the superscript $1$ and $2$ indicate the rotational centres of the first and second cylinders, respectively. To conduct numerical computations, each cylinder surface $S_B$ is discretized into $N_B=845$ flat panels, as shown in figure 13, whereas the corresponding extended interior surface $S_E$ is discretized into $N_E=153$ flat panels. The other parameters or $M_D$ and $M_G$ for the truncation of infinite summations are taken to be the same as those in § 4.2. These are found to be sufficient to provide the converged hydrodynamic forces.

Figure 13. General view of the geometry and distribution of panels on the truncated vertical circular cylinder.

The computed diagonal terms of the added mass and damping coefficient in the $O$–$xz$ plane for the first cylinder with $x_0^1=0$ are shown in figures 14 and 15, respectively, against wave number $k_0$. The corresponding wave exciting force is presented in figure 16. For full open water or $h=0$, it can be observed from the figures that when there is another body at $x_0^2$, the hydrodynamic forces on the first body at $x_0^1=0$ show that results oscillate around those of a single body. The results may become more oscillatory as $x_0^2-x_0^1$ increases. However, the amplitude of oscillation decays, which suggests the interaction between the two bodies becomes weak. This can be partially explained through the approximate formula in Srokosz & Evans (Reference Srokosz and Evans1979) based on wide spacing approximation. They showed that the results would oscillate with a period of $2k_0(x_0^2-x_0^1)$. However, the wave generated by a body in open water will decay at the rate proportional to the square root of the distance from the body. Thus, its effect in a region far away from the body will diminish. This can be seen in figures 14–16. For the case of $x_0^2=40$, the results for the first cylinder are almost the same as those for a single cylinder.

Figure 14. Added mass $\mu _{jj}$ of the first cylinder at $x_0^1=0$ against wave number $k_0$ with the second cylinder at different longitudinal position $x_0^2$: (a,c,e) ice channel; (b,d,f) open water ($D/a=0.5$, $(y_0^1,z_0^1)=(0,-D/2)$, $(y_0^2,z_0^2)=(0,-D/2)$, $b=5$, $\beta ={\rm \pi} /4$, $a=10\ \mathrm {m}$ is taken as the characteristic length scale).

Figure 15. Damping coefficient $\lambda _{jj}$ of the first cylinder at $x_0^1$ against wave number $k_0$ with the second cylinder at different longitudinal position $x_0^2$. See the caption of figure 14 for further information.

Figure 16. Wave exciting force $f_{E,j}$ on the first cylinder at $x_0^1$ against wave number $k_0$ with the second cylinder at different longitudinal position $x_0^2$. See the caption of figure 14 for further information.

In the case of the ice channel, the wave generated by the body does not always decay, because there may exist those wave components of $\alpha _j$ which oscillate with $x$ periodically as shown in § 4.1. This means that as $x_0^2-x_0^1 \to +\infty$ although the wave component with wave number $k_0$ will tend to be zero, the interactions due to the wave components of $\alpha _j$ will still be there. Then it can be expected that no matter how large $x_0^2-x_0^1$ is, the interaction effect for two bodies floating on the ice channel will always be there unless $N=0$. The interaction effect is given in figures 14–16, which show that even at $x_0^2=40$, the results for the first body still oscillate around those for a single body.

As can be seen in table 1, the values of $\alpha _j$ as well as $N$ change with $k_0$. On the other hand, the interactions between the two cylinders are expected to depend very much on $k_0(x_0^2-x_0^1)$ and $\alpha _j(x_0^2-x_0^1)$, $j=1,\ldots ,N$. As $\alpha _j$ do not form a linear relationship with $k_0$, the oscillatory behaviours of the results due to the interaction do not change periodically with $k_0$. In fact, the oscillation seems to highly erratic. In some cases, several peaks and troughs are very close to each other, especially at larger $x_0^2-x_0^1$. At larger $k_0$, the effect of the second cylinder on the added mass seems to have decreased significantly. The effect on damping and exciting force is, however, still rather strong. In fact, when the damping of the single cylinder is not small, it means that its generated wave is not negligible. Thus, the wave generated by one cylinder will still affect the other, or their mutual interactions will remain to be significant, as can be seen from figures 15(a) and 15(e).