2.1 Experimental device and protocol, measurement and processing techniques

The experiments reported below made use of silicone oil as the upper fluid, while the lower fluid was either distilled water or a mixture of distilled water and glycerin with a $79\,\%$ glycerin volume fraction. The device and measurement equipment are sketched in figure 1. Experiments were carried out in a 40 cm high glass tank with a $20~\text{cm}\times 20~\text{cm}$ cross-section. Two sides of the tank were made of B270 Superwite^TM glass to limit optical distortion. To avoid formation of a meniscus along the interface, the upper part of the glass walls was coated with the hydrophobic compound Rain-X^TM. Spheres were initially held with an inverted clamp and released gently at the top of a 35 mm long tube; the use of an inverted clamp allows the initial rotation of the spheres to be drastically reduced. To also reduce their lateral drift to a minimum, the tube diameter was selected to be slightly larger than that of the sphere in each case, i.e. different tubes were used according to the sphere diameter. In most cases, the top of the tube stood 9 cm above the interface, thus allowing the spheres to settle in the upper liquid over a 5.5 cm distance; this distance was determined to be sufficient for the spheres to almost reach their terminal velocity before encountering the interface. However, with the least viscous silicone oil, inertia effects may be large and increase the distance required for the spheres to reach their terminal velocity. Therefore another stand was used to support the tube, the top of which was located 12 cm above the interface in that case. All experiments were carried out at room temperature, in the range $20\pm 2\,^{\circ }\text{C}$ . Liquids were replaced every day or every two days. Each time they were changed, the tank was washed with a detergent liquid, then rinsed out with tap water approximately 10 times, and dried with a dry duster.

The sphere and interface contours were recorded with a PCO pco.dimax S4 high-speed video camera with a resolution of $2016\times 2016$ pixels. The acquisition rate varied from 10 frames per second in the case of very slow sphere motion to 500 frames per second for the fastest settling velocity. To reduce optical distortion caused by the interface, an Opto Engineering TC 4M 120 telecentric lens was used. The detected field of view was approximately $15.5~\text{cm}\times 8.1~\text{cm}$ .

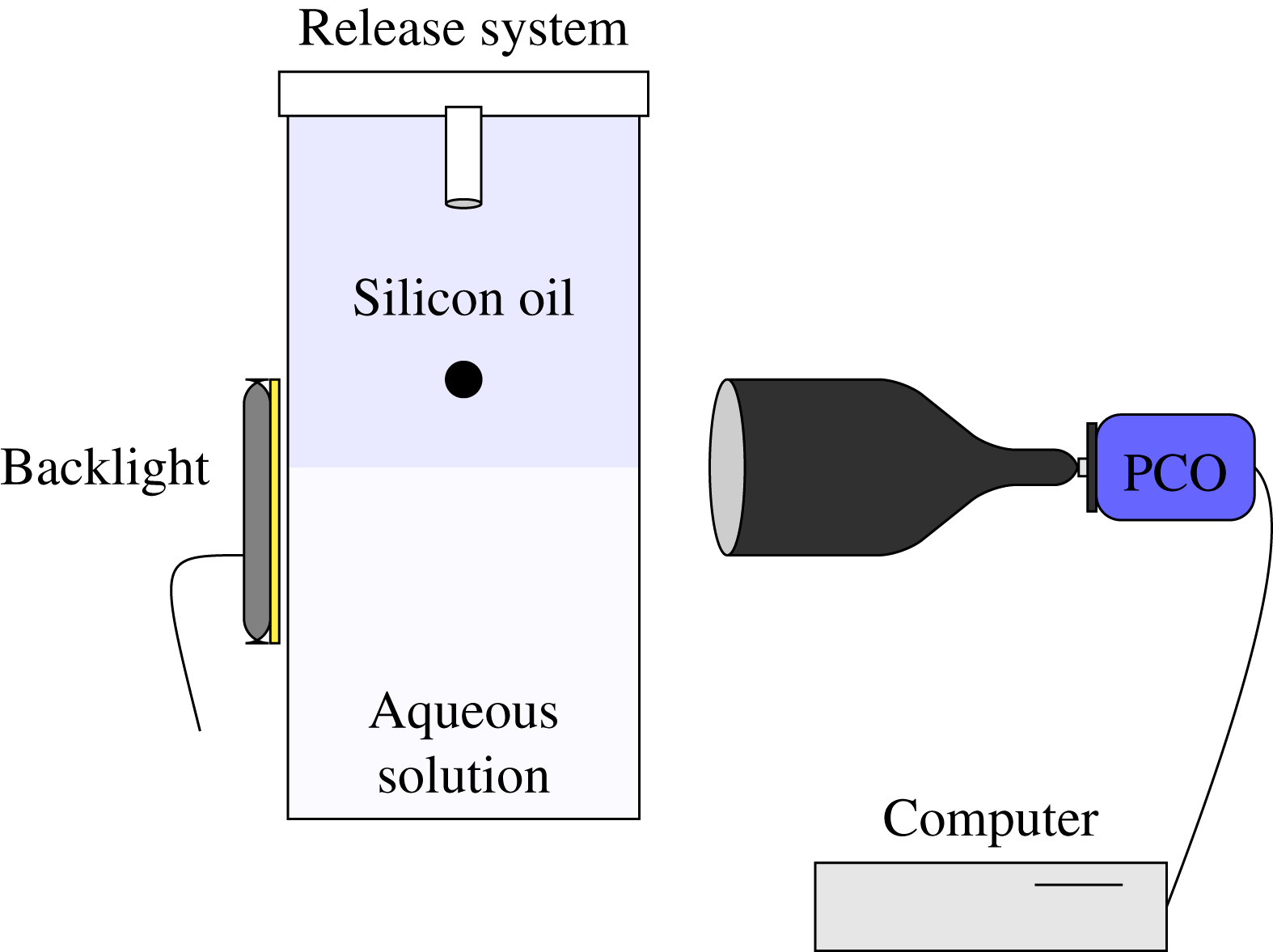
Figure 1. Sketch of the experimental device and optical measurement system.

The contours of the sphere and liquid–liquid interfaces were generally detected using a thresholding method. However, in cases where the lower fluid involved a high percentage of glycerin, the optical contrast between the two fluids was small, making this detection method inaccurate. Therefore, a gradient method was used in such cases. Whatever the detection method, the positions and surfaces detected in each frame were then tracked using a maximum likelihood detection procedure. During the interface breakthrough, optical distortions may affect the detection of the sphere contour. A Hough transform, which allows circles to be recognized on a frame, was then used to extract this contour properly.

Each experiment was repeated at least three times by waiting long enough in between two successive tests for the bath to come back to rest. The results revealed an excellent repeatability, except in the close vicinity of the flotation/sinking transition, where small disturbances, such as dust captured at the sphere surface or variation in the room temperature, could affect the time required for the sphere to detach from the interface significantly. Obviously, in cases exhibiting non-axisymmetric tails, repeatability holds only in a statistical sense, since each realization yields a different instantaneous interface geometry. Nevertheless, these restrictions do not affect the bounds of the various settling regimes to be described in § 3. Repeatability in the axisymmetric regimes can be fully appreciated in PM2, were numerous figures include data provided by the three successive tests. These data are seen to collapse very well in all cases.

2.2 The physical characteristics of the fluids and spheres

The ‘spheres’ were precision marbles manufactured by Marteau et Lemarié, made of stainless steel, aluminium, glass, Teflon (PTFE) and polyacetal (POM) respectively. For each material, we used marbles with nominal diameters of 4, 7, 10 and 14 mm. These diameters were controlled with a $\pm 0.02$  mm accuracy with a sliding calliper. The manufacturer’s specifications indicate that their departure from sphericity ranges from $0.13~\unicode[STIX]{x03BC}\text{m}$ for small steel marbles to $25~\unicode[STIX]{x03BC}\text{m}$ for polyacetal marbles, the former having a maximum roughness of $0.014~\unicode[STIX]{x03BC}\text{m}$ . Their weights were measured with a Mettler Toledo balance with a 1 mg accuracy. The corresponding material densities are given in table 1. They were obtained by averaging over five marbles made of the same material, all with a 7 mm diameter to minimize effects of volume uncertainty. The resulting accuracy of these material densities was $\pm 10~\text{kg}~\text{m}^{-3}$ .

Table 1. Marble densities.

Table 2. The physical properties of the various liquids measured at a temperature of  $20\,^{\circ }\text{C}$ .

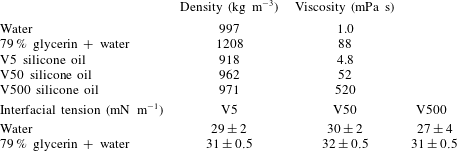
Table 2 gathers the physical properties of the various fluids or fluid pairs at a reference temperature of $20\,^{\circ }\text{C}$ . Liquid densities were measured with a glass gravity hydrometer from Thermo Fisher Scientific with a $\pm 1~\text{kg}~\text{m}^{-3}$ accuracy. Viscosities were determined with a Haake Mars III rheometer (also from Thermo Fisher Scientific). The corresponding relative uncertainty, estimated by determining the viscosity of a given liquid using different ranges of torque, was approximately 3 % for the least viscous oil and was a decreasing function of viscosity. It must be noticed that the viscosity of the water–glycerin mixture is highly temperature-dependent, varying by approximately $25\,\%$ in between $18\,^{\circ }\text{C}$ and $22\,^{\circ }\text{C}$ . Interfacial tensions were measured with a Kruss DSA 100 tensiometer using the pendant drop technique. The corresponding uncertainty depended on the pair of fluids under consideration. This is because small density differences result in approximately spherical drops, which makes the determination of the interfacial tension more difficult. The uncertainty indicated in table 2 was determined by considering maximum and minimum values obtained over five tests.

Finally, we determined the macroscopic contact angle at the marble surface in two pairs of fluids, namely V5 oil/water and V5 oil/glycerin+water. We focused on the least viscous silicone oil because it is expected to lead to the fastest film drainage; hence, it is the most favourable to a partial dewetting of the marble surface (De Gennes et al. Reference De Gennes, Brochard-Wyart and Quéré2003). To measure the contact angle, a strip of the material of which the marble was made, or in some cases the marble itself, was introduced into a bath of silicone oil. A drop of water was then released on the solid surface and the contact angle was determined using a Kruss DSA100 tensiometer. The corresponding values (averaged over three tests) are gathered in table 3, and some views of the corresponding three-phase systems are provided in figure 2. These pictures indicate that the solid surface was completely wetted by silicone oil, except in the case of glass, for which partial wetting by water was observed. However, in the course of the dynamic experiments reported below, we never observed the formation of a contact line, even with glass marbles. This is why in the theoretical models described below (and in the computational approach to be described in PM2), we always consider that the sphere is entirely surrounded by a film of silicone oil, i.e. it is never in contact with the lower fluid.

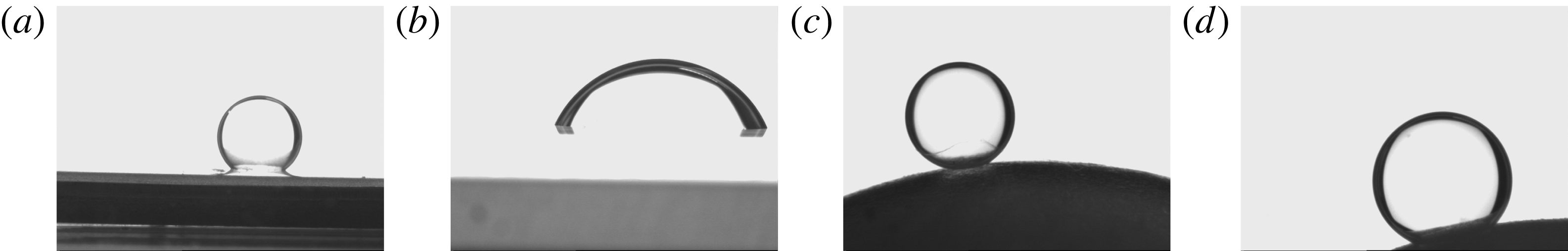
Figure 2. Contact between a drop of water and a solid surface immersed in a bath of V5 silicone oil. (a–d) Steel, glass, Teflon and polyacetal.

Table 3. The contact angle in V5 silicone oil. The superscripts $s$ and $m$ indicate that measurements were performed on a strip of material or directly on the marble respectively.

3.1 Dimensionless numbers and presentation of observations

Before we start to describe the experimental observations, a prerequisite is the definition of a proper set of dimensionless numbers characterizing the three-phase system. In what follows, index 1 (respectively 2) refers to the upper (respectively lower) fluid, while index $p$ refers to the sphere. Assuming that the tank is large enough for confinement effects to be negligible, this system is entirely defined by eight quantities, namely the three densities $\unicode[STIX]{x1D70C}_{1},\unicode[STIX]{x1D70C}_{2},\unicode[STIX]{x1D70C}_{p}$ , the two viscosities $\unicode[STIX]{x1D707}_{1},\unicode[STIX]{x1D707}_{2}$ , the interfacial tension $\unicode[STIX]{x1D6FE}$ , the sphere radius $R$ and gravity $g$ . Hence, five independent dimensionless numbers may be formed. We select the viscosity ratio $\unicode[STIX]{x1D706}=\unicode[STIX]{x1D707}_{2}/\unicode[STIX]{x1D707}_{1}$ , the fluid and solid-to-fluid density contrasts with respect to the upper fluid $\unicode[STIX]{x1D701}=\unicode[STIX]{x1D70C}_{2}/\unicode[STIX]{x1D70C}_{1}-1$ and $\unicode[STIX]{x1D701}_{p}=\unicode[STIX]{x1D70C}_{p}/\unicode[STIX]{x1D70C}_{1}-1$ respectively, the interfacial Bond number $Bo=g(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})R^{2}/\unicode[STIX]{x1D6FE}$ and the Archimedes number $Ar=\unicode[STIX]{x1D70C}_{1}(\unicode[STIX]{x1D701}_{p}g)^{1/2}R^{3/2}/\unicode[STIX]{x1D707}_{1}$ , which is merely a Reynolds number based on the gravitational velocity $(\unicode[STIX]{x1D701}_{p}gR)^{1/2}$ . With the set of fluids described in § 2.2, $\unicode[STIX]{x1D706}$ may be varied by nearly four orders of magnitude, from $\unicode[STIX]{x1D706}=1.9\times 10^{-3}$ with the combination V500/water to $\unicode[STIX]{x1D706}=18.3$ with V5/glycerin + water. With the same two pairs of fluids, $\unicode[STIX]{x1D701}$ varies by only one order of magnitude, from $\unicode[STIX]{x1D701}\approx 0.03$ to $\unicode[STIX]{x1D701}\approx 0.32$ . The sphere-to-fluid density contrast ranges from $0.40$ for polyacetal spheres in V500 oil to $7.65$ for steel spheres in V5. The interfacial Bond number varies by two orders of magnitude, from $0.038$ for the smallest spheres ( $R=2$ mm) with the V500/water pair to $4.5$ for the largest ones ( $R=7$  mm) with the V5/glycerin–water pair. Finally, the Archimedes number varies by more than three orders of magnitude, from $0.33$ with the smallest polyacetal spheres in the V500 oil to $968$ with the largest steel spheres in V5. However, in the lower fluid, the relevant gravitational velocity and viscosity are $((\unicode[STIX]{x1D70C}_{p}/\unicode[STIX]{x1D70C}_{2}-1)gR)^{1/2}$ and $\unicode[STIX]{x1D707}_{2}$ respectively, so that the relevant Archimedes number, say $Ar_{l}$ , is $Ar_{l}=(1/\unicode[STIX]{x1D706})\left((\unicode[STIX]{x1D701}_{p}-\unicode[STIX]{x1D701})(1+\unicode[STIX]{x1D701})/\unicode[STIX]{x1D701}_{p}\right)^{1/2}Ar$ . Hence, when the viscosity contrast is large, $Ar$ and $Ar_{l}$ may differ by several orders of magnitude for a given sphere. For instance, in the latter two cases with $Ar=0.33$ and $Ar=968$ , one respectively obtains $Ar_{l}=169$ and $Ar_{l}=4815$ when the lower fluid is water. To analyse some specific phenomena, it may also be appropriate to replace $Bo$ , which only involves the fluid density contrast, by a Bond number based on the solid-to-fluid density contrast with respect to the lower fluid, i.e. $Bo_{l}=g(\unicode[STIX]{x1D70C}_{p}-\unicode[STIX]{x1D70C}_{2})R^{2}/\unicode[STIX]{x1D6FE}=(\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}-1)Bo$ .

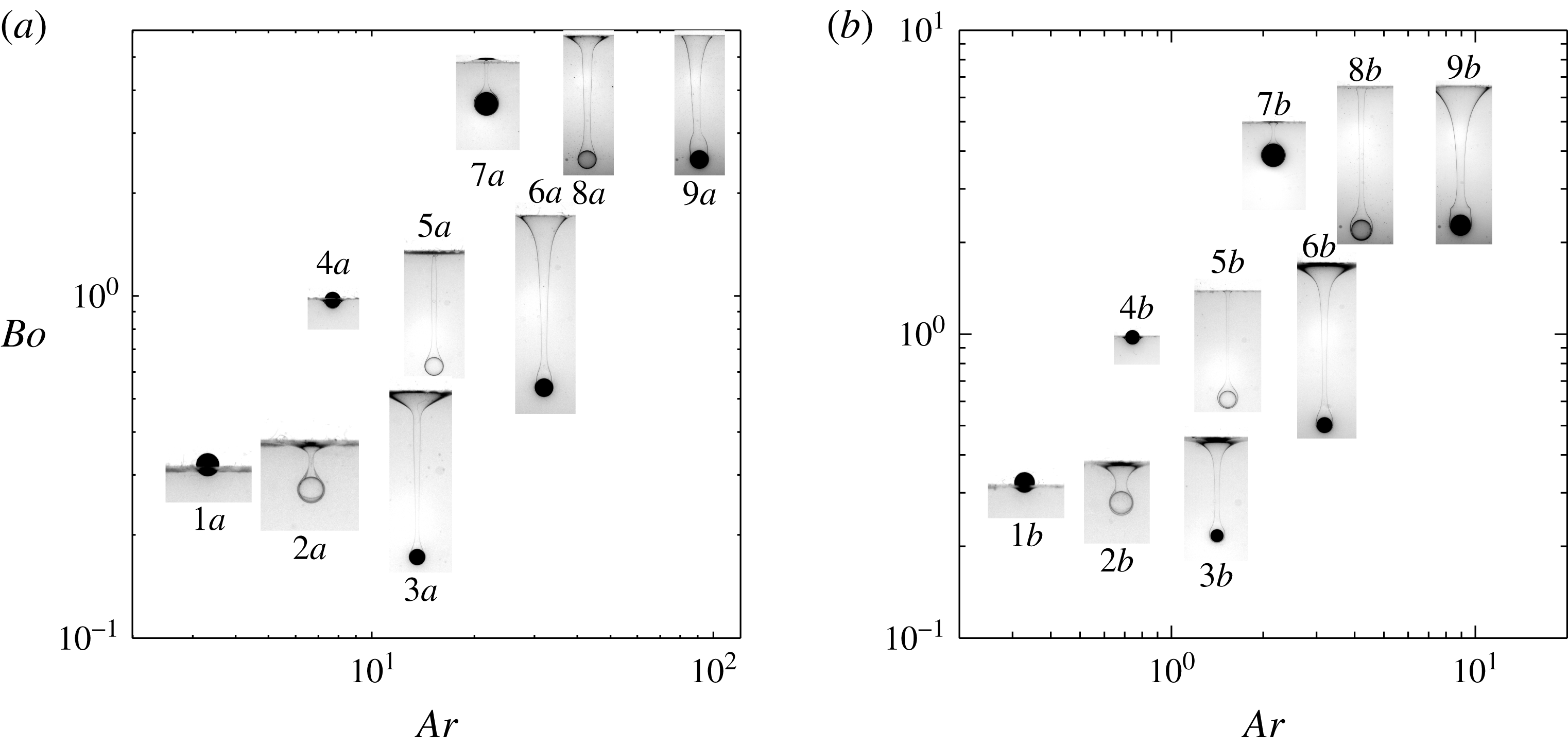
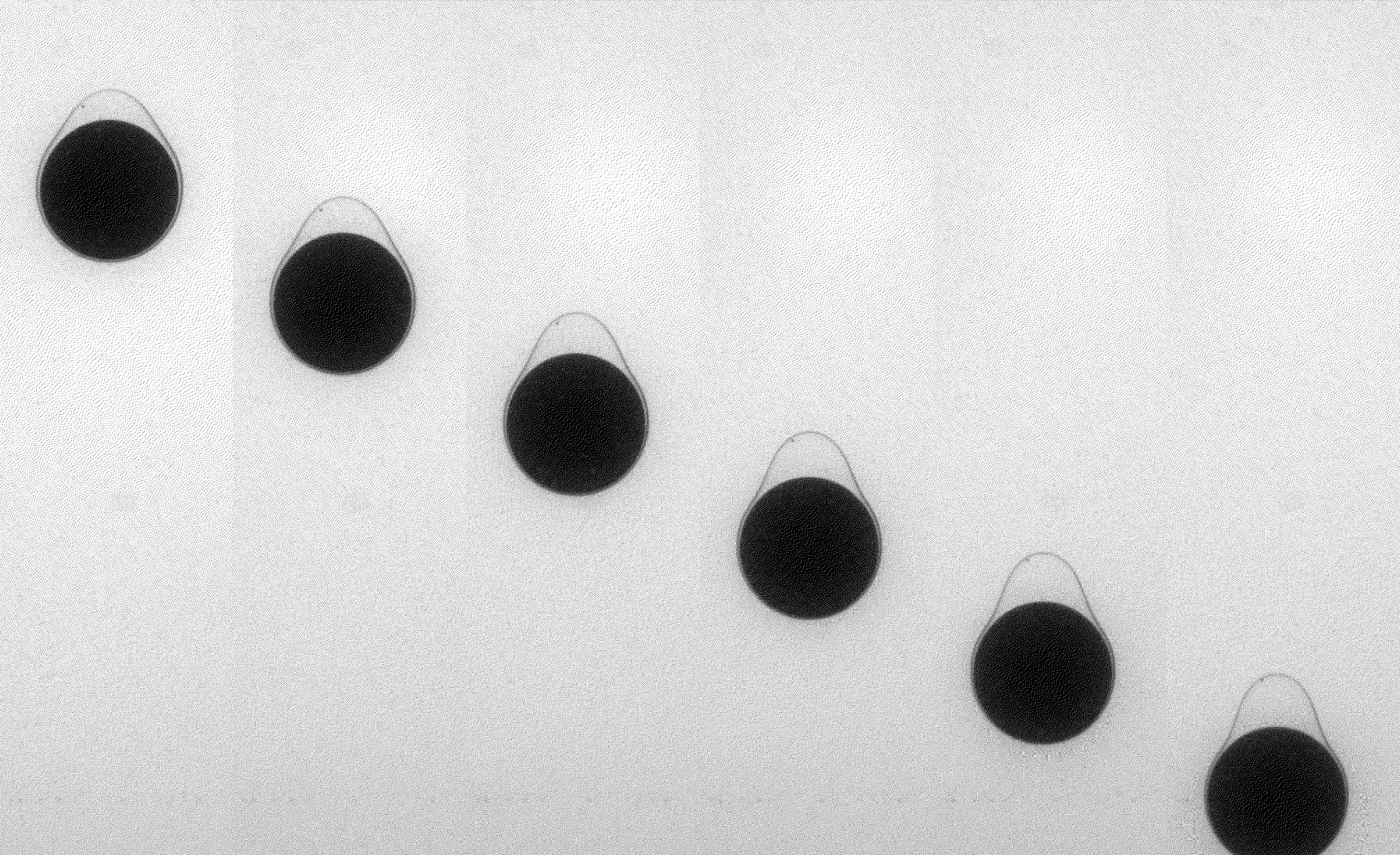
Figure 3. Some selected settling configurations for (a) the V50/water–glycerin pair $(\unicode[STIX]{x1D706}=1.7,\unicode[STIX]{x1D701}=0.26)$ and (b) the V500/water–glycerin pair $(\unicode[STIX]{x1D706}=0.17,\unicode[STIX]{x1D701}=0.24)$ . In each panel, from left to right, polyacetal, glass and steel spheres; from top to bottom, $R=7,3.5$ and 2 mm. The vertical and horizontal medians of each image are positioned on the appropriate values of $Ar$ and $Bo$ respectively.

Figure 4. The same as figure 3 for (a) the V5/water–glycerin pair $(\unicode[STIX]{x1D706}=18.3,\unicode[STIX]{x1D701}=0.32)$ and (b) the V5/water pair $(\unicode[STIX]{x1D706}=0.21,\unicode[STIX]{x1D701}=0.09)$ .

To discuss experimental observations in the five-dimensional parameter space $(\unicode[STIX]{x1D706},\unicode[STIX]{x1D701},\unicode[STIX]{x1D701}_{p},Bo,Ar)$ , we select a representation in which, for a given pair of fluids, i.e. a given $(\unicode[STIX]{x1D706},\unicode[STIX]{x1D701})$ pair, a typical view of the sphere + fluid system corresponding to a given $\unicode[STIX]{x1D701}_{p}$ is positioned in the $(Ar,Bo)$ plane. We focus on three materials, namely polyacetal, glass and steel, which, for a given sphere radius, yield ascending values of $Ar$ . We also select three sphere radii, namely 2, 3.5 and 7 mm, which, for a given material, yield ascending values of both $Ar$ and $Bo$ . We found it most convenient to split the results into three series. The first of them corresponds to fluid pairs with $\unicode[STIX]{x1D706}>0.1$ and spheres such that $Ar<10^{2}$ and $Ar_{l}<10^{2}$ , which yields strictly or approximately axisymmetric configurations (see the discussion in § 3.3). The second series covers the same range of viscosity ratios but focuses on spheres such that $Ar$ is large enough (i.e. typically ${>}10^{2}$ ) for significant non-axisymmetric effects to take place in the upper fluid and possibly in the lower one. Finally, the third series focuses on fluid pairs with small viscosity ratios $(\unicode[STIX]{x1D706}\leqslant 0.02)$ and spheres such that the flow is axisymmetric in the upper fluid $(Ar<10^{2})$ but may turn three-dimensional in the lower one because $Ar_{l}\gg Ar$ . The various snapshots showing typical interface deformations in the upcoming figures were taken at different times. Time is of course irrelevant in the case of spheres floating steadily at the interface. In tailing configurations, most pictures were taken before the tail and the sphere separated from each other. However, in figures 4 and 5, we selected some snapshots captured right after the tail pinched off, to emphasize that the primary pinch-off may take place either close to the initial position of the interface or close to the sphere. (Mechanisms yielding these two distinct pinch-off locations will be discussed in § 3.4.) Some snapshots revealing massive fragmentation in the tail are also included in figures 4(b) and 5(a). Obviously, the various snapshots do not provide any insight into the evolution of the sphere dynamics, or in most cases the evolution of the tail dynamics, especially after pinch-off has occurred. These aspects are discussed in detail in PM2 for selected axisymmetric configurations taken from figures 3–5. Nevertheless, it may be pointed out here that, in tailing regimes, pinch-off has little direct influence on the sphere velocity, large decelerations/accelerations only being observed well before it occurs.

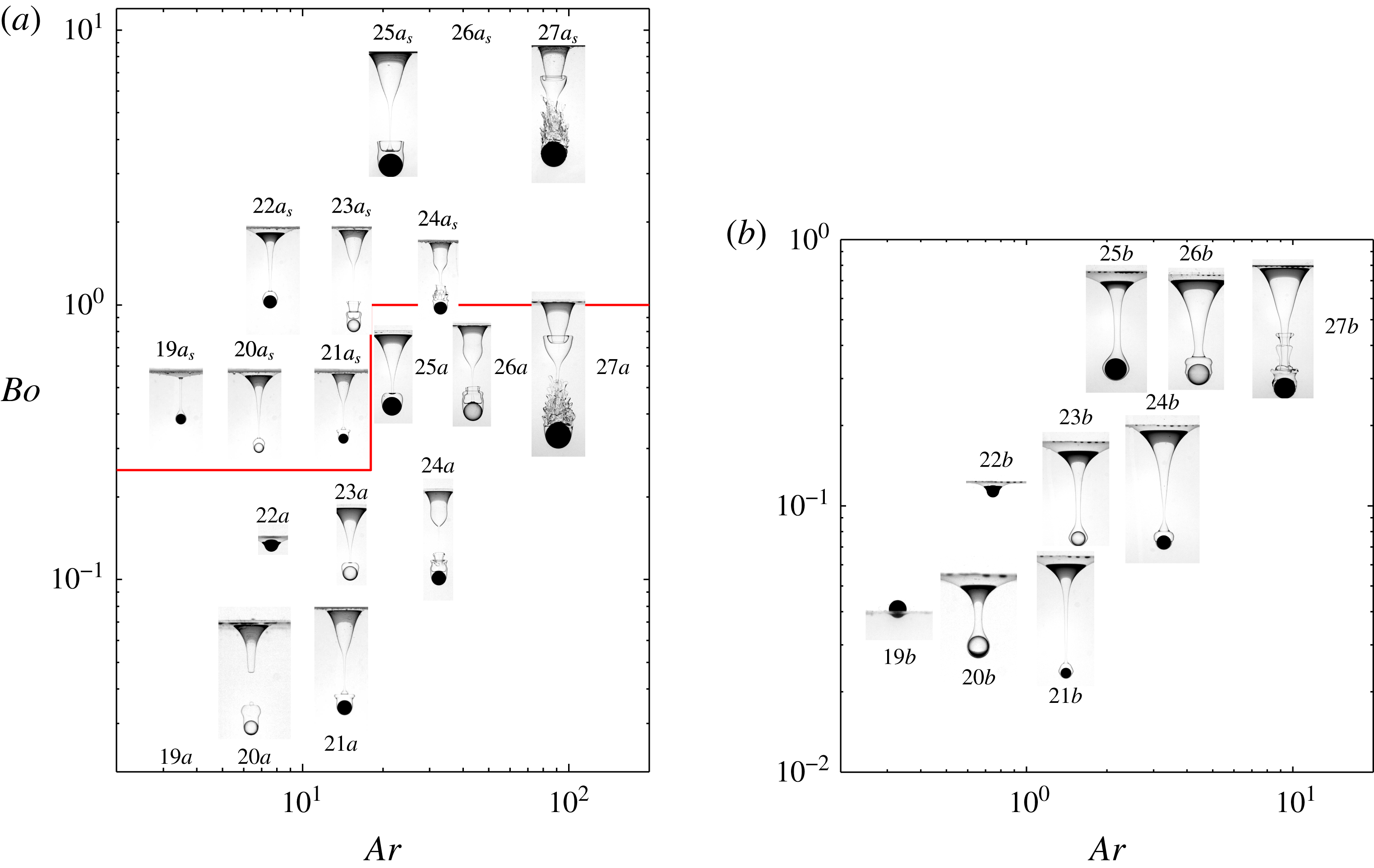
Figure 5. The same as figure 3 for (a) the V50/water pair ( $\unicode[STIX]{x1D706}=1.9\times 10^{-2}$ , $\unicode[STIX]{x1D701}=0.04$ ) and (b) the V500/water pair ( $\unicode[STIX]{x1D706}=1.9\times 10^{-3}$ , $\unicode[STIX]{x1D701}=0.03$ ). In (a), images located above the broken line were obtained in the presence of Triton X-100; in the lower (respectively upper) series, the image corresponding to the smallest polyacetal (respectively largest glass) sphere was not saved.

3.2 General observations

Figure 3 displays the first series of observations corresponding to spheres such that $Ar<10^{2}$ and $Ar_{l}<10^{2}$ in two fluid pairs, corresponding to $\unicode[STIX]{x1D706}=1.7$ and $0.17$ respectively (among the various experiments, these viscosity ratios actually vary in the ranges $1.7\pm 0.2$ and $0.17\pm 0.2$ respectively, due to the influence of temperature on the viscosity of the water–glycerin mixture). In both cases, the settling of the smallest two polyacetal spheres yields a configuration in which the sphere floats steadily at the interface (configurations $1a{-}b$ and $4a{-}b$ in the figure respectively). In contrast, the largest polyacetal sphere and the smallest glass sphere (configurations $7a{-}b$ and $2a{-}b$ respectively) succeed in crossing the interface in a slow quasi-static manner. In all other cases, although it is slowed down by capillary effects when it reaches the interface, the sphere quickly crosses its initial position and settles through the lower fluid while pulling a long tail of the upper fluid. The corresponding images in figure 3 reveal that the geometry of this tail may vary significantly from one case to another. It is almost cylindrical up to the undisturbed interface position in configurations $5a{-}b$ in both pairs of fluids as well as for $8b$ in the V500/water–glycerin pair. Moving towards the right on each row, one observes that, far above the sphere, the tail becomes more conical with a flared base for steel spheres (configurations $3a{-}b$ , $6a{-}b$ and $9a{-}b$ ), which corresponds to a larger entrainment of the upper fluid. Another feature may be noticed by examining the shape of the tail just at the back of these three spheres. From bottom to top, it is seen that this shape changes from conical to nearly cylindrical, even almost forming an angle with the rest of the tail in the case of configuration $9b$ . These changes are reminiscent of those experienced by the streamlines past a sphere in a homogeneous fluid as the Reynolds number increases, the nearly cylindrical configuration corresponding to a well-developed standing eddy. Comparing figures 3(a) and 3(b), it can be observed that tails pulled by steel spheres are thicker, especially in the top region, in the case of the most viscous oil, i.e. of the smallest $\unicode[STIX]{x1D706}$ . The same remark holds for the thin film around the sphere, which can still be discerned in most cases in (b) but not in (a), indicating that the larger $\unicode[STIX]{x1D706}$ is the faster the drainage is. Lastly, although no pinch-off has occurred by the time of the frames reported in the two panels, its precursor is present in most cases in the form of a neck slightly above the sphere. In contrast, pinch-off is about to occur at the top of the tail in configuration  $5a$ , very close to the initial position of the interface.

Figure 4 gathers observations corresponding mostly to spheres such that $Ar>10^{2}$ in two fluid pairs with $\unicode[STIX]{x1D706}=18.3$ (actually $18.3\pm 2.2$ depending on the room temperature) and $0.21$ respectively. It should be noted that for a given sphere, $Ar$ has the same value in (a) and (b) since the upper fluid is the same. Hence, differences observed between them are due to the influence of the lower fluid. This influence is obvious, starting with the smallest glass spheres (configurations 11 $a{-}b$ ) and the intermediate polyacetal spheres (configurations 13 $a{-}b$ ). The former two, which correspond to $Ar\approx 70$ , exhibit an axisymmetric tail in both pairs of fluids. However, pinch-off takes place at dramatically different locations. In configuration  $11a$ , the tail breaks right at the initial position of the interface; later on, the sphere settles with a slender column of light fluid attached to its top. In contrast, pinch-off occurs in the lower part of the tail, close to the top of the sphere, in configuration  $11b$ . The latter then goes on settling with a drop of light fluid attached to it, the volume of which is nearly three times its own volume. Similarly, the intermediate polyacetal sphere (configuration  $13a$ ) experiences a quasi-static detachment from the interface, whereas a long non-axisymmetric column of light fluid is still developing past the sphere in configuration  $13b$ .

The three-dimensional nature of the tail is prominent in most other cases, especially when $Ar$ and/or $\unicode[STIX]{x1D701}_{p}$ are/is large. In several of them, the topology of the tail strongly resembles that of vortices shed in the wake of a sphere translating in a homogeneous fluid, displaying hairpin-like structures (Sakamoto & Haniu Reference Sakamoto and Haniu1991). For a given sphere with a non-axisymmetric tail, comparison of (a) and (b) makes it clear that three-dimensional effects are significantly stronger in the latter (e.g. configurations 14–16). This is not unlikely since, for a given $Ar$ , the actual Archimedes number in the lower fluid, $Ar_{l}$ , is typically 90 times larger in (b), making the Reynolds number $Re=\unicode[STIX]{x1D70C}_{2}VR/\unicode[STIX]{x1D707}_{2}$ ( $V$ being the sphere velocity) much larger in the V5/water combination and forcing the corresponding wakes to be in a more advanced transitional stage. In the most inertial case (configuration $8b$ ), fragmentation giving rise to a broad range of droplets and ligaments of light fluid is seen to occur in (b). Premises of fragmentation may also be discerned in configuration $17b$ : thin axisymmetric corollas or ‘inverted skirts’ form at the back of the sphere and propagate downstream, becoming thinner as the distance from the sphere increases.

Observations carried out in fluid pairs with very small viscosity ratios $(\unicode[STIX]{x1D706}<0.02)$ and with spheres for which $Ar<10^{2}$ are summarized in figure 5. Under such conditions, the flow is axisymmetric in the upper fluid but may be three-dimensional in the lower one. Some three-dimensionality may be observed in both panels in the most inertial cases, especially in the fragmented region past the most inertial sphere in (a). Actually, this panel displays two distinct series of results. Those located below the broken line were obtained under nominal conditions. In contrast, configurations located above this line (with index $s$ standing for ‘surfactant’) were recorded in the presence of Triton X-100 surfactant deliberately added to the distilled water to get some more insight into the influence of the Bond number. We mixed water with a volume of $0.6~\text{g}~\text{l}^{-1}$ of Triton X-100 (which is soluble in water but not in silicone oil), corresponding to four times the critical micellar concentration, in order to saturate the interface and prevent the occurrence of any Marangoni effect, even in the presence of strong deformations. While this addition does not modify the viscosity of water, it reduces the interfacial tension by an order of magnitude, yielding $\unicode[STIX]{x1D6FE}=3.3\times 10^{-3}\pm 0.1~\text{Nm}^{-1}$ for the V50/water $+$ Triton X-100 fluid pair, instead of $30\times 10^{-3}~\text{Nm}^{-1}$ in the nominal case.

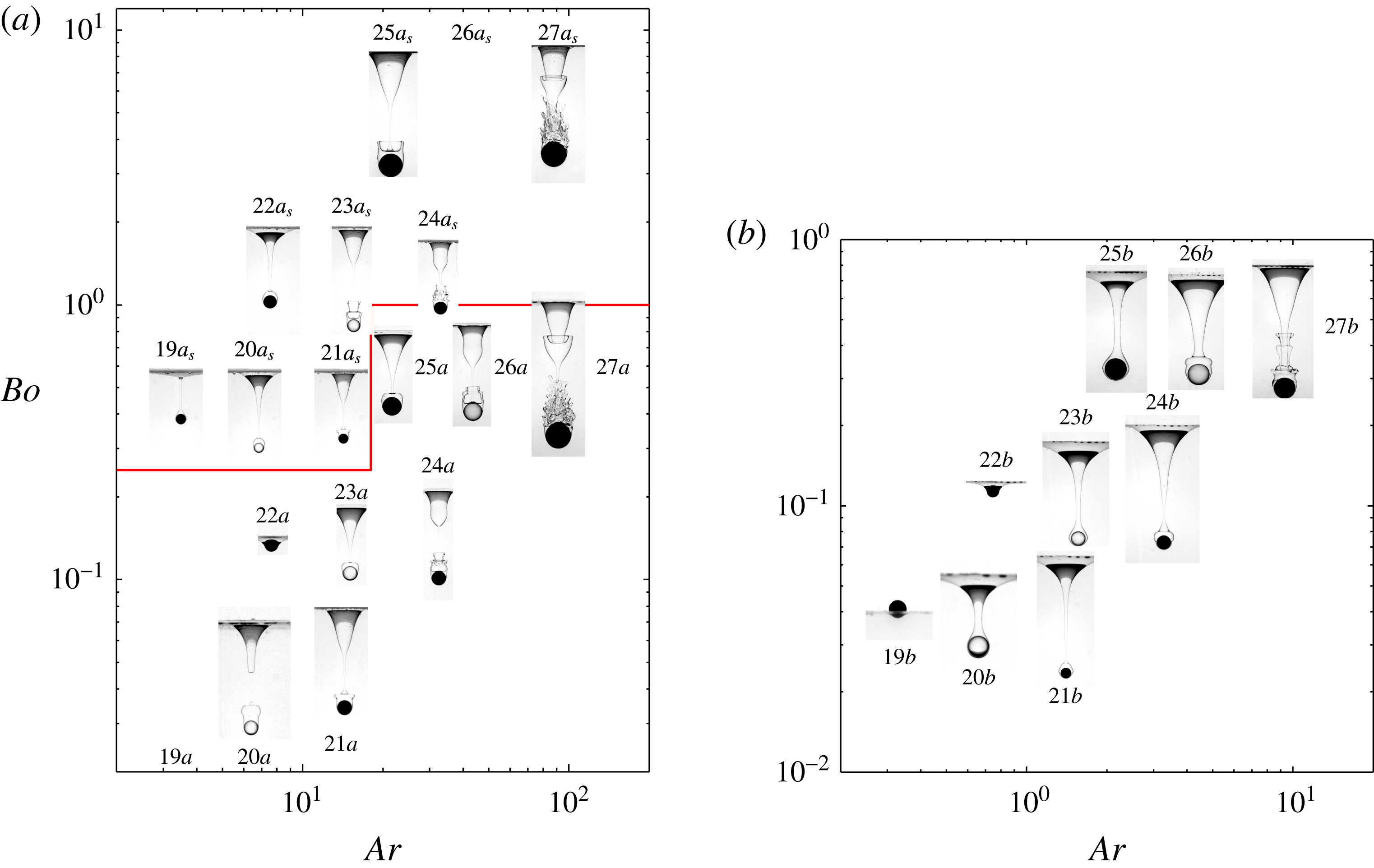
Although the smallest two polyacetal spheres float at the interface of the V50/distilled water pair (configurations $19a$ and $22a$ ; the image of the former is missing), they are seen to entrain a long tail when the interface is contaminated by Triton X-100 (configurations $19a_{s}$ and $22a_{s}$ ). These two strikingly different behaviours underline the possibility for small light spheres to float or sink, depending on the magnitude of capillary effects. In contrast, the tails developing behind a given steel sphere without or with Triton X-100 (configurations $21a-a_{s}$ , $24a-a_{s}$ and $27a-a_{s}$ respectively) exhibit very similar shapes. This indicates that capillary effects do not play a significant role when the sphere has enough inertia, at least regarding the large-scale geometry of the tail (see the discussion in § 6.2 for their effect on the small-scale structure). Again, thin corollas prefiguring fragmentation and travelling upward along the tail are visible in several cases, especially in configurations $26a$ , $23a_{s}$ and $27b$ . One should note the bulge at the surface of the film that surrounds the sphere in configurations $21b$ , $23b$ , $24b$ and $26b$ . The presence of this bulge suggests that the corollas may have some connection with disturbances born at the surface of the film; the underlying mechanism will be discussed in detail in PM2. A common feature encountered in both pairs of fluids is that tails always neck and later pinch off close to the top of the sphere, giving birth to a drop that remains stuck to it. The volume of this drop may be much larger than that of the sphere itself, as for instance in configuration  $20a$ . Finally, consideration of a given sphere successively in (a) and (b) makes it clear that the viscosity of the upper fluid still influences the evolution of the system at the stage displayed in the figure: in some cases, pinch-off has already taken place in (a) whereas it is still to occur in (b); in some others, fragmentation is already present in (a) whereas only its precursor is visible in (b), etc.

3.3 Regime maps

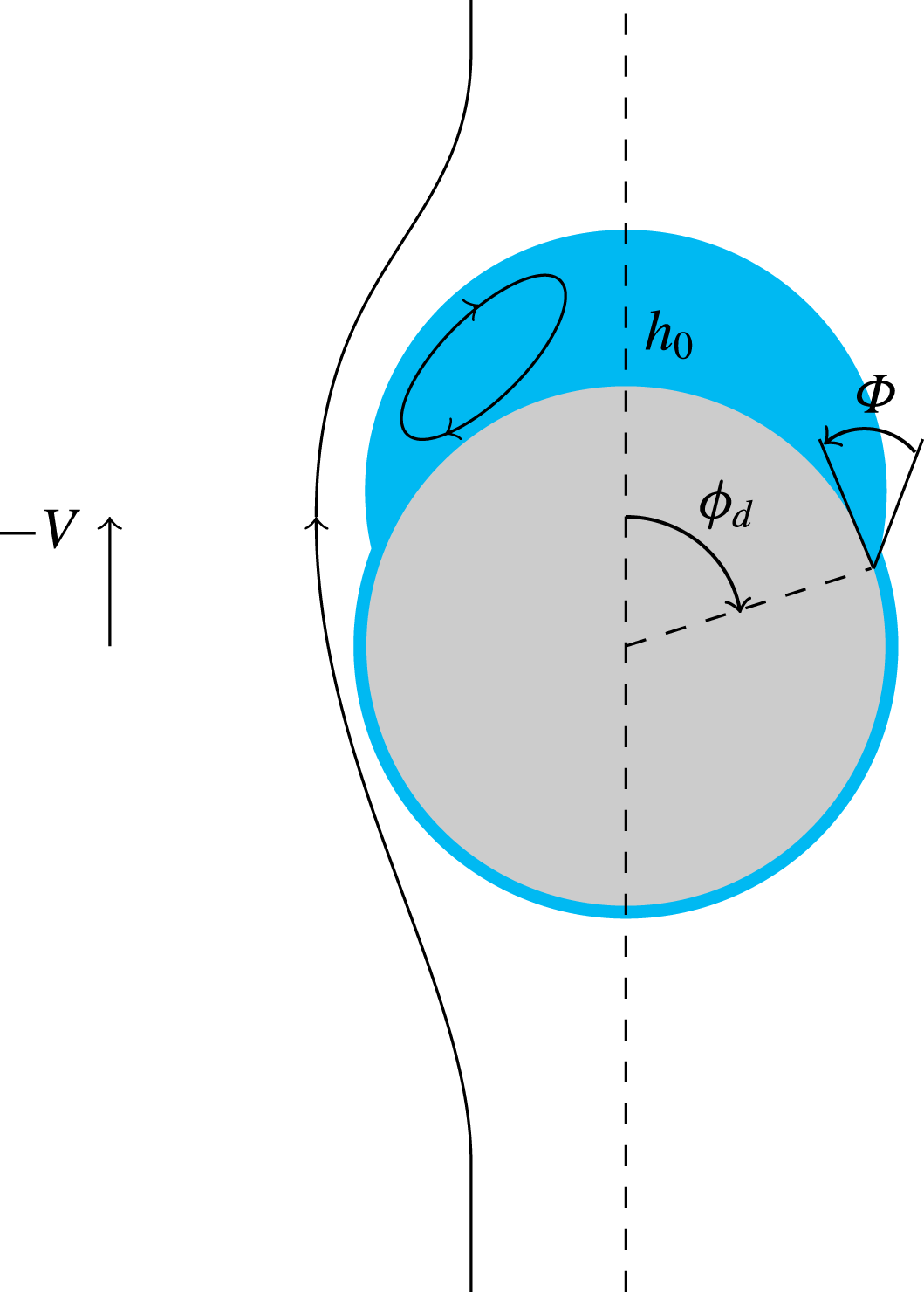
The previous observations reveal a broad variety of phenomena and some classification is desirable. It can be achieved according to the most prominent features seen in the various snapshots of figures 3–5, although such a choice is not unique. According to the mechanisms involved, we consider that the most meaningful classification is one that identifies six distinct regimes, namely flotation at the interface, quasi-steady detachment, axisymmetric and three-dimensional tailing, tailing with peripheral corollas (which may be axisymmetric or three-dimensional) and tail fragmentation. In tailing regimes, subclasses could be introduced according to the position where pinch-off takes place, or, in the post-pinch-off stage, according to the way in which the tail recedes towards the initial position of the interface. We do not consider the latter aspect here, although it will become salient in PM2. It should be noted also that the phenomenon of drops captured by the sphere is common to the quasi-steady detachment and tailing configurations and does not form a specific regime per se.

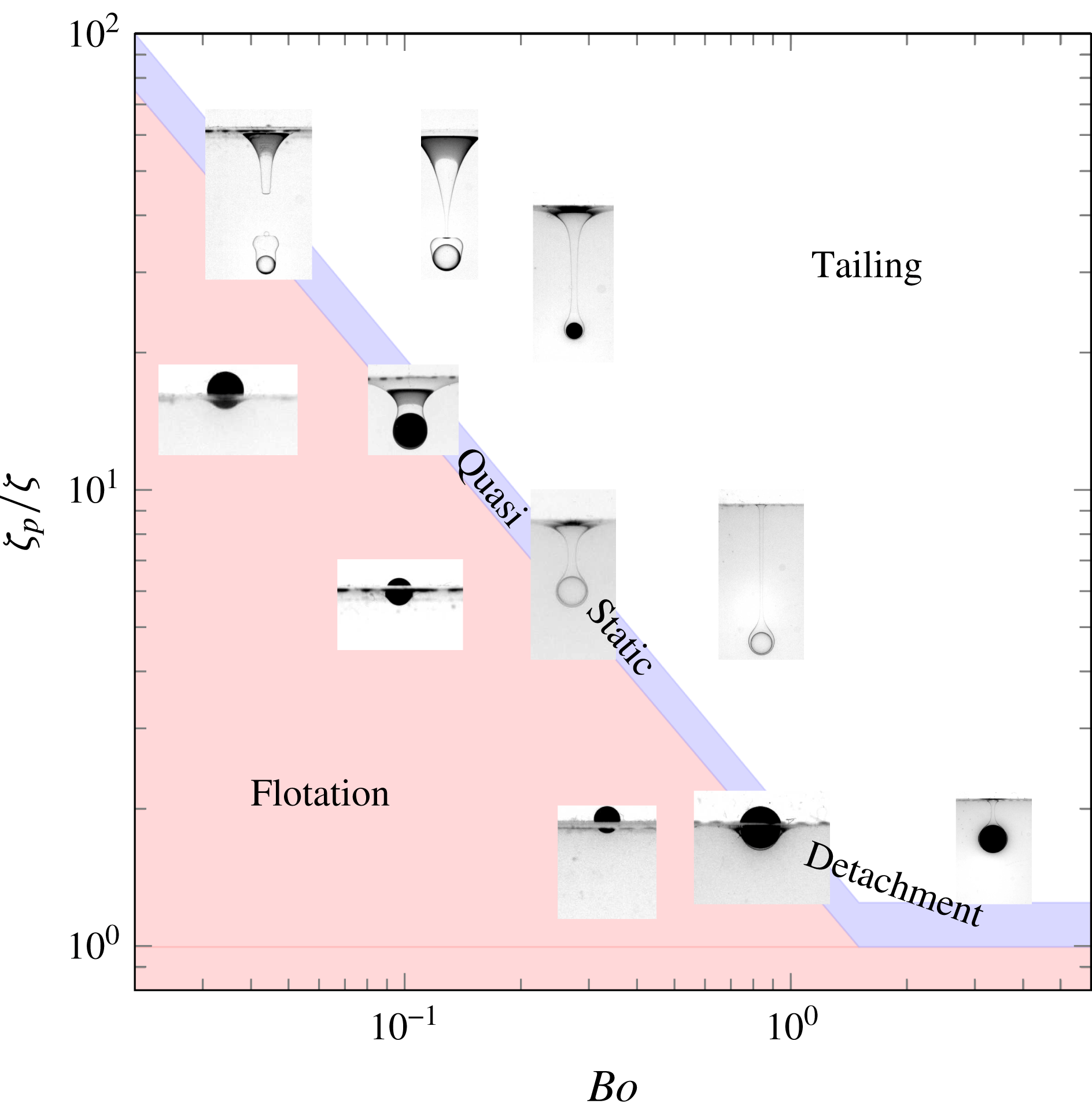
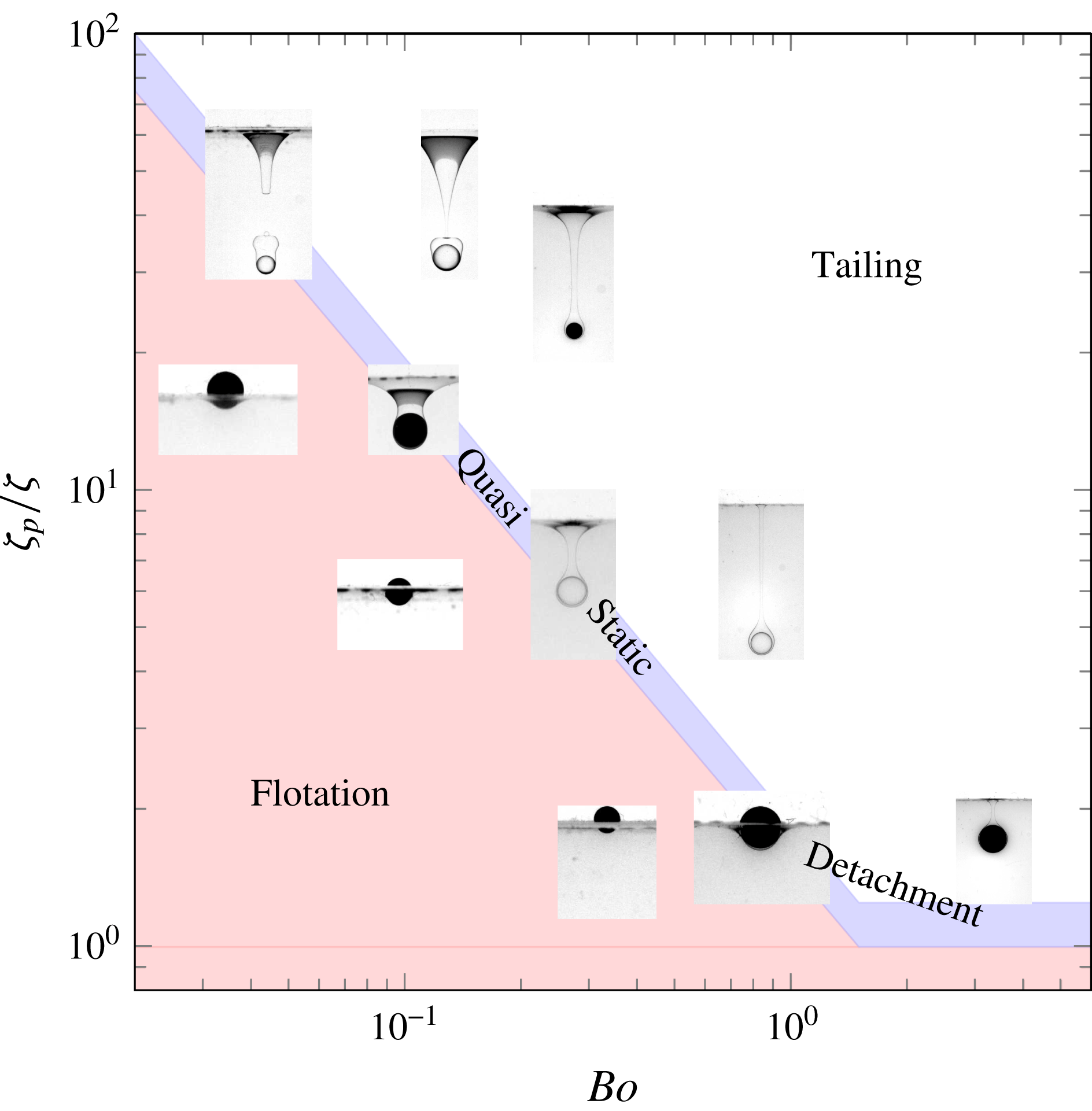
Figure 6. Regime map showing the distribution of flotation, quasi-static detachment and tailing configurations in the ( $Bo,\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ ) plane.

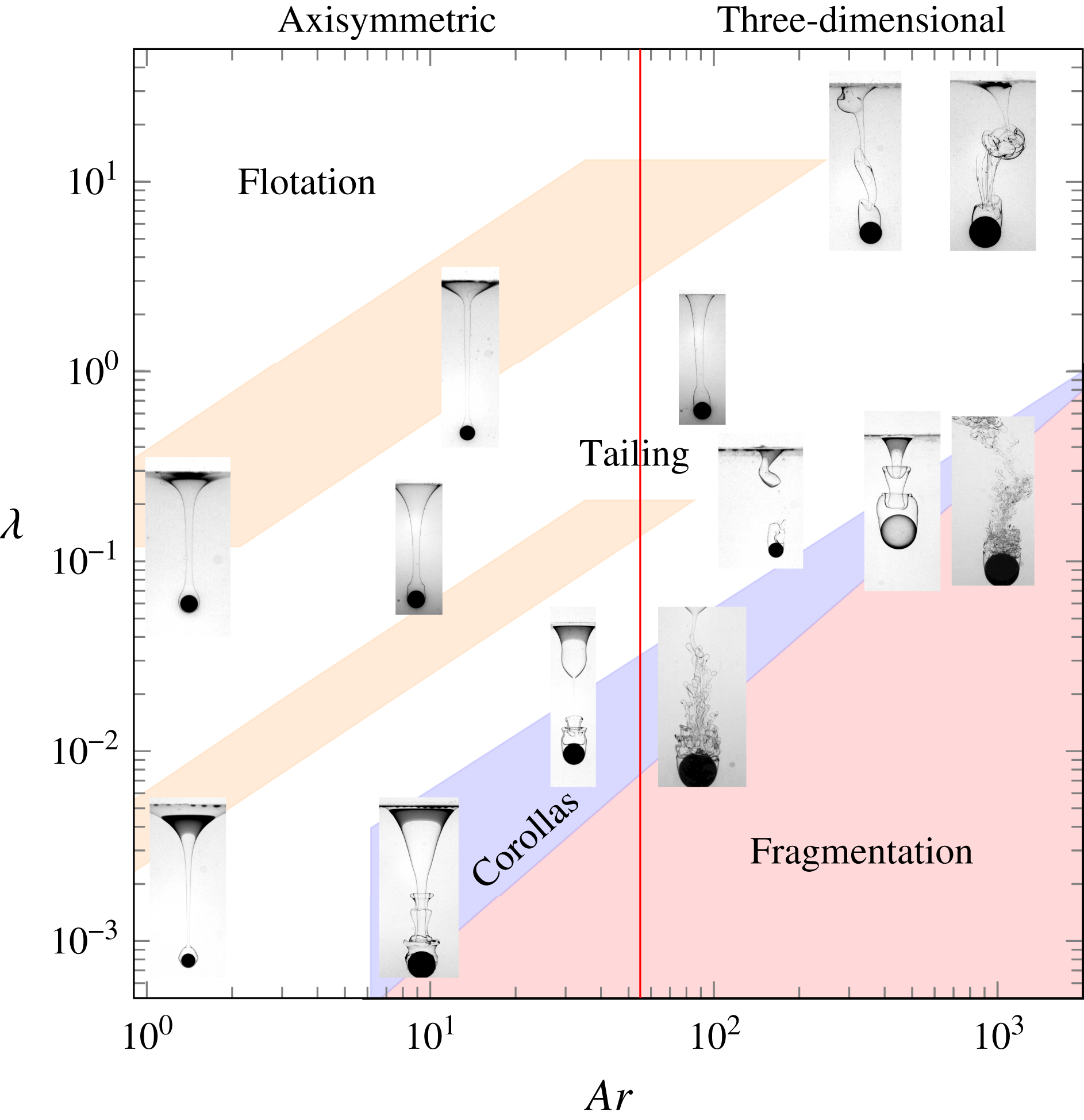
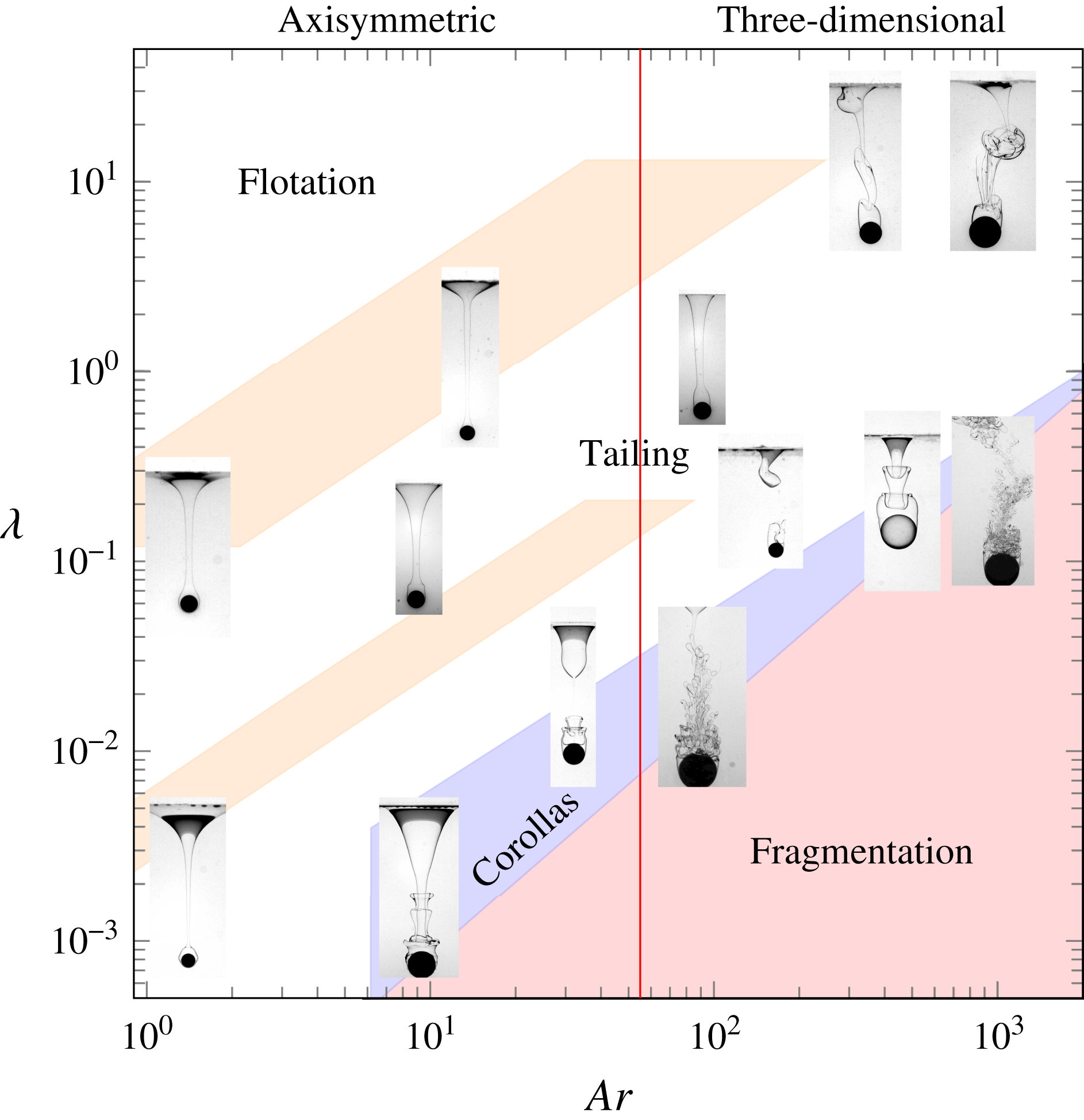
Figure 7. Regime map showing the distribution of the various tailing configurations, including those with peripheral corollas and tail fragmentation, in the ( $Ar,\unicode[STIX]{x1D706}$ ) plane. The upper (respectively lower) inclined stripe below the flotation region corresponds to the approximate position of the flotation/tailing transition and quasi-steady detachment regimes for set-ups involving the water–glycerin mixture (respectively water alone) as the lower fluid. The vertical line $Ar=55.0$ corresponds to the transition from axisymmetric to three-dimensional flow past the sphere.

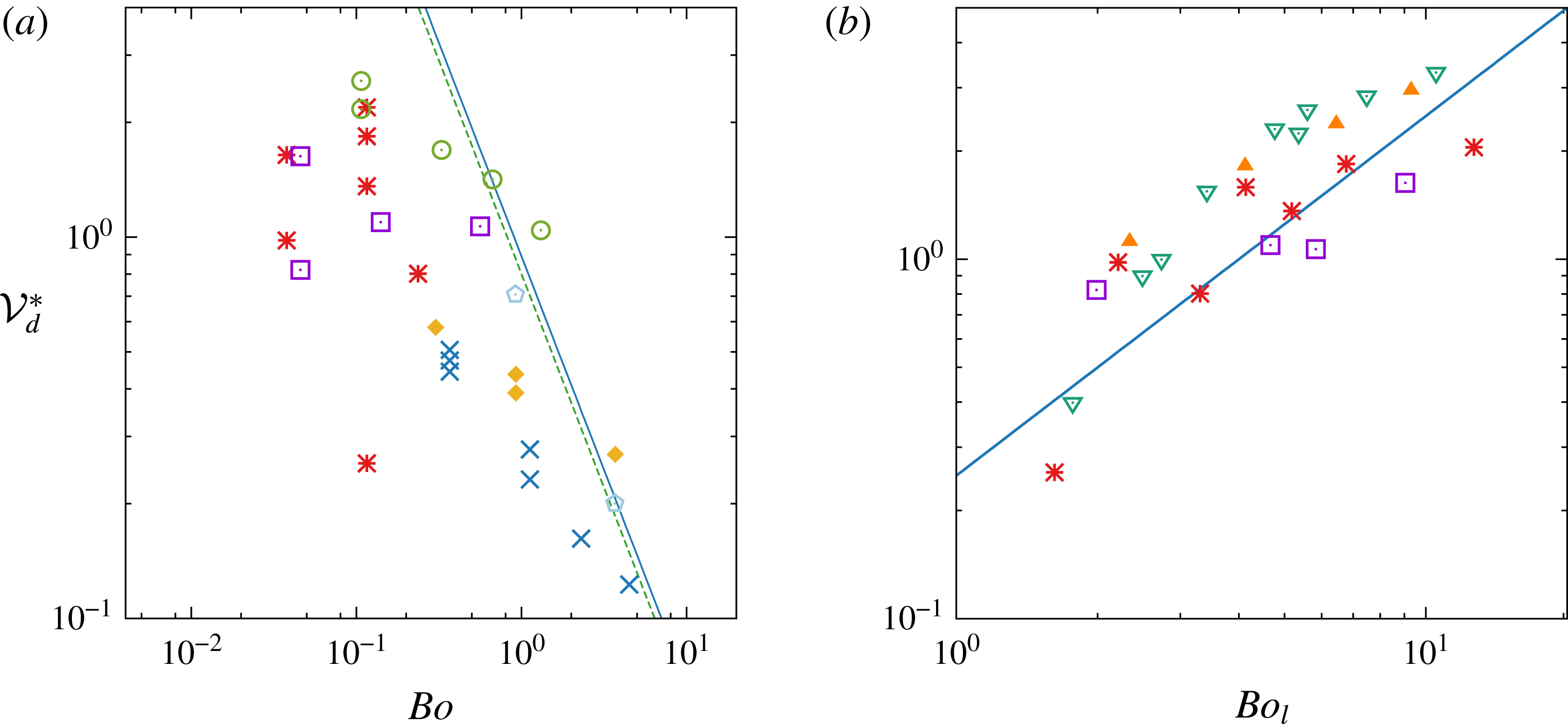
As stated in § 3.1, the problem under consideration depends on five independent parameters. However, their relative influences are not the same in all regimes, making it possible to draw approximate regime maps by selecting those that are locally most relevant. In figures 3–5, flotation and quasi-static detachment are observed near the bottom-left-hand corner, i.e. with ‘small’ ‘light’ spheres. This is an indication that, under the present conditions, these regimes are primarily governed by static forces, i.e. those due to interfacial tension, gravity and buoyancy, although dynamical effects may become important when the sphere kinetic energy is large enough, as we shall see in § 4.3. Balancing these three static forces and normalizing by the buoyancy force induced by the fluid density contrast introduces $Bo$ and $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ as the two key parameters. Figure 6 shows how some selected configurations that were found to correspond to flotation or quasi-static detachment in figures 3–5 gather in the ( $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701},Bo$ ) diagram, irrespective of the fluid pair under consideration. All floating spheres fall below a critical curve which reveals a sharp decrease of the maximum solid-to-fluid density contrast that allows the sphere to float as the Bond number increases: while capillary effects may sustain spheres much heavier than the lower fluid when $Bo\ll 1$ , buoyancy remains the only mechanism that can counteract the extra weight of the sphere when $Bo\gg 1$ (Vella Reference Vella2015). This maximum density contrast is seen to vary by more than an order of magnitude across the range spanned by the Bond number in the present experiments; its variation will be studied in more detail in § 4. Configurations in which spheres are observed to detach from the interface in a quasi-static manner gather within a narrow stripe which follows the above critical curve. The tailing regime takes place beyond this intermediate region.

Near the top-right-hand corner of figures 3–5, capillary effects do not influence the large-scale features of the observed configurations, although they may still affect the shape of the entrained column locally or the characteristics of structures that form at its periphery (corollas) or within it (ligaments and droplets). The same remark holds for the fluid density contrast, whereas the solid-to-fluid density contrast essentially determines the sphere velocity $(V\propto (\unicode[STIX]{x1D701}_{p}gR)^{1/2})$ . Therefore, the corresponding configurations are, to leading order, governed by the balance between inertial and viscous effects in both fluids, i.e. by the parameters $Ar$ and $\unicode[STIX]{x1D706}$ . Based on these remarks, figure 7 displays 13 snapshots extracted from figures 3–5, obtained in the six different pairs of fluids, in the $(Ar,\unicode[STIX]{x1D706})$ plane. Qualitative boundaries are drawn to make the succession of regimes apparent. One of these boundaries, namely the straight line corresponding to $Ar=Ar_{c}=55.0$ , is actually exact. It corresponds to the threshold beyond which flow axisymmetry past a freely falling sphere breaks down and the sphere does not follow a strictly vertical path any more, first switching to a slightly oblique path (Fabre, Tchoufag & Magnaudet Reference Fabre, Tchoufag and Magnaudet2012). Intuitively, one could think that the proper criterion to assess flow three-dimensionality in the present case should rather be based on the viscosity of the lower fluid, i.e. on $Ar_{l}$ rather than $Ar$ . However, the wake develops within the tail, i.e. within the upper fluid, making $Ar$ the relevant parameter as far as the sphere remains attached to the tail. This argument is fully confirmed in figure 7, where three-dimensional tail geometries are only observed for $Ar>Ar_{c}$ , even in cases where $Ar_{l}$ is very large (up to $5\times 10^{3}$ ). Not surprisingly, the representation used in figure 7 is of little relevance close to the flotation/tailing transition, which fails to collapse onto a single curve and actually results in two distinct stripes in the left part of the figure. Indeed, for a given fluid pair, i.e. a given $\unicode[STIX]{x1D706}$ , the flotation/tailing transition, including the quasi-static detachment regime, spans a non-negligible range of $Ar$ , the lower (respectively upper) bound of which corresponds to the smallest (respectively largest) sphere-to-fluid density contrast and Bond number at which this transition is observed in this fluid set-up. Moreover, consider a given sphere settling in two different fluid pairs, say $A$ and $B$ , which differ only by the viscosities of each fluid, i.e. $\unicode[STIX]{x1D707}_{1}^{A}\neq \unicode[STIX]{x1D707}_{1}^{B}$ and $\unicode[STIX]{x1D707}_{2}^{A}\neq \unicode[STIX]{x1D707}_{2}^{B}$ , but have the same $\unicode[STIX]{x1D706}$ , i.e. $\unicode[STIX]{x1D706}^{A}=\unicode[STIX]{x1D706}^{B}$ . Then, although $Bo$ and $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ are unchanged, the Archimedes numbers associated with the sphere in the two pairs are such that $Ar^{A}/Ar^{B}=\unicode[STIX]{x1D707}_{1}^{B}/\unicode[STIX]{x1D707}_{1}^{A}=\unicode[STIX]{x1D707}_{2}^{B}/\unicode[STIX]{x1D707}_{2}^{A}$ . If $Bo$ and $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ have values corresponding to the flotation/tailing transition in figure 6, the two points $(Ar^{A},\unicode[STIX]{x1D706})$ and $(Ar^{B},\unicode[STIX]{x1D706})$ are distinct in figure 7, and the larger the difference between $\unicode[STIX]{x1D707}_{2}^{B}$ and $\unicode[STIX]{x1D707}_{2}^{A}$ is, the larger their separation is. As the water–glycerin mixture has a viscosity that is 88 times that of water, the critical $Ar$ corresponding to this transition for a given $\unicode[STIX]{x1D706}$ is much larger in the series involving water alone, which yields the two distinct stripes in figure 7.

On increasing $Ar$ along an iso- $\unicode[STIX]{x1D706}$ line, two different successions of regimes are observed, depending on whether $\unicode[STIX]{x1D706}$ is larger or smaller than a critical value $\unicode[STIX]{x1D706}_{c}\approx 0.1$ . For $\unicode[STIX]{x1D706}\geqslant \unicode[STIX]{x1D706}_{c}$ , starting from flotation and quasi-static detachment, one successively encounters axisymmetric tails (when the lower fluid is the water–glycerin mixture), then three-dimensional tails, followed by tails with peripheral corollas and eventually tail fragmentation. In contrast, for $\unicode[STIX]{x1D706}<\unicode[STIX]{x1D706}_{c}$ (which is achieved only when water is used as the lower fluid), peripheral corollas develop directly on axisymmetric tails and fragmentation takes place for values of $Ar$ of the order of $Ar_{c}$ (as may be noticed in figure 5, the present experiments with $\unicode[STIX]{x1D706}=1.9\times 10^{-3}$ were limited to $Ar\approx 10$ , so that fragmentation was not observed at such small viscosity ratios, leaving the fragmentation threshold undetermined). For $Ar\gtrsim 10$ , decreasing $\unicode[STIX]{x1D706}$ while keeping $Ar$ constant, one progressively moves from an axisymmetric or three-dimensional tailing regime to tailing with peripheral corollas and eventually tail fragmentation. Keeping in mind that the sphere settles within a fluid at rest at infinity, this succession may be understood by considering the flow region within which velocity gradients are most intense: large (respectively small) $\unicode[STIX]{x1D706}$ corresponds to situations in which shear is large within (around) the tail. Hence, for small $\unicode[STIX]{x1D706}$ , the flow within the tail looks essentially like a plug-type jet peeled off by a strong outer shear, a situation known to be prone to fragmentation (Villermaux Reference Villermaux2007).

3.4 Where does pinch-off take place?

In tailing regimes, the observations reported in figures 3–5 show that the tail may break first very close to the initial position of the interface (e.g. configurations $5a$ , $11a$ and $14a$ ), close to the sphere (e.g. $11b$ , $12b$ and $20a$ ) or at some intermediate position (e.g. $16b$ and $17a$ ) in strongly three-dimensional configurations. Among these distinct pinch-off locations, the first two are reminiscent of the ‘shallow seal’/‘deep seal’ classification defined by Aristoff & Bush (Reference Aristoff and Bush2009) in the case of a sphere impacting an air–water surface. To better appreciate how the pinch-off position is influenced by the various control parameters in the present case, we considered all axisymmetric or weakly three-dimensional tailing configurations identified experimentally (including those observed with Teflon and aluminium spheres which do not appear in figures 3–5), plus some results from direct numerical simulations carried out by varying $Bo,Ar,\unicode[STIX]{x1D706},\unicode[STIX]{x1D701}$ and $\unicode[STIX]{x1D701}_{p}$ independently (the corresponding numerical approach is described in § 2 of PM2). On analysing these results, we noticed that $Ar$ has a weak effect on this position, while $\unicode[STIX]{x1D701}$ and $\unicode[STIX]{x1D701}_{p}$ influence it very significantly but essentially through the ratio $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ , similarly to what happens for the flotation/tailing transition. We also observed significant changes with the Bond number in the range $0.1<Bo<5$ , and a fairly sharp transition with the viscosity contrast for $\unicode[STIX]{x1D706}\approx 0.1$ .

Figure 8. Pinch-off configurations observed in experiments and axisymmetric simulations in fluid set-ups with (a) $\unicode[STIX]{x1D706}>0.1$ and (b) $\unicode[STIX]{x1D706}\leqslant 0.1$ . In (a), ▿, quasi-static detachment in V5/wg, V50/wg and V500/wg (wg stands for water–glycerin); ▴ (brown) and ▵, shallow and ‘hesitant’ seals in V5/wg respectively; ▪ (brown), ▫ and ▾ (green), shallow, ‘hesitant’ and deep seals in V50/wg respectively; $\odot$ and 

, ‘hesitant’ and deep seals in V500/wg respectively; ♦ (brown) and ⚫ (green), shallow and deep seals in V5/w respectively (w stands for water); $\times$ , $+$ and *, shallow, ‘hesitant’ and deep seals with $\unicode[STIX]{x1D706}=1$ (numerical). In (b), ▿, quasi-static detachment in V5/w and V50/w; ▫ and  ▪, ‘hesitant’ and deep seals in V50/w respectively; ♢ and ♦, ‘hesitant’ and deep seals in V500/w respectively; ▴, deep seal in V50/w+Triton X-100; *, deep seal with $\unicode[STIX]{x1D706}=0.1$ (numerical).

Based on these observations, figure 8 provides the distribution of the pinch-off style (i.e. ‘shallow’ or ‘deep’) in the $(Bo,\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})$ plane, separately for $\unicode[STIX]{x1D706}>0.1$ and $\unicode[STIX]{x1D706}\leqslant 0.1$ . In the former case, only axisymmetric or weakly three-dimensional configurations have been considered, since the tail dynamics is enslaved to wake instabilities in fully three-dimensional cases, and pinch-off can then occur at various positions, depending on the details of the shedding process. Some other configurations could not be included because pinch-off took place out of the field of view of the camera, so that its position could not be guaranteed. In addition to shallow and deep types, figure 8 also identifies situations corresponding to quasi-static detachment plus ‘hesitant’ configurations, where pinch-off occurs almost simultaneously at the top and near the bottom of the tail (an example of such situations is discussed in § 4.2 of PM2). According to figure 8(b), only deep-seal-type events are observed beyond the quasi-static detachment regime when $\unicode[STIX]{x1D706}\leqslant 0.1$ . In contrast, for $\unicode[STIX]{x1D706}>0.1$ , such events mostly occur either for $Bo\gtrsim 3$ or for $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}\gtrsim 10$ . For $Bo\lesssim 3$ and $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}\lesssim 10$ , most pinch-off events take place near the top part of the tail, corresponding to the shallow-seal configuration, but a significant number of ‘hesitant’ configurations also exist. Some complex behaviours may be noticed in figure 8(a), suggesting more subtle influences than just those of $Bo$ and $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ . For instance, at $Bo\approx 0.3$ , pinch-off is found to be ‘shallow’ for both $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}\approx 12$ and $30$ in the V50/water–glycerin set-up, whereas it is ‘deep’ for $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}\approx 16$ and 20 in the V5/water set-up.

To rationalize these observations, scaling arguments are developed in appendix A. As far as the sphere velocity $V$ does not change significantly during the breakthrough, i.e. it still scales as $(\unicode[STIX]{x1D701}_{p}gR)^{1/2}$ , the main conclusions are summarized in (A 1). According to these predictions, a ‘shallow’ pinch-off due to capillary effects occurs at a dimensionless time $\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D6FE}}^{\ast }$ (normalized by $(\unicode[STIX]{x1D701}_{p}g/R)^{1/2}$ ) such that $\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D6FE}}^{\ast }\sim (\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})Bo^{1/2}$ . It is readily seen that $\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D6FE}}^{\ast }$ represents the dimensionless length of the tail by the time pinch-off occurs in that configuration; the $Bo^{1/2}$ scaling is confirmed by the tails seen in figure 4 for configurations $14a$ and $11a$ : the former is $1.8$ longer than the latter, close to the $1.75$ ratio of the square roots of the corresponding Bond numbers. Conversely, a ‘deep’ pinch-off due to buoyancy effects occurs at a dimensionless time $\unicode[STIX]{x1D70F}_{g}^{\ast }\sim (\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})^{1/3}$ , and the transition between the two regimes takes place at a critical Bond number $Bo_{t}\sim (\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})^{-1/3}$ , only deep (respectively shallow) seal being possible at larger (respectively smaller) Bond numbers. The scaling $Bo_{t}\sim (\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})^{-1/3}$ suggests a fairly sharp transition between the two styles of pinch-off, as $Bo_{t}$ only varies by a factor of $2$ when $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ is varied by one order of magnitude. Unfortunately, the data gathered in figure 8 for which the assumption of a nearly constant settling velocity is realistic (i.e. essentially those corresponding to the V50/water–glycerin set-up) do not cover a sufficient range of density contrasts to check this prediction.

The situation is more complex than expected on the basis of the above scalings in most cases, due to the acceleration or deceleration of the sphere after it has crossed the interface. As shown in appendix A, variations in the sphere velocity result in an additional ‘buoyancy’ effect which shortens (respectively lengthens) the characteristic time $\unicode[STIX]{x1D70F}_{g}^{\ast }$ when the sphere accelerates (respectively decelerates), thus decreasing (respectively increasing) the effective critical Bond number $Bo_{t}$ according to (A 2). The amplitude of the settling velocity variations may be estimated through the ratio of the terminal velocity in the lower fluid to that in the upper one. According to (A 4), this ratio varies approximately as ${\mathcal{C}}(1-\unicode[STIX]{x1D701}/\unicode[STIX]{x1D701}_{p})^{3/5}$ , with ${\mathcal{C}}=\unicode[STIX]{x1D706}^{-1/5}(1+\unicode[STIX]{x1D701})^{-2/5}$ , provided that $Ar$ and $Ar_{l}$ are large enough. In the V50/water (respectively V500/water) pairs of fluids, ${\mathcal{C}}$ is approximately $2.15$ (respectively $3.4$ ). All spheres considered here are such that $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}>10$ in these set-ups, so that they all experience significant acceleration effects, which favour deep seal. Figure 8 confirms this trend, as no ‘shallow’ pinch-off is observed in (b), especially in the range $Bo<1,10<\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}<30$ , where several shallow configurations are identified in (a). In the V5/water–glycerin mixture set-up, ${\mathcal{C}}\approx 0.50$ , implying a strong deceleration whatever the sphere-to-fluid density contrast. This is why, in that set-up, there exists a range of shallow-seal configurations extending beyond the critical Bond number predicted under the assumption of a constant $V$ . In contrast, the V5/water and V50/water–glycerin set-ups have ${\mathcal{C}}\approx 1.33$ and $0.82$ respectively, so that the settling velocity of dense enough spheres is expected to slightly increase in the former and experience little variation in the latter. In figure 8(a), examination of the critical Bond number corresponding to the shallow seal/deep seal transition in the range $4\lesssim \unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}\lesssim 6$ fully confirms the influence of acceleration/deceleration effects anticipated on the above basis: while this transition takes place in the range $1<Bo<2$ in the V50/water–glycerin set-up ( ${\mathcal{C}}\approx 1$ ), it occurs in the range $0.3<Bo<0.6$ in the V5/water system ( ${\mathcal{C}}>1$ ) and most probably beyond $Bo=3$ in the V5/water–glycerin set-up ( ${\mathcal{C}}<1$ ) since a shallow pinch-off is still detected for $Bo=2.2$ . The above findings indicate that, under the present conditions, the viscosity ratio primarily affects the pinch-off dynamics through the sphere acceleration or deceleration, although other viscous effects are possible when $\unicode[STIX]{x1D706}$ is large, as mentioned in appendix A.

The pinch-off configurations encountered here are reminiscent of those previously described in the context of spheres impacting a free surface (Truscott et al. Reference Truscott, Epps and Belden2014). However, despite obvious similarities, such as the role of capillary and buoyancy forces in the shallow and deep styles of pinch-off respectively, many differences exist. For instance, no splash is observed at the interface in the present experiments, whereas spectacular splash curtains are known to take place when a hydrophobic sphere impacts a free surface with a large enough velocity, a situation yielding a specific ‘surface-seal’ configuration (Aristoff & Bush Reference Aristoff and Bush2009). Connections and fundamental differences between the tail dynamics that develop in liquid–liquid systems and the dynamics of air cavities that form in impact situations are further discussed in appendix B.

4.1 A static model in the low- and high-Bond-number limits

The flotation of small objects at a fluid interface is a generic problem with a wide range of applications, from the removal of impurities in steel elaboration to the locomotion of water-walking insects, through the design of encapsulation processes (Vella Reference Vella2015). To rationalize the present observations, a model predicting the conditions under which a sphere with a relative density contrast $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ can float steadily at an arbitrary Bond number is required. In this section, we establish such a model under strictly static conditions and compare its predictions with our full set of observations. To derive this model, which has several parts in common with previous work on the subject (Maru et al. Reference Maru, Wasan and Kintner1971; Vella, Lee & Kim Reference Vella, Lee and Kim2006; Bonhomme et al. Reference Bonhomme, Magnaudet, Duval and Piar2012), we assume that the sphere surface stands entirely in fluid 1, its bottom part, say $z\leqslant z_{s}$ (with $z_{s}\leqslant 0$ ), being coated with a very thin film of that fluid, which defines the cap angle $\unicode[STIX]{x1D713}$ from the negative $z$ semi-axis to the pseudo-contact line between the sphere and the plane $z=z_{s}$ (figure 9).

Neglecting the film thickness and assuming total wetting, the vertical component of the capillary force acting on the pseudo-contact line is $F_{\unicode[STIX]{x1D6FE}}=2\unicode[STIX]{x03C0}\unicode[STIX]{x1D6FE}R\text{sin}^{2}\unicode[STIX]{x1D713}$ . The buoyancy force experienced by the sphere may be shown to be

(4.1) $$\begin{eqnarray}F_{B}=g\{\unicode[STIX]{x1D70C}_{1}({\mathcal{V}}-{\mathcal{V}}_{spc})+\unicode[STIX]{x1D70C}_{2}{\mathcal{V}}_{spc}+(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1}){\mathcal{V}}_{cyl}\}.\end{eqnarray}$$

The first two contributions in (4.1) correspond to the buoyancy force that would exist if there were no meniscus, ${\mathcal{V}}_{spc}=(1/3)\unicode[STIX]{x03C0}R^{3}(2-3\text{cos}\unicode[STIX]{x1D713}+\text{cos}^{3}\unicode[STIX]{x1D713})$ being the volume of the spherical cap located below the plane $z=z_{s}$ . The last contribution is due to the shift introduced by the meniscus between that plane and the undisturbed interface $z=0$ . Keller (Reference Keller1998) (see also Mansfield, Sepangi & Eastwood (Reference Mansfield, Sepangi and Eastwood1997)) showed that this shift results in an additional buoyancy force proportional to the density difference $\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1}$ and acting on the vertical cylinder of volume ${\mathcal{V}}_{cyl}=-z_{s}\unicode[STIX]{x03C0}(R\text{sin}\unicode[STIX]{x1D713})^{2}$ which stands on the pseudo-contact line and is limited by the above two planes. The sphere can float at the interface only if the sum of the above two forces exceeds its weight $F_{P}=-\unicode[STIX]{x1D70C}_{p}{\mathcal{V}}g$ . Introducing the dimensionless ‘submergence’ $z_{s}^{\ast }=z_{s}/R$ , this condition yields the inequality

(4.2) $$\begin{eqnarray}\frac{3}{2Bo}\text{sin}^{2}\unicode[STIX]{x1D713}-\frac{\unicode[STIX]{x1D701}_{p}}{\unicode[STIX]{x1D701}}+\frac{1}{4}(2-3\text{cos}\unicode[STIX]{x1D713}+\text{cos}^{3}\unicode[STIX]{x1D713})-\frac{3}{4}z_{s}^{\ast }\text{sin}^{2}\unicode[STIX]{x1D713}\geqslant 0.\end{eqnarray}$$

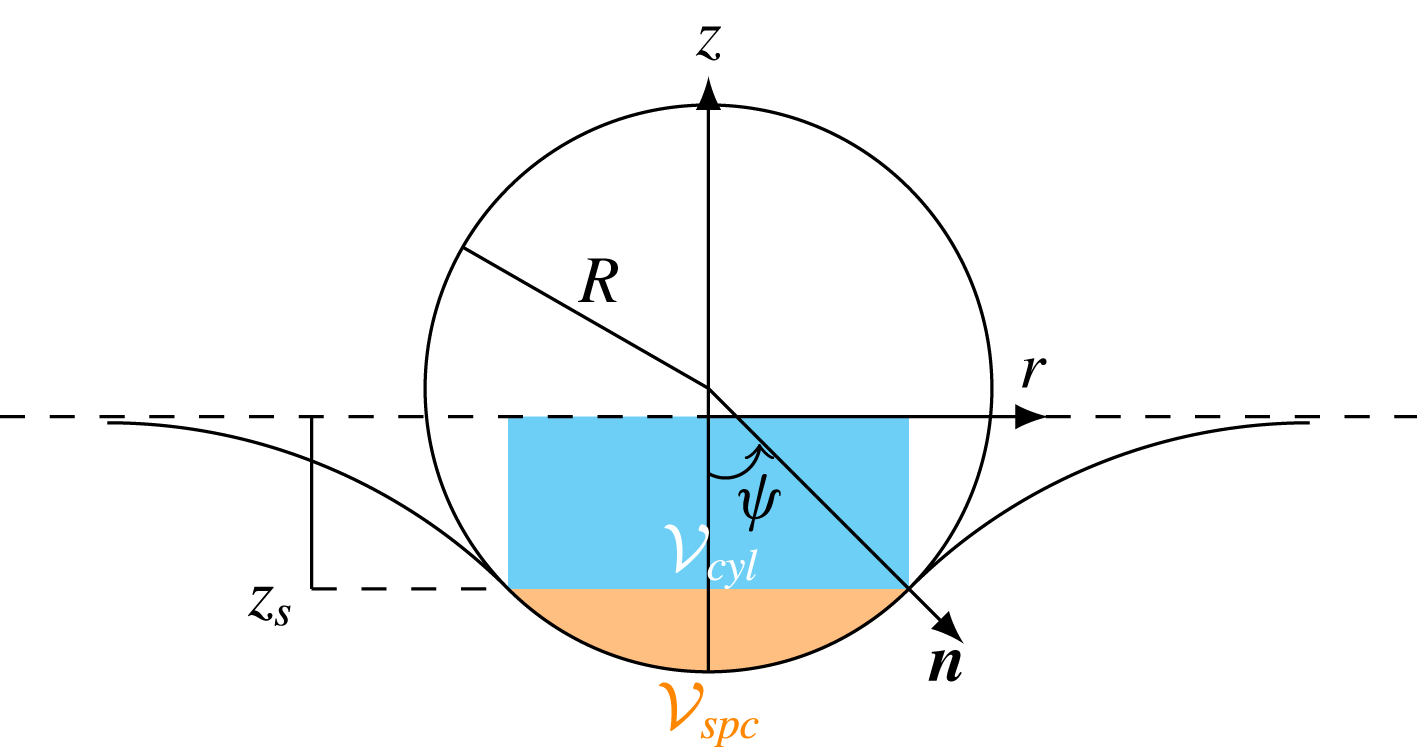
Figure 9. A sphere standing at the interface.

Evaluation of the left-hand side of (4.2) requires $z_{s}$ to be known as a function of $\unicode[STIX]{x1D713}$ , which amounts to determining the shape of the meniscus. This shape is governed by the Young–Laplace equation $(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})gz=\unicode[STIX]{x1D6FE}\unicode[STIX]{x1D735}\boldsymbol{\cdot }\boldsymbol{n}(z)$ , where $\boldsymbol{n}$ is the unit normal to the meniscus surface, subject to suitable boundary conditions. The solution of the corresponding problem can only be obtained numerically when the Bond number is finite. However, in the small-Bond-number limit, the problem was solved by O’Brien (Reference O’Brien1996), using a matched asymptotic expansion procedure directly inspired by that developed by James (Reference James1974) for a circular cylinder. In the present notation, his result for the submergence $z_{s}^{\ast }$ is written, assuming total wetting, as

(4.3) $$\begin{eqnarray}\displaystyle z_{s}^{\ast }(\unicode[STIX]{x1D713})=\left(\unicode[STIX]{x1D6FE}_{E}-\text{log}\frac{4}{\sqrt{Bo}(\text{sin}\unicode[STIX]{x1D713}(1+\text{cos}\unicode[STIX]{x1D713}))}\right)\text{sin}^{2}\unicode[STIX]{x1D713}+O\left(Bo\left[\text{log}(\sqrt{Bo})\right]^{2}\right),\end{eqnarray}$$

where $\unicode[STIX]{x1D6FE}_{E}\simeq 0.577$ is the Euler constant. The submergence is seen to be a decreasing function of the Bond number. Indeed, for a given $\unicode[STIX]{x1D713}$ , the smaller $Bo$ is the larger the energy required to bend the interface is, and hence the broader the region over which it deviates from its initial flat shape is. Inserting (4.3) into (4.2), one first has to notice that, for small Bond numbers, the most efficient configuration regarding the magnitude of positive terms on the left-hand side is reached when $\unicode[STIX]{x1D713}=\unicode[STIX]{x03C0}/2$ . Hence, flotation is possible only for spheres such that

(4.4) $$\begin{eqnarray}\frac{\unicode[STIX]{x1D701}_{p}}{\unicode[STIX]{x1D701}}\leqslant \frac{3}{2Bo}+\frac{1}{2}+\frac{3}{4}\left(\text{log}\frac{4}{\sqrt{Bo}}-\unicode[STIX]{x1D6FE}_{E}\right).\end{eqnarray}$$

The leading-order term on the right-hand side is identical to that obtained by Vella et al. (Reference Vella, Lee and Kim2006), still in the low-Bond-number limit. It is worth noting that comparison of the submergence determined by solving the exact Young–Laplace problem numerically (see PM2) with the low- $Bo$ asymptotic prediction (4.3) reveals that the latter (hence the flotation criterion (4.4)) remains a valid approximation up to $Bo=O(1)$ : for $Bo=1$ , (4.3) overestimates the submergence by $20\,\%$ , so that (4.4) overestimates the critical $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ only by $6\,\%$ .

It must be stressed that (4.4) only provides a necessary condition for flotation to be possible. As it was derived in the framework of a strictly static approach, it is unable to account for the influence of any initial sphere motion, which is expected to lower the critical density ratio, allowing the sphere to float, i.e. its velocity to vanish before the meniscus snaps. Incorporation of dynamic effects requires the viscous drag acting on the sphere to be evaluated. This was empirically achieved by Lee & Kim (Reference Lee and Kim2011) in the case of a sphere crossing a gas–liquid interface, by considering that the local velocity and pressure distributions over the part of the sphere surface immersed in the liquid are identical to those found in the Stokes flow past a sphere settling in an unbounded fluid. Extension of this approach to the present two-fluid problem is appealing, but the viscosity contrast would generate a pressure jump across the interface, making the conclusions doubtful.

For finite Bond numbers, the shape of the meniscus can only be determined through a numerical solution of the Young–Laplace problem. Nevertheless, a rough model assuming that the meniscus takes the form of an arc of a circle with a radius $r_{\unicode[STIX]{x1D713}}$ has been derived to evaluate the minimum size allowing rigid spheres or gas bubbles to fall or rise across an interface (Maru et al. Reference Maru, Wasan and Kintner1971; Bonhomme et al. Reference Bonhomme, Magnaudet, Duval and Piar2012). The requirement that the meniscus be tangent to the sphere on the plane $z=z_{s}$ as well as on the undisturbed interface yields $r_{\unicode[STIX]{x1D713}}=\{-1+[1+4Bo(1-\cos \unicode[STIX]{x1D713})]^{1/2}\}\{2Bo(1-\cos \unicode[STIX]{x1D713})\}^{-1}$ , from which the submergence $z_{s}^{\ast }=-r_{\unicode[STIX]{x1D713}}(1-\cos \unicode[STIX]{x1D713})$ is obtained. In the limit of large Bond numbers, this model predicts $z_{s}^{\ast }\propto Bo^{-1/2}$ , so that the dominant terms in (4.2) are now those due to the buoyancy force. Consequently, the cap angle $\unicode[STIX]{x1D713}_{max}$ at which this force is maximum is close to $\unicode[STIX]{x03C0}$ . More precisely, one finds $\unicode[STIX]{x1D713}_{max}\approx \unicode[STIX]{x03C0}-2(2Bo)^{-1/4}$ , for which $z_{s}^{\ast }\approx -(2/Bo)^{1/2}$ . It should be noted that the column enclosed within the meniscus is very thin under such conditions (its minimum dimensionless radius is $2(2Bo)^{-1/4}$ ), but pinch-off is not reached yet. Use of these results in (4.2) yields the approximate flotation condition

(4.5) $$\begin{eqnarray}\frac{\unicode[STIX]{x1D701}_{p}}{\unicode[STIX]{x1D701}}\leqslant 1+\frac{3}{2Bo}+O(Bo^{-3/2}).\end{eqnarray}$$

The criteria (4.4) and (4.5) coincide for $Bo\approx 2.5$ . Fortuitously, the prefactor of the $Bo^{-1}$ term is the same in both of them. Hence, as may be seen in figure 10, there is not much difference between the two predictions in the low- $Bo$ range, although (4.5) is not expected to be valid there.

4.2 Observations versus model predictions

Figure 10. The configurations reached by spheres settling in various pairs of fluids in the $(\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}=(\unicode[STIX]{x1D70C}_{p}-\unicode[STIX]{x1D70C}_{1})/(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1}),Bo=(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})gR^{2}/\unicode[STIX]{x1D6FE})$ plane. Closed (respectively open) symbols refer to cases in which the sphere floats at (respectively crosses) the interface. Solid line, low- $Bo$ criterion (4.4); dashed line, high- $Bo$ criterion (4.5); dash-dotted line, exact solution of the Young–Laplace problem; squares, V5/water–glycerin; circles, V50/water–glycerin; triangles (up), V500/water–glycerin; pentagons, V5/water; diamonds, V50/water; triangles (down), V500/water. Data from Maru et al. (Reference Maru, Wasan and Kintner1971) corresponding to $+$ , floating conditions (plexiglas and Teflon spheres in a mineral oil/water- $96\,\%$ glycerin set-up); $\times$ , breakthrough conditions (glass spheres in a cyclohexanol/water- $62\,\%$ glycerin set-up).

Figure 10 gathers observations performed in the six pairs of fluids in which, depending on the sphere density and size, either flotation or tailing configurations were noticed. Some data recorded by Maru et al. (Reference Maru, Wasan and Kintner1971) have also been included. Not surprisingly, the shape of the frontier separating the two regimes is qualitatively similar to that drawn in the phase diagram of figure 6. In addition to the approximate criteria (4.4) and (4.5), we also plot the prediction obtained by solving the Young–Laplace equation numerically throughout the whole range of Bond numbers (the corresponding numerical procedure is described in appendix B of PM2). This numerical solution allowed us to obtain the submergence $z_{s}^{\ast }(\unicode[STIX]{x1D713},Bo)$ for arbitrary values of $\unicode[STIX]{x1D713}$ . With this information at hand, we could determine the optimum cap angle $\unicode[STIX]{x1D713}_{o}(Bo)$ maximizing $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ in the inequality (4.2) for each value of $Bo$ ( $\unicode[STIX]{x1D713}_{o}(Bo)$ ranges from $\unicode[STIX]{x03C0}/2$ for $Bo\lesssim 1$ to approximately $3\unicode[STIX]{x03C0}/4$ for $Bo=10$ ). The difference between this exact prediction and those provided by the approximate criteria is clearly small, with a maximum of approximately $15\,\%$ in the range $1.5\lesssim Bo\lesssim 3$ . Overall, the behaviour observed in experiments is consistent with the theoretical predictions. Not surprisingly, some experiments corresponding to conditions located slightly below the critical curve provided by (4.4) resulted in a tailing configuration. They may be considered as a confirmation of the fact that the above two criteria only provide a necessary condition for flotation to be possible. However, experimental uncertainty regarding the interfacial tension may also play some role, since it is directly reflected in the critical value of $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ . Departures from the total wetting assumption may also have also some influence in these marginal cases: if the meniscus makes a finite angle, say $\unicode[STIX]{x1D6F7}$ , with the tangent to the sphere at the pseudo-contact line, it is readily shown that the $\sin ^{2}\unicode[STIX]{x1D713}$ factor in front of the first term on the right-hand sides of (4.2) and (4.3) is changed into $\sin \unicode[STIX]{x1D713}\sin (\unicode[STIX]{x1D713}-\unicode[STIX]{x1D6F7})$ , whereas the $\cos \unicode[STIX]{x1D713}$ term in (4.3) is changed into $\cos (\unicode[STIX]{x1D713}-\unicode[STIX]{x1D6F7})$ . For small $Bo$ , the total buoyancy force sustaining the sphere is then maximized when $\unicode[STIX]{x1D713}\approx \unicode[STIX]{x03C0}/2+\unicode[STIX]{x1D6F7}/2$ instead of $\unicode[STIX]{x1D713}=\unicode[STIX]{x03C0}/2$ , so that its dominant contribution is reduced by a factor of $(1/2)\cos ^{2}\unicode[STIX]{x1D6F7}$ .

Two situations observed in the V5/water–glycerin pair may be noticed to lead to tailing configurations, although the corresponding values of $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ clearly satisfy (4.4). These are the two points $Bo=0.37$ , $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}=4.29$ and $Bo=1.12$ , $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}=1.53$ in figure 10. The latter, which is analysed in detail in PM2 with the help of numerical simulations, corresponds to configuration 13 $a$ in figure 4(a), which was seen to experience a quasi-steady detachment. It is of interest to notice that, among the various combinations of fluids we employed, the V5/water–glycerin pair is the one in which inertia effects induced by the sphere motion are the largest in the upper fluid, and deformation of the interface is the most energy-consuming, due to the large viscosity ratio ( $\unicode[STIX]{x1D706}=18.3$ ) and the significant fluid density contrast ( $\unicode[STIX]{x1D701}=0.32$ ). Consequently, the deceleration experienced by the sphere when it reaches the interface is maximum in this pair of fluids, and one can expect the corresponding dynamical effects to deeply alter the quasi-static force balance (4.2), and hence the flotation criterion (4.4).

4.3 How do dynamical effects alter the static balance?

The qualitative picture of these effects is as follows. The sphere velocity in the upper fluid is of $O((\unicode[STIX]{x1D701}_{p}gR)^{1/2})$ and the sphere almost comes to rest within a distance of $O(R)$ when it reaches the interface. Hence, it undergoes a negative acceleration $\text{d}V/\text{d}t=V\,\text{d}V/\text{d}z$ which is initially of $O(\unicode[STIX]{x1D701}_{p}g)$ and produces a downward inertia force $-(4/3)\unicode[STIX]{x03C0}R^{3}(\unicode[STIX]{x1D70C}_{p}+\unicode[STIX]{x1D70C}_{1}C_{M}(t))\,\text{d}V/\text{d}t$ , where $C_{M}(t)$ denotes the added-mass coefficient. In the present situation, this coefficient depends on time, increasing as the sphere gets closer to the interface (Milne-Thomson Reference Milne-Thomson1962). This inertia force provides an additional dimensionless contribution of the order of $-(\unicode[STIX]{x1D701}_{p}+1+C_{M}(t))\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ on the left-hand side of (4.2). The magnitude of this force decreases as the sphere decelerates and is reduced to a fraction of its initial value by the time the sphere velocity vanishes (which happens for $\unicode[STIX]{x1D713}\approx \unicode[STIX]{x03C0}/2$ , as figures 3(c) and (j) of PM2 show). However, a ‘history’ force, qualitatively similar to the Boussinesq–Basset force in the low-Reynolds-number regime, has taken over in the meantime. This force is due to the downthrust produced by the progressive collapse of the sphere wake. At time $t$ , it depends on the cumulated changes induced by the deceleration on the wake over previous times through a kernel $K(t-\unicode[STIX]{x1D70F})$ , with $-\infty \leqslant \unicode[STIX]{x1D70F}\leqslant t$ . This kernel is inertial by nature in the present situation where $Ar\gg 1$ , since wake disturbances are advected with the velocity $V\gg \unicode[STIX]{x1D707}_{1}/(\unicode[STIX]{x1D70C}_{1}R)$ . The closely related situation of a sudden stop of the sphere at $t=0$ was considered by Lovalenti & Brady (Reference Lovalenti and Brady1993) in the Oseen regime. They showed that the corresponding ‘history’ effect results in a force that reduces the net drag and is written at leading order as $6\unicode[STIX]{x03C0}\unicode[STIX]{x1D707}_{1}RV[(3/4)R(Vt)^{-1}]=(9/2)\unicode[STIX]{x03C0}\unicode[STIX]{x1D707}_{1}R^{2}t^{-1}$ . This prediction was extended to arbitrary Reynolds number by Lawrence & Mei (Reference Lawrence and Mei1995). Their analysis, confirmed by numerical simulations, showed that the ratio of the ‘history’ contribution to the steady drag at the corresponding Reynolds number is still $-(3/4)R(|V|t)^{-1}$ . Here, the steady drag balances the net weight of the sphere in the upper fluid, so that the contribution of the ‘history’ effect to the left-hand side of (4.2) in the case of a sudden stop would be $-(3/4)R(|V|t)^{-1}\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ , i.e. it would be of $O(\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})$ over a time period of $O(R/|V|)=O(\{R/(\unicode[STIX]{x1D701}_{p}g)\}^{1/2})$ . Although the sphere does not experience a strict sudden stop in the present experiments, the characteristic time $(R/(\unicode[STIX]{x1D701}_{p}g))^{1/2}$ is much smaller than the viscous time scale $\unicode[STIX]{x1D70C}_{1}R^{2}/\unicode[STIX]{x1D707}_{1}$ , making the sudden stop model qualitatively relevant.

To summarize, the above analysis shows that in situations where the stopping of the sphere takes place over an $O(R)$ distance, which is the case when $Ar\gg 1$ and $\unicode[STIX]{x1D706}\gg 1$ , the various inertia effects due to this stopping result in an additional negative contribution proportional to $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ on the left-hand side of (4.4). As the prefactor of this contribution is of $O(1)$ during the entire deceleration stage and even during some time after the sphere velocity has almost vanished, due to the $t^{-1}$ decay of the ‘history’ force, the actual critical density ratio below which the sphere can float is reduced by a factor of the order of 2 when $\unicode[STIX]{x1D713}=\unicode[STIX]{x03C0}/2$ compared with the prediction (4.4). Under such conditions, it is clear that the aforementioned two spheres released in the V5/water–glycerin pair cannot float. According to figure 10, the only sphere that is observed to float in this pair of fluids is the smallest polyacetal sphere (configuration 10(a) in figure 4(a)) with $Bo=0.36$ and $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}=1.57$ , a density ratio nearly four times smaller than the critical value predicted by the static criterion (4.4).

5.1 Overall force balances

Theoretical determination of the final drop volume, say ${\mathcal{V}}_{d}$ , under general conditions is a very complex free-boundary problem which is beyond the scope of this study. Nevertheless, the wide range of conditions covered in the present experiments makes it desirable to develop a qualitative understanding of the influence of the various flow parameters on ${\mathcal{V}}_{d}$ . Previous studies of this problem have focused on strictly static aspects by solving the Young–Laplace equation in order to determine the shape of the interface for a given drop volume (Shoukry, Hafez & Hartland Reference Shoukry, Hafez and Hartland1975; Smith & Van de Den Reference Smith and Van de Den1985). However, other effects come into play when the sphere moves, due to the fluid motion within and around the drop, as the transient stage described by figure 11 makes clear. Such effects were first considered theoretically by Johnson (Reference Johnson1981) in the limit of thin drops, but buoyancy effects were not included. Here, to better understand the consequences of the sphere motion on the volume of entrained drops, we use as a guide the stationary force balance on the compound ‘sphere $+$ drop’ body, and on the attached drop itself.

We establish these balances in the limit of vanishingly small inertia effects ( $Ar\ll 1$ ). The drag force acting on the compound body settling with velocity $V$ may be written in the form $F_{D}=-6\unicode[STIX]{x03C0}\unicode[STIX]{x1D707}_{2}(1+K)RV$ , where $K$ is a correction factor to Stokes’ law resulting from the prolate geometry induced by the presence of the drop (which tends to increase the drag) and influenced by the recirculating flow within it, which can be significant if $\unicode[STIX]{x1D706}$ is of $O(1)$ or larger and may even decrease the overall drag if $\unicode[STIX]{x1D706}$ is large enough (Johnson Reference Johnson1981). Taking into account the weight and buoyancy forces yields at equilibrium

(5.1) $$\begin{eqnarray}\unicode[STIX]{x1D70C}_{2}({\mathcal{V}}+{\mathcal{V}}_{d})g-(\unicode[STIX]{x1D70C}_{p}{\mathcal{V}}+\unicode[STIX]{x1D70C}_{1}{\mathcal{V}}_{d})g-6\unicode[STIX]{x03C0}\unicode[STIX]{x1D707}_{2}(1+K)RV=0.\end{eqnarray}$$

Let us now examine the force balance on the drop itself. We assume that the drop no longer interacts with the thin film that still coats the sphere in the region located ahead of the cap angle $\unicode[STIX]{x1D719}=\unicode[STIX]{x1D719}_{d}$ (with $\unicode[STIX]{x1D719}=0$ at the top of the sphere). Although there is no strict contact line there, this position corresponds to a pseudo-contact line where the tangent to the drop/outer-fluid interface makes a finite angle $\unicode[STIX]{x1D6F7}$ with the sphere surface, as figure 13 shows. Interfacial tension induces a net downward force at $\unicode[STIX]{x1D719}=\unicode[STIX]{x1D719}_{d}$ , the vertical component of which is $F_{\unicode[STIX]{x1D6FE}_{C}}=-2\unicode[STIX]{x03C0}\unicode[STIX]{x1D6FE}R\sin \unicode[STIX]{x1D719}_{d}\sin (\unicode[STIX]{x1D719}_{d}+\unicode[STIX]{x1D6F7})$ . The hydrostatic pressure component acting over the drop surface yields the usual buoyancy force $F_{B}=g(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1}){\mathcal{V}}_{d}$ . However, the drop/sphere interface also contributes to the overall balance though capillary and hydrostatic effects. Introducing the radius of curvature, $R_{0}$ , of the drop at its apex, and the distance, $h_{0}$ , from the apex to the top of the sphere (see figure 13), it is readily shown that the corresponding contribution yields a net vertical force with an upward capillary component $F_{\unicode[STIX]{x1D6FE}_{S}}=2\unicode[STIX]{x03C0}\unicode[STIX]{x1D6FE}(R^{2}/R_{0})\sin ^{2}\unicode[STIX]{x1D719}_{d}$ and a ‘downward buoyancy’ force $F_{BS}=-(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})g{\mathcal{V}}_{0}$ , where ${\mathcal{V}}_{0}=\unicode[STIX]{x03C0}R^{2}\{h_{0}\sin ^{2}\unicode[STIX]{x1D719}_{d}+R(1-3\cos ^{2}\unicode[STIX]{x1D719}_{d}+2\cos ^{3}\unicode[STIX]{x1D719}_{d})/3\}$ is the volume enclosed within the cylindrical surface with radius $R\sin \unicode[STIX]{x1D719}_{d}$ in between the sphere surface and the horizontal plane tangent to the drop apex. Thus, the net vertical component of the capillary force acting on the drop is $F_{\unicode[STIX]{x1D6FE}}=F_{\unicode[STIX]{x1D6FE}_{C}}+F_{\unicode[STIX]{x1D6FE}_{S}}=-2\unicode[STIX]{x03C0}\unicode[STIX]{x1D6FE}R\sin \unicode[STIX]{x1D719}_{d}\{\sin (\unicode[STIX]{x1D719}_{d}+\unicode[STIX]{x1D6F7})-(R/R_{0})\sin \unicode[STIX]{x1D719}_{d}\}$ , whereas the net buoyancy force is $F_{B}+F_{BS}$ . The former acts to stick the drop onto the sphere only if the contribution of the pseudo-contact line dominates, which requires $R_{0}\geqslant R\sin \unicode[STIX]{x1D719}_{d}/\!\sin (\unicode[STIX]{x1D719}_{d}+\unicode[STIX]{x1D6F7})$ . Similarly, the latter is directed upwards only if the drop volume exceeds ${\mathcal{V}}_{0}$ .

Figure 13. The geometry of the attached drop configuration in a reference frame translating with the sphere.

Within the drop, the fluid is set in motion by the stress distribution applied by the outer fluid on their common interface. The condition of zero flow rate across the drop at any angular position $\unicode[STIX]{x1D719}$ implies that this internal flow recirculates, reducing to a lubrication flow with a parabolic velocity profile in the limit of thin drops ( $h_{0}/R\ll 1$ ), and generating a traction at the drop/sphere interface that is half of that at the drop/outer-fluid interface (Johnson Reference Johnson1981). Hence, in that limit, the shear stress applied by the outer fluid results in an upward force on both interfaces, the sum of which, say $F_{Ds}$ , is proportional to the area of the spherical cap covered by the drop, i.e. to $1-\cos \unicode[STIX]{x1D719}_{d}$ . Still in the limit $h_{0}/R\ll 1$ , the pressure (more generally the normal stress) is almost constant across the drop at a given $\unicode[STIX]{x1D719}$ , thus contributing to the net viscous force only through a small form drag, say $F_{Dn}$ , proportional to the difference between the areas of the two interfaces, which itself is proportional to $(1-\cos \unicode[STIX]{x1D719}_{d})h_{0}/R$ , i.e. to the drop volume. The total drag acting on the drop, $F_{Dd}=F_{Ds}+F_{Dn}$ , may be formally written as $F_{Dd}=-6\unicode[STIX]{x03C0}\unicode[STIX]{x1D707}_{2}K_{d}RV$ , with $K_{d}$ depending on both the viscosity ratio and the drop geometry.

Collecting all contributions finally yields the vertical force balance on the drop in the form

(5.2) $$\begin{eqnarray}-2\unicode[STIX]{x03C0}R\unicode[STIX]{x1D6FE}\text{sin}\unicode[STIX]{x1D719}_{d}\left\{\sin (\unicode[STIX]{x1D719}_{d}+\unicode[STIX]{x1D6F7})-\frac{R}{R_{0}}\sin \unicode[STIX]{x1D719}_{d}\right\}+(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})({\mathcal{V}}_{d}-{\mathcal{V}}_{0})g-6\unicode[STIX]{x03C0}\unicode[STIX]{x1D707}_{2}K_{d}RV=0.\end{eqnarray}$$

Condition (5.1) governs the settling velocity of the compound body, while (5.2) may be thought of as a global constraint relating the geometrical parameters of the drop, namely $\unicode[STIX]{x1D719}_{d}$ , $\unicode[STIX]{x1D6F7}$ , $R_{0}$ , $h_{0}$ and ${\mathcal{V}}_{d}$ . Injecting the solution of (5.1) into (5.2) yields

(5.3) $$\begin{eqnarray}\frac{3}{2Bo}\sin ^{2}\unicode[STIX]{x1D719}_{d}\left(\frac{\sin (\unicode[STIX]{x1D719}_{d}+\unicode[STIX]{x1D6F7})}{\sin \unicode[STIX]{x1D719}_{d}}-\frac{R}{R_{0}}\right)+{\mathcal{V}}_{0}^{\ast }-{\mathcal{V}}_{d}^{\ast }-\left(\frac{\unicode[STIX]{x1D701}_{p}}{\unicode[STIX]{x1D701}}-1-{\mathcal{V}}_{d}^{\ast }\right)\frac{K_{d}}{1+K}=0,\end{eqnarray}$$

where ${\mathcal{V}}_{d}^{\ast }$ and ${\mathcal{V}}_{0}^{\ast }$ stand for ${\mathcal{V}}_{d}^{\ast }/{\mathcal{V}}$ and ${\mathcal{V}}_{0}/{\mathcal{V}}$ respectively. When the sphere is at rest, the last term becomes irrelevant and (5.3) is then simply an integral constraint resulting from the Young–Laplace problem that governs the classical pendant drop configuration. In contrast, when the sphere moves with respect to the fluid, the drop volume is influenced by the last term on the left-hand side, and hence by the sphere-to-fluid density contrast through the factor $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}-1=(\unicode[STIX]{x1D70C}_{p}-\unicode[STIX]{x1D70C}_{2})/(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})$ . With $K_{d}>0$ , this term tends to make the drop volume decrease compared with the static situation.

Figure 14. The volume of attached drops under various experimental conditions: (a) ${\mathcal{V}}_{d}^{\ast }$ versus $Bo$ for various spheres in $\times$ , V5/water–glycerin ( $\unicode[STIX]{x1D701}=0.32$ , $\unicode[STIX]{x1D706}=18.3$ ); ♦, V50/water–glycerin ( $\unicode[STIX]{x1D701}=0.26,\unicode[STIX]{x1D706}=1.7$ );  $\unicode[STIX]{x2B20}$ , V500/water–glycerin ( $\unicode[STIX]{x1D701}=0.24$ , $\unicode[STIX]{x1D706}=0.17$ );  $\odot$ , V5/water ( $\unicode[STIX]{x1D701}\approx 0.09,\unicode[STIX]{x1D706}=0.21$ ); ▫, V50/water ( $\unicode[STIX]{x1D701}\approx 0.04,\unicode[STIX]{x1D706}=1.9\times 10^{-2}$ ); *, V500/water ( $\unicode[STIX]{x1D701}\approx 0.03,\unicode[STIX]{x1D706}=1.9\times 10^{-3}$ ); solid and dashed lines, empirical fit for the pendant drop configuration, ${\mathcal{V}}_{d}^{\ast }=0.89(1-0.37\unicode[STIX]{x1D6F7}^{2})Bo^{-1.12}$ for $\unicode[STIX]{x1D6F7}=0$ and $\unicode[STIX]{x03C0}/6$ respectively; (b)  ${\mathcal{V}}_{d}^{\ast }$ versus $Bo_{l}$ for various spheres in ▫, V50/water; *, V500/water; solid line, guide-to-the-eye line with a $+1$ slope. Panel (b) also shows data from Pitois, Moucheront & Weill (Reference Pitois, Moucheront and Weill1999) obtained in a PDMS oil/water system ( $\unicode[STIX]{x1D701}=2.9\times 10^{-2}$ , $\unicode[STIX]{x1D706}=1.\times 10^{-4}$ ); ▿, steel spheres with various radii; ▴, glass spheres with various radii.

5.2 Experimental results and scaling laws for the drop volume

We determined the drop volumes by post-processing raw images using a contour detection technique and assuming axisymmetric shapes. To compare the corresponding results with drop volumes found with a sphere at rest, we examined the numerical predictions obtained by Shoukry et al. (Reference Shoukry, Hafez and Hartland1975) (for $\unicode[STIX]{x1D6F7}=0^{\circ }$ , up to $Bo=100$ ) and Smith & Van de Den (Reference Smith and Van de Den1985) (for all $\unicode[STIX]{x1D6F7}$ , up to $Bo=1$ ). We found that these results are accurately fitted (at least up to $\unicode[STIX]{x1D6F7}=30^{\circ }$ , the largest pseudo-contact angle observed in our experiments) by the empirical formula ${\mathcal{V}}_{d}^{\ast }=0.89(1-0.37\unicode[STIX]{x1D6F7}^{2})Bo^{-1.12}$ . It should be noted that in this formula the Bond number has a slightly larger negative exponent than suggested by the explicit $Bo^{-1}$ dependence in (5.3). This is because the drop geometry, i.e. the relation between $h_{0}$ , $R_{0}$ and $\unicode[STIX]{x1D719}_{d}$ , also varies with $Bo$ .

Figure 14(a) displays the drop volumes determined from a number of experimental tests versus the Bond number, using log–log coordinates. Also drawn in the figure are two lines corresponding to the aforementioned fit for $\unicode[STIX]{x1D6F7}=0^{\circ }$ and $30^{\circ }$ respectively. First of all, it is clear from this figure that, apart from two exceptions, all measured volumes are smaller than those found in the static configuration. This confirms that the flow at the drop surface acts against capillary effects, reducing the size of drops that can remain attached to the sphere. This figure also reveals that data obtained in the V50/water and V500/water pairs of fluids with spheres of different densities and diameters do not exhibit any clear trend, implying that the Bond number is not a relevant scaling parameter under such conditions. To understand why, one has to notice that, among the pairs of fluids considered here, these are the two with the smallest density (and viscosity) contrasts. According to (5.3), the smaller $\unicode[STIX]{x1D701}$ is, the larger the influence of the last term on the left-hand side, i.e. of the sphere motion, is on the drop volume. Hence, the corresponding problem has little in common with the static pendant drop configuration and there is no reason why volumes of drops attached to spheres with different $\unicode[STIX]{x1D701}_{p}$ values should exhibit any systematic variation with respect to $Bo$ in that limit. Then, it appears relevant to consider (5.3) in the limit $\unicode[STIX]{x1D701}\rightarrow 0$ . As terms due to buoyancy effects then become negligibly small, (5.3) may be simplified to

(5.4) $$\begin{eqnarray}\frac{3}{2Bo_{l}}\sin ^{2}\unicode[STIX]{x1D719}_{d}\left(\frac{\sin (\unicode[STIX]{x1D719}_{d}+\unicode[STIX]{x1D6F7})}{\sin \unicode[STIX]{x1D719}_{d}}-\frac{R}{R_{0}}\right)\approx \frac{K_{d}}{1+K},\end{eqnarray}$$

with $Bo_{l}=(\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}-1)Bo$ as defined earlier. Condition (5.4) suggests that drop volumes primarily depend on $Bo_{l}$ when $\unicode[STIX]{x1D701}$ is small. Figure 14(b) confirms this conjecture for the aforementioned two series which have $\unicode[STIX]{x1D701}=0.04$ and $0.03$ respectively. Although the plot contains a non-negligible scatter, drop volumes are seen to increase consistently with $Bo_{l}$ , mostly in a linear manner. The figure also displays data from Pitois et al. (Reference Pitois, Moucheront and Weill1999), who, under quite similar conditions, concluded that ${\mathcal{V}}_{d}^{\ast }$ varies approximately as the logarithm of $Bo_{l}$ .

The other three series in figure 14(a) behave more consistently, all of them exhibiting a clear decrease of ${\mathcal{V}}_{d}^{\ast }$ as $Bo$ increases. However, the average slope in each series is always less than $-0.8$ , and hence significantly smaller than the one observed in the pendant drop configuration. Although materials are not specified in the figure, the sphere density increases from right to left in the two series involving the water–glycerin mixture, i.e. those with the largest $\unicode[STIX]{x1D701}$ (since small ‘light’ spheres float, only small ‘heavy’ spheres are capable of entraining a drop). The role of this parameter may be readily appreciated in the case of the V5/water–glycerin pair, for which $\unicode[STIX]{x1D706}=18.3$ . With this large $\unicode[STIX]{x1D706}$ , the shear stress at the drop surface is almost zero, making the drag force on the drop, $F_{Dd}$ , reduce essentially to the contribution of the normal stress, $F_{Dn}$ . As we showed above, in the limit $h_{0}/R\ll 1$ , the latter is proportional to the drop volume. Hence, in (5.3), $K_{d}\propto {\mathcal{V}}_{d}^{\ast }$ , so that the term $(\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}-1)K_{d}/(1+K)$ merely acts as an additional buoyancy force, thus reducing the drop volume for a given $Bo$ (in the limit ${\mathcal{V}}_{d}^{\ast }\ll 1$ , $K\approx -1/3$ because the presence of the low-viscosity film and drop makes the compound body behave nearly as a bubble, and the drag force on a spherical bubble is $2/3$ of that on a rigid sphere). A comparison of corresponding data in figure 14(a) with the fit determined for static pendant drops reveals how dramatic the reduction of ${\mathcal{V}}_{d}^{\ast }$ due to the solid-to-fluid density contrast is: while the drop volume measured for the largest and lightest sphere ( $Bo=4.5,\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}-1\approx 0.53$ ) is close to that predicted by the fit for this specific $Bo$ , it is roughly four times less for the smallest and heaviest sphere ( $Bo=0.37,\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}-1\approx 9.1$ ). Hence, in figure 14(a), the reduction of the drop volume through the influence of the solid-to-fluid density contrast is larger on the left part of the series (small $Bo$ , large $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ ) than on the right part (large $Bo$ , small $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ ), resulting in variations with the Bond number looking weaker than those of static pendant drops. This implies that a proper representation of the ${\mathcal{V}}_{d}^{\ast }$ variations should make use of the three-dimensional space $({\mathcal{V}}_{d}^{\ast },Bo,\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701})$ , the representation in the $({\mathcal{V}}_{d}^{\ast },Bo)$ plane mixing two distinct effects. However, there is a priori no reason why the static exponent $-1.12$ should be recovered for a given $\unicode[STIX]{x1D701}_{p}/\unicode[STIX]{x1D701}$ in that three-dimensional representation because the influence of the outer flow certainly alters the drop geometry, i.e. the relation between ${\mathcal{V}}_{d}$ , $h_{0},R_{0}$ and $\unicode[STIX]{x1D719}_{d}$ , as the sequence in figure 11 suggests. Unfortunately, we have no means to evaluate this possible alteration at the present stage, as this would require significantly more data with each material in each pair of fluids.

The situation described above for fluid pairs having significant density contrasts indicates that none of the terms on the left-hand side of (5.3) are really negligible under such conditions. This state of affairs, combined with the subtle interdependence of the geometric parameters, $R_{0}$ , $h_{0}$ and $\unicode[STIX]{x1D719}_{d}$ , makes the prediction of exact scaling laws for the drop volume based on simple models for the drag factors $K_{d}$ and $K$ presumably out of reach. The situation simplifies in the limit $\unicode[STIX]{x1D701}\rightarrow 0$ , where the linear increase of ${\mathcal{V}}_{d}^{\ast }$ with $Bo_{l}$ can be readily inferred from (5.4). For this, one first has to notice that the corresponding drops have a quite flat top (see panels (d–f) in figure 12). Thus, the radius of curvature $R_{0}$ is larger than $R$ , and the positive difference $1-R/R_{0}$ increases with $h_{0}$ , and hence with ${\mathcal{V}}_{d}^{\ast }$ (one would have $1-R/R_{0}\approx h_{0}/R$ for a thin spherical drop). For small $\unicode[STIX]{x1D6F7}$ and $\unicode[STIX]{x1D719}_{d}\approx \unicode[STIX]{x03C0}/2$ (which is qualitatively consistent with the aforementioned panels), the left-hand side of (5.4) is then virtually directly proportional to ${\mathcal{V}}_{d}^{\ast }/Bo_{l}$ . In the present experiments, pairs of fluids with small $\unicode[STIX]{x1D701}$ also have small $\unicode[STIX]{x1D706}$ , so that the outer fluid almost obeys a no-slip condition at the drop surface. In the limit $h_{0}/R\ll 1$ , the drag force on the drop, $F_{Dd}$ , is then dominated by the shear-induced contribution, $F_{Ds}$ . As shown above, the latter is proportional to the area of the spherical cap covered by the drop, implying $K_{d}\propto 1-\cos \unicode[STIX]{x1D719}_{d}$ . In the same limit, $1+K\rightarrow 1$ , because the compound body behaves essentially as a solid sphere, so that the right-hand side of (5.4), $K_{d}/(1+K)$ , tends towards a non-zero value which only depends on the cap angle $\unicode[STIX]{x1D719}_{d}$ (note the difference with the case discussed above for $\unicode[STIX]{x1D706}\gg 1$ , where $K_{d}/(1+K)\propto {\mathcal{V}}_{d}^{\ast }$ ). Balancing both sides of (5.4) then requires ${\mathcal{V}}_{d}^{\ast }$ to increase linearly with $Bo_{l}$ .

Finally, it is important to keep in mind that in the present experiments, pairs of fluids with small $\unicode[STIX]{x1D701}$ also have small $\unicode[STIX]{x1D706}$ , while those with significant $\unicode[STIX]{x1D701}$ have moderate-to-large $\unicode[STIX]{x1D706}$ . Therefore, it is not clear how far the observed tendencies for ${\mathcal{V}}_{d}^{\ast }$ to decrease with increasing $Bo$ for $\unicode[STIX]{x1D701}\gtrsim 0.1$ , and to increase with increasing $Bo_{l}$ for smaller $\unicode[STIX]{x1D701}$ , are independent of $\unicode[STIX]{x1D706}$ . Additional experiments with selected fluid pairs having significant $\unicode[STIX]{x1D701}$ but small $\unicode[STIX]{x1D706}$ and vice versa are desirable to complete this picture.

6.1 Observations and underlying mechanisms

Figure 15. Fragmentation in the tail past a 14 mm steel sphere in (a) the V5/water pair (configuration $18b$ ; $\unicode[STIX]{x1D706}=0.21,\unicode[STIX]{x1D701}=0.09,\unicode[STIX]{x1D701}_{p}=7.6,Ar=970,Bo=1.3$ ), (b) the V50/water pair (configuration $27a$ ; $\unicode[STIX]{x1D706}=0.02,\unicode[STIX]{x1D701}=0.04,\unicode[STIX]{x1D701}_{p}=7.2,Ar=91,Bo=0.55$ ) and (c) the V50/water pair with Triton X-100 (configuration $27a_{s}$ ; same parameters as in (b) except that here $Bo=5.1$ ).

Figure 15 displays three sequences showing the early stages of the fragmentation process in the tail past a $14~\text{mm}$ steel sphere falling in water (these correspond to configurations 18b, 27a and 27 $a_{s}$ in § 3.2 respectively). All three cases therefore share the same value of $Ar_{l}$ , namely $Ar_{l}\approx 4.8\times 10^{3}$ . They essentially differ by the viscosity of the upper fluid, which is 10 times larger in (b) and (c) than in (a), and the presence of Triton X-100 in (c), which makes the Bond number $Bo_{l}$ rise up to $Bo_{l}=1.01\times 10^{3}$ , whereas $Bo_{l}=115$ and $111$ in (a) and (b) respectively. Videos displaying the corresponding three sequences are available online in the supplementary material. No fragmentation takes place in the wake of the same steel sphere with the V500/water pair of fluids (configuration 27b), although $Ar_{l}$ is unchanged and the Bond number is similar to those of cases (a) and (b) ( $Bo_{l}=123$ ). This is a clear indication that, for a given kinetic energy of the sphere and given levels of capillary and viscous stresses exerted by the outer fluid, fragmentation can only occur if the viscosity of the inner fluid is low enough to allow large deformation of fluid parcels. The development of the breakup process in cases (a) and (b), as well as the absence of breakup in the aforementioned case, can be appreciated in more detail in the videos available as supplementary material https://doi.org/10.1017/jfm.2017.747.

In all three sequences of figure 15, longitudinal ligaments, which are usual precursors of fragmentation in shear flows (Villermaux Reference Villermaux2007), develop at the back of the sphere, invading the entire tail progressively. However, these ligaments start to form immediately after the sphere crosses the interface in the case of the least viscous oil, whereas the tail remains axisymmetric and exhibits prominent corollas during a significant period of time in (b) and (c). The reason for this difference must be sought in the upper fluid: as the corresponding $Ar$ is of $O(10^{3})$ with the least viscous oil, the flow in the wake becomes unstable well before the sphere reaches the interface, quickly generating three-dimensional vortex structures and small but non-zero horizontal displacements and rotations of the sphere. This may be appreciated in the top part of the sequence (a), where the funnel-shaped entrained column just below the interface is seen to be significantly non-vertical. In contrast, in (b) and (c), $Ar$ is of $O(10^{2})$ , so that the flow past the sphere is still almost axisymmetric when it reaches the interface (see § 3.3). Wake instability, and hence three-dimensionality, then takes a finite time to develop while the sphere settles in the lower fluid, and it is only after this time lapse that ligaments start to form.

In the case of liquid jets sheared by a gas stream, the Rayleigh–Taylor instability has been shown to be key in ligament formation (Marmottant & Villermaux Reference Marmottant and Villermaux2004). Here, the capillary length $l_{c}$ in (b) is approximately $9.3~\text{mm}$ , i.e. $1.3R$ , due to the weak density contrast between the two fluids. As the radius of the entrained column is of $O(R)$ , the largest wavelength that can form in the azimuthal direction corresponds to a wavenumber $k_{m}\approx R^{-1}$ . Thus, $k_{m}\approx 1.3l_{c}^{-1}$ , which is beyond the cutoff wavenumber $k_{co}=l_{c}^{-1}$ of the Rayleigh–Taylor instability (Chandrasekar Reference Chandrasekar1961). Thus, this mechanism cannot be responsible for the formation of the ligaments in that case. Due to the presence of Triton X-100, $l_{c}$ is decreased to ${\approx}\!3~\text{mm}$ in (c), i.e. $k_{m}l_{c}\approx 0.45$ , which makes the largest structures in the tail potentially unstable through the Rayleigh–Taylor mechanism. Nevertheless, most of the three-dimensional structures seen to develop in (c) are of much smaller size, again making this mechanism unable to explain their formation. Wake instability, which eventually results in a turbulent wake, is thus responsible for the generation of three-dimensional structures, and hence of longitudinal ligaments, in all three sequences of figure 15.

Figure 16. The evolution of the fragmented wake of a 14 mm steel sphere in the V5/water pair (a) and the V50/water pair (b).

Visual inspection of these three sequences also makes it clear that the average size of the ligaments and large droplets that form in the wake is smaller in (a) than in (b). This may be even better appreciated from figure 16, which provides a closer view of the wake evolution for these two cases. In figure 16(b), one notices the fairly cylindrical shape of the ligaments, at the tip of which droplets are seen to form; the ligaments clearly exhibit more wrinkled shapes in figure 16(a). We confirmed the difference in the average size of the large droplets formed during wake fragmentation by processing images taken at later time in the three sequences (with the sphere standing approximately $25R$ below the initial interface). More precisely, we identified a group of 40–80 nearly circular drops in each series of images using a contour detection routine, and employed Hough transform to determine the circle that best fitted each of them. We repeated the analysis with three separate runs in each series of experiments, to reduce statistical uncertainty. Unfortunately, the detection technique could not properly identify fragments smaller than 3–5 pixels, limiting our analysis to droplets larger than $0.30\pm 0.08$  mm in diameter with the present optical device. This restriction and the too limited available samples of drops prevented us from determining other statistical properties of the size distribution, especially its probability density function. For the population of ‘large’ droplets we considered, the above procedure yielded mean radii of $0.625$ , $0.84$ and $0.78~\text{mm}$ in (a), (b) and (c) respectively, with a 1 pixel uncertainty amounting to $\pm 0.04~\text{mm}$ . Since the pairs of fluids in series (a) and (b) have almost identical interfacial tensions, the noticeable difference in the corresponding two mean radii underlines the strong influence of the viscosity of the upper fluid (i.e. the one that forms the tail) on the outcome of the fragmentation process. In contrast, the fairly small difference in the mean radii found for series (b) and (c), which have identical viscosities but interfacial tensions differing by one order of magnitude, is quite remarkable.

6.2 A phenomenological statistical model

The influence of surface tension and viscosity on the statistical characteristics of drop and bubble distributions resulting from turbulent breakup is well known, and considerable effort has been invested to quantify it in various types of flows, especially in the context of chemical engineering applications (see, e.g., Coulaloglou & Tavlarides (Reference Coulaloglou and Tavlarides1977), Tsouris & Tavlarides (Reference Tsouris and Tavlarides1994), Luo & Svendsen (Reference Luo and Svendsen1996) and references therein). However, the present situation, in which the wake is in a transitional state, makes it useful to revisit the underlying stress balances in the more general context of inertia-dominated carrying flows in which the efficient spatial scales comparable with the droplet size do not necessarily obey the usual scaling laws of high-Reynolds-number turbulence.

If $\unicode[STIX]{x1D706}$ is large, making the effects of the internal viscosity negligible, breakup is expected to occur as soon as the pressure difference $\unicode[STIX]{x0394}p(\mathfrak{r})$ along the surface of a ligament with radius $\mathfrak{r}$ exceeds the restoring capillary pressure, which is of $O(\unicode[STIX]{x1D6FE}/\mathfrak{r})$ . Assuming that the motion in the outer fluid is dominated by inertia, one has $\unicode[STIX]{x0394}p(\mathfrak{r})\propto \unicode[STIX]{x1D70C}_{2}(\unicode[STIX]{x0394}u(\mathfrak{r}))^{2}\approx \unicode[STIX]{x1D70C}_{2}(\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})\mathfrak{r})^{2}$ , where $\unicode[STIX]{x0394}u(\mathfrak{r})$ denotes the norm of the velocity variation over the distance $\mathfrak{r}$ and $\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})$ is the norm of the corresponding velocity gradient, both quantities being defined as ensemble averages if the flow is turbulent. In that case, assuming that the flow satisfies the various hypotheses on which Kolmogorov’s K41 theory is grounded (Frisch Reference Frisch1995), the root-mean-square value of the velocity gradient at scale $\mathfrak{r}$ within the inertial subrange depends only on $\mathfrak{r}$ and on the averaged dissipation rate per unit volume, $\unicode[STIX]{x1D716}_{K}$ , so that $\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})\propto (\unicode[STIX]{x1D716}_{K}/\mathfrak{r}^{2})^{1/3}$ . Inserting this estimate into $\unicode[STIX]{x0394}p(\mathfrak{r})$ and equating to $\unicode[STIX]{x1D6FE}/\mathfrak{r}$ yields the classical equilibrium mean drop radius, which may be thought of as the statistical average of the maximum stable drop size, as (Kolmogorov Reference Kolmogorov1949; Hinze Reference Hinze1955)

(6.1) $$\begin{eqnarray}\mathfrak{r}=\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}\propto (\unicode[STIX]{x1D6FE}/\unicode[STIX]{x1D70C}_{2})^{3/5}\unicode[STIX]{x1D716}_{K}^{-2/5},\end{eqnarray}$$

which implicitly assumes that $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ stands within the inertial subrange. Although still dominated by inertia, the flow in the outer fluid may be laminar or at least governed by non-local characteristics with a well-defined spatial scale, in which case $\unicode[STIX]{x1D6E4}_{u}$ becomes independent of $\mathfrak{r}$ for distances smaller than the smallest of these scales. Hence, $\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})=\unicode[STIX]{x1D6E4}_{0}$ , where $\unicode[STIX]{x1D6E4}_{0}$ is governed by outer scales. Setting $\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})=\unicode[STIX]{x1D6E4}_{0}$ in $\unicode[STIX]{x0394}p(\mathfrak{r})$ now yields

(6.2) $$\begin{eqnarray}\mathfrak{r}=\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}\propto (\unicode[STIX]{x1D6FE}/\unicode[STIX]{x1D70C}_{2})^{1/3}\unicode[STIX]{x1D6E4}_{0}^{-2/3}.\end{eqnarray}$$

Both scalings indicate that the equilibrium drop radius increases with $\unicode[STIX]{x1D6FE}$ , but the two exponents differ, the influence of surface tension being almost twice as large in the fully turbulent case.

The above classical reasoning assumes that capillarity is the only cohesive force present in the system. However, as already recognized by Hinze (Reference Hinze1955), a viscous drop offers more resistance to breakup than a bubble with a vanishingly small viscosity because the dissipation associated with internal straining motions consumes a part of the energy supplied by the outer flow. The remark at the beginning of this section about the absence of fragmentation in the wake of the largest steel sphere falling in the V500/water pair of fluids provides a clear illustration of this influence. The fraction of the available energy transmitted by fluid 2 to a ligament of fluid 1 results in internal velocity differences over a distance $\mathfrak{r}$ that are proportional to $(1+\unicode[STIX]{x1D701})^{1/2}\unicode[STIX]{x1D6E5}u(\mathfrak{r})$ (due to kinetic energy conservation), thus inducing viscous stresses of $O((1+\unicode[STIX]{x1D701})^{1/2}\unicode[STIX]{x1D707}_{1}\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r}))$ . If $\unicode[STIX]{x1D707}_{1}$ is very large or $\unicode[STIX]{x1D6FE}$ is very small, this fraction is close to unity, so that the capillary force does not play any further role and the equilibrium mean drop radius is obtained by equating the viscous stress to $\unicode[STIX]{x1D70C}_{2}(\unicode[STIX]{x0394}u(\mathfrak{r}))^{2}$ , which yields either

(6.3) $$\begin{eqnarray}\mathfrak{r}=\mathfrak{r}_{0\unicode[STIX]{x1D707}}\propto (1+\unicode[STIX]{x1D701})^{-3/8}(\unicode[STIX]{x1D707}_{1}/\unicode[STIX]{x1D70C}_{1})^{3/4}\unicode[STIX]{x1D716}_{K}^{-1/4},\end{eqnarray}$$

if the carrying flow is fully turbulent, or

(6.4) $$\begin{eqnarray}\mathfrak{r}=\mathfrak{r}_{0\unicode[STIX]{x1D707}}\propto (1+\unicode[STIX]{x1D701})^{-1/4}(\unicode[STIX]{x1D707}_{1}/\unicode[STIX]{x1D70C}_{1})^{1/2}\unicode[STIX]{x1D6E4}_{0}^{-1/2},\end{eqnarray}$$

if it is ‘laminar’. Again, the dependence upon fluid properties is stronger in the turbulent case.

Applying (6.1) (respectively (6.2)) to series (b) and (c), in which viscosities are identical, predicts that the equilibrium mean radius should be $3.75$ (respectively $2.1$ ) larger in case (b), whereas experiments indicate a bare $1.08$ ratio. Similarly, predictions (6.3) (respectively (6.4)) applied to series (a) and (b), which have almost the same surface tension, yield an equilibrium mean radius $5.85$ (respectively $3.25$ ) larger in the latter case, which is in stark contrast to the observed $1.35$ ratio. Clearly, none of the above predictions which only consider effects of surface tension or viscosity capture the actual stress balance controlling the fragmentation process properly. In other words, the actual equilibrium drop radius results from both capillary and internal viscous stresses, and their interaction mitigates the influence of each of the two physical properties taken separately.

In the general case where both types of effects act together, the total cohesive stress involved in the system may be formally written in the form $(\unicode[STIX]{x1D6FE}/\mathfrak{r}){\mathcal{H}}({\mathcal{G}})$ , where ${\mathcal{H}}$ is an unknown function and ${\mathcal{G}}(\mathfrak{r})\propto (1+\unicode[STIX]{x1D701})^{1/2}(\unicode[STIX]{x1D707}_{1}/\unicode[STIX]{x1D6FE})\mathfrak{r}\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})$ is the ratio of the viscous and capillary stresses. Since the initial estimate yielded $\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r}_{0\unicode[STIX]{x1D6FE}})\approx (\unicode[STIX]{x1D6FE}/(\unicode[STIX]{x1D70C}_{2}\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}^{3}))^{1/2}$ , one also has ${\mathcal{G}}(\mathfrak{r})\approx (1+\unicode[STIX]{x1D701})^{1/2}\unicode[STIX]{x1D707}_{1}(\unicode[STIX]{x1D70C}_{2}\unicode[STIX]{x1D6FE}\mathfrak{r})^{-1/2}\mathfrak{r}^{3/2}\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})/(\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}^{3/2}\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}))=Oh_{1}(\mathfrak{r})(\mathfrak{r}/\mathfrak{r}_{0\unicode[STIX]{x1D6FE}})^{3/2}$ $\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r})/\unicode[STIX]{x1D6E4}_{u}(\mathfrak{r}_{0\unicode[STIX]{x1D6FE}})$ , where $Oh_{1}(\mathfrak{r})=\unicode[STIX]{x1D707}_{1}/(\unicode[STIX]{x1D70C}_{1}\unicode[STIX]{x1D6FE}\mathfrak{r})^{1/2}$ is the Ohnesorge number at scale $\mathfrak{r}$ in fluid 1. In the simplest case, the equilibrium mean radius, $\mathfrak{r}_{0a}$ , depends on four physical properties ( $\unicode[STIX]{x1D70C}_{1},\unicode[STIX]{x1D70C}_{2},\unicode[STIX]{x1D707}_{1},\unicode[STIX]{x1D6FE}$ ), one length scale ( $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ ) and one strain rate ( $\unicode[STIX]{x1D6E4}_{0}$ or $(\unicode[STIX]{x1D716}_{k}\mathfrak{r}_{0a}^{-2})^{1/3}$ ). Hence, the problem involves four dimensionless parameters, three of which are $\mathfrak{r}_{0a}/\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ , $\unicode[STIX]{x1D70C}_{2}/\unicode[STIX]{x1D70C}_{1}=1+\unicode[STIX]{x1D701}$ and $Oh_{1}(\mathfrak{r}_{0a})$ . The last one is, for instance, the characteristic drop Reynolds number, $Re_{1}(\mathfrak{r}_{0a})=\unicode[STIX]{x1D70C}_{1}\unicode[STIX]{x1D716}_{K}^{1/3}\mathfrak{r}_{0a}^{4/3}/\unicode[STIX]{x1D707}_{1}$ or $Re_{1}(\mathfrak{r}_{0a})=\unicode[STIX]{x1D70C}_{1}\unicode[STIX]{x1D6E4}_{0}\mathfrak{r}_{0a}^{2}/\unicode[STIX]{x1D707}_{1}$ , according to the nature of the outer flow. The unknown function ${\mathcal{H}}$ therefore depends on these four parameters, and this dependence must be such that ${\mathcal{H}}\rightarrow 1$ when $Oh_{1}\rightarrow 0$ and must also guarantee that the scalings (6.3) and (6.4) are recovered in the limit $Oh_{1}\rightarrow \infty$ . Seeking ${\mathcal{H}}$ in the form of products of these parameters and applying the above constraints provides the ratio of the equilibrium mean radius $\mathfrak{r}_{0a}$ for an arbitrary triplet $(Oh_{1},Re_{1},\unicode[STIX]{x1D701})$ to its value $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ in the pure capillary limit $Oh_{1}\rightarrow 0$ as

(6.5) $$\begin{eqnarray}\left(\frac{\mathfrak{r}_{0a}}{\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}}\right)^{5/3}=1+A_{T}(1+\unicode[STIX]{x1D701})^{3/16}Re_{1}^{3/8}(\mathfrak{r}_{0a})Oh_{1}(\mathfrak{r}_{0a})\left(\frac{\mathfrak{r}_{0a}}{\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}}\right)^{5/6},\end{eqnarray}$$

in the fully turbulent case, or

(6.6) $$\begin{eqnarray}\left(\frac{\mathfrak{r}_{0a}}{\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}}\right)^{3}=1+A_{L}(1+\unicode[STIX]{x1D701})^{1/8}Re_{1}^{1/4}(\mathfrak{r}_{0a})Oh_{1}(\mathfrak{r}_{0a})\left(\frac{\mathfrak{r}_{0a}}{\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}}\right)^{3/2},\end{eqnarray}$$

under ‘laminar’ conditions, $A_{T}$ and $A_{L}$ being two unknown constants. The overall validity of an equivalent of (6.5) has been confirmed by Calabrese, Chang & Dang (Reference Calabrese, Chang and Dang1986) and Wang & Calabrese (Reference Wang and Calabrese1986), who performed extensive experiments with numerous liquid–liquid systems, varying $\unicode[STIX]{x1D706}$ and $\unicode[STIX]{x1D6FE}$ over three and nearly two orders of magnitude respectively.

The mean radii found under conditions (a), (b) and (c) defined above yield $Oh_{1}(\mathfrak{r}_{0a})\approx 0.037$ , $0.34$ and $1.05$ respectively. In the turbulent case, $Re_{1}(\mathfrak{r})$ may be evaluated assuming that $\unicode[STIX]{x1D716}_{K}\propto V^{3}/R$ $\propto ((\unicode[STIX]{x1D701}_{p}-\unicode[STIX]{x1D701})/(1+\unicode[STIX]{x1D701})g)^{3/2}R^{1/2}$ , which yields $Re_{1}(\mathfrak{r})=((\unicode[STIX]{x1D701}_{p}-\unicode[STIX]{x1D701})/(\unicode[STIX]{x1D701}_{p}(1+\unicode[STIX]{x1D701})))^{1/2}Ar(\mathfrak{r}/R)^{4/3}$ . In the ‘laminar’ situation, one first has to identify the relevant outer scales that determine $\unicode[STIX]{x1D6E4}_{0}$ . The sphere settling velocity $V$ and the thickness $\unicode[STIX]{x1D6FF}_{2}$ of the boundary layer that develops in the outer fluid along the tail seem to be natural candidates, yielding $\unicode[STIX]{x1D6E4}_{0}=S=V/\unicode[STIX]{x1D6FF}_{2}$ , where $S$ stands for the average shear rate in the boundary layer. Nevertheless, since the tail/outer-fluid interface is close to vertical, baroclinic effects are also likely to take place. Over the same distance $\unicode[STIX]{x1D6FF}_{2}$ (which is the smallest length scale involved in the system), the ratio of baroclinic to shear effects may be expressed through the Richardson number $Ri=N^{2}/S^{2}$ , with $N^{2}=2(\unicode[STIX]{x1D70C}_{2}-\unicode[STIX]{x1D70C}_{1})/(\unicode[STIX]{x1D70C}_{2}+\unicode[STIX]{x1D70C}_{1})(g/\unicode[STIX]{x1D6FF}_{2})$ , and one might alternatively choose $\unicode[STIX]{x1D6E4}_{0}=N$ . The boundary layer thickness $\unicode[STIX]{x1D6FF}_{2}(t)$ evolves as $\unicode[STIX]{x1D6FF}_{2}(t)=(\unicode[STIX]{x1D707}_{2}t/\unicode[STIX]{x1D70C}_{2})^{1/2}\propto (\unicode[STIX]{x1D707}_{2}L(t)/(\unicode[STIX]{x1D70C}_{2}V))^{1/2}$ , i.e. $(RL(t)/Ar_{l})^{1/2}$ , where $L(t)$ is the depth (measured from the initial interface) reached by the sphere at the time $t$ when $\mathfrak{r}_{0a}$ is determined. Use of the above estimate for $\unicode[STIX]{x1D6FF}_{2}$ reveals that the ratio $N/S$ does not exceed a few per cent ( $3\,\%$ in the case of the V5/water pair, which has the largest density contrast among the fluid systems in which fragmentation was observed). This confirms $S$ as the relevant choice to characterize the strain rate applied by the outer fluid to the tail, whereas baroclinic effects only play a minor role. Hence, with $\unicode[STIX]{x1D6E4}_{0}=S=((\unicode[STIX]{x1D701}_{p}-\unicode[STIX]{x1D701})/(1+\unicode[STIX]{x1D701})gR)^{1/2}/\unicode[STIX]{x1D6FF}_{2}(t)$ , one finds $Re_{1}(\mathfrak{r})=((\unicode[STIX]{x1D701}_{p}-\unicode[STIX]{x1D701})/(\unicode[STIX]{x1D701}_{p}(1+\unicode[STIX]{x1D701}))Ar_{l}(R/L))^{1/2}Ar(\mathfrak{r}/R)^{2}$ . Each of the two definitions of $Re_{1}$ involves an arbitrary prefactor, but the latter does not vary among the three experimental sequences, so that it can be lumped into the corresponding constant $A_{T}$ or $A_{L}$ .

With $\mathfrak{r}_{0a}$ , $Oh_{1}(\mathfrak{r}_{0a})$ and $Re_{1}(\mathfrak{r}_{0a})$ at hand in each case, (6.5) and (6.6) may be used to estimate $A_{T}$ and $A_{L}$ and possibly determine which scaling is the most appropriate. To this end, we employed the following procedure. First, noting that $Oh_{1}$ is small in case (a), the corresponding $\mathfrak{r}_{0a}$ is close to $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ and may be used as an initial guess to determine the latter. Starting with this guess, we computed a first estimate of $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ for case (c) (in which $\unicode[STIX]{x1D6FE}$ is one order of magnitude smaller), using either (6.1) or (6.2), and extracted a first estimate of $A_{T}$ and $A_{L}$ by solving (6.5) and (6.6), still for case (c), which has the largest $Oh_{1}$ , and hence the largest sensitivity to viscous effects. Then, we used these values of $A_{T}$ and $A_{L}$ in (6.5) and (6.6) to obtain a first correction to $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ for case (a). We repeated this process until convergence, obtaining $A_{T}\approx 2.07$ (respectively $A_{L}\approx 2.18$ ) and $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}=0.52$  mm (respectively $0.57~\text{mm}$ ) in case (a), and hence $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}=0.14$  mm (respectively $0.275~\text{mm}$ ) in case (c). Since the interfacial tension is virtually the same in fluid pairs (a) and (b), so is $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ , and we could then make use of the previously determined $\mathfrak{r}_{0\unicode[STIX]{x1D6FE}}$ to assess which value of $A_{T}$ (respectively $A_{L}$ ) is necessary for (6.5) (respectively (6.6)) to predict the experimentally observed value of $\mathfrak{r}_{eq}$ in case (b). This procedure yielded $A_{T}\approx 1.30$ and $A_{L}\approx 1.80$ . Hence, the two values of $A_{L}$ obtained from cases (a) and (c) are close to one another ( $20\,\%$ difference), whereas those of $A_{T}$ clearly differ by nearly $60\,\%$ . This suggests that, in the flow under consideration, the appropriate scaling almost corresponds to ‘laminar’ conditions, the larger ‘fully turbulent’ exponents associated with the physical properties $\unicode[STIX]{x1D6FE}$ and $\unicode[STIX]{x1D707}_{1}$ in (6.1) and (6.3) overestimating the sensitivity of $\mathfrak{r}_{0a}$ to these properties. This conclusion supports the view that the shear resulting from the boundary layer that develops around the tail is the main source of fragmentation under the present conditions. This does not mean that turbulence is absent from the wake, but rather that its intensity is not large enough in the relevant range of scales for the resulting strain rates to compete with the strong shear provided by the boundary layer. This situation is reminiscent of the findings of Eastwood, Armi & Lasheras (Reference Eastwood, Armi and Lasheras2004), who performed experiments in a turbulent water jet with known characteristics, in which small amounts of various liquids were released on the centreline through a needle. They observed that droplets resulting from the breakup process did not follow the Hinze–Kolmogorov scaling (6.1), whereas bubbles produced in the same device using the same protocol did, as previously noticed by Martinez-Bazán, Montañes & Lasheras (Reference Martinez-Bazán, Montañes and Lasheras1999). They attributed this remarkable difference to the fact that the small-scale nearly isotropic turbulent motions present in the jet are sufficiently energetic to break air filaments, whereas liquid filaments with much larger densities and viscosities can only break under the action of large-scale organized vortices.

Assuming an ‘averaged’ value $A_{L}=2.0$ , (6.6) predicts $\mathfrak{r}_{0a}=0.625$ , $0.89$ and $0.75$  mm for cases (a), (b) and (c) respectively. Given the $0.08$  mm uncertainty on the optical determination of $\mathfrak{r}_{0a}$ and the significant statistical uncertainty due to the limited sample of drops we considered, these predictions may be considered to compare well with the experimental values of $\mathfrak{r}_{0a}=0.625$ , $0.84$ and $0.78$ mm in all three cases. Hence, (6.5) with $A_{L}=2.0$ appears to be a simple but potentially useful model for predicting the average size of drops resulting from fragmentation in ‘quasi-laminar’ liquid–liquid systems involving significant viscous effects in the dispersed phase, at least down to $\unicode[STIX]{x1D706}=O(10^{-2})$ . More experiments covering a wider range of physical properties and flow conditions, supplemented by extensive data processing, are of course necessary to confirm this provisional conclusion.