Hostname: page-component-745bb68f8f-g4j75 Total loading time: 0 Render date: 2025-02-11T22:29:27.791Z Has data issue: false hasContentIssue false
  • English
  • Français

Coherent structures in the inner part of a rough-wall channel flow resolved using holographic PIV

Published online by Cambridge University Press:  19 September 2012

Siddharth Talapatra  and
Joseph Katz
Siddharth Talapatra
Affiliation:
The Johns Hopkins University, Baltimore, MD 21218, USA
Joseph Katz*
Affiliation:
The Johns Hopkins University, Baltimore, MD 21218, USA
*
†Email address for correspondence: katz@jhu.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

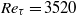Microscopic holographic PIV performed in an optically index-matched facility resolves the three-dimensional flow in the inner part of a turbulent channel flow over a rough wall at Reynolds number . The roughness consists of uniformly distributed pyramids with normalized height of 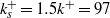. Distributions of mean flow and Reynolds stresses agree with two-dimensional PIV data except very close to the wall (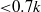) owing to the higher resolution of holography. Instantaneous realizations reveal that the roughness sublayer is flooded by low-lying spanwise and groove-parallel vortical structures, as well as quasi-streamwise vortices, some quite powerful, that rise at sharp angles. Conditional sampling and linear stochastic estimation (LSE) reveal that the prevalent flow phenomenon in the roughness sublayer consists of interacting U-shaped vortices, conjectured in Hong et al. (J. Fluid Mech., 2012, doi:10.1017/jfm.2012.403). Their low-lying base with primarily spanwise vorticity is located above the pyramid ridgeline, and their inclined quasi-streamwise legs extend between ridgelines. These structures form as spanwise vorticity rolls up in a low-speed region above the pyramid’s forward face, and is stretched axially by the higher-speed flow between ridgelines. Ejection induced by interactions among legs of vortices generated by neighbouring pyramids appears to be the mechanism that lifts the quasi-streamwise vortex legs and aligns them preferentially at angles of 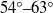to the streamwise direction.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 711 , 25 November 2012 , pp. 161 - 170
Copyright
Copyright © Cambridge University Press 2012

References

1. Adrian, R. J. 2007 Hairpin vortex organization in wall turbulence. Phys. Fluids 19, 041301.CrossRefGoogle Scholar
2. Adrian, R. J. & Moin, P. 1988 Stochastic estimation of organized turbulent structure: homogeneous shear flow. J. Fluid Mech. 190, 531–559.CrossRefGoogle Scholar
3. Antonia, R. A. & Luxton, R. E. 1971 The response of a turbulent boundary layer to a step change in surface roughness. Part 1. Smooth to rough. J. Fluid Mech. 48, 721–761.CrossRefGoogle Scholar
4. Finnigan, J. J., Shaw, R. H. & Patton, E. G. 2009 Turbulence structure above vegetation canopies. J. Fluid Mech. 637, 387–424.CrossRefGoogle Scholar
5. Hong, J., Katz, J., Meneveau, C. & Schultz, M. P. 2012 Coherent structures and associated subgrid-scale energy transfer in a rough-wall turbulent channel flow. J. Fluid Mech., doi:10.1017/jfm.2012.403.CrossRefGoogle Scholar
6. Hong, J., Katz, J. & Schultz, M. P. 2011 Near-wall turbulence statistics and flow structures over 3D roughness in a turbulent channel flow. J. Fluid Mech. 667, 1–37.CrossRefGoogle Scholar
7. Ikeda, T. & Durbin, P. A. 2007 Direct simulations of a rough-wall channel flow. J. Fluid Mech. 571, 235–263.CrossRefGoogle Scholar
8. Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
9. Jimenez, J. 2004 Turbulent flows over rough walls. Annu. Rev. Fluid Mech. 36, 173–196.CrossRefGoogle Scholar
10. Jimenez, J. & Pinelli, A. 1999 The autonomous cycle of near-wall turbulence. J. Fluid Mech. 389, 335–359.CrossRefGoogle Scholar
11. Ligrani, P. M. & Moffat, R. J. 1986 Structure of transitionally rough and fully rough turbulent boundary layers. J. Fluid Mech. 162, 69–98.CrossRefGoogle Scholar
12. Monty, J. P., Allen, J. J., Lien, K. & Chong, M. S. 2011 Modification of the large-scale features of high Reynolds number wall turbulence by passive surface obtrusions. Exp. Fluids 51, 1755–1763.CrossRefGoogle Scholar
13. Raupach, M. R., Antonia, T. A. & Rajagopalan, S. 1991 Rough wall boundary layers. Appl. Mech. Rev. 44, 1–25.CrossRefGoogle Scholar
14. Schoppa, W. & Hussain, F. 2002 Coherent structure generation in near-wall turbulence. J. Fluid Mech. 453, 57–108.CrossRefGoogle Scholar
15. Schultz, M. P. & Flack, K. A. 2009 Turbulent boundary layers on a systematically varied rough wall. Phys. Fluids 21, 015104.CrossRefGoogle Scholar
16. Sheng, J., Malkiel, E. & Katz, J. 2006 Digital holographic microscope for measuring 3D particle distribution and motions. Appl. Opt. 45, 3893–3901.CrossRefGoogle ScholarPubMed
17. Sheng, J., Malkiel, E. & Katz, J. 2008 Using digital holographic microscopy for simultaneous measurements of 3D near wall velocity and wall shear stress in a turbulent boundary layer. Exp. Fluids 45, 1023–1035.CrossRefGoogle Scholar
18. Tachie, M. F., Bergstrom, D. J. & Balachandar, R. 2003 Roughness effects in low- open channel turbulent boundary layers. Exp. Fluids 35, 338–346.CrossRefGoogle Scholar
19. Talapatra, S. & Katz, J. 2012 Scope of microscopic inline digital holography for resolving the 3D flow in the roughness sublayer. Meas. Sci. Technol. (in press).Google Scholar
20. Yang, Q. & Wang, M. 2009 Computational study of roughness induced boundary-layer noise. AIAA J. 47, 2417–2429.CrossRefGoogle Scholar