3.1 The UGT-like model with AIDS: basic assumptions

Besides endogenous fertility, the building block of the model is represented by the assumption of endogenous child and adult survival. Survival probabilities are taken as increasing functions of human capital to reproduce, in the absence of HIV, a regular DT pattern triggered by the interplay of increasing survival, education, and fertility, aiming to reflect the pre-AIDS setting in SSA countries where the DT was ongoing prior to HIV onset. In the presence of HIV, these survival probabilities are assumed to scale with infection prevalence to mirror the disruption of human capital caused by AIDS-related mortality on the assumption that all HIV-infected individuals die of AIDS. Unlike previous studies on development and the DT, which identified the ultimate trigger of the fertility transition in the decline in child mortality [Kalemli-Ozcan (Reference Kalemli-Ozcan2002), Doepke (Reference Doepke2005), Fioroni (Reference Fioroni2010)], our model also predicts a key role played by adult survival. Consequently, we eventually predict the possibility of an HIV-induced fertility reversal due to the upsurge in adult mortality caused by AIDS. AIDS mortality reduces people's need to make provision for the future, ultimately eroding resources for education and further human capital growth [Kalemli-Ozcan (Reference Kalemli-Ozcan2012)].

We consider an OLG-closed economy accounting for fertility, mortality, education, and human capital accumulation along the UGT narrative. The economy is populated by a continuum of rational and identical individuals (mothers) of size N _t per generation. Time is discrete and indexed by t = 0, 1, 2, … and the length of each period is conventionally set at 20 years. The individual's lifetime is divided among three periods: childhood/adolescence, young adulthood (or adulthood, which represents the working period) and old age. Newborns of generation t may either die early (that is, before parents can spend time on their education), with probability 1 − Γ_t+1, or they may survive, with probability Γ_t+1 ∈ [0, 1], thus receiving education (e _t+1) according to parents' decisions. As adolescents, they do not make economic decisions and do not work, but can become sexually active and be exposed to HIV infection. If they survive at the onset of adulthood (time t + 1), they become economically active. In particular, Γ_t+1 is assumed to depend positively on the level of human capital available at time t + 1 (h _t+1) and negatively on the proportion i _t+1 of HIV-infective parents (representing HIV prevalence among adults, which we simply term HIV prevalence), i.e., Γ_t+1 = Γ(h _t+1, i _t+1). As adults, they maximize their expected lifetime utility, they may acquire HIV infection, and have a probability Π_t+1 = Π(h _t+1, i _t+1) of surviving up to the onset of old age. As for child survival, adult survival positively depends on the existing endowment of human capital and negatively on HIV prevalence. The assumption that child and adult mortality depend endogenously on human capital (through education) follows Blackburn and Cipriani's (Reference Blackburn and Cipriani2002) argument that a higher education increases individual awareness of healthier behaviors and lifestyles. The endowment of labor is supplied to firms in exchange for wage income w _t+1 per unit of effective labor. The (expected) lifetime utility function captures the individual preferences toward material consumption (c _t+1) and the number (quantity) of surviving children (n _t+1) during young adulthood, as well as the total human capital of the surviving children during old age. The following relationship holds:

(1)$$n_{t + 1} = \Gamma _{t + 1}n_t^g, $$

relating the number of born children of generation t ($n_t^g$) with the resulting number of surviving children, who become economically active at time t + 1 20 years later. The size of the young adult population living at time t + 1 is $N_{t + 1} = n_tN_t = n_{t-1}^g \Gamma _tN_t$.

3.2 Disease transmission

We represent HIV spread by following Chakraborty et al. (Reference Chakraborty, Papageorgiou and Pérez Sebastián2010, Reference Chakraborty, Papageorgiou and Pérez Sebastián2016), who first proposed a Diamond-like OLG growth model incorporating the temporal diffusion of long-lasting fatal infectious diseases by explicitly representing the transmission process rather than considering a mere mortality shock. This allowed for both intra-generational transmission between young adults and inter-generational transmission between young adults and adolescents (Figure 2).

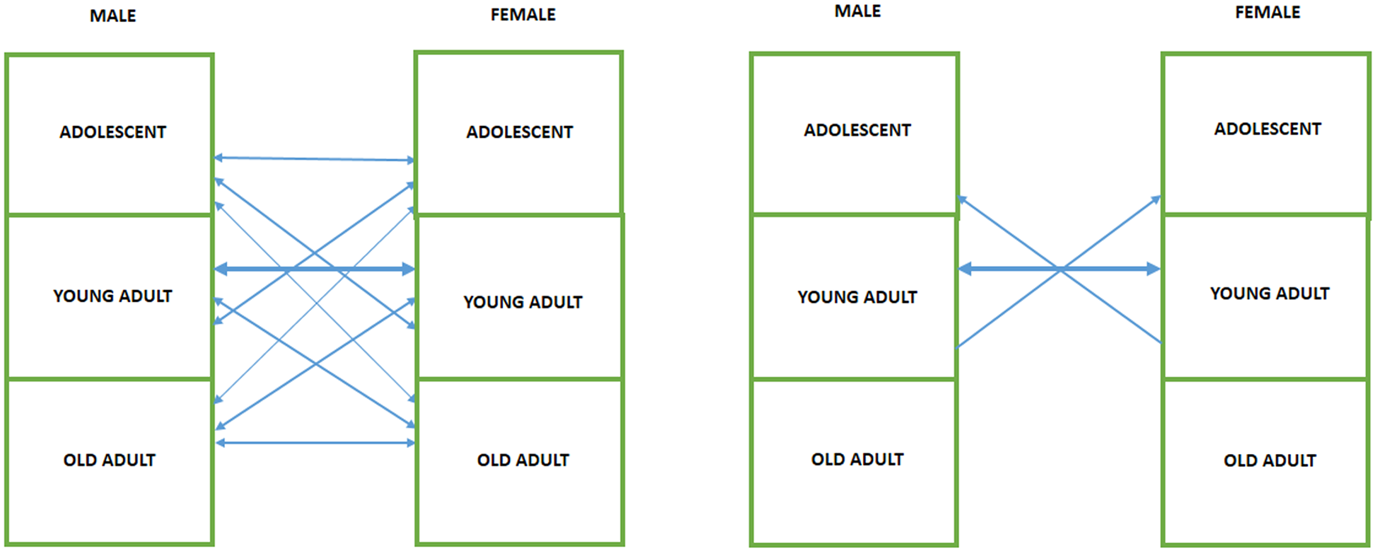
Figure 2. Mechanism of infection diffusion. Left panel: realistic patterns of HIV heterosexual transmission. Right panel: the stylized mechanism of HIV diffusion considered in Chakraborty et al. (Reference Chakraborty, Papageorgiou and Pérez Sebastián2010, Reference Chakraborty, Papageorgiou and Pérez Sebastián2016) and used in our model.

Let p _t+1 be the probability that an HIV-susceptible young adult acquires HIV infection. Following Chakraborty et al. (Reference Chakraborty, Papageorgiou and Pérez Sebastián2016), this can be represented as a function of HIV prevalence among individuals of generation t + 1, that is

(2)$$p_{t + 1} = 1-(1-\lambda \,i_{t + 1})^\mu, $$

where 0 < λ ≤ 1 is the constant probability of being infected per sexual partnership with an infected individual and μ > 0 represents the average number of sexual partnerships of a young adult individual during his/her entire adulthood. If the population is large, the prevalence rate at time t + 2 among young adults converges to the probability of an adult being HIV-infected, i.e., i _t+2 = p _t+1. Therefore, ultimately

(3)$$i_{t + 1} = 1-(1-\lambda \,i_t)^\mu. $$

Equation (3) describes the natural history of HIV in the absence of any control measures.

3.3 The model: individual choices

By normalizing the utility from death to zero, preferences of the representative mother born at time t, becoming economically active at time t + 1, are captured by the following inter-temporal (expected) utility function:

(4)$$U_{t + 1} = \ln (c_{t + 1}) + \rho \ln (n_{t + 1}) + z\Pi _{t + 1}\ln (n_{t + 1}h_{t + 2}),$$

where ρ > 0 captures the relative taste for children and z > 0 is a scaling parameter tuning the relative degree of altruism. This allows the mother to receive utility (with certainty) from material consumption and the quantity of children during young adulthood, and from the total human capital embedded in all surviving children (n _t+1h _t+2) during old age. The quality of children is represented by their own level of human capital through education. As in Blackburn and Cipriani (Reference Blackburn and Cipriani2002) and Chakraborty (Reference Chakraborty2004), weighting the flow of utility when old with the adult survival probability Π_t+1 captures the decision maker's responses to changing mortality and fertility conditions, thereby triggering—in the absence of HIV/AIDS—the child quantity/quality switch and ultimately the DT through the changes in child and adult mortality.Footnote ³ As we will show below, HIV/AIDS—whose dramatic mortality mainly frightens young adults (rather than children)—has the potential to break the quantity/quality switch in SSA, by preventing parents from enjoying any utility flow from educated children and altering the fertility transition.

A t-generation individual choice is made at time t + 1 subject to the budget constraint:

(5)$$c_{t + 1} = w_{t + 1}\left( {m-\displaystyle{{\psi \,n_{t + 1}} \over {\Gamma_{t + 1}}}-\phi_{t + 1}n_{t + 1}-e_{t + 1}n_{t + 1}} \right),$$

where m is the time endowment. Equation (5) implies that material consumption is constrained by the amount of resources available after accounting for the portions of time for giving birth to $n_{t + 1}^g$ children (ψ), and raising (ϕ _t+1) and educating (e _t+1) those who survive (n _t+1).

The human capital of each child (h _t+2) depends on the human capital already available (h _t+1) and their time expenditure in education according to:

(6)$$h_{t + 2} = q(x + e_{t + 1})h_{t + 1}^\alpha, $$

where q > 0 and 0 < α ≤ 1. As x + e _t+1 > 0, it follows that h _t+1 ∈ (0, + ∞) for any t ≥ 0 as h ₁ >0 must hold. The term x > 0 guarantees that children's human capital is positive even if parents do not invest in education.

3.4 The model: main characteristics of individual choices and related literature

For clarity, before moving onto the analysis and results, we discuss the previous modeling choices and hypotheses in relation to the existing literature.

4.1 Solution of the individual problem

Maximization of the utility function (4) with respect to consumption, the number of children and the time spent in education, subject to the budget constraint (5), rule (6) for human capital accumulation, and the condition c _t+1 > 0, gives the solution to the individual optimization program. This solution can be either interior (e _t+1 > 0) or on the corner (e _t+1 = 0). The interior solution is:

(7)$$e_{t + 1} = \displaystyle{{z\Pi _{t + 1}} \over {\rho \, \Gamma _{t + 1}}}[\Gamma _{t + 1}(\phi _{t + 1}-x) + \psi ]-x,$$

(8)$$n_{t + 1} = \displaystyle{{m\rho \, \Gamma _{t + 1}} \over {[\Gamma _{t + 1}(\phi _{t + 1}-x) + \psi ](1 + \rho + z\Pi _{t + 1})}},$$

(9)$$c_{t + 1} = \displaystyle{{m \, w_{t + 1}} \over {1 + \rho + z\Pi _{t + 1}}},$$

provided that (1) $\Pi _{t + 1} \gt \overline \Pi _{t + 1}$, where

$$\overline \Pi _{t + 1} = \overline \Pi (h_{t + 1},i_{t + 1}): = \displaystyle{{\rho x \, \Gamma _{t + 1}} \over {z[\Gamma _{t + 1}(\phi _{t + 1}-x) + \psi ]}},$$

is the threshold value of the adult survival probability [from equation (7)] ensuring that education is strictly positive at the interior equilibrium, and (2) Γ_t+1(ϕ _t+1 − x) + ψ > 0 must hold to guarantee that the precautionary demand for children is strictly positive at the interior equilibrium. If $\Pi _{t + 1} \le \overline \Pi _{t + 1}$, the solution of the individual optimization program is on the corner and it is given by:

(10)$$e_{t + 1} = 0,$$

(11)$$n_{t + 1} = \displaystyle{{m \, \Gamma _{t + 1}(\rho + z\Pi _{t + 1})} \over {(\phi _{t + 1}\Gamma _{t + 1} + \psi )(1 + \rho + z\Pi _{t + 1})}},$$

(12)$$c_{t + 1} = \displaystyle{{m \, w_{t + 1}} \over {1 + \rho + z\Pi _{t + 1}}}.$$

The interior solution (7)–(9) and the corner solution (10)–(12) are in line with those obtained by Galor and Weil (Reference Galor and Weil2000), de la Croix and Doepke (Reference de la Croix and Doepke2003, Reference de la Croix and Doepke2004), Zhang and Zhang (Reference Zhang and Zhang2005), Fioroni (Reference Fioroni2010), Yakita (Reference Yakita2010) and Constant (Reference Constant2019).Footnote ⁶ The individual optimal behaviour can be interpreted (see Yakita, Reference Yakita2010) by comparing marginal benefits and marginal costs of education (ceteris paribus) versus those of giving birth to an extra child. The marginal benefit of additional education is represented by the marginal utility of child quality, that is $\displaystyle{{\partial U_{t + 1}} \over {\partial h_{t + 2}}}\displaystyle{{\partial h_{t + 2}} \over {\partial e_{t + 1}}} = \displaystyle{{z\Pi _{t + 1}} \over {x + e_{t + 1}}}$, and the corresponding marginal opportunity cost is $\displaystyle{{\partial (w_{t + 1}e_{t + 1}n_{t + 1})} \over {\partial e_{t + 1}}} = w_{t + 1}n_{t + 1}$. The marginal benefit of giving birth to an extra child is represented by the marginal utility of child quantity (fertility), that is $\displaystyle{{\partial U_{t + 1}} \over {\partial n_{t + 1}}} = \displaystyle{{\rho + z\Pi _{t + 1}} \over {n_{t + 1}}}$, and the corresponding marginal opportunity cost is given by the partial derivative of the lifetime budget constraint (5) with respect to n _t+1, which is given by $w_{t + 1}\left( {\displaystyle{\psi \over {\Gamma_{t + 1}}} + \phi_{t + 1} + e_{t + 1}} \right)$. Therefore, comparison of marginal benefits and costs of child quality and child quantity at the optimum means that parents will be indifferent if $\displaystyle{{z\Pi _{t + 1}} \over {x + e_{t + 1}}} = w_{t + 1}n_{t + 1}$ and $\displaystyle{{\rho + z\Pi _{t + 1}} \over {n_{t + 1}}} = w_{t + 1}\left( {\displaystyle{\psi \over {\Gamma_{t + 1}}} + \phi_{t + 1} + e_{t + 1}} \right)$ hold, respectively. Now, indifference between educating or not educating a child involves comparison between the previous two equalities at e _t+1 = 0. The indifference condition holds when $\Pi _{t + 1}-\overline \Pi _{t + 1} = 0$. If $\Pi _{t + 1}-\overline \Pi _{t + 1} \gt 0$, the parent's net returns in terms of enjoying a higher future utility from well-educated children during her old age is positive and she prefers to invest in child quality rather than having more children. If $\Pi _{t + 1}-\overline \Pi _{t + 1} \lt 0$, the parent's net returns in terms of enjoying a higher future utility from well-educated children during her old age is negative and she prefers to invest in child quantity by changing the precautionary demand for children at e _t+1 = 0.

Regardless of HIV, this result highlights the existence of an endogenous mechanism allowing the switch from a low development regime, where both fertility and mortality (of children and adults) are “high” and the rate of human capital accumulation is low, to a high development regime, where education is positive, the rate of human capital accumulation is higher and hence both fertility and mortality (of children and adults) are set on a steadily declining path of DT.

To sum up, our analysis shows the existence of a critical threshold value of adult survival probability ($\overline \Pi _{t + 1}$) below which individual behaviors entrap the economy in its corner solution, where a positive relationship exists between fertility and adult survival. In this regime, individuals have no incentive to start investing in child quality and any external shocks in the standard of living temporarily increasing adult survival will simply be reflected in higher fertility, therefore implying a Malthusian relationship [this result resembles Tabata (Reference Tabata2003) and Fioroni (Reference Fioroni2010), who found a positive relationship between fertility and human capital in the low development regime]. This is because in the case of no education an increase in human capital raises adult survival prospects, allowing parents to live longer and expand the amount of resources for childrearing. Indeed, the related opportunity cost is lower than the opportunity cost of additional education, such that providing education to their children is still expensive relative to parents' income. On the other hand, above $\overline \Pi _{t + 1}$ individual behaviors let the economy lie on its interior development trajectory where a negative (resp. positive) relationship appears between fertility (resp. education) and adult survival. In this regime, an individual has an incentive to invest in child quality and increasingly reduces the precautionary demand of children as she becomes richer. The reason for the switch from quantity to quality of children depends on the higher probability of receiving a utility inflow in old age due to increasing adult survival. The main effect for the next period output is a higher level of human capital due to a larger investment in education. Besides the standard dependence of quality and quantity of children on child survival, which is the cornerstone of the UGT narrative allowing the main stylized facts of the DT to be captured [Doepke (Reference Doepke2005), Fioroni (Reference Fioroni2010)], we stress here the existing unambiguous relationship between education and adult survival and between fertility and adult survival.

Once HIV is introduced into the model, adult survival obviously also reflects HIV prevalence. The resulting feedback of AIDS-related mortality of adults on both the quality and quantity of children on demo-economic transition in SSA is the main feature of our modified UGT model and will be discussed later. Following Cervellati and Sunde (Reference Cervellati and Sunde2005), the current work represents a theoretical attempt to emphasize the role of adult survival (of the decision maker or parent) as a key factor for fertility transition. Results are also in line with Soares (Reference Soares2005), who emphasized the role of (child and adult) mortality in affecting individual incentives to have children (fertility) and being one of the determinants of the process of long-term growth and development.

4.2 Production

The production of final output (Y _t) occurs under perfect competition according to the technology $Y_{t + 1} = H_{t + 1} + \theta L_{t + 1}{\kern 1pt} $ that can also be written as $Y_{t + 1} = (h_{t + 1} + \theta ){\kern 1pt} L_{t + 1}$, where H _t+1 = h _t+1L _t+1 is the aggregate stock of human capital (or total human capital of adult individuals), where $L_{t + 1} = \left( {m-\displaystyle{{\psi \,n_{t + 1}} \over {\Gamma_{t + 1}}}-\phi_{t + 1}n_{t + 1}-e_{t + 1}n_{t + 1}} \right)N_{t + 1}$ is the labour input and θ ≥ 0 is a parameter capturing workers’ productivity. By normalising the price of output to one, profit maximisation means that labour is paid according to its marginal product, that is w _t+1 = h _t+1 + θ. Unlike Chakraborty et al. (Reference Chakraborty, Papageorgiou and Pérez Sebastián2010, Reference Chakraborty, Papageorgiou and Pérez Sebastián2016), who considered a model with physical capital, we deliberately ruled out any negative feedback of HIV on labor productivity not only because HIV-infected individuals are asymptomatic and in good health until they develop full-blown AIDS (as stated above), but especially because (in our model) the wage only affects material consumption and it has no effects on education, fertility, or the dynamics of the system [as in de la Croix and Doepke (Reference de la Croix and Doepke2003)]. As regard the assumption of perfect substitutability of the inputs, we departed from the formulation of Chakraborty et al. (Reference Chakraborty, Papageorgiou and Pérez Sebastián2010) that adds to the Cobb–Douglas production function (including physical capital and labor) a component representing natural endowment (land and animals), mimicking low-resource settings such as SSA. The technology used here represents a parsimonious version that slightly modifies their function by replacing the Cobb–Douglas term by a component only dependent on human capital. This is because we wanted to stress the primary effect of HIV/AIDS, namely the disruption of human capital through additional mortality, and the possibility of (a reversal of) the quantity/quality switch induced by the epidemic. Including total human capital in the production function is in line with Cervellati and Sunde (Reference Cervellati and Sunde2005, p. 1655): “…human capital is a central factor of production, and at the same time helps to improve the longevity and productivity of future generations.” Clearly, we could use a more standard technology e.g., Cobb–Douglas [as in Galor and Weil (Reference Galor and Weil2000)], that is $Y_{t + 1} = AH_{t + 1}^\alpha X_{t + 1}^{1-\alpha}$, where X _t+1 = θL _t+1 (for sufficiently large θ, consumption will be above one, and the utility will never be negative), but this would not affect our main findings. This is because the dynamics of the economy result only from human capital accumulation and HIV spread, and neither of these variables depend on the wage rate, which only affects material consumption [see equations (7)–(9) and (10)–(12)]. Therefore, using a production function including either perfect or imperfect substitutability between factor inputs has no influence. We also recall that a purely human-capital-dependent technology is central in the Boserupian mechanism, where skills acquired from learning-by-doing increase human capital and represent the key factor for agricultural economies to escape from the Malthusian trap in the demographic tradition [Boserup (Reference Boserup1981)].Footnote ⁷

4.3 General equilibrium

By using the solution of the individual problem [equations (7)–(9) or (10)–(12)] together with equation (6) describing human capital accumulation, we obtain the following two-dimensional map describing the evolution of human capital and HIV prevalence over two subsequent periods, which completely characterizes the dynamics of the economy:

(13)$$\eqalign{h_{t + 2} = & \left\{ \matrix{qxh_{t + 1}^\alpha \quad {\rm if}\;\Pi_{t + 1} \le \overline \Pi_{t + 1} \hfill \cr \displaystyle{{qz\Pi_{t + 1}[(\phi_{t + 1}-x)\Gamma_{t + 1} + \psi ]} \over {\rho \, \Gamma_{t + 1}}}h_{t + 1}^\alpha \quad {\rm if}\;\Pi_{t + 1} \gt \overline \Pi_{t + 1} \hfill} \right. \cr i_{t + 2} = & 1-(1-\lambda i_{t + 1})^\mu.} $$

Remark 1 In the absence of HIV (i _t = 0), the model becomes:

(14)$$h_{t + 2} = \left\{ \matrix{qxh_{t + 1}^\alpha \quad {\rm if}\;\Pi_{t + 1} \le \overline {\overline \Pi}_{t + 1} \hfill \cr \displaystyle{{qz\Pi_{t + 1}[(\phi_{t + 1}-x)\Gamma_{t + 1} + \psi ]} \over {\rho \, \Gamma_{t + 1}}}h_{t + 1}^\alpha \quad {\rm if}\;\Pi_{t + 1} \gt \overline{\overline \Pi}_{t + 1} \hfill} \right.,$$

where $\overline{\overline \Pi} _{t + 1} = \overline{\overline \Pi} (h_{t + 1},0)$, predicting convergence toward either a low development regime or a high development regime depending on the relative size of adult survival, which in turn depends only on human capital (education) in that case.

Importantly, the UGT literature, whose main goal is to explain the fertility transition, has pinpointed different channels based on different thresholds. Such thresholds have been identified in terms of technological progress [Galor and Weil (Reference Galor and Weil2000)], in terms of individual heterogeneity [de la Croix and Doepke (Reference de la Croix and Doepke2003)], in child survival [Kalemli-Ozcan (Reference Kalemli-Ozcan2002), Fioroni (Reference Fioroni2010)] or in the individual's ability to acquire human capital [Cervellati and Sunde (Reference Cervellati and Sunde2005)].

The natural history of HIV in the absence of any control measures [equation (3)] is summarized in the following proposition (of which we omit the easy proof).

Remark 2 The threshold parameter $\mu \, \lambda$ in Proposition 1 has a convenient epidemiological interpretation: sinceμ represents the number of different sexual partners of the representative agent during his/her sexually active life and λ represents the transmission probability per single sexual partnership, the product $\mu \, \lambda$ represents the expected number of new infections caused by a single infective agent during her entire sexually active life provided all her partners are susceptible to infection. The ensuing interpretation is that the infection can become endemic only if it is “reproductive,” i.e., each infective agent causes more than one secondary lifetime infection. Consequently, according to epidemiological and demographic jargon, the product $\mu \, \lambda$ represents the basic reproduction number of the pathogen.

Given that the HIV equation is independent of other variables, the dynamics of HIV follows Proposition 1. Therefore, in the below-threshold case $\mu \, \lambda \lt 1$, as HIV eventually goes extinct (i _t → 0), the medium-long-term dynamics of the economy is essentially the one described by (14), where i _t = 0. Here, by medium-long-term we mean a period of time such that the infection has reached its steady-state equilibrium (be this the extinction or the endemic steady state), but all the remaining demographic and economic variables—which are affected by the dynamics of HIV—are still in their transient phase. By contrast, in the above-threshold case $\mu \, \lambda \gt 1$ HIV will eventually approach its long-term steady-state equilibrium i*. This in turn means that all relevant demo-economic variables depending on HIV prevalence will obey a medium-long-term pathway depending only on i*. In particular, when HIV has achieved its endemic steady-state equilibrium i*, the medium-long-term dynamics of the survival functions Π_t+1 = Π(h _t+1, i _t+1) and Γ_t+1 = Γ(h _t+1, i _t+1) in the absence of any control measures are: Π_t+1 = Π(h _t+1, i*) and Γ_t+1 = Γ(h _t+1, i*). Consequently, the medium-long-term form of human capital accumulation as described in (13) is the following:

(15)$$h_{t + 2} = \left\{ \matrix{qxh_{t + 1}^\alpha \quad {\rm if}\;\Pi_{t + 1} \le \overline \Pi (h_{t + 1},i^{^\ast}) \hfill \cr \displaystyle{{qz\Pi_{t + 1}[(\phi_{t + 1}-x)\Gamma_{t + 1} + \psi ]} \over {\rho \, \Gamma_{t + 1}}}h_{t + 1}^\alpha \quad {\rm if}\;\Pi_{t + 1} \gt \overline \Pi (h_{t + 1},i^{^\ast}) \hfill} \right..$$

5.1 Model parameterization

5.1.1 Functional forms for child and adult survival

Consistent with the hypotheses of section 3.1, we modeled the child survival probability Γ_t+1 as follows:

(16)$$\Gamma _{t + 1} = \Gamma _{t + 1}^{NA} (1-\delta \, i_{t + 1}),$$

where $\Gamma _{t + 1}^{NA}$ represents the child survival probability in the absence of HIV and 0 < δ ≤ 1 is the conditional probability that a child of HIV-infected parents is vertically transmitted at birth, such that $\delta \, i_{t + 1}$ is the probability of a child being born infected (and dying soon after). The adopted functional form for $\Gamma _{t + 1}^{NA}$ is as follows:

(17)$$\Gamma _{t + 1}^{NA} : = \Gamma _{{\rm pre}} + (\Gamma _{{\rm post}}-\Gamma _{{\rm pre}})\displaystyle{{b\ln (1 + h_{t + 1})} \over {1 + b\ln (1 + h_{t + 1})}},$$

where Γ_pre, Γ_post ∈ [0, 1] are, respectively, child survival at birth in the Malthusian regime prevailing before mortality decline and child survival at birth in the modern regime at the end of the mortality transition [Galor (Reference Galor, Aghion and Durlauf2005, Reference Galor2011)], and b > 0 is a parameter tuning the shape of the mortality transition along the growth of human capital. The functional form (17) depicts a pattern of increasing child survival during the mortality transition (in the absence of HIV), departing from its Malthusian level Γ_pre and eventually approaching its modern level Γ_post by the end of the mortality transition.

Similarly, the probability Π_t+1 is modeled as follows:

(18)$$\Pi _{t + 1} = \Pi _{t + 1}^{NA} (1-i_{t + 1}),$$

where $\Pi _{t + 1}^{NA}$ represents the adult survival probability in the absence of HIV, which is assumed to take the following form:

(19)$$\Pi _{t + 1}^{NA} : = \Pi _{{\rm pre}} + (\Pi _{{\rm post}}-\Pi _{{\rm pre}})\displaystyle{{B\ln (1 + h_{t + 1})} \over {1 + B\ln (1 + h_{t + 1})}},$$

where Π_pre, Π_post ∈ [0, 1] and B > 0 have the corresponding meaning of Γ_pre, Γ_post, and b in equation (16).

Finally, the cost of raising children is represented by an increasing function of human capital accumulation as in (17) and (18), that is:

(20)$$\phi _{t + 1} = \phi _{{\rm pre}} + (\phi _{{\rm post}}-\phi _{{\rm pre}})\displaystyle{{\ln (1 + h_{t + 1})} \over {1 + \ln (1 + h_{t + 1})}}.$$

5.1.2 Intervention programs against HIV by external donors

Intervention programs against HIV are modeled by an exogenous reduction in the transmission probability per partnership λ (it would be equivalent to intervening on the number of sexual partners μ). As discussed in the Introduction, this is motivated by the fact that the vast majority [up to 90% of AIDS funding in low-income countries in 2013, UNAIDS (2017)] are paid for through external financial donations. The chosen approach can capture a range of different medical treatments, e.g., anti-retroviral, with the potential to reduce individual infectivity, as well as non-medical interventions, e.g., aiming to increase awareness of HIV risk and favor spontaneous behavior changes, such as reducing the number of sexual partners and increasing the use of condoms. We believe that this is a good compromise between realism and the simplicity of the theoretical framework adopted. Indeed, a more realistic description of the complex set of interventions against HIV (to prevent new infections) and against AIDS (to treat the sick) would require a much more detailed modeling set-up. The intervention is introduced at time t = T and the evolution of λ is modeled in the simplest possible way, that is:

(21)$$\lambda _t: = \left\{ {\matrix{ \lambda \hfill & {{\rm if}\;t \lt T} \hfill \cr {\lambda_c} \hfill & {{\rm if}\;t \ge T} \hfill \cr}} \right.,$$

where λ represents the HIV transmission probability in the absence of any interventions and λ _c < λ represents the corresponding probability after a control program initiated at time t = T. In what follows, we will focus on a fully successful control $\mu \, \lambda _c \lt 1$, which will bring the HIV epidemic on an extinction path according to the first part of Proposition 1.

5.1.3 Parameter assignment

Parameterization was carried out to broadly match the main quantitative traits of the DT and HIV spread in SSA. To this end, we attempted to pursue the following three sub-goals: namely (A) coarsely reproducing the scale of fertility and mortality transition in SSA; (B) matching HIV prevalence data; and (C) keeping in a reasonably correct empirical balance the relative time scales of the epidemic and of the fertility transition.

Demographic parameters were assigned from UN data for SSA [UN (2019)] in order to (i) reproduce the range of TFR decline during the fertility transition in SSA, departing from a pre-transitional level of about 6 and ending at about 2 (i.e., the replacement level) in line with UN projections medium variant [UN (2015)], and (ii) the pre- and post-transitional levels of the survival probabilities Π_pre, Π_post and Γ_pre, Γ_post in (17) and (19).

Parameters (μ,λ) of the HIV equation (2) were assigned to bound at equilibrium (in the absence of any intervention) the range of prevalence levels observed in the adult population in medium-high prevalence SSA countries, according to UNAIDS last estimates [UNAIDS (2017)]. This was motivated by the fact that in most cases such estimates showed a constant trend for several years before 2015 (Figure 1a). We considered the following idealized scenarios in terms of the HIV equilibrium prevalence (i*): “low”: i* = 0.1, “medium”: i* = 0.15, “high”: i* = 0.2, “very high”: i* = 0.3.

Summary information about model parameters is reported in Table 1. Parameter values were either borrowed from the literature or assigned for simulation purposes.

Table 1. Summary information about assignment of model parameters

t denotes time in OLG units i.e., it represents the length of an OLG time (set to 20 years in the model). Values of Π_pre, Π_post and Γ_pre, Γ_post were assigned to match the corresponding observed probabilities to survive from age 20 to 40 (for a person alive at age 20), and from birth to age 20, respectively.

5.2 Simulation scenarios: the benchmark case of an uncontrolled HIV epidemic

In the absence of HIV, our model predicts the onset of fertility transition—with the escape from the poverty trap—and its gradual completion as an endogenous response of education investments to the increase in survival of both children and young adults. This is represented by the S-shaped TFR decline from about 6 to a replacement level slightly in excess of 2 (the black curve represented on the left axis in Figure 3, both panels). Though in section 3 we focused on female fertility, meant as the average number of daughters of a representative mother during her adult phase in the absence of mortality, here we prefer to refer to total fertility and focus more intuitively on the trend of the TFR.

Figure 3. The predicted trajectories of SSA fertility in the absence of HIV (left axis) and of HIV prevalence (right axis) under uncontrolled HIV epidemics of different intensity. Left panel: the onset of HIV occurs at an advanced stage of the fertility transition. Right panel: the onset of HIV occurs at an earlier stage of the fertility transition.

We initialize the HIV epidemic for $\mu \, \lambda \gt 1$ along section 5.1.3 by assuming that the economy already lies on its development trajectory with a strictly positive level of education.Footnote ⁸ The onset and growth of HIV reduces adult survival (by way of AIDS mortality) and therefore impacts negatively on education and human capital, and ultimately brings fertility up along the direct effect shown in equations (7) and (8), as well as along the general equilibrium feedback effects due to the overall dynamics of the economy. We also point out that, in principle, nothing prevents HIV bringing a growing economy back to its corner solution, that is on its pre-transitional underdevelopment path. However, as this seems to require higher levels of HIV prevalence than those observed so far in SSA, we will rule out this case from subsequent simulations.

The HIV epidemic in the absence of control takes off and gradually approaches its equilibrium prevalence i*, where it persists thereafter (the four colored curves depicted on the right axes in Figure 3, both panels). The two panels of Figure 3 can be mutually contrasted to mirror the empirically documented variability in the stage of the fertility transition of SSA countries at the onset of large HIV epidemics. In this regard, the left panel depicts a situation where the epidemic began at an advanced stage of the fertility transition (TFR about 3.5), such as for South Africa, while the right panel depicts a situation where the epidemic began at an early stage of the transition (TFR still in the range 5–6), such as for Kenya.Footnote ⁹

The impact of a persistent uncontrolled HIV epidemic on the fertility transition in SSA would be dramatic (Figure 4), with fertility reaching levels well above the replacement threshold. For high endemic levels of HIV, the TFR eventually stalls on levels higher than three children per woman. Moreover, medium-high HIV epidemics have the potential to cause a sharp reversal in the fertility transition pathway. Two main qualitative scenarios emerge depending on whether the onset of HIV occurred either at an advanced or at an earlier stage of the fertility transition as in Figure 3. In the former case, at the onset of HIV the TFR has already experienced most of its decline. The reversal occurs through a marked increase in TFR which essentially remains constant thereafter. Instead, in the latter case the TFR shows a temporary relapse, after which it resets on a declining path. This is because the fertility decline triggered by mortality progress from causes of deaths other than HIV had already initiated, so that there remains considerable room for further reductions in fertility attributable to such causes.

Figure 4. Potential impact of uncontrolled HIV epidemics on the fertility transition in SSA for different levels of HIV endemic equilibrium prevalence (i*). Left panel: the HIV onset occurs at an advanced stage of the transition. Right panel: the HIV onset occurs at an earlier stage of the transition.

Importantly, the HIV-induced fertility relapse is not a full reversal in the clock of history with fertility returning to its pre-transitional level. The range of the fertility relapse is indeed strictly bounded by the relapse in the mortality of adults, which is only one of the two components of the fertility transition, the other being the decline in child mortality, which is only slightly affected (at the aggregate level) by HIV.

The causation chain (illustrated in Figure 5 for the scenario of HIV onset at an advanced stage of the fertility transition, as in Figures 3 and 4, left panel) goes as follows: (a) AIDS mortality breaks-down the long-term declining trend in adult mortality, which suddenly upturns (Figure 5, left panel); (b) the upturn in adult mortality reverses the positive trend on education investments (Figure 5, central panel); and (c) the collapse in education investment sets the growth rate of human capital at lower levels, which are insufficient to further fuel the quantity–quality switch, and therefore to further promote fertility decline (Figure 5, right panel). Note that at its long-term equilibrium in the absence of intervention the model, which had been calibrated on data from Lesotho, mirrors the observed drop in the survival probability Π_t+1 from more than 90% in the pre-HIV period to less than 65% in proximity of the most dramatic phase of the epidemic in 2005 (Figure 6). Note also that the observed trajectory in Figure 6 shows a gradual relapse after 2010 due to the onset of interventions against HIV, while the predicted curve in the left panel of Figure 5 just stabilizes because no intervention is assumed.

Figure 5. Fertility reversal in SSA under an uncontrolled HIV epidemic for different levels of HIV endemic equilibrium prevalence. Temporal trends of: adult survival probability Π_t+1 (left panel), education e _t+1 (center panel), and growth rate of human capital h _t+1 (right panel).

Figure 6. Observed trend of the survival probability Π_t+1 that a (female) individual who just entered young adulthood survives until entering old age in Zimbabwe and Lesotho, 1950–2015. The function Π_t+1 was estimated by the conditional survival probability p ₄₀/p ₂₀, where p _x represents the survival function up to exact age x [source: UN (2019)].

5.3 Simulation scenarios: the effects of interventions

The analysis in the previous section described a useful theoretical benchmark against which to ground the predicted effects of the current realistic scenarios of HIV control [WHO (2016), UNAIDS (2017)] on fertility. We now report the implications of exogenous interventions noted in section 5.1. For the sake of simplicity, we only consider interventions able to eventually eliminate HIV, and compare the effects of different timings, contrasting an early intervention, initiating shortly before the epidemic has reached its equilibrium prevalence, with a few delayed interventions (Figure 7). We hypothesize that the time span needed to bring HIV to elimination from the intervention onset is roughly comparable with the time span that the epidemic took to reach its peak (Figure 7, left panel), which in SSA lasted approximately two-three decades [UNAIDS (2017)]. The fact that it is hopeless to eliminate HIV more rapidly is motivated, on the one hand, by the large proportions of current seropositive individuals still unaware of their own health status that might remain infective for decades in the absence of large-scale screening, and, on the other, by the fact that effective anti-retroviral therapies will extend the duration of the sero-positive period for treated individuals [WHO (2016)].

Figure 7. HIV onset at an advanced stage of the fertility transition. Temporal trend of HIV prevalence (left panel) and total fertility (right panel) under different effective intervention programs able to eventually achieve HIV elimination. The intervention programs differ in the timing of initiation (t = 80, 100, 120).

For brevity, we report predictions of controlled HIV scenarios (Figure 7) just focusing on the case of late HIV onset (Figures 3 and 4, left panel). Bringing HIV under full control (Figure 7, left panel) will gradually remove obstacles to investments in education, such that the TFR will eventually settle on its replacement level (Figure 7, right panel). Nonetheless, even under the early intervention scenario, the time span needed to bring HIV to elimination, has the potential to delay the completion of fertility transition. Obviously, this scenario could substantially worsen in the presence of delays in the effectiveness of interventions (right panel).