INTRODUCTION
Phonological development, and in particular the acquisition of consonants, is a complex process which is determined by, inter alia, physiological and motoric aspects of speech articulation, perception-related issues, the child's developing grammar and lexicon, and ambient language factors (Rose, Reference Rose, Pellegrino, Marsico, Chitoran and Coupé2009). For instance, consonants that are relatively easy to articulate (e.g. stops) will probably be acquired earlier than consonants that are more difficult to articulate (e.g. laterals and trills), especially because young children's articulatory apparatus is physiologically still different from adults' and because they have more limited motor control abilities than adults (Kent & Miolo, Reference Kent, Miolo, Fletcher and MacWhinney1995). In addition, children seem to pick up perceptually salient parts of the input first (Ferguson & Garnica, Reference Ferguson, Garnica, Lenneberg and Lenneberg1975). Furthermore, an approach based on representational complexity in the child's grammar predicts that the phonologically simplest or least marked structures are acquired before more complex or more marked structures (Rose, Reference Rose, Pellegrino, Marsico, Chitoran and Coupé2009). Lastly, the child's language environment may affect consonant acquisition in the sense that the consonants that are most frequent or distinguish most minimal pairs in the child's input also tend to be acquired first (Ingram, Reference Ingram1989). The present article zooms in on one particular factor in this complex system, namely the child's language input.
In what respect does the ambient language determine the order in which children acquire segments? Intuitively speaking, it could be argued that the more a child hears a particular segment, the sooner that segment will be acquired. In other words, input frequency (henceforth: IF), the relative frequency of a particular segment in the ambient language, determines its acquisition order (e.g. Stokes & Wong, Reference Stokes and Wong2002; Tsurutani, Reference Tsurutani2007). Alternatively, it could be argued that the more a segment is used in the ambient language to differentiate one word from another, the sooner it will be acquired. This notion of relative use, which can be traced back to Martinet (Reference Martinet1955), is often referred to as the functional load (henceforth: FL) of a particular language element, such as a segment or a segmental contrast. FL refers to the extent to which a language makes use of that element (Pye, Ingram & List, Reference Pye, Ingram, List, Nelson and van Kleek1987; Stokes & Surendran, Reference Stokes and Surendran2005; Surendran & Niyogi, Reference Surendran and Niyogi2003). For instance, Ingram (Reference Ingram1989) estimates the FL of the consonant /ð/ in English to be fairly low: if all instances of /ð/ became /d/, communication would hardly be hampered. If English lost the /d/–/ð/contrast, listeners would not be able to distinguish then and den out of context, but such minimal pairs are not very frequent in English.
The role of IF and FL in the acquisition of consonants
IF has been shown to have an effect on the order of emergence and the accuracy of production of consonants in the speech of children acquiring different languages, such as English and Cantonese (Stokes & Surendran, Reference Stokes and Surendran2005). A negative correlation was found between the age of emergence and the IF of word-initial consonants (r=−0·79, p<0·01 for Cantonese; r=−0·52, p<0·01 for English): consonants that are produced frequently in the input language are acquired earlier than consonants that are produced rarely.
Furthermore, IF is also related to the incidence of consonants in children's speech. Zamuner, Gerken and Hammond (Reference Zamuner, Gerken and Hammond2005) showed that the frequency distribution of codas in the speech of English-speaking children (N=59, age range: 0 ; 11–2 ; 1) was significantly correlated with the relative frequency of those codas in child-directed speech (CDS): consonants that are frequently produced by adults are also frequently produced by children in the early lexical period. Thus children appear to be sensitive to the frequency of patterns in the ambient language when building and organizing their phonological knowledge (Amayreh & Dyson, Reference Amayreh and Dyson2000; Stites, Demuth & Kirk, Reference Stites, Demuth, Kirk, Brugos, Micciulla and Smith2004).
According to Pye et al. (Reference Pye, Ingram, List, Nelson and van Kleek1987) FL significantly correlates with the order of acquisition of (word-initial) consonants in Quiché-speaking children (N=5, age range: 1 ; 7–3 ; 0) and English-speaking children (N=15, age range: 1 ; 5–2 ; 2). Additional support for the role of FL is provided by Stokes & Surendran (Reference Stokes and Surendran2005), who report significant negative correlations between FL and the order of acquisition in English-speaking children (N=7, age range: 0 ; 8–2 ; 1), meaning that segments that carry a smaller FL tend to be acquired later. Corroborating evidence is also offered by Amayreh and Dyson (Reference Amayreh and Dyson2000), Catano, Barlow and Moyna (Reference Catano, Barlow and Moyna2009) and So and Dodd (Reference So and Dodd1995), though no specific statistical analyses are reported.
The role of IF and FL for the order in which consonants are acquired is not unequivocally supported. For example,Levelt and van Oostendorp (Reference Levelt, van Oostendorp, Los and van Koppen2007) studied the distribution of word-initial consonants in CDS selected from the van de Weijer corpus (van de Weijer, Reference van de Weijer1999) and concluded that it did not resemble the order of emergence of these segments in six Dutch-speaking toddlers selected from the CLPF corpus (in the CHILDES database; MacWhinney, Reference MacWhinney2000). As to FL, Stokes and Surendran (Reference Stokes and Surendran2005) found a statistically significant correlation between FL and the order of acquisition of consonants in English-speaking children, but they did not find such a relationship in children acquiring Cantonese (N=51, age range: 1 ; 3–2 ; 6).
These divergent findings may be (partly) explained by differences between the languages investigated concerning the phonetic attributes and the articulatory complexity of the target inventory, the phonotactics of the target language, the FL of other phonological structures, and possibly additional factors. For example, FL is divided over segments and tones in a tonal language, whereas in a non-tonal language FL can only be attested for segments (Zhu, Reference Zhu2002). This may imply that children rely more on the FL of segments in non-tonal languages, such as English and Dutch, than in a tonal language, such as Cantonese.
Although these cross-linguistic differences may at least partly explain the divergent findings for the impact of IF/FL on consonant acquisition, there are considerable methodological issues (and even unclarities) in the studies reviewed. A first methodological issue concerns the type of ambient speech data used. Ideally, corpora of speech of the primary caretakers directed to the children participating in the study should be analyzed. However, none of the prior investigations used such ‘child-directed speech’.
A second methodological issue that is not clarified in every study concerns the treatment of variation in production forms. A well-known characteristic of spontaneous speech is its variation. For instance, the Dutch word natuurlijk /nɑtyrlək/ ‘of course’, ‘naturally’ can be found in casual speech in various different forms, including [nɑty(r)lək, ntylək, nətyk, ntyk, tylək, tylək] (Ernestus, Reference Ernestus2000). Some forms closely resemble but others only very remotely resemble the word's canonical transcription. Some variants in this example start with the same consonant as the canonical transcription, but others do not. Hence it should at least be clarified whether the canonical form or the actually produced form is used. For instance, Zamuner et al. (Reference Zamuner, Gerken and Hammond2005) analyzed parental speech extracted from English corpora in the CHILDES database (MacWhinney, Reference MacWhinney2000). Only an orthographic transcription was available to which a standard phonetic transcription from the electronic version of Webster's Dictionary of American English was added. Consequently, the typical variation in the form of spontaneous productions was completely ignored, and it remains unclear to what extent this influenced the findings.
Probably the most important methodological issue relates to the exact definition of FL. Although at first sight the intuition underlying FL appears to be shared in the literature, far less agreement exists about the exact formal computation of FL. The principle of FL has been applied in several disciplines concerned with language and speech, such as synchronic and diachronic linguistics (e.g. Greenberg, Reference Greenberg1966; King, Reference King1967; Martinet, Reference Martinet1955), speech recognition (e.g. Wang, Reference Wang1967), and first and second language acquisition (e.g. Brown, Reference Brown1988; Munro & Derwing, Reference Munro and Derwing2006; Pye et al., Reference Pye, Ingram, List, Nelson and van Kleek1987; So & Dodd, Reference So and Dodd1995; Stokes & Surendran, Reference Stokes and Surendran2005). In this study the focus is on the definitions in language acquisition studies. They will be briefly introduced in this section and formally defined in Appendix I.
Pye et al. (Reference Pye, Ingram, List, Nelson and van Kleek1987) were probably the first child language researchers to propose FL as a determining factor of segmental acquisition. They defined FL as the frequency of occurrence of consonants in the word types attempted by children. However, although the incidence of consonants across lexical types may be part of a definition of FL, it is primarily a measure of IF. Two years later, Ingram (Reference Ingram1989: 217–18) proposed another way of computing FL: “FL should be measured in terms of the number of oppositions or minimal pairs in which a consonant occurs.” Hence, in this view the minimal pairs of the ambient language come into play: the FL of a segment is determined by the minimally distinct word pairs differing only in that segment. For instance, in calculating the FL of word-initial /d/, minimal pairs such as dough–though, dark–park, etc. play a decisive role. The third definition of FL was introduced in Surendran and Niyogi (Reference Surendran and Niyogi2003) and first applied in language acquisition research by Stokes and Surendran (Reference Stokes and Surendran2005). The basis of their definition of the FL of consonants is the FL of a binary opposition between two consonants, and is computed as the amount of information that would be lost if the opposition were lost. According to Stokes and Surendran (Reference Stokes and Surendran2005: 580): “The FL of a contrast C in language L requires the construction of a language LC, which is the language L if contrast C is not available. For example, if English lost the /d/-/ð/ contrast, the listener could not distinguish between then and den in the absence of context.”
Perhaps the most comprehensive coverage of the concept of FL stems from second language acquisition research. Brown (Reference Brown1988) discusses a number of aspects of the FL of segments and segmental contrasts: FL is dependent on a number of characteristics that are not mutually exclusive.
A first important aspect concerns (a) the cumulative frequency of the consonant pair /x,y/, defined as the sum of the individual frequencies of the consonants /x/ and /y/. A consonant pair with a high cumulative frequency is of greater importance than a consonant pair with a low cumulative frequency. That is, if the contrast between /x/ and /y/ disappeared from the language, or in other words, if /x/ and /y/ merged, then the intelligibility of a speaker would be much more reduced in case of a high cumulative frequency, as opposed to a contrast with a much lower cumulative frequency. Intuitively speaking it is clear that the more often particular segments occur, the greater the chance that they are the distinguishing segments in minimal pairs. However, the cumulative frequency masks the fact that one member of the consonant pair may occur less frequently than the other member. Therefore, (b) the discrepancy in the incidence between the members of the consonant pair should be considered as well. The closer the frequencies of both members of the pair are, the greater the potential confusion will be when merging them. (c) The frequencies can be computed on the basis of word type information (i.e. type frequency) or word token information (i.e. token frequency). Brown remarks that type frequency as well as token frequency should be considered.
Aspects (a), (b) and (c) concern the incidence of segments. Not only the sheer frequency of the segments but also (d) the number of minimal word pairs in which they occur, is important (cf. Ingram, Reference Ingram1989; King, Reference King1967). If a language contains a lot of minimal word pairs differing only with respect to, for instance, the consonant pair /p/–/b/ (e.g. back–pack, bet–pet, buy–pie), this pair may be rated as relatively important in that language. The discrepancy in frequency of occurrence between the members of a minimal word pair should also be taken into account (e): if one member of a minimal word pair occurs infrequently, this minimal pair is relatively unimportant in comparison to a highly frequent pair.
The next aspect concerns (f) the articulatory and/or acoustic similarity of segments. For example, the FL of only those contrasts that are typically conflated by language learners, i.e. consonants with high articulatory and/or acoustic similarity, should be taken into account, according to Brown (Reference Brown1988). More specifically, consonants differing in only one articulatory feature (e.g. /p/–/b/) are more likely to be confused than consonants differing in two or three articulatory features (e.g. /s/–/b/). Consonants /p/ and /b/ differ only with respect to voicing, whereas /s/ and /b/ differ with respect to voicing as well as to manner and place of articulation.
An additional factor is (g) the structural distribution of the consonants: the FL of a consonant pair (e.g. /p/–/b/) may differ depending on word position. It may well be that the opposition /p/–/b/ distinguishes many more minimal pairs in word-initial position than in other positions. Hence the FL of /p/–/b/ is higher in word-initial position. Therefore, word position should be taken into account when measuring FL.
The two last factors mentioned by Brown (Reference Brown1988) are (h) the number of minimal word pairs belonging to the same part of speech and (i) the number of inflections of the minimal word pairs. Factor (h) relates to the grammatical category of the words in a minimal pair. Brown refers to the fact that, for instance, English words beginning with /ð/ are predominantly function words (such as they, those, then, though). It seems unlikely that those function words would be confused with content words, such as day, doze, den and dough. Hence, in the computation of FL those oppositions should not be considered. The last factor (i) relates to a lexical/morphological issue: minimal pairs can be calculated in terms of lexemes (talk, talks, talked, talking count as one) or in terms of individual items (all four word forms are included).
As mentioned before, Brown (Reference Brown1988) clearly describes the potential relevance of several aspects for the computation of FL, but he does not propose an overarching formal procedure that incorporates them all. Instead he computes each of them separately. By evaluating and comparing the values corresponding to each aspect, he ranks the FL values of the consonant pairs.
Minimal pairs are central in the computation of Ingram's FL as well as Brown's FL. In the recent speech perception literature, opposing findings are reported on whether or not children in the earliest lexical stage are able to discriminate minimal pairs, and thus whether or not children encode and store sufficiently detailed representations of the word-initial consonants in their lexicon to discriminate minimal pairs (for an overview, see Gerken, Reference Gerken2002). In short, Stager and Werker (Reference Stager and Werker1997) found that infants aged 1 ; 2 are not able to discriminate minimal pairs in a perception experiment. However, other researchers provided corroborating evidence that even younger children can successfully discriminate two minimally distinct word forms (Swingley, Reference Swingley2009; Swingley & Aslin, Reference Swingley and Aslin2002; Jusczyk & Aslin, Reference Jusczyk and Aslin1995; Yoshida, Fennell, Swingley & Werker, Reference Yoshida, Fennel, Swingley and Werker2009). Thus, if in the current study a correlation is found between the order of emergence of consonants and FL measured using minimal pairs (as in Brown's FL and Ingram's FL), the hypothesis that children can discriminate minimal word pairs in speech perception will be corroborated, because such a finding would indicate that minimal pairs in the child's linguistic environment (at least potentially) facilitate consonant acquisition.
If IF and/or FL prove to be predictive of the order in which word-initial consonants emerge in children's speech, then a crucial issue for acquisition theory turns up. In the operationalization of both concepts frequency is a core element. But, as already indicated, frequency can be defined in terms of types or in terms of tokens. For instance, the IF of a word-initial segment can be defined as the raw number of tokens of that segment or as the number of word types in which it occurs as the initial segment. In the former case, the child has to track the raw token frequency – the raw number of exposures to the word-initial segment – and in the latter case the type frequency – the incidence of the segment in unique words in the lexicon. In the literature there is no unequivocal answer to the question whether phonological knowledge depends on type frequency or token frequency. For instance, speech accuracy (measured in non-word repetition tasks) has been found to correlate with phonotactic probability (inter aliaZamuner et al., Reference Zamuner, Gerken and Hammond2005), and both have been found to correlate with lexicon size, i.e. lexical types (Edwards, Beckman & Munson, Reference Edwards, Beckman and Munson2004). This leads to the assumption that “knowledge of sublexical units emerges from generalizations made over lexical items” (Edwards et al., Reference Edwards, Beckman and Munson2004: 421; see also Hoff, Core & Bridges, Reference Hoff, Core and Bridges2008). But in investigations (also in non-word repetition tasks) of the relative influence of lexical types and lexical tokens on phonotactic learning, Richtsmeier, Gerken and Ohala (Reference Richtsmeier, Gerken, Ohala, Chandlee, Franchini, Lord and Rheiner2009; Reference Richtsmeier, Gerken and Ohala2011) could not rule out the role of lexical tokens, and concluded that children's productions are “most sensitive to a combination of type and token frequency” (Richtsmeier et al., Reference Richtsmeier, Gerken and Ohala2011: 951). In the present article, naturalistic data are used to assess the influence of type as well as token frequency on the acquisition of word-initial consonants.
Aims and objectives
The present study aims at determining the predictive power of IF and FL for the order of acquisition of word-initial consonants. For this purpose a large corpus of spontaneous interactions between thirty children acquiring Dutch and their primary caregiver(s) is used. The children's speech forms the basis for assessing the order of acquisition of word-initial consonants, and the language addressed to them is used for the frequency counts and the computation of FL. In the foregoing, the need for a formal definition of what exactly constitutes IF and FL was highlighted. Consequently, the first purpose is to provide exact mathematical equations that pinpoint precisely which aspects of the input are considered and in what way they contribute in defining the measures. In this way, correlating the measures of the ambient language with the acquisition order in the children's speech can be achieved transparently.
But IF and FL, as represented by the definitions of Brown (Reference Brown1988), Ingram (Reference Ingram1989) and Stokes and Surendran (Reference Stokes and Surendran2005), were also shown to be composite measures. Hence, in addition to formally defining the measures, a computational procedure is implemented that permits the computation of each component and its relation to the order of acquisition of segments. Moreover, the procedure is parameterized so that the impact of each separate component on the correlation with the order of segmental acquisition can be assessed.
METHOD
Child language corpus
In order to explore consonant development, a corpus of children's speech (henceforth: CSC, Children's Speech Corpus) was collected. Longitudinal data of spontaneous interactions between thirty Dutch-speaking children and their parent(s) were collected on a monthly basis. The children were aged 0 ; 6 at the start of the data collection and 2 ; 0 when it ended. Each child (14 girls and 16 boys) lived in Flanders, i.e. the northern, Dutch-speaking part of Belgium. The infants were recruited on the basis of the following selection criteria: no health or developmental problems, normally hearing, monolingual and at ages 1 ; 0, 1 ; 6 and 2 ; 0 no repeated scores below percentile 1 on the Dutch version of the MacArthur Communicative Development Inventories, i.e. the N-CDI (Zink & Lejaegere, Reference Zink and Lejaegere2002). The parents were all normally hearing, spoke standard Dutch and had a mid to high socioeconomic status. The procedure of the study was institutionally approved and the parent(s) signed an informed consent form.
Video- and audio-recordings of naturalistic interactions between the children and their parent(s) were made in the children's homes on a monthly basis. All recordings were done with a JVC digital camera (type GZ-MG77E) and a built-in, multidirectional microphone. The observations ranged between 50 minutes and 3 hours. Immediately following the recording sessions, the investigator selected 20 voluble minutes of spoken interactions. These recordings constitute the data analyzed in the present article. On the basis of the visual and acoustic signal, three researchers made orthographic and broad phonetic transcriptions. In order to discriminate words from prelexical material, the procedure proposed by Vihman and McCune (Reference Vihman and McCune1994) was applied. The procedure takes into account several criteria in identifying a vocalization as a word. These include contextual criteria such as the mother's identification of a vocalization as a particular word, and the child's repeated use of the vocalization in a particular (referential) context. In addition the vocalization's shape is taken into account, i.e. how close is it to an adult word? Do all instances exhibit the same (or a similar) phonological shape (segmental, prosodic)?
All nineteen Dutch consonants with phonemic status were transcribed (see Table 1). Three additional consonants were also transcribed, namely /g/, /ʃ/ and /ʒ/. These consonants are put between parentheses in Table 1, because they only occur in loanwords (/g/) or as an assimilation of /s/ or /z/ with /j/ (/ʃ/ and /ʒ/ respectively). According to the phonology of Dutch, all consonants in Table 1 occur in word-initial position, except for the velar nasal /ŋ/ (Booij, Reference Booij1995).
Table 1. An overview of the Dutch consonants

Intra-rater and inter-rater reliability checks were carried out on a large sample of the transcriptions: 2,444 words were retranscribed by the original transcriber and 2,699 words were retranscribed by a colleague transcriber. The phonetic characters of the transcription pairs were automatically aligned with a computer program implementing a dynamic alignment algorithm (based on ADAPT; Elffers, Van Bael & Strik, Reference Elffers, Van Bael and Strik2005). The percentage of agreement between the original transcription and the retranscription of consonants and the kappa score were computed. The intra-rater agreement scores amounted to 84·17% agreement and Cohen's kappa=0·83; inter-rater agreement scores amounted to 70·43% agreement and Cohen's kappa=0·68. For these comparisons consonants in the two transcriptions were judged on an equal/not equal basis. When the place of articulation of the pair of consonants was considered separately, intra-rater agreement was 92·08%, kappa=0·83, and inter-rater agreement 81·14%, kappa=0·71. For manner of articulation intra-rater agreement was 91·72%, kappa=0·89, and inter-rater agreement 81·03%, kappa=0·74. For voice intra-rater agreement was 87·50, kappa=0·76, and inter-rater agreement 81·03%, kappa=0·74. Because a considerable large sample of the total corpus (15%) was retranscribed, it can be assumed that the agreement scores are sufficiently representative for the total corpus.
The observation sessions were aligned according to the language age of the children. Language age 0 ; 0 corresponds to the cumulative ten-word point, i.e. the age point at which the child produced his/her tenth word type. At that point the children's chronological ages ranged from 1 ; 1 to 1 ; 8. Language age 0 ; 1 denotes the month following the cumulative ten-word point, etc. The period analyzed starts at language age 0 ; 0 and ends at language age 0 ; 5, since from language age 0 ; 6 onwards, no sufficient amounts of data were present to determine the ‘average’ order of acquisition of segments reliably (cf. Van Severen, van den Berg, Molemans, Govaerts & Gillis, Reference Van Severen, van den Berg, Molemans, Govaerts and Gillis2010).
Order of acquisition
For every child the following procedure was implemented for establishing the order of acquisition of word-initial consonants: at each age a bootstrapping procedure was set up (cf. Van Severen, Molemans, van den Berg & Gillis, Reference Van Severen, Molemans, van den Berg and Gillis2012), which consists of four steps:
1. 1,000 random non-identical selections of N word-initial consonant tokens were drawn from the original data of a child at a particular age. Each selection is denoted by Si (0 <i <=1,000). N is equal for all 1,000 selections (for all children and all ages).
2. For each random selection Si, the relative frequencies of the nineteen word-initial (WI) consonants were computed.
3. The mean frequency of occurrence of each consonant and the 95% confidence interval around the mean were calculated over the 1,000 selections. In this way a good estimate of the relative frequency of the consonant was obtained for the child at each age.
4. If the 95% confidence interval surpassed one, the consonant was considered acquired by the child at a particular age.
For each child, this procedure was used to determine the child's age of acquisition of word-initial consonants. Finally, the median age of acquisition for all word-initial consonants was computed over all children and used for further analyses.
Ambient language corpora
For the evaluation of the ambient language factors two corpora were used: a corpus of adult-directed speech retrieved from the database Corpus Gesproken Nederlands ‘Spoken Dutch Corpus’ (for more details see http://tst.inl.nl/cgndocs/doc_Dutch/start.htm), and a corpus of child-directed speech, i.e. speech collected for the purpose of the present study.
The Corpus Gesproken Nederlands (CGN) consists of several types of speech from which spontaneous, face-to-face conversations between Dutch-speaking adults living in the northern part of Belgium were sorted out. A selection of 234,356 phonemically transcribed word tokens were used for further analyses.
The Child-Directed Speech Corpus (CDSC) consists of the adult speech directed at the thirty children participating in this study. Analyses were applied to the CDSC as a whole, which contains a total of 493,084 word tokens.
Ambient language measures
The exact procedures and equations used to compute IF and FL, as represented by the definitions of Brown (Reference Brown1988), Ingram (Reference Ingram1989) and Stokes and Surendran (Reference Stokes and Surendran2005), are formally defined in Appendix I. These formal definitions were implemented in a Python program so as to be able to process relatively large amounts of data. Moreover, the software was amply parameterized. In defining IF and FL, a number of parameters were identified. These concerned choices that can be made in the computation of the actual measures. For instance, the ambient language frequencies used can be drawn from adult-directed speech or from child-directed speech. Or, in determining the set of likely mergers of consonant /x/, S(x), three possible definitions were discussed of what constitutes a likely merger. Instead of taking an a priori decision about which alternative to implement, the software was constructed in such a way that it allowed for all the parameters to be tested. The rationale behind this choice was that although it is sensible to assume that, for instance, child-directed speech is the better option for assessing the distributional regularities of the language children hear, it may well turn out that those regularities coincide with the ones found in adult-directed speech, and hence no significant difference in the influence on the order of acquisition would be found. Table 2 provides an overview of the parameters that were systematically tested in this study.
Table 2. An overview of the parameters used in the computation of IF, Ingram's FL, Stokes and Surendran's FL and Brown's FL

legend: CDSC: Child-Directed Speech Corpus; CGN=Corpus Gesproken Nederlands [Spoken Dutch Corpus]; incl.=inclusive; excl.=exclusive; V=vowel; C=consonant; WI=word-initial; S(x)=set of likely mergers of consonant x; PoA=Place of Articulation; art. =articulatory; P(x)=incidence of consonant x; P x(y)=incidence of consonant y; E(P x,y)=entropy of the distribution of consonant x and its likely merger y; X=the parameter is applicable for a given formula; /=parameter not used in the formula.
Consequently, each of the measures for input IF and FL was not computed once, but a number of times: for each possible value of the parameters mentioned in Table 2, and for each possible combination of parameters, the measures were computed. The number of possible combinations of parameter (values) differed between the four ambient language factors. For instance, twelve combinations were possible for IF, whereas twenty-four combinations were possible for Ingram's FL.
RESULTS
The results are reported in the following sequence. First of all, the question of which ambient language factor (IF, Ingram's FL, Stokes & Surendran's FL, and Brown's FL) predicts the age of acquisition most accurately will be answered. Next, the influence of the various parameters will be assessed by means of a decision tree analysis. In addition, the ‘best predicting’ definition and the ‘best predicting’ parameter values will be determined. Finally, the interaction between IF and FL in predicting the order of acquisition of word-initial consonants will be analyzed.
Best predicting ambient language factor
In Figure 1, the Spearman rank correlations between the age of acquisition of word-initial consonants and the four ambient language factors are displayed. In each definition of IF and FL a number of parameters are used. Each possible value of these parameters (enumerated in Table 2) as well as all possible combinations were systematically computed, and yielded a corresponding correlation coefficient represented by a separate dot in Figure 1. For each type of ambient language separately, the median Spearman's ρ-values (and ranges) are reported in Table 3.

Fig. 1. Spearman's ρ correlation coefficients between IF and FL and the age of acquisition of word-initial consonants.
Table 3. Correlations between the four ambient language factors and the order of acquisition of word-initial consonants

IF and FL are negatively correlated with the order of acquisition of word-initial consonants, meaning that the higher the IF or FL of a word-initial consonant, the sooner that consonant is acquired. The strength of the correlations differs between the four ambient language factors. The strongest correlations are obtained for IF (ρ=−0·729, p<0·001) and for Ingram's FL and Stokes and Surendran's FL (both ρ =−0·786, p<0·001). Significantly less strong Spearman rank correlations are obtained for Brown's FL. A Kruskal–Wallis test reveals a significant difference in the strength of the Spearman rank correlations between the four ambient language factors (χ 2(3, N=588)=34·934, p<0·001). Table 4 presents the results of two-tailed Mann–Whitney U tests applied in paired comparisons of the four measures.
Table 4. Differences between the predictive value of IF/FL measures on the order of acquisition of word-initial consonants

* p<0·05; ** p<0·01.
The strongest ρ-value for Ingram's FL and the strongest ρ-value for Stokes and Surendran's FL are equal (ρ=−0·786, p<0·001). The corresponding combinations of parameter settings are also the same in both cases, namely the two FL formulas are based on the word type frequencies in the CDSC. The set of segments, i.e. S(x), that form an opposition with the target consonant are consonants which differ in only one feature from the target consonant. No weighting is added to the formulas. This finding suggests that, given equal parameter values, Ingram's FL and Stokes and Surendran's FL tap the same empirical phenomenon. For each ambient language factor with the strongest ρ-value, the IF or FL values for the word-initial consonants are reported in Appendix III.
Most predictive parameter values: an exploration
Which parameter values lead to the highest correlations with the order of acquisition of word-initial consonants? For IF, the number of parameter settings is relatively small. The correlation between the order of acquisition of word-initial consonants and IF was systematically varied in the following way: the frequencies were drawn from a corpus of adult-directed speech (CGN) versus a corpus of child-directed speech (CDSC). From these corpora the word-type frequencies versus the word-token frequencies were computed. In the latter case either the standard pronunciation or the actually produced word forms were used. Finally, the selection of words was restricted to words with an initial consonant versus all words (including those with an initial vowel).
Figure 2 shows that the strongest correlation is found for the child-directed speech corpus from which the actually produced word forms are used for the token frequency counts. Thus, IF derived from CDSC leads to higher correlations than frequency derived from CGN. Token frequency results in higher correlations than type frequency. The age of acquisition of word-initial consonants is correlated more strongly with the token frequency based on the actually produced word forms than with the token frequency based on the (normalized) standard pronunciation of the words. These results show that speech addressed to the children is a better predictor of their acquisition of consonants than adult-directed speech. Moreover, retaining the variability in the pronunciation which is typical for conversational speech also turns out to be beneficial for predicting the acquisition order of consonants. The parameter concerned with the inclusion or exclusion of words with an initial vowel has no influence at all. As a result, the same ρ-values are obtained for both parameter values. Consequently, half of the values in Figure 2 are identical and only six symbols instead of twelve are visible.

Fig. 2. Spearman's ρ correlations between IF and age of acquisition of word-initial consonants for adult-directed speech (CGN) and child-directed speech (CDSC).
For FL a different approach is taken. The data were entered into a decision tree analysis in SPSS and the growing method Chi-squared Automatic Interaction Detection (i.e. CHAID) was applied, i.e. a stepwise procedure that results in a decision tree. At each step, CHAID chooses the parameter that has the strongest interaction with the dependent variable, i.e. the Spearman's ρ. Parameter values are merged if they are not significantly different with respect to the dependent variable. A node presents each interacting parameter value or definition. The parameters are hierarchically and conditionally ordered: the higher the parameter value is situated, the more influence the parameter value has. A lower-situated parameter value implies the presence of the higher-ordered parameter value(s).
Figure 3 shows the decision tree evaluating the definitions and parameters values that lead to the highest correlations. A unique combination of a FL definition and particular parameter values is defined as a case. In every node, the parameter values and the mean ρ-value computed over all cases that include the parameter value are given. The decision tree in Figure 3 only shows the nodes that lead to the combination of parameter values with the highest predictive value (highlighted in bold). Note that for the lower nodes the number of cases is small, so that caution is required in interpreting the results.

Fig. 3. Decision tree for the most predictive FL definitions and parameter values for the order of acquisition of word-initial consonants.
The parameters that lead to the highest predictive values are presented in hierarchical order. The first branching relates to the type of speech data that is used. Higher FL values are obtained if FL is computed for CDSC as compared to CGN. The second branching relates to the set of consonants that form an opposition with the target consonant /x/, i.e. S(x). The most predictive S(x) is the set of consonants that differ in one articulatory feature from consonant /x/, e.g. S(b)={p, d, g, m, w, v}. The next branching concerns the specific definition of FL. Overall, Ingram's FL is more predictive for the order of acquisition of word-initial consonants than Stokes and Surendran's FL, which in turn better predicts acquisition order than Brown's FL. The node below Ingram's FL relates to S(x) again. The highest correlations occur when a deletion is not included in S(x), for example, the word pair leg–egg /lʵg/–/ʵg/ is not considered to be a minimal pair. Below the Stokes and Surendran's FL node, two additional branches are discovered: Stokes and Surendran's FL is more predictive if the FL values are derived from the standard pronunciation of the word tokens and if no weighting with P(x) is added to the FL formula. Other weighting components, P x(y) and E(P x,y), do not influence the ρ-values.
The results of the exploratory decision tree analysis are summarized in Table 5. For each FL formula, the most predictive parameter value is reported. The parameters are classified in three categories: the type of speech that is selected, the set of likely mergers of consonant /x/, i.e. S(x), and the weighting applied in the computation of FL. Note that not all parameters are used in all FL formulas, as is indicated by a backslash.
Table 5. Best predicting parameter settings of the three FL measures

legend: FL=functional load; CDSC=Child-Directed Speech Corpus; CGN=Corpus Gesproken Nederlands [Spoken Dutch Corpus]; incl.=inclusive; excl.=exclusive; V=vowel; C=consonant; WI=word-initial; S(x)=set of likely mergers of consonant x; art.=articulatory; P(x)=incidence of consonant x; P x(y)=incidence of consonant y; E(P x,y)=entropy of the distribution of consonant x and its likely merger y; U=Mann−Whitney U; X2=Chi Square; D=degrees of freedom; /=parameter not used in the formula; ≈ no significant difference between the two parameter settings; * p<0·05; ** p<0·01.
In addition to the decision tree analysis, a non-parametric statistical test (Mann–Whitney U test, Kruskal–Wallis test) was applied to determine whether a particular parameter value is a significantly better predictor for the order of acquisition than its counterpart value, computed over all three FL formulas. The parameter values that make significantly better predictions are marked with an asterisk in Table 5. For example, within the category type of speech, ‘CDSC’, ‘word types’ and ‘standard form’ are significantly better predictors than ‘CGN’, ‘word tokens’ and ‘actually produced form’, respectively. The set of likely mergers of the target consonant that includes consonants that differ from the target consonant in one articulatory feature is a significantly better predictor for order of acquisition than the sets including all consonants or the consonants that have the same place of articulation. For some parameters the various values are not significantly different predictors, namely the inclusion or exclusion of words with a word-initial vowel and the inclusion or exclusion of the weighting components.
Interaction between FL and IF
IF and FL are negatively correlated with the order of acquisition of word-initial consonants in Dutch-speaking children. In particular, the strongest Spearman's rank order correlation (ρ=−0·729, p<0·001) between IF and order of acquisition is derived from the actually produced word tokens in CDSC. The strongest Spearman's rank order correlation (ρ=−0·786, p<0·001) between FL and the order of acquisition is attained for Ingram's definition of FL using the word types in CDSC and when the set of likely mergers, S(x), consists of consonants that differ in only one articulatory feature from the target consonant.
Because these correlations for IF and FL are very similar, it may well be the case that a consonant with a high frequency of occurrence also has a high FL. Indeed, there is a significant positive correlation between the two ambient language factors (ρ=−0·728, p<0·001). Consonants with a high FL tend to have a high IF. In order to determine the unique contribution of each factor, a partial rank correlation coefficient for Spearman's ρ was computed (Sheskin, Reference Sheskin2004: 1071). The correlation between FL and acquisition order after any linear relationship between IF and FL and between IF and acquisition order has been removed is significant (ρ=−0·545, p<0·02). However, the correlation between IF and acquisition order after any linear relationship between FL and IF and between FL and acquisition order has been removed is not significant (ρ=−0·368, p>0·10). This means that when the impact of IF on acquisition order is removed, FL still exerts a significant influence, while the reverse is not true: IF does not have an additional influence on acquisition order.
In Figure 4 the IF of the word-initial consonants is plotted against their values for Ingram's FL. In addition, Appendix III lists the IF and Ingram's FL values for each consonant. The set of word-initial consonants with a high FL value (>200 minimal pairs) and/or a high word token frequency (>6%) consists of /p/, /b/, /t/, /d/, /m/, /k/, /j/, /n/ and /w/. In Figure 4, these consonants are located outside the area delineated by the lines corresponding to a FL of 200 and IF of 6%. These consonants are also the consonants that appear earliest and are most frequently used in the speech of young Dutch-speaking children. Word-initial consonants with a FL value less than 200 and which occur in less than 6% do not appear in the speech of an average Dutch-speaking child before language age 0 ; 5. This set of ‘late’ consonants mainly consists of fricatives and liquids.

Fig. 4. IF values plotted against Ingram's FL values (consonants located outside the delineated area are acquired before language age 0 ; 4).
DISCUSSION
The principal goal of this article was to investigate the relation between age of acquisition of word-initial consonants and patterns in the ambient language. Inspection of the literature revealed two commonly used measures: IF and FL. The IF of a consonant is the relative frequency of occurrence of that consonant in the ambient language. The FL of a consonant refers to the relative importance of that consonant. In this study, the effect of IF and FL on the age of acquisition of word-initial consonants was scrutinized for children acquiring Dutch. Because in the literature formal definitions of IF and FL are largely lacking, this study compares various IF/FL definitions and translates them into mathematical equations that pinpoint precisely which components of the input are considered. The definitions with the most predictive values for the order of consonant acquisition are the token frequency (IF) of segments and FL computed on minimally different word types in CDS. But IF and FL correlate significantly, hence a segment with a high IF tends to have a high FL, so that the question arises whether IF has an additional predictive power for acquisition order when the effect of FL is partialed out, and – mutatis mutandis – if FL has an additional benefit once the effect of IF is partialed out. A partial rank correlation using the most predictive definitions of IF and FL shows that FL still correlates significantly with acquisition order when the effect of IF is removed from the model. The reverse is not true: there is no additional benefit of IF when the effect of FL is withdrawn: IF has only a small, non-significant additional impact on the age of consonant acquisition.
Effect of ambient language on age of acquisition of word-initial consonants
In the present study a decisive impact of FL on the age of acquisition of word-initial consonants was established. The higher the FL of a word-initial consonant in the ambient language, the sooner that consonant is acquired by Dutch-speaking children. This finding suggests that the function of consonants, i.e. distinguishing words and their meanings, plays a role in their acquisition. The impact of the FL was already established for the development of consonants in children acquiring English, Quiché, Arabic and Spanish (Amayreh & Dyson, Reference Amayreh and Dyson2000; Catano et al., Reference Catano, Barlow and Moyna2009; Stokes & Surendran, Reference Stokes and Surendran2005). However, no such effect was found for children acquiring Cantonese (Stokes & Surendran, Reference Stokes and Surendran2005).
In addition, the present study shows a negative correlation between age of acquisition and IF. That is, the more frequently a consonant occurs in the ambient language, the earlier it appears in the children's speech. This finding confirms the results of prior studies, which also found an effect of IF on the acquisition of consonants, i.e. the age of emergence, the accuracy of production and the frequency of occurrence of consonants in the speech of young children (Amayreh & Dyson, Reference Amayreh and Dyson2000; Stokes & Surendran, Reference Stokes and Surendran2005; Tsurutani, Reference Tsurutani2007; Zamuner et al., Reference Zamuner, Gerken and Hammond2005). Moreover, the effect of IF on consonant acquisition was found for a number of languages, including Arabic, Japanese, English and Cantonese. Frequency effects are not only restricted to phonological phenomena: they were also revealed in the acquisition of other language domains, such as lexical acquisition (e.g. Goodman, Dale & Li, Reference Goodman, Dale and Li2008; Naigles & Hoff-Ginsberg, Reference Naigles and Hoff-Ginsberg1998) and the acquisition of syntax (e.g. Lieven, Reference Lieven2010; Theakston, Lieven, Pine & Rowland, Reference Theakston, Lieven, Pine and Rowland2004).
Differences between languages
Closer inspection of the reported Spearman rank order correlation coefficients between the order of acquisition and ambient language patterns reveals differences between languages. For instance, the largest correlation between the age of acquisition of word-initial consonants is found for Dutch (r=−0·79, p<0·01). Stokes and Surendran (Reference Stokes and Surendran2005) report a similar r-value of −0·74 (p<0·01) for English. However, they did not find a significant correlation for Cantonese. Similar cross-linguistic differences are also apparent with respect to the correlation between the order of acquisition and IF: a substantially stronger correlation was observed for Cantonese (r=−0·79, p<0·01; cf. Stokes & Surendran, Reference Stokes and Surendran2005) than for English (r=−0·52, p<0·05; cf. Stokes & Surendran, Reference Stokes and Surendran2005) and for Dutch (r=−0·59, p<0·01).
There are a number of possible explanations for these differences. First, the differences with respect to the correlations between the order of acquisition and IF/FL may be (partly) attributed to the methodology employed. The methodological differences relate to the children's speech data as well as to the ambient language data. As to children's speech data, studies differ in the number and homogeneity of the group of participants, the sizes of the speech samples, and the time intervals between the speech samples. That is, the more children participate in the investigation, the more accurately the ‘average’ age of acquisition can be determined. In the current study, speech samples were selected from spontaneous speech of a relatively large group (N=30) of children, while in Pye et al. (Reference Pye, Ingram, List, Nelson and van Kleek1987) and in Stokes and Surendran (Reference Stokes and Surendran2005) speech samples of respectively nine and seven English-speaking children were analyzed. Furthermore, in the present study a longitudinal corpus of monthly observations was used, so that the age of acquisition of each individual consonant could be determined for each individual child. In previous studies cross-sectional data were analyzed, resulting in different participants in each cross-section (Amayreh & Dyson, Reference Amayreh and Dyson2000; Catano et al., Reference Catano, Barlow and Moyna2009; Stokes & Surendran, Reference Stokes and Surendran2005).
Other methodological differences concern the analysis of the ambient language, and more specifically the computation of the FL values of segments. Previous studies disagree on the exact equation specifying FL, as well as on the computational procedure. In the present study the FL values of word-initial consonants were computed by means of three different formulas. Although the intuition underlying the concept of FL may be quite similar, the three formulas differ considerably as to their computational details. Ingram's FL of a consonant is simply defined by the number of minimal pairs in which that consonant occurs. Stokes and Surendran's FL is the sum of the FL of the binary oppositions of the target consonant and other segments of the language. The FL of a binary opposition is defined by the amount of information (or entropy) that is lost if the binary opposition were not available. Brown's FL in its turn takes still other elements into account: information about the number and the frequency of the minimal word pairs in which the target consonant occurs, the frequency of occurrence of the minimal consonant pairs, and the articulatory similarity between the consonants of the consonant pair. Thus, given the discrepant operationalizations of FL, it is hard to directly compare the outcomes of the various studies.
The systematic computation of the mathematical formulas reveals that the different FL measures compute different FL values for a particular consonant. Furthermore, Ingram's FL and Stokes and Surendran's FL are significantly more strongly correlated with the order of acquisition of word-initial consonants in Dutch than Brown's FL. Ingram's FL and Stokes and Surendran's FL – when combined with the same parameter values – yield the same rank order of FL values, which means that adding entropy to the FL formula (as in Stokes & Surendran's FL) does not influence the rank orders of the FL values. As a matter of course, future language acquisition studies should preferably use the same measure of FL. The results of the current study indicate that Ingram's FL is to be preferred, be it only for the sake of simplicity: Ingram's FL represents the simplest formula to compute FL.
Next, the cross-linguistic differences in the relationship between the FL of word-initial consonants on the age of emergence of these consonants may also be related to variations between the investigated languages concerning the phonetic and phonological characteristics of the target language. For example, the reliance on FL of consonants is substantially larger in non-tonal languages such as English and Dutch than in the tonal language Cantonese, for which the FL of consonants may be quite reduced in comparison to the FL of tone (Zhu, Reference Zhu2002). Further research is required to investigate the role of FL of tone and other phonological structures, such as stress and syllables, on consonant acquisition in various languages.
Most predictive parameter values
A number of parameters are used in the computation of FL and IF. These parameters are related to the type of speech from which IF and FL values are derived, the degree of articulatory similarity between the word-initial segments in a minimal pair, and the addition of a particular weighting to the FL formula.
The first parameter relates to the interactive context. It almost seems a truism that in order to arrive at a valid estimate of the frequencies with which children hear particular sounds, child-directed speech needs to be analyzed. Adults' adjustments of their language when they interact with young children are well documented (Gallaway & Richards, Reference Gallaway and Richards1994; Lee & Davis, Reference Lee and Davis2010; Snow & Ferguson, Reference Snow and Ferguson1977). Thus it appears appropriate to study possible influences of IF and FL on the order of acquisition of consonants on the basis of a quantitative assessment of child-directed speech instead of adult-directed speech. Nevertheless, studies investigating the influence of characteristics of the ambient language on phonological development often use all kinds of speech data, except child-directed speech (e.g. Pye et al., Reference Pye, Ingram, List, Nelson and van Kleek1987; Stokes & Surendran, Reference Stokes and Surendran2005). Moreover, characteristics of language addressed to young children vary depending on the age of the child, gradually giving way to more mature speech styles over time (Bellinger, Reference Bellinger1980; Garnica, Reference Garnica, Snow and Ferguson1977). Therefore, speech directed to children of the same age as the age period under investigation should be preferred. However, in previous research in which child-directed speech was examined, mostly speech directed to children younger or older than the age period under investigation was used (e.g. Stites et al., Reference Stites, Demuth, Kirk, Brugos, Micciulla and Smith2004; Stokes & Surendran, Reference Stokes and Surendran2005; Tsurutani, Reference Tsurutani2007). Finally, data often consist of speech directed to one single child (inter alia, Gillis, Reference Gillis, Gillis and Schaerlaekens2000; Levelt, Schiller & Levelt, Reference Levelt, Schiller and Levelt2000; Levelt & van Oostendorp, Reference Levelt, van Oostendorp, Los and van Koppen2007), but it is unclear how generalizable characteristics of adult input are and/or how representative the analyzed child-directed speech is.
In the present study, adults' speech directed to thirty children (CDSC) was analyzed. The correlation of this CDSC with the order of acquisition of consonants was compared to the correlations with CGN, a corpus of adult-directed speech. Results show that the order of acquisition is more strongly related to the IF/FL estimated from transcripts of parent–child interaction (CDSC) than from transcripts of adult–adult conversations (CGN).
A second parameter relates to the kinds of words used to compute IF and FL. The acquisition of consonants in children's speech is shown to be more dependent on the FL of segments occurring in word types as opposed to word tokens. This means that the best predictor of the order of acquisition is the frequency of a contrast in the lexicon of word types, and not the token frequency of that contrast in actual language use. The reverse holds for IF, namely IF derived from word tokens appears to be more predictive for the age of acquisition of consonants than IF derived from word types. Moreover, the token frequency in the actually produced forms better predicts the age of acquisition than their frequency in the standard pronunciation. Nevertheless, prior studies investigating IF in child-directed speech did not always analyze phonetic transcriptions of the adult's actual productions, but analyzed canonical phonetic transcriptions (e.g. Stokes & Surendran, Reference Stokes and Surendran2005; Zamuner et al., Reference Zamuner, Gerken and Hammond2005).
The next parameters are only relevant for FL since they concern minimal pairs. The order of acquisition of word-initial consonants depends on the articulatory similarity between the members of a minimal pair. Significantly better correlations were obtained when the initial consonants in a minimal pair differed in only one articulatory feature (either manner, place or voice), than when the differences were larger (variation of two or three articulatory features). Stokes and Surendran (Reference Stokes and Surendran2005) advocate a third possibility, viz. minimal pairs differing in manner or voice only. They argue that the set of consonants with which a consonant is likely to merge is related to the types of production errors language learners tend to make (cf. Brown, Reference Brown1988): the most frequent error is a substitution that shares the same place of articulation, but differs in manner or voicing (Paschall, Reference Paschall, Irwin and Wong1983). It was shown in the current study that FL values are significantly weaker when the set of likely mergers S(x) equals the set of consonants with the same place of articulation (cf. Stokes & Surendran, Reference Stokes and Surendran2005) than when S(x) equals the set of consonants differing in one articulatory feature, not restricted to place of articulation.
Lastly, in Stokes and Surendran's computation of FL a number of weights can be applied, viz. weighting by the frequency of occurrence of a consonant, the frequency of occurrence of the set of likely mergers of the consonant, and the discrepancy between these frequencies. Although Brown (Reference Brown1988) suggests that these weights play a significant role in the computation of FL, no significantly better correlations between FL and the age of acquisition of word-initial consonants were obtained in this study for any of these weights. Thus, although analytically speaking various weighting schemes may enrich the concept of FL, they were not validated empirically in the present study.
The role of minimal pairs
The present study reveals a significant tie between FL of word-initial consonants in the ambient language and the order of emergence of those consonants in children's actual speech. The strongest correlations were noted for FL defined in terms of the number of minimal pairs in adult speech addressed to children. If minimal pairs play a role, children must be able to discriminate the members of minimal pairs and represent them appropriately. Prior speech perception studies report contradictory findings about infants' ability to discriminate minimal word pairs. Stager and Werker (Reference Stager and Werker1997) reported that infants aged 1 ; 2 could not discriminate newly learned minimal pairs in a perception experiment. Later studies show that children are able to discriminate familiar words when they are presented in minimal pairs (Jusczyk & Aslin, Reference Jusczyk and Aslin1995; Swingley, Reference Swingley2009; Swingley & Aslin, Reference Swingley and Aslin2002; Yoshida et al., Reference Yoshida, Fennel, Swingley and Werker2009). These studies are all experimental ones, while the current investigation analyzed speech from the infants' natural environments, in which words occur in variable forms and over a relatively long period. Whether these natural learning conditions are superior to the concentrated exposure provided in the training task in a laboratory setting (Yoshida et al., Reference Yoshida, Fennel, Swingley and Werker2009) cannot really be decided on the basis of the present results. However, the present study entails that young children are able to discriminate minimal word pairs given that the order of emergence of consonants correlates with the FL of those consonants as defined by the amount of minimal pairs in which the consonants occur. This also seems to imply that these words are encoded and stored with enough phonetic detail to tell them apart.
Minimal pairs were defined very strictly in this study as pairs of words that differ only in their initial segment, e.g. /pʵt/–/bʵt/. The effect of extending this notion to include ‘near minimal pairs’ such as /pʵt/–/bʵk/ or even /pʵt/–/bɑt/, still needs to be studied. Extending the focus of the investigation from strict minimal pairs to ‘near minimal pairs’ broadens the scope to lexical neighborhoods, and entails a reformulation of the research question: Is there an effect of the density of lexical neighborhoods in the input on the order of acquisition of word-initial consonants? That is, instead of counting the frequency in the input of pairs like /pʵt/–/bʵt/, the study is extended to an analysis of the set of items that cluster together as neighbors in lexical space (Stoel-Gammon, Reference Stoel-Gammon2011). It remains to be investigated if word-initial consonants that appear in dense neighborhoods emerge earlier than consonants from less dense neighborhoods.
Teasing apart multiple determinants of acquisition order
This study computed the correlations between the order of emergence of word-initial consonants and two factors of the input: the frequency of the consonants in the ambient language and their functional load. Both factors were found to correlate significantly with the order of emergence, although both factors measure different aspects of child-directed speech. Thus the logical next question is: What is the relative contribution of these factors to the order of acquisition of word-initial consonants? FL appears to correlate more substantially with the order of acquisition than IF: a partial correlation reveals that when the effect of IF is partialed out, FL still shows an additional significant effect. Consequently, this result also sheds some light on the importance of lexical types and tokens: since the FL of segments in word types has a higher correlation with the order of emergence of word-initial segments, children must tally the incidence of segments in unique words in the lexicon. However, since IF and FL are highly correlated, these analyses are not able to pull apart the unique contribution of each factor, nor can they pull apart the exact role of lexical type frequency and token frequency as such. Experimental manipulations of these factors may provide the only way to actually control them.
A similar observation holds with respect to other determinants of acquisition order. In the current article, the effect of IF/FL was found to be highly significant: word-initial segments with the highest IF/FL tend to be acquired earliest. But, as Rose (Reference Rose, Pellegrino, Marsico, Chitoran and Coupé2009) points out, the consonants with the highest IF/FL may also be the ones with the least articulatory complexity and/or the highest perceptual salience, and in addition they may be representationally the simplest. And indeed, IF/FL predict that coronals, labials and plosives are acquired first. But plosives, coronals and labials are articulatorily the least complex (e.g. Kent, Reference Kent, Ferguson, Menn and Stoel-Gammon1992), and they are universally unmarked (Gilbers & van der Linde, Reference Gilbers, van der Linde, Beers and Peters1999). This implies that IF/FL, articulatory complexity and representational complexity make similar predictions with respect to the developmental order of consonants in Dutch. Thus the unique contribution of each of these factors remains to be determined. In order to pull apart the unique contributions of these various factors, cross-linguistic research may offer an outcome. A case in point is offered by Pye et al. (Reference Pye, Ingram, List, Nelson and van Kleek1987): in Quiché /l/, /tʃ/and /χ/ have a much higher IF/FL than in English, and these consonants are acquired sooner by Quiché-speaking than by English-speaking children, hence confirming the impact of FL of consonants in the ambient language on consonant development. A similar finding is reported by Catano et al. (Reference Catano, Barlow and Moyna2009): Spanish-speaking children acquire the liquid /l/ earlier than English-speaking children, because /l/ has a higher FL and token frequency in Spanish than in English.
Another direction of research that may prove fruitful for detecting the contributions of IF and FL concerns the study of individual learning profiles. In the present study, the median order of emergence of consonants was used, averaging over all children. In addition, the CDS was compiled into one dataset. Instead, by studying the correlation between the order of emergence of consonants in the child and IF/FL of consonants in the caregivers' speech in each individual child–caregiver dyad, the impact of differences in IF and FL can be determined. Subtle differences in IF/FL may lead to differences in the order of acquisition of consonants.
Note that it is also possible that the use of ‘language age’ in the present study, as opposed to real age, has the effects of blurring important aspects of variability. For example, children with language age 0 ; 0 vary substantially regarding their real age (1 ; 1–1 ; 8). Obviously, children aged 1 ; 8 have more advanced motor control abilities and a more adult-like vocal tract shape than infants aged 1 ; 1. If motor aspects of speech articulation influence consonant development, the route and rate of consonant development may vary between early and later talkers.
CONCLUSION
The current investigation shows a negative correlation between patterns in the ambient language and the age of acquisition of word-initial consonants. More specifically, the higher the IF and the higher the FL of a word-initial consonant, the earlier the consonant appears in the spontaneous speech of Dutch-speaking children. The predictive value of a number of parameters involved in the computation of IF and FL revealed that the degree of correlation between age of acquisition and IF/FL depends on the interactive context, the type–token frequency, and the degree of articulatory similarity between consonants involved in the computation of FL. A partial rank correlation between the most predictive IF and FL definition appoints FL as the determinant factor, but attributes only a small non-significant role to IF. These findings suggest that the discriminatory function of word-initial consonants in minimal pairs of words produced in the speech directed to a child has a decisive influence on the age of acquisition of word-initial consonants in the child's word productions.
APPENDIX I
Ambient language measures
The first ambient language factor is IF. The frequency of a word-initial consonant is defined as the sum of the frequencies of the words starting with that consonant. IF incorporates four parameters. The first parameter concerns the type of speech data, i.e. adult-directed speech (CGN) or child-directed speech (CDSC). The second parameter concerns the kind of words for which the frequency is computed: word types (i.e. type frequency) versus word tokens (i.e. token frequency). A word type denotes a unique adult word. A word token stands for any attempt to actually produce a word type. For example, if a parent produces cat /kæt/ five times, the type frequency of the initial consonant /k/ is one, and its token frequency is five. Note that cat may be pronounced differently at different occasions, e.g. four times as [kæt] and once as [gæt]. If only the actually produced word forms are taken into account, the token frequency of /k/ is four instead of five in that case. Therefore, a third parameter was introduced, which distinguishes token frequency based on the standard pronunciation and token frequency based on the actual pronunciation of the word forms. No such distinction is made for word types, for which only the standard pronunciation is considered. The fourth parameter relates to which words are selected. There are two possibilities: either all words are selected, or only words with a word-initial consonant are selected.
The second ambient language factor is FL. The general idea underlying the concept FL is fairly well agreed upon in the literature. However, a precise mathematical definition is subject to much discussion. Consequently, three definitions of FL were used in this study (cf. Ingram, Reference Ingram1989; Stokes & Surendran, Reference Stokes and Surendran2005; Brown, Reference Brown1988). An overview of the definitions and their corresponding parameters are provided in this section.
Before turning to the exact definitions, some general concepts need to be elucidated. A language is defined as a list of words and is denoted by L. This means that the single word is considered as the basic element of the language (cf. Vihman & Croft, Reference Vihman and Croft2007). Each word, commonly indicated by w, occurs a finite number of times in a language. The frequency of a word, relative to the set of all words in the language, is denoted by P(w). Furthermore, two words form a minimal pair if they are equal, except for the word-initial segment (e.g. dough–though, hat–bat, park–dark).
Ingram's FL
Ingram (Reference Ingram1989: 217–18) defines the FL of a segment in terms of the number of oppositions or minimal pairs in which the segment occurs. Four parameters can be used in the computation of Ingram's FL.
The first parameter concerns the source of the language (L). The language can be constructed given the words in the adult-directed speech corpus or given the words in the child-directed speech corpus.
The second parameter relates to which words are taken from those two sources to create the respective languages. There are two possibilities: either all words are selected, or only words with a word-initial consonant. In the former case, the Dutch words /pɔp/, /sɔp/ and /ɔp/ belong to the language, while in the latter case only /pɔp/ and /sɔp/ do. Hence this parameter has an impact on the minimal pairs in the language.
The third parameter relates to the set of segments a particular word-initial consonant, say /x/, is likely to form an opposition with; this set is denoted as S(x). S(x) can be unrestricted, which means that all minimal pairs are considered. But, following Brown (Reference Brown1988), this set can be restricted to those consonants that are articulatorily similar. Articulatory similarity can be expressed in terms of the number of articulatory features involved in the substitutions. The articulatory features used in this study are voicing (voiceless, voiced), place of articulation features (labial, coronal, dorsal) and manner of articulation features (plosive, fricative, nasal, liquid, glide). Three definitions of S(x) will be explored: (1) S(x) is restricted to the consonants with the same place of articulation; (2) S(x) is restricted to the consonants that differ in one articulatory feature; and (3) S(x) is not restricted at all so that all possible substitutions leading to minimal pairs are allowed. For instance, if the language consists of four words, viz. L={/pɔp/, /sɔp/, /bɔp/, /kɔp/}, then according to the first restriction, S(p)={b} since /s/ and /k/ do not have the same place of articulation as /p/. Consequently there is only one minimal pair in the language, viz. /pɔp/–/bɔp/. According to the second restriction, S(p)={b, k}, and S(p) defines two minimal pairs in the language, viz. /pɔp/–/bɔp/ and /pɔp/–/kɔp/. According to the third restriction, S(p)={b, s, k}, and thus /pɔp/ forms a minimal pair with the other three words in the language.
Finally, the fourth parameter determines whether or not a deletion is allowed in defining S(x). For instance, if the word-initial /p/ is deleted, the words pop /pɔp/ ‘doll’ and op /ɔp/ ‘on’ form a minimal pair as well.
Given the four parameters and their respective possible values, computing Ingram's FL does not result in one single outcome, but each combination of parameter values results in a FL measure. Thus, for instance, using the child-directed speech corpus yields twelve FL values, and so does using the adult-directed speech corpus.
Stokes and Surendran's FL
According to Stokes and Surendran (Reference Stokes and Surendran2005), FL measures the amount of information that is lost when a particular consonantal opposition would be lost (see also Hockett, Reference Hockett1967). The amount of information of a language is measured by determining the entropy of that language. Entropy is a statistical measure that handles frequencies logarithmically, proportional to the diversity of a frequency distribution. Higher entropy means higher diversity. Consider the frequency distribution of a language, P, with m elements. The frequency of element i is denoted by p i. The entropy of this distribution, E(P), is defined in formula (1).
The basis of Stokes and Surendran's FL of a consonant is the weighted sum of the FL of the binary opposition between two consonants. The FL of a binary opposition of two consonants can be measured as the relative loss of diversity if the two consonants become a new, previously unused consonant. The frequency distribution attained by merging two consonants /x/ and /y/ is denoted by P xy. For example, suppose P is defined as {(dark, 10); (park, 5); (bat, 4); (hat, 6)}. If d and p are merged, P dp is defined as {(Δark, 15); (bat, 4); (hat, 6)}, where Δ is a new, previously unused symbol. The FL of the binary opposition between consonants x and y, is defined in formula (2).
In formula (3), the FL of a consonant /x/ is defined as the weighted sum of the FL of the binary oppositions between /x/ and the members of S(x), i.e. the set of likely mergers of consonant /x/.
The FL of a binary opposition between /x/ and its likely merger /y/ is weighted by the frequency of /y/ relative to all the likely mergers of /x/, as defined in formula (4).
 $$\hfill P_{x} \lpar y\rpar \equals {{P\lpar y\rpar } \over {\sum\limits_{z \in S\lpar x\rpar } {P\lpar z\rpar } }}\hfill $$
$$\hfill P_{x} \lpar y\rpar \equals {{P\lpar y\rpar } \over {\sum\limits_{z \in S\lpar x\rpar } {P\lpar z\rpar } }}\hfill $$The parameters that can be tweaked are largely the same as those discussed with respect to IF and/or Ingram's FL: the type of adult speech (i.e. adult-directed versus child-directed speech), the kind of words (i.e. word tokens versus word types), the pronunciation of the word tokens (i.e. standard pronunciation versus actual pronunciation), the inclusion or exclusion of words with a vowel in word-initial position, the set of likely mergers of /x/, i.e. S(x), and the inclusion or exclusion of deletions in S(x). Three additional weighting parameters can be defined for Stokes and Surendran's FL. First of all, FL(x, y) in formula (3) can be weighted with P x(y), i.e. the relative frequency of the likely merger of /x/. This is the weighting originally proposed by Stokes and Surendran (Reference Stokes and Surendran2005). Alternatively, FL(x,y) can be weighted with P(x), i.e. the frequency of the consonant /x/ in the language, which results in formula (5).
Third, FL(x,y) can be weighted with the discrepancy in frequency between consonants /x/ and /y/. Let P be the distribution of a language. Then, P x,y is the distribution limited to the two consonants /x/ and /y/. The discrepancy between /x/ and /y/ is measured as the entropy of the x,y-limited distribution P x,y, denoted by E(P x,y). The adjusted FL definition is presented in formula (6).
The three weighting parameters can be switched on and off, and all possible combinations can be computed, which results in a set of different weighting schemes.
Brown's FL
Starting from Brown's (Reference Brown1988) enumeration of aspects relevant for FL, a formal definition can be constructed. Let L be a language, then |L| denotes the size (number of elements) of the language. Given P, a frequency distribution, and w, a word, then P(w) represents the relative frequency of w. Two important sets need to be defined. The first set is the set of all the words in language L that start with a particular consonant /x/, i.e. the target consonant for which the FL is computed. This set is denoted by startsWith L(x). The second set consists of the words that form a minimal pair with a word w, including w, and it is denoted by MP(w). The factors defining the contribution of word w with word-initial consonant /x/ to the FL of /x/ can now be formulated.
First of all, a word w∊startsWith L(x), which is not involved in any minimal pair with another word in the language, does not contribute to the FL of consonant /x/. For this purpose Δ w is introduced, which equals 0 if word w is not involved in a minimal pair, and 1 otherwise.
The fraction of the language that is covered by MP(w) can now be calculated by formula (7).
The distribution of frequencies in MP(w) is weighted by W w (see formula (8)). If all words in MP(w) occur equally likely, W w will have its highest value: the FL of consonant /x/ is high. If, on the other hand, the words in MP(w) are very unequally represented in the language, this factor will have a low value.
 $$\hfill W_w \equals {1 \over {1 \plus \sum\limits_{v \in MP\lpar W\rpar } {\left\vert {{1 \over {MP\lpar w\rpar }} \minus } \right.\left. {{{P\lpar v\rpar } \over {C_w}}} \right\vert} }}\hfill $$
$$\hfill W_w \equals {1 \over {1 \plus \sum\limits_{v \in MP\lpar W\rpar } {\left\vert {{1 \over {MP\lpar w\rpar }} \minus } \right.\left. {{{P\lpar v\rpar } \over {C_w}}} \right\vert} }}\hfill $$Finally, the contribution of w is weighted by its frequency relative to all words starting with /x/. This factor, denoted by P x(w), is defined in formula (9).
The FL of consonant /x/ can now be defined as a combination of the factors Δ w, C w, W w and P x(w) of all the words in the language that start with /x/, as in formula (10).
This definition of FL takes into account most aspects discussed by Brown (Reference Brown1988), except for those aspects which relate to the lexical and grammatical characteristics of the input (aspects (h) and (i) mentioned in the introductory section). These aspects require a morphosyntactic annotation of the data, which was not available for the empirical part of the current study.
The parameters that can be tweaked are the same as for Ingram's FL, including one additional parameter, i.e. the kinds of words. Brown's FL incorporates information of both word types and word tokens, while Ingram's FL only uses information about word types.
Implementation
Table I.1 provides an overview of the different aspects of FL starting from the Brown's (Reference Brown1988) enumeration. As can readily be seen in Table I.1, Ingram's definition of FL is a very simple one in comparison to the two other measures. Only the number of minimal pairs (d) is involved. Stokes and Surendran's definition takes into account four aspects identified by Brown: in addition to the number minimal pairs (d), also aspects (e), (f) and (g) are considered. Although non-trivial, it is shown in Appendix II that aspect (e) is indeed also covered. Brown's definition of FL is by far the most extensive one. In the present study aspects (a)–(g) are implemented.
Table I.1. Aspects of the input in the computation of FL
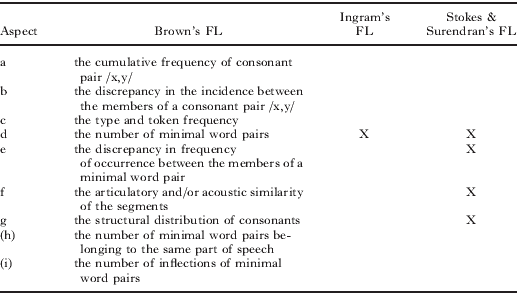
APPENDIX II
In order to prove that Stokes and Surendran's definition of FL incorporates Brown's aspect (e), it has to be shown that the FL is maximal when the two members of a minimal pair occur equally likely and is non-maximal otherwise. The Stokes and Surendran FL measures the relative difference in entropy between the original distribution P of the language units and the distribution P' in which all minimal pairs (of a particular pair of consonants) have been merged. For the sake of simplicity, assume, without loss of generality, that there is only one minimal pair in the distribution. Call the members of the minimal pair X and Y. The frequency of these elements is respectively x and y. Assume further that distribution P consists of n+2 elements. The elements, differing from X and Y, are simply denoted by their frequencies: x1 to xn. For the ease of notation, we introduce a shorthand for the sum of the frequencies of the elements (without X and Y), called C (11), and the sum of weighted logs of the frequencies of the elements of P (again, without elements X and Y), called E (12):
Note that C is a positive real number smaller than or equal to one and E is a negative real number. The frequency of element Y can be rewritten as y=1−C−x.
The analysis now boils down to finding the value of x for which the relative difference in entropy between distributions P and P' is maximal. Some basic computation of the relative difference in entropy results in (13):
 $${{\E\lpar P\rpar \minus E\lpar P'\rpar } \over {E\lpar P\rpar}} \cr \hfill\openup3 \eqalign{ \tab \equals 1 \minus {{ \minus \lpar x \plus y\rpar \log \lpar x \plus y\rpar \minus E} \over { \minus x\log x \minus y\log y \minus E}} \cr \tab \equals 1 \plus {{ \minus \lpar x \plus y\rpar \log \lpar x \plus y\rpar \plus E} \over { \minus x\log x \minus y\log y \minus E}} \cr}\hfill$$
$${{\E\lpar P\rpar \minus E\lpar P'\rpar } \over {E\lpar P\rpar}} \cr \hfill\openup3 \eqalign{ \tab \equals 1 \minus {{ \minus \lpar x \plus y\rpar \log \lpar x \plus y\rpar \minus E} \over { \minus x\log x \minus y\log y \minus E}} \cr \tab \equals 1 \plus {{ \minus \lpar x \plus y\rpar \log \lpar x \plus y\rpar \plus E} \over { \minus x\log x \minus y\log y \minus E}} \cr}\hfill$$From the fact that P is a distribution it can be inferred that x+y+C=1. Consequently, x+y <1. This means that (x+y) log(x+y) is a negative number. As a result, equation I.1 is a sum of a positive number (1) and a negative number (the fraction). It can be inferred that the relative difference in entropy is thus maximized when the denominator of the negative part is maximized. The value of the denominator is dependent on the values of x and y, i.e. the respective frequencies of elements X and Y. It was already established that y depends on x and that E is independent of x and y. Thus to maximize −xlog x−ylog y−E, −xlog x−ylog y needs to be maximized, which can be reformulated as −xlog x−(1−C−x)log(1−C−x). Fig. II.1 shows this function of x, for four different values of C. The maximum of this function depends on the value of C, but is always located at x=(1-C)/2. The value of y is then y=1 – C – x=1 – C – (1-C)/2=(1-C)/2. This proves our point: the relative difference in entropy of merging elements X and Y is maximal if the frequencies of elements X and Y are equal.

Fig. II.1. The trajectory of y =−xlog x−(1−C−x)log(1−C−x) for different values of C.
Appendix III
Table III.1. Word-initial consonants in Dutch: order of acquisition, IF and FL computed by means of the most predictive formula and combination of parameter values















