Introduction
Hyperthermia is used for cancer therapy. Clinical results demonstrated that temperature in the range of 41–45 °C is needed for hyperthermia, but care should be taken to keep the temperature of surrounding healthy tissue below 41 °C. The effect of the thickness of fat overlying the muscle layer is an important aspect of research for microwave hyperthermia. The fat layer behaves like a shielding structure due to much smaller conductivity and dielectric constant than muscle layer, and hence restricts microwave to effectively penetrate into the muscle tissue to heat the tumors [Reference Chuang1]. Also, the large thickness of fat layer causes less power to reach the muscle tissue region and hence less heating of the muscle tissue, which necessitates higher power to be fed to the applicator for effective hyperthermia. The effect of fat thickness on the heating pattern of the microwave applicator is investigated in reference [Reference Chou2, Reference Curto3]. Various applicators terminated in planar fat-muscle phantom are reported in the literature, for example, multimodal applicator [Reference Lin, Kantor and Ghods4], Lucite-cone waveguide applicator [Reference Van Rhoon, Rietveld and Van Der Zee5], and rectangular aperture source [Reference Guy6]. In reference [Reference Guy6], theoretical expressions for electromagnetic (EM) fields and associated heating pattern in layered biological tissue due to a rectangular aperture source are reported, in which the source generates linearly polarized aperture field distribution. Further, circularly polarized and implantable antennas are also reported for bio-medical applications [Reference Maity, Barman and Bhattacharjee7, Reference Bhattacharjee8].
Since the induced field distribution and heating pattern in inhomogeneous bi-layered media due to exposure of the media to near field of the applicator are complicated functions of many bio-media and applicator parameters, it is very important to study the effects of fat thickness on the interface reflection parameters and specific absorption rate (SAR) distribution in the bio-media for hyperthermia applications. Treatment quality can be optimized with the help of EM and thermal simulators. Thermal simulation can be performed for achieving desired temperature distribution as well as optimizing heating of tumor compared with normal tissue [Reference Paulides9].
Recently, Singh and Singh [Reference Singh and Singh10, Reference Singh and Singh11] demonstrated the characteristics of water-loaded metal diagonal horn (MDH) in direct contact with homogeneous muscle-medium for hyperthermia application. In realistic hyperthermia applicator, muscle along with skin and fat layers is present. Tri-layered skin-fat-muscle tissue models with fixed layer thicknesses have provided further details in an effort to approach towards realistic scenario but do not quantify the effects for a range of fat layer thickness in human tissue [Reference Gupta and Singh12, Reference Trefna13]. It is to be noted that the study of the effect of fat thickness on the applicator's characteristic is very important. Hence, the present study focuses on the theoretical computation of SAR distributions in the planar fat-muscle bi-layered phantom owing to water-loaded MDH, and other antenna related parameters at industrial, scientific, and medical (ISM) frequency of 915 MHz. Additionally, to visualize the hyperthermia performance of the applicator, simulations were performed in which the tri-layered bio-media without and with the embedded tumor is in direct contact with the applicator. To the best of the authors’ knowledge, no theoretical and/or simulation work has been done on water-loaded MDH demonstrating the effect of fat thickness on the characteristic of hyperthermia applicator.
The objectives of the present paper are to investigate, theoretically/through simulation, the SAR and/or temperature distributions in the phantom bi-layered bio-media (fat and muscle layers)/tri-layered bio-media (skin, fat, and muscle layers) with direct-contact water-loaded MDH at 915 MHz for hyperthermia. The effects of fat thickness on the input reflection coefficient/reflection coefficient at the interface between the MDH and bi-layered media, applicator's aperture admittance and the peak SAR in the muscle layer are also studied through simulation and/or theoretically at 915 MHz. The plane wave spectral technique is employed for theoretical analysis. The heating process is simulated using EM and thermal solver. For EM simulations, CST Microwave studio (MWS) software is used while CST Multi-physics software is employed for thermal simulations [14].
Design and theory
Applicator design
The design procedure given by Love [Reference Love15] for empty horn terminated in free space and given in reference [Reference Singh and Singh11] for water-loaded MDH terminated in homogeneous muscle medium was adopted to achieve water-loaded MDH terminated in bi-layered media (fat and muscle) at ISM frequency of 915 MHz. For the sake of brevity, the detailed design procedure of the present antenna is not reported here. The optimized dimensions of the MDH antenna designed at 915 MHz are given in Figs 1(a) and 1(b). The structure of water-loaded MDH terminated in bi-layered bio-media is shown in Fig. 1(c). The conducting ground plane of size 250 × 250 mm2 is used in xy-plane of the aperture of the antenna for significant suppression of fringing electric field.
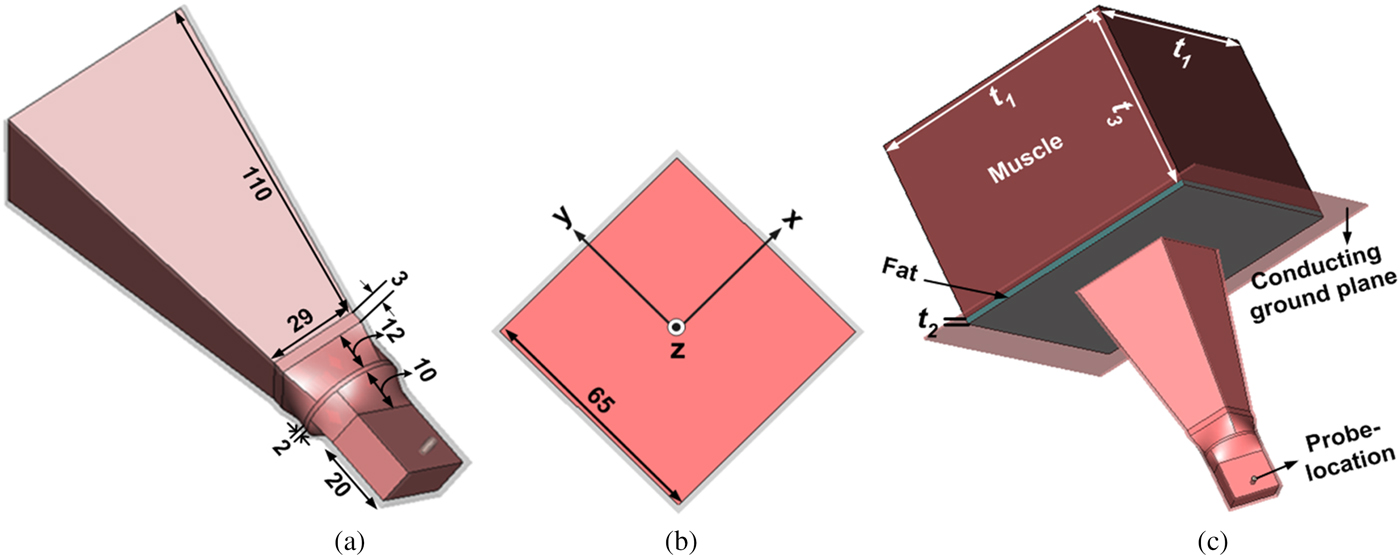
Fig. 1. Geometry of MDH (a) 3-D view (b) front view (c) terminated in a bi-layered bio-media (all dimensions are in mm).
Theory
Analysis of field components inside the bi-layered media (Fat and muscle layers)
The analysis of fields in bi-layered media is carried out using the plane wave spectral technique discussed by Compton [Reference Compton16] and Harrington [Reference Harrington17]. Recently, the induced field components in homogeneous muscle medium due to known field distributions at the apertures of water-loaded MDHs designed at 915 and 2450 MHz was determined using plane wave spectral technique [Reference Singh and Singh11]. In the present paper, the plane wave spectral technique has been employed to theoretically evaluate the induced field components in an inhomogeneous two-layered bio-media (fat and muscle) due to the water-loaded MDH designed at 915 MHz. In the present analysis, the fat layer is assumed to be of finite thickness “t 2” followed by an infinite muscle layer. Fat and muscle layers have complex relative permittivities of 
$\varepsilon _1^* $ and 
$\varepsilon _2^* $ respectively. Reference [Reference Singh and Singh11] can be referred for the detailed analysis of induced field components in the homogeneous muscle medium due to MDH.
The x-, y-, and z-components of electric and magnetic fields in the ith inhomogeneous bio-layer (i = 1 for fat layer and i = 2 for muscle layer) and hence, the electric field induced in the bi-layered bio-media (fat and muscle layers) due to the applicator are determined as given in the reference [Reference Singh and Singh11, Reference Compton16] for homogeneous bio-medium.
The resultant electric field intensity in the ith biological layer is given by
$$\left \vert {E_i{\kern 1pt}} \right \vert ^2 = \left \vert {E_{x_i}} \right \vert ^2 + \left \vert {E_{y_i}} \right \vert ^2 + \left \vert {E_{z_i}} \right \vert ^2,$$where 
$E_{x_i}$, 
$E_{y_i},$ and 
$E_{z_i}$ are the x-, y- and z- components of the induced electric field in the i th biological layer.
The SAR in the i th biological layer (i = 1, 2) can be evaluated by
$${\rm SAR} = \displaystyle{{\sigma _i{\left \vert {E_i} \right \vert} ^2} \over {2\rho _i}},$$where σ i and ρ i are the conductivity and density of i th biological layer respectively, and |E i| is the magnitude of total induced electric field strength inside the i th biological layer.
Aperture admittance and reflection coefficient at the interface between the antenna aperture and bi-layered bio-media
The aperture admittance [Reference Singh and Singh11, Reference Compton16] of the MDH terminated in phantom bi-layered (fat and muscle) bio-media is given by
$$\eqalign{Y_A = &\int\limits_{ - d/2}^{d/2} \int\limits_{ - d/2}^{d/2} \bigg\{ {\overrightarrow E} _{x1}(x,y,0).{\overrightarrow H} _{y1}^ {^\ast} (x,y,0) \cr & - {\overrightarrow E} _{y1}(x,y,0).{\overrightarrow H} _{x1}^ {^\ast} (x,y,0) \bigg\} {\rm d}x{\rm d}y,}$$where 
$\overrightarrow E _{x1}(x,y,0),\overrightarrow E _{y1}(x,y,0)$ and 
$\overrightarrow H _{x1}^ * (x,y,0),\overrightarrow H _{y1}^* (x,y,0)$ are the x and y components of electric and magnetic fields at the MDH aperture (z = 0) respectively, and d (=65 mm) is the aperture dimension of the MDH designed at 915 MHz.
Reflection occurs due to changes in physical properties at the interface of MDH and fat-layer. Therefore, reflection coefficient at the interface can be written as
$$\Gamma = \displaystyle{{Z_A - Z_0} \over {Z_A - Z_0}},$$where Z A (=1/Y A) is the impedance at the MDH aperture, Z 0 is the characteristic impedance of the MDH [Reference Singh and Singh11].
Results and discussion
Applicator's characteristics and SAR distributions in bi-layered bio-media
The theoretical variations of aperture admittance and reflection coefficient at the interface between the MDH antenna and the fat layer, and normalized SAR distributions in the bi-layered bio-media (fat and muscle layers) for fat thickness (t 2) of 5 mm were obtained at 915 MHz by solving the equations given in the section “Design and theory” using MATLAB software. In the present investigation, muscle layer is assumed to be of infinite thickness. The applicator's design data along with dielectric properties and density of phantom bi-layered bio-media (fat-muscle media) given in reference [Reference Singh and Singh11], Table 1 and Fig. 1 have been used to obtain the theoretical and simulated SAR distributions. The theoretical variations of aperture admittance and reflection coefficient at the interface between the MDH and fat layer versus fat thickness were determined using equations (3) and (4) and the results are illustrated in Fig. 2. The variation of reflection coefficient obtained at the interface between the MDH aperture and fat layer (that is dependent upon aperture as well as characteristic admittances of the MDH) with fat thickness indicates that as the thickness of fat layer increases, impedance matching between the MDH aperture and the fat layer becomes progressively worse.
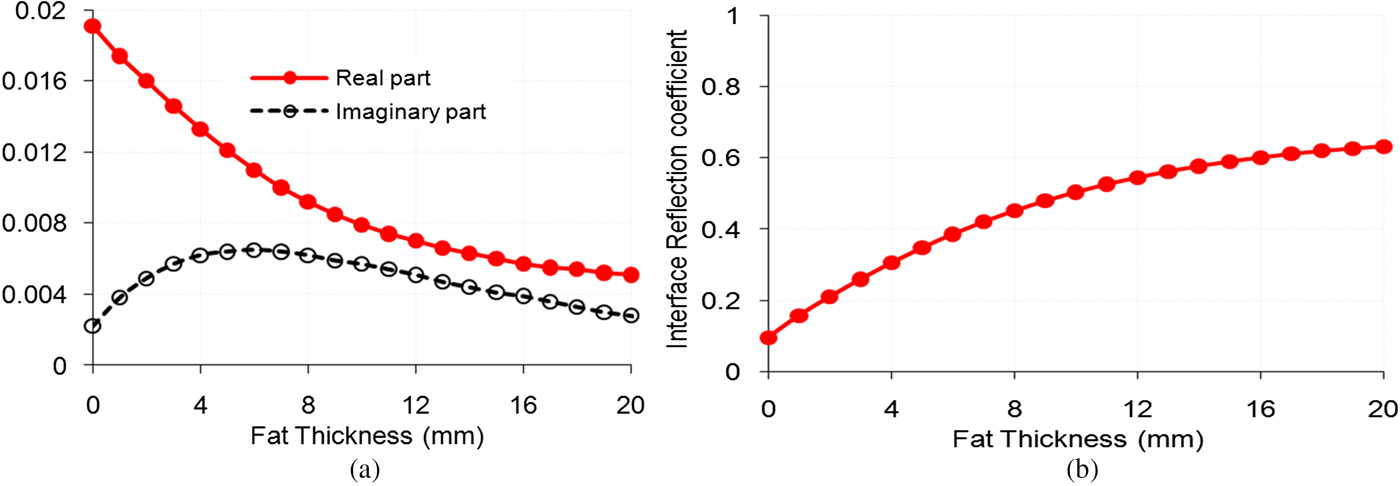
Fig. 2. Variations of theoretical (a) aperture admittance (b) reflection coefficient at the interface of the MDH and bi-layered tissue as functions of fat thickness.
Table 1. Dielectric properties of bio-media [Reference Gabriel18]
The theoretical results for normalized SAR distribution in the bi-layered bio-media due to the MDH evaluated at 915 MHz using equations provided in the section “Design and theory” are given in Fig. 4.
The simulated variations of input reflection coefficient and peak SAR in the muscle layer of phantom bi-layered bio-media of cross section t 1 × t 1 = 200 × 200 mm2 as functions of fat thickness, and normalized SAR distribution in the bi-layered bio-media for fat thickness (t 2) of 5 mm and muscle thickness (t 3) of 150 mm (for which input reflection coefficient was found to be 0.3077 (=−10.2 dB)) owing to the water-loaded applicator designed at 915 MHz were determined using CST MWS software and the simulation results are shown in Figs 3 and 4, respectively. Initially, 1 W power fed to the antenna was taken in the simulation study. The SAR values are normalized with respect to the maximum value of SAR in the muscle layer. As the SAR value beyond 60 mm in muscle region is negligible at the design frequency of 915 MHz, muscle thickness t 3 = 150 mm taken in simulation study is equivalent to an infinitely thick bio-medium.
Fig. 3. Variations of simulated input reflection coefficient for the MDH and peak SAR inside the muscle layer as functions of fat thickness.
Fig. 4. Normalized SAR distributions inside the bi-layered bio-media due to MDH along (a) z-direction (b) x-/y-direction at z = 10 mm.
It is apparent from Fig. 3 that as the fat thickness increases, the effective relative permittivity of the bi-layered bio-media becomes less, and hence the impedance matching at the interface between the aperture of the MDH and the effective bi-layered bio-media deteriorates, which increases the reflection coefficient at the interface, and hence, the input reflection coefficient also. Peak SAR value in muscle layer reduces as interface reflection is increased with fat thickness.
Figure 4(a) shows the normalized SAR distribution in the inhomogeneous bi-layered media in z-direction due to the MDH. The values of simulated and theoretical penetration depth (depth where SAR value is down to 13.5% of the maximum in the muscle) extracted from Fig. 4(a) are given in Table 2 for the antenna designed at 915 MHz. The normalized SAR distributions along x-/y-direction in the muscle layer due to the MDH designed at 915 MHz are depicted in Fig. 4(b). The values of simulated and theoretical effective field size (EFS), which is defined as the area enclosed within 50% SAR contour inside the phantom bio-medium are extracted from Fig. 4(b) and the results are listed in Table 2. It can be seen from Fig. 4 and Table 2 that the simulated and theoretical results are almost in agreement. Hence, close agreement between theoretical and simulated results proves the validity of plane wave spectral technique which provides theoretical SAR distribution. The little variation in the theoretical and simulated results may be due to the approximations made in the theoretical analysis.
Table 2. SAR parameters inside the muscle medium due to the MDH antenna at 915 MHz (fat thickness t 2 = 5 mm)
a Corresponds to homogeneous muscle medium only.
Figure 5 depicts the results for simulated SAR distribution results in the bi-layered bio-media for different fat thicknesses. From Fig. 5, the following observations can be made: for thin fat layer, the effective dielectric constant of the bi-layered bio-media is higher which results in reduced interface reflection. Since muscle layer forms a major part of the effective bio-medium, SAR in muscle layer is high. But when fat thickness is sufficiently increased, the reflections at the interfaces of the muscle-fat layer and MDH aperture-fat layer become significant, which shifts the focusing spot of SAR from muscle layer to fat layer (Figs 5(d) and 5(e)).
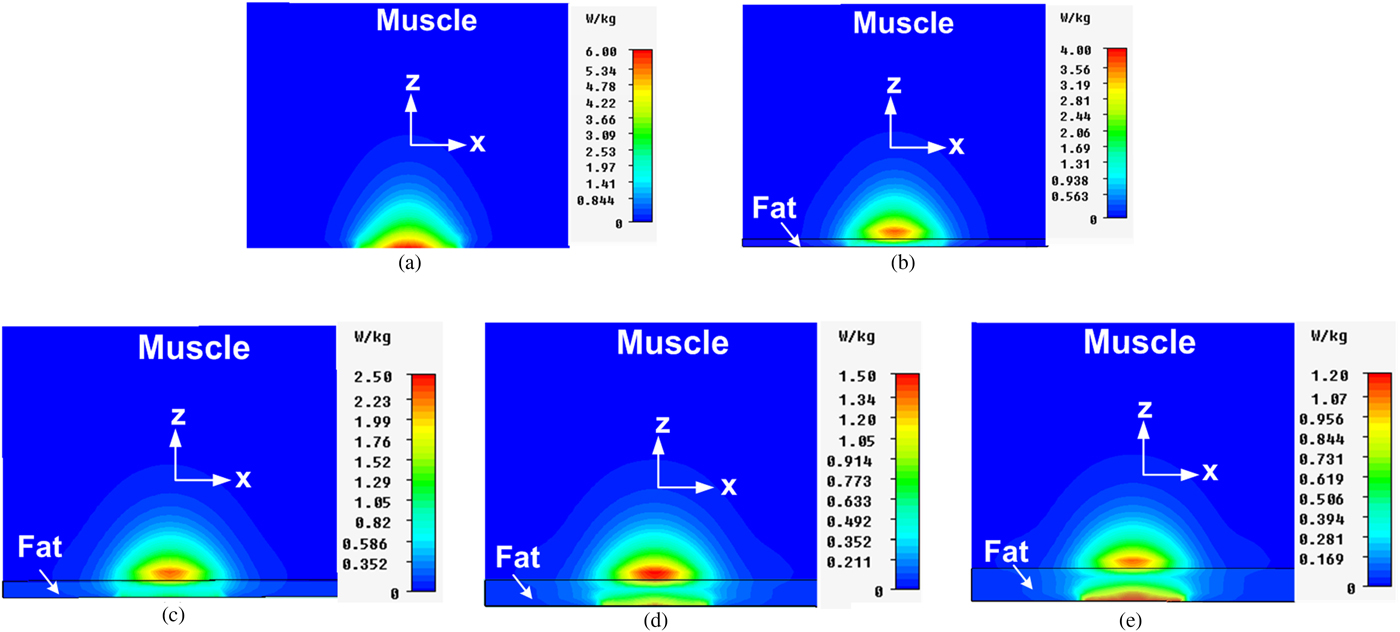
Fig. 5. Simulated SAR distributions inside the bi-layered bio-media due to the MDH for different values of fat thickness (a) t 2 = 0 mm (b) t 2 = 5 mm (c) t 2 = 10 mm (d) t 2 = 15 mm (e) t 2 = 20 mm.
It can be observed from Table 2 that presence of 5 mm thick fat layer reduces the penetration depth to reasonable extent whereas the change in the value of EFS is insignificant. This may be due to the significant reflections at the interfaces of antenna aperture-fat layer and fat-muscle layers because of great differences in the dielectric properties of water and fat layer as well as fat and muscle layers and in the impedances at corresponding interfaces.
Effect of proposed MDH-tissue separation on hyperthermia characteristics
The proposed MDH is in direct contact with inhomogeneous bi-layered bio-media. Direct contact applicators are more desirable as compared with spaced applicators because they reduced leakage (unwanted radiation). In order to study the effect of separation between the proposed MDH and tissue on input reflection coefficient and peak SAR at 915 MHz, simulations were conducted for four values of antenna-tissue separation (0, 1, 3, and 5 mm) and the results are given in Table 3. It is clear from Table 3 that as we increase the antenna-tissue separation, the value of peak SAR in the phantom muscle medium reduces since the coupling between the antenna and phantom reduces. However, the value of input reflection coefficient increases with antenna-tissue separation. As the separation between the antenna and tissue increases, the impedance matching between the antenna and tissue deteriorates since the larger thickness of the medium (free space) having a dielectric constant of 1 is interposed between the two media having much higher dielectric constant as compared with free space medium.
Table 3. Proposed MDH parameters at 915 MHz (fat thickness t 2 = 5 mm)
Polarization effect of the proposed MDH on hyperthermia characteristics
The proposed MDH is a diagonal horn antenna in which the mode of propagation within the horn is principally such that the electric vector is parallel to one of its diagonals when the electric field is excited diagonally, and hence it is referred to as diagonal horn. Hence, the proposed MDH is a diagonally polarized horn antenna. The 3-D view of the proposed MDH is shown in Fig. 1. It can be observed from Fig. 1 that standard rectangular waveguide carrying TE 10 mode is gradually transformed to circular waveguide carrying the TE 11 mode. Another gradual transition converts the circular cross-sections into the square, and the horn then flares out to the desired aperture size. All the cross-sections of the proposed antenna are square including the aperture. It is clear from Fig. 1 that both square waveguide and aperture cross-sections are rotated by 45° with respect to the input rectangular waveguide. The two diagonals of the output opening (of square cross-section) of the circular-to-square transition coincide with the corresponding diagonals of the square waveguide and the antenna aperture. Due to this configuration, the wave which propagates in the slowly flaring proposed horn is composed of two equal amplitude and equi-phase conventional modes (TE 10 and TE 01) appropriate to the square waveguide. The electric field vector distribution at the aperture of the proposed MDH which is in direct contact with inhomogeneous bi-layered bio-media is shown in Fig. 6(a). It can be observed from Fig. 6(a) that distributions of E-fields are along the diagonal at the aperture of the horn. Further, the electric field distribution over the aperture of the proposed horn is identical in E- and H-planes, resulting in circularly symmetric field distribution.
Fig. 6. Electric field distributions at the antenna aperture for different angles of rotation of polarization vector with respect to aperture diagonal (a) 45° (proposed MDH), (b) 30°, (c) 15°, (d) 0° at 915 MHz.
In order to observe the polarization effect of the proposed MDH, the portion of the antenna structure of square cross-section is rotated for different angles with respect to input rectangular waveguide and the results for different rotation angles are given in Table 4. It is clear from Table 4 that as the angle of rotation changes, the polarization vector with respect to its aperture diagonal changes (Fig. 6), due to which the values of peak SAR, EFS and input reflection coefficient are altered (Table 4). Although little changes in the values of input reflection coefficient and peak SAR for different rotation angles are observed, however circularly symmetric EFS in the muscle region due to the proposed MDH is converted into elliptical distribution as the rotation angle for the polarization vector changes with respect to aperture diagonal of the proposed horn. Due to circularly symmetric field distribution, the proposed horn is a better choice for treatment of localized spherical (or near spherical) tumors in superficial region of the body.
Table 4. Effect of rotation angle of polarization vector (with respect to diagonal of antenna aperture) on peak SAR and input reflection coefficient at 915 MHz
SAR distributions in tri-layered bio-media
The performance of the MDH applicator is investigated through simulation for three tri-layered bio-models (one without tumor, and the other two consisting of superficial oval-and irregular-shaped abdominal/limb tumors) lying inside the muscle tissue taken one at a time as shown in Fig. 7. The locations of the oval- and irregular-shaped tumors embedded within second and third tri-layered bio-models are provided in Fig. 8(a). In all, two tumors of different shapes (tumor1 is of oval-shaped and tumor2 is of irregular-shaped) were considered in the present study (Fig. 8(b)).
Fig. 7. Structure of MDH antenna terminated in tri-layered bio-media with embedded tumor.
Fig. 8. (a) Two-dimensional realistic tri-layered bio-model with embedded tumor1/tumor2, and (b) Enlarged view of tumor1/tumor2.
For heating of tumors designated as tumor1 and tumor2, water-loaded MDH antenna designed at 915 MHz which is in direct contact with tri-layered bio-media containing the aforesaid tumors (one at a time) was considered. A cancerous tissue has higher water content [Reference Michaelson and Lin19] compared with healthy tissue, and hence higher dielectric constant and conductivity. The size and dielectric properties of tumors are given in Fig. 8/Table 5.
Table 5. Properties of tri-layered bio-media and tumor [Reference Gabriel18]
The absolute value of peak SAR in the bio-model is dependent on input power, tissue composition and frequency for the given wave polarization and applicator-tissue separation. Figure 9 shows the simulated variations of peak SAR (inside muscle layer) with power fed to the MDH designed at 915 MHz which is in direct contact with a bio-model without tumor by taking the composition of bio-model as a parameter and keeping the wave polarization unchanged. It can be observed from Fig. 9 that as input power increases, peak SAR in the muscle layer increases for both bio-models. But for same input power, the value of peak SAR is smaller for the tri-layered skin-fat-muscle model as compared with the homogeneous bio-model. This effect was observed due to the existence of reflections at various interfaces of the tri-layered bio-model.
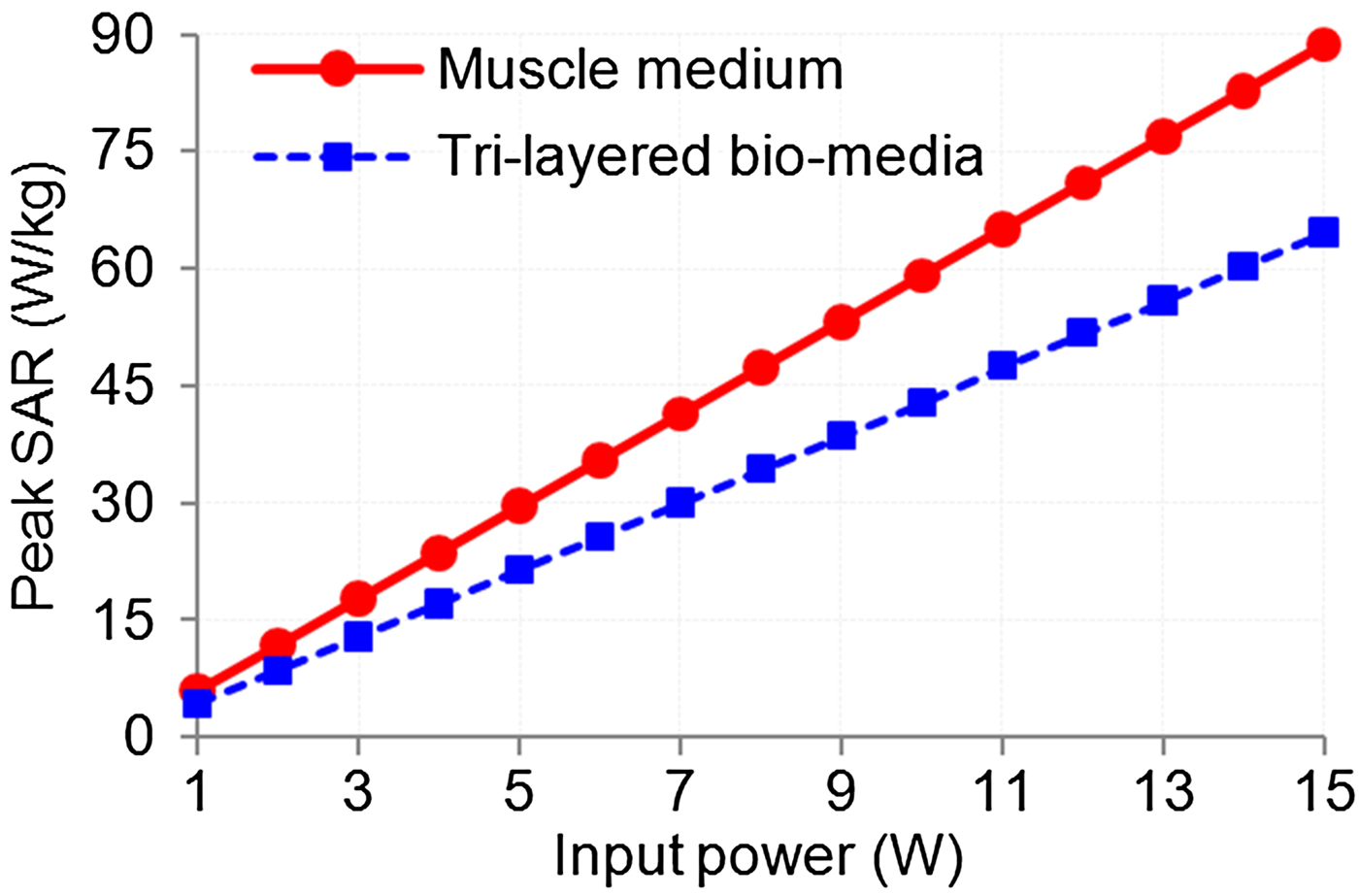
Fig. 9. Variations of peak SAR with input power.
Simulations were also performed for absolute SAR distributions in tri-layered bio-media (skin, fat, and muscle) without and with embedded tumors (taken one at a time) due to the MDH to which 1 W input power was applied and the results are shown in Figs 10 and 11. Figure 10 shows the simulated variations of absolute SAR distribution in the tri-layered bio-media without and with embedded oval-/irregular-shaped tumors (taken one at a time), along z-direction (for x = y = 0) whereas Fig. 11 shows the variations of absolute SAR distributions in the aforesaid configurations of bio-media along x-/y-direction due to the MDH. The values of penetration depth and EFS extracted from Figs 10 and 11 are given in Table 6. It can be inferred from Table 6 that very small changes are observed in penetration depth and EFS (in the middle of tumor) due to the presence of a tumor.
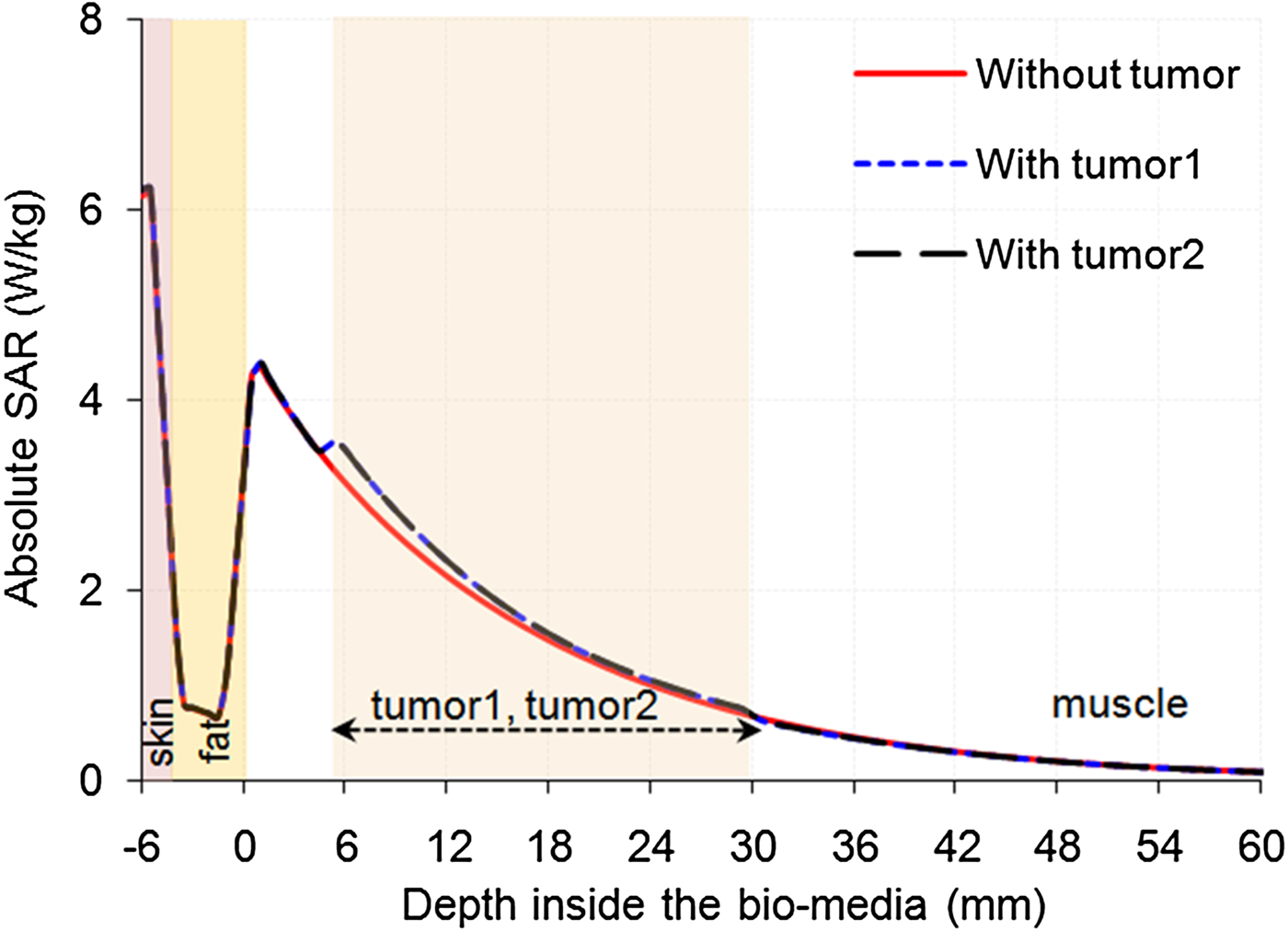
Fig. 10. Simulated absolute SAR distributions inside the tri-layered bio-model without and with embedded tumor along z-direction (x = y = 0) due to the MDH for 1 W input power.
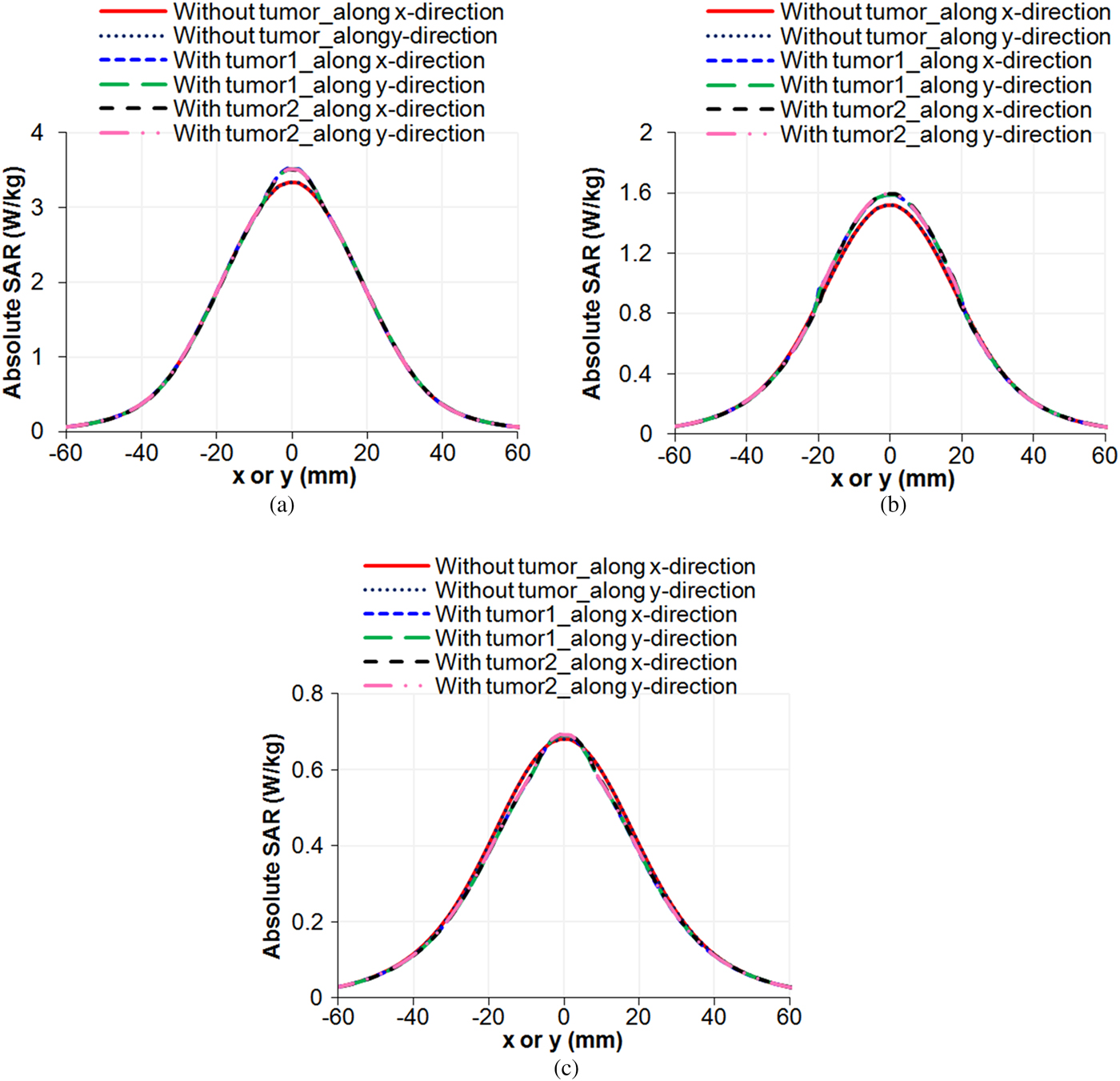
Fig. 11. Simulated absolute SAR distributions inside the tri-layered bio-model without tumor, with embedded tumor1 and with embedded tumor2 along x-/y-direction (y/x = 0) due to the MDH for 1 W input power at (a) z = 5 mm (b) z = 17.5 mm (c) z = 30 mm.
Table 6. Hyperthermia performance due to the proposed water-loaded MDH
a EFS and heating area are defined in the middle of tumor.
Temperature distributions in tri-layered bio-media
The performance of hyperthermia applicator is characterized through temperature distribution in the tissue. The heat generated due to EM energy absorption in the tissue is proportional to SAR. The temperature distribution inside the realistic tri-layered tissue model was evaluated using Pennes’ bio-heat equation (BHE) [Reference Pennes20].
In order to demonstrate the hyperthermia treatment system, inhomogeneous tri-layered bio-models fed through water-loaded MDH designed at 915 MHz was considered. The thermal simulation was carried out using CST multiphysics simulator software at an initial tissue temperature of 37 °C in which convective heat transfer coefficient between tissue and fluid of water-loaded applicator was assumed to be zero. The simulation provides open thermal boundary conditions at a background temperature of 25 °C. The thermal parameters of bio-models used in the simulation are listed in Table 7.
Table 7. Thermal parameters of tri-layered bio-media and tumor1/tumor2 for the BHE [Reference Gong and Wang21, Reference Nikawa22]
Figure 12 shows the variations of temperature as a function of depth in the tri-layered bio-model without tumor due to the MDH designed at 915 MHz by taking input power level as a parameter. It can be seen from Fig. 12 that as power fed to the applicator increases, the temperature in the bio-media rises and decreases exponentially with depth inside the bio-media. It can also be inferred from Fig. 12 that for the present MDH antenna, the input power of 13 W was optimum for obtaining the desired hyperthermia temperature range (41–45 °C).
Fig. 12. Temperature distribution inside the tri-layered bio-media without tumor for different input power levels along z-direction (x = y = 0) due to the MDH.
Figure 13 shows the variations of temperature in the biological media with time owing to the MDH designed at 915 MHz for normal bio-model without tumor and the model with embedded irregular tumor (tumor2) by taking depth in the muscle as a parameter. The power fed to the applicator for each bio-model was taken as 13 W. It is noticed that tumor temperature in each case reaches to a higher value as compared with normal tissue temperature (Fig. 13). This would support in effective heating of tumor in the superficial region of the body compared with normal tissue. Further, it can be observed from Fig. 13 that initial rate of rise of temperature is higher at a lower depth in the realistic tri-layered bio-media without and with embedded tumor2. The rate of rise of temperature in each case slows down after 40 min of heating and reaches a steady value after about 50 min of heating at a given depth. The results demonstrate that tissue temperature approaches saturation after 50 min of heating at a given depth and shows decreasing trend as the depth in the muscle medium increases.
Fig. 13. Variations of temperature in the realistic tri-layered bio-media without and with embedded tumor2 versus time due to the MDH by taking depth in the tumor as a parameter for x = y = 0.
Figure 14 shows the profiles of temperature along z-direction respectively, in the tri-layered bio-media without and with embedded tumor (tumor1/tumor2) due to the water-loaded applicator for an optimum input power of 13 W by taking tumor configuration as a parameter. It is clear from Fig. 14 that temperature profiles are similar for different tumor shapes. Moreover, the applicator provides sufficient heat for the tumor embedded in the bio-model (Fig. 14). Further, the depth at which temperature elevation is half of the maximum with respect to initial temperature (=ΔT/2) (derived from Fig. 14 in each case) is given in Table 6. Further, it is observed from Fig. 14 that the presence of tumor of one configuration or another affects to a certain degree the temperature distribution along the depth at the frequency of interest.
Fig. 14. Simulated temperature distributions inside the tri-layered bio-models without and with embedded tumor1/tumor2 along z-direction (x = y = 0) due to the MDH for 13 W optimum input power at 915 MHz.
Figure 15(a)–15(c) depicts the profiles of temperature distribution in the bio-models without tumor, with embedded tumor1 and embedded tumor2 along x-/y-direction (y/x = 0) at different depths of tumor due to the applicator for optimum input power of 13 W. The results show that the MDH is able to heat whole tumor volume effectively.
Fig. 15. Simulated temperature distributions inside the tri-layered bio-model without tumor, with embedded tumor1 and with embedded tumor2 along x-/y-direction (y/x = 0) at (a) z = 5 mm (b) z = 17.5 mm (c) z = 30 mm due to the MDH at 915 MHz for optimum input power of 13 W.
Figure 16(a)–16(d) shows the cross-sectional profiles of temperature distribution in the bio-models without and with tumor1 in xz/yz plane(s) for (y/x = 0), with embedded tumor1 in xy plane for z = 6, 17.5, and 29 mm, without and with embedded tumor2 in yz/xz plane(s) for (y/x = 0) and with embedded tumor2 in xy-plane for z = 6, 17.5, and 29 mm respectively, owing to the MDH at 915 MHz for 13 W optimized input power. It can be seen from Fig. 16 that desired temperature range (41–45 °C) is maintained in the whole volume of superficial tumors for effective hyperthermia. Symmetrical temperature distributions in the tissue medium due to the applicator can be observed in the transverse xy-plane (Figs 16(b) and 16(d)).
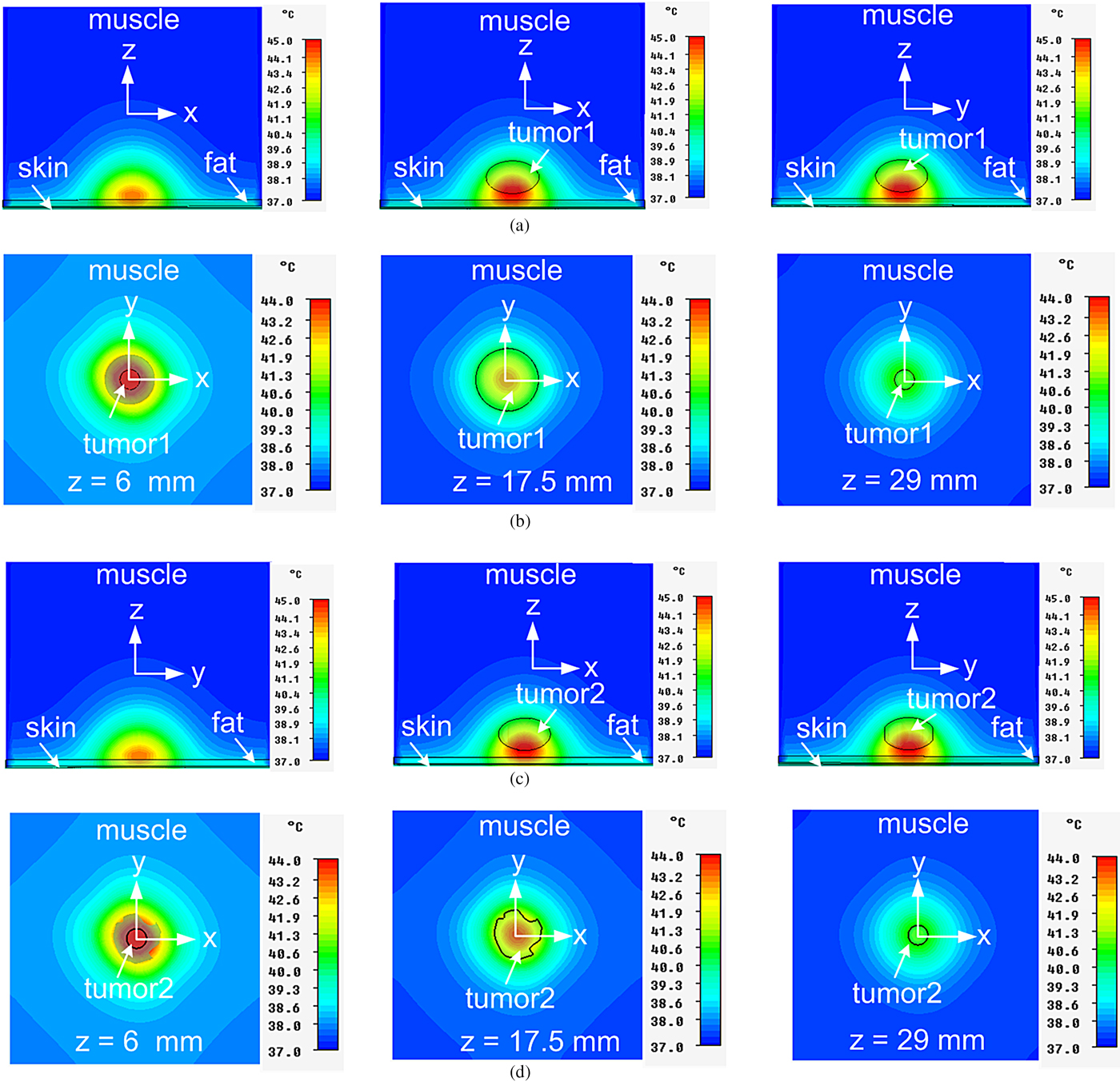
Fig. 16. Temperature distributions inside the realistic tri-layered bio-media due to MDH at 915 MHz for 13 W optimum input power (a) without and with embedded tumor1 in xz/yz planes for (y/x = 0) (b) with embedded tumor1 in xy-plane (c) without and with embedded tumor2 in yz/xz plane(s) for (x/y = 0) (d) with embedded tumor2 in xy-plane.
Conclusion
The hyperthermia performance of water-loaded MDH terminated in bi-layered/tri-layered tissue media has been investigated theoretically and through simulation at 915 MHz. Theoretical and/or simulated SAR and temperature distributions in the phantom bi-layered (fat, muscle) bio-media/realistic tri-layered bio-media due to the MDH at 915 MHz have been determined. Results achieved through theoretical study indicate that reasonably good impedance matching between the MDH aperture and the bi-layered bio-media has been achieved. Further, as fat thickness increases, reflection at the interface of the MDH aperture and bi-layered bio-media increases. The results obtained through simulation study corroborate the annotation that peak value of SAR in the muscle layer decreases and magnitude of input reflection coefficient increases as fat thickness increases. It can be inferred from the simulation study of temperature distributions in the realistic tri-layered bio-models that the water-loaded MDH designed at 915 MHz with 13 W input power can be used for hyperthermia treatment of irregular-/oval-shaped tumors in superficial abdominal/limb region of the body.
Acknowledgement
Soni Singh is thankful to the Ministry of Human Resource Development, Government of India for providing the financial assistance in the form of teaching assistantship.
Soni Singh was born in Deoria, UP, India in 1989. She received the B.Sc. degree in Electronics from DDU Gorakhpur University, India in 2009 and M.Sc. degree in Electronics from Banasthali University, Rajasthan, India in 2011. She completed her Ph.D. work in 2016 from the Department of Electronics Engineering, IIT (BHU), Varanasi, India. She is author or co-author of 11 journal papers and 20 international/national conference papers. Her major research activity includes dielectric loaded metal horn antenna, medical applications of microwaves, and antenna technology.
Bhagirath Sahu received the Bachelor of Engineering (B.E.) degree in Electronics and Communication Engineering from RGPV, Bhopal, India, in 2010, and the M.Tech degree in Electronics Engineering (Microwave Engineering) from Indian Institute of Technology (Banaras Hindu University), Varanasi, India, in 2013. He is currently working towards the Ph.D. degree in Electronics Engineering (Microwave Engineering) from Indian Institute of Technology (Banaras Hindu University), Varanasi, India. He is author or co-author of about 12 Journal papers and 20 international/national conference papers. His research interests include microstrip filters, filtering antennas, dielectric resonator antennas, antennas for wireless/biomedical applications and metamaterials. Mr. Sahu is a student member of the Institute of Electrical and Electronics Engineers (IEEE) Microwave Theory and Techniques Society (MTT-S). He is founder chairperson of IEEE MTT-S Student Branch Chapter (SBC) at IIT (BHU) Varanasi in the Uttar Pradesh (UP) Section, India since May, 2017.
Surya Pal Singh (corresponding author) was born in Mendia, UP, India in 1951. He received the B.Sc. degree in Science, B.Tech. degree in Electronics Engineering, M.Tech. and Ph.D. degrees in Electronics Engineering (Microwave Engineering) from Banaras Hindu University (BHU), Varanasi, India, in 1971, 1976, 1979 and 1989, respectively. He had been employed at Department of Electronics Engineering, IT, BHU (now IIT (BHU)), Varanasi since 1980 and retired as Professor on January 31, 2016. Presently, he is re-employed as Institute Professor in the same institute. He has been Coordinator of the UGC funded CAS program during 2005–2011, and Head of the Department during 2010–2013. He has supervised/co-supervised 12 Ph.D. theses and 64 M.Tech. Dissertation projects. He has authored/co-authored over 190 research papers in international/national journals and conference proceedings. His areas of current research and publications include bio-electromagnetics, microwave antennas, and microwave measurements. Prof. Singh is a Life Senior Member of IEEE, USA and a Life Fellow of IETE, India.
