The humility principle and Fermi's paradox

Humility Principle, also referred as the Copernican or Mediocrity Principle, is the fundamental pillar to the pluralist (optimistical) view according to which there must be an elevated number of technological civilizations coexisting in the Milky Way galaxy (and presumably in other typical galaxies too). The basic idea behind the Humility Principle is that the emergence of life and self-conscious intelligence on Earth must be typical processes happening commonly through the Galaxy, as well as other galaxies in the universe. So, one could expect to find a multitude of inhabited exoplanets and a lot of technological civilizations spread through the cosmos as well. Such an apparently reasonable hypothesis seems quite plausible, but perhaps there is a limit of validity to it. Ellis (Reference Ellis1975, Reference Ellis1978) has argued that Humility Principle should be treated with caution, because it is based on philosophical rather than observational grounds. Moreover, as mentioned by Lineweaver and Davis (Reference Lineweaver and Davis2003), Earth's case can tell little about how common life is in the universe, because even if the probability of biogenesis on a terrestrial exoplanet were infinitesimally small and there were only one life-harbouring planet in the cosmos we would, necessarily, find ourselves on that planet.

On the other hand, Fermi's Paradox is the fundamental stone for singularist (pessimistic) views, according to them we may be possibly alone in Milky Way galaxy, the number of civilizations existing in a typical galaxy being very small. The question ‘Where are they?’ was first proposed by Enrico Fermi in 1950 and derives from the absence of evidence that aliens have visited Earth until now, in spite of our Galaxy being more than 10 Gyr old. If many civilizations existed in Milky Way, at least one should have been successful in colonizing the Galaxy. Such a task would be possible by interstellar flight even at non-relativistic velocities in a timescale of order 10⁶ years, which represents only about 1% of the Galaxy's total age (Jones, Reference Jones1976; von Hoerner, Reference von Hoerner1978). Fermi's Paradox contradicts the Humility Principle, and was first extensively analysed by Hart (Reference Hart1975), who demonstrated that all alternative explanations (physical, sociological or temporal) for the absence of extraterrestrials on Earth now must be rejected. Tipler (Reference Tipler1980) also proposed that extraterrestrial intelligent beings do not exist.

Several authors have criticized Hart's and Tipler's conclusions, beginning with Cox (Reference Cox1976). At the same time, new classes of explanations have been presented (e.g. Wesson, Reference Wesson1990; Teske, Reference Teske1993) but they also indicate a very low number of SCITSs. For reviews of the wide variety of proposed solutions to Fermi's Paradox, see Goldsmith (Reference Goldsmith1980), Zuckermann and Hart (Reference Zuckerman and Hart1995), and Webb (Reference Webb2002). In recent years, Fermi's Paradox has gained force with the discovery of a large variety of extremophile microorganisms on Earth (see, e.g. Pikuta et al., Reference Pikuta, Hoover and Tang2007) and with the discovery of thousands of exoplanets (e.g. Udry and Santos, Reference Udry and Santos2007; Winn and Fabrycky, Reference Winn and Fabrycky2015), combined with the persistent negative results of SETI radio and optical search programs until now (Garcia and Tarter, Reference Garcia and Tarter2019). So, we think that a pluralist view about extraterrestrial intelligence can not be further considered as valid. We take seriously the strong restriction on the number of civilizations in the Galaxy imposed by Fermi's Paradox. It can not be ignored, and we will apply it in the next sections of this paper.

Extraterrestrial intelligence equations

Our aim in this section is briefly to look at several equations that have been proposed in the astrobiological literature in order to calculate the probable number of existing civilizations in our Galaxy. We will comment on Oparin–Fesenkov, Drake, Ward-Brownlee and Grinspoon equations. Rigorously speaking, those equations do not calculate exactly the same thing, as we shall see below.

The Oparin–Fesenkov equation

Oparin and Fesenkov (Reference Oparin and Fesenkov1956) were probably the first scientists to obtain an estimate for the number of planets where life could have appeared in our Galaxy. They took into account factors such as stellar age and stability, the characteristics of planetary orbits, as well as planetary masses and distances (Barcelos, Reference Barcelos1997). The now almost forgotten Oparin–Fesenkov equation may be written in the form:

(1)$$N_{\rm l} = N_{\rm e} \times F_{\rm o} \times F_{\rm e} \times F_{\rm d} \times F_{\rm m}, \;$$

where N _l: the number of planets where life could have emerged; N _e: number of stars not belonging to binary or multiple stellar systems; F _o: fraction of planets with adequate orbits; F _e: fraction of stars with longevity and energy stability; F _d: fraction of stars with planets in suitable orbits; F _m: fraction of stars with adequate mass planets.

They assumed N _e = 3 × 10¹⁰, F _o = 0.1, F _e = 0.1, F _d = 0.1 and F _m = 0.01 so that N _l = 3 × 10⁵ planets. Today we believe that the number of planets with life in our Galaxy may be surely much larger. Perhaps in the Milky Way there could be around 4 × 10¹⁰ exoEarths (Petigura et al., Reference Petigura, Howard and Marcy2013) and the total number of exoplanets may be of order 10¹² (Swift et al., Reference Swift, Johnson, Morton, Creep, Montet, Fabrycky and Muirhead2013).

The Drake's or Green Bank equation

Drake's equation is perhaps the most famous of all astrobiological equations. It was originally proposed in 1961 and combines astrophysical, biological and sociological quantities. The Green Bank equation estimates the number of telecommunicating societies supposed to be present in the Milky Way galaxy today. It may be written as:

(2)$$N_{\rm D} = R_\ast{\times} f_{\rm p} \times n_{\rm e} \times f_{\rm l} \times f_{\rm i} \times f_{\rm c} \times L, \;$$

where N _D: number of civilizations with interstellar communication capability; R_*: mean star formation rate in the Galaxy; f _p: fraction of stars with planets; n _e: number of Earth-like planets per star; f _l: fraction of Earth-like planets where life emerges; f _i: fraction of Earth-like planets where intelligence appears; f _c: fraction of civilizations with interstellar communication capability; L: mean life time for a communicating civilization phase.

A recent review of Drake's equation may be found in Frank and Sullivan (Reference Frank and Sullivan2016). Note that N _D is not the total number of civilizations N probably existing in the Galaxy, but only that fraction of it which develops interstellar communication capability. Original values for factors in Drake's equation were R_* = 1 star yr⁻¹, f _p = 0.2–0.5, n _e = 1–5; f _l = 1, f _i = 1 and f _c = 0.1–0.2. It was assumed that f _l ≈ f _i ≈ 1, that is, if life appears then intelligence will be a corollary sooner or later. The most controversial and difficult factor to determine is L. Values ranging from 10³ to 10⁸ yr were considered. Calculation using the minor values yields N _D = 20. Using the major values, we get N _D = 50 × 10⁶. It is easy to see that N _D must be of order of L, that is, N _D ≈ L, so that there would be about 10³–10⁸ civilizations with interstellar communication capability in the Galaxy. These numbers strongly violate Fermi's Paradox restriction, and even do not agree with other modern observational limits.

Today several Drake's equation factors are better known than in the 1960s. Modern values for factors in Drake's equation could be as follows: R_* = 7 stars yr⁻¹ (then we adopt 10), see e.g. Diehl et al. (Reference Diehl, Halloin, Kretschner, Giselher, Volker, Strong, von Kienlin, Wang, Jean, Knödlseder, Roques, Weidenspointner, Schanne, Hartmann, Winkler and Wunderer2006), f _p = 1 [exoplanets; Swift et al. (Reference Swift, Johnson, Morton, Creep, Montet, Fabrycky and Muirhead2013) estimate 1.0 ± 0.1 planet per star], n _e = 1 to about 10 (based on known exoplanetary systems; here we conservatively will adopt 1), and f _l = 1 (from extremophile organisms discoveries from 1977 on). Currently, only f _i, f _c and L remain considerably uncertain. We will return to this point later.

The Ward–Brownlee or Rare-Earth equation

Obviously Oparin–Fesenkov's nor Drake's equations consider all relevant factors needed to get a precise estimate of the quantity of civilizations probably existing in our Galaxy. In order to correct such a situation, Ward and Brownlee (Reference Ward and Brownlee2000) have proposed a new equation, including additional factors. They consider N _D from Drake's equation as the total number of civilizations in the Galaxy, but we have mentioned earlier that this is not probably the case. Ward–Brownlee equation may be written as follows:

(3)$$N_{{\rm RE}} = N\ast \times f_{\rm p} \times f_{{\rm mp}} \times n_{\rm e} \times n_{\rm g} \times f_{\rm i} \times f_{\rm c} \times f_{\rm l} \times f_{\rm m} \times f_{\rm j} \times f_{{\rm me}}, \;$$

where N _RE: ‘number of civilizations’; N*: number of stars in the Milky Way; f _p: fraction of stars with planets; f _mp: fraction of metal-rich planets; n _e: number of planets in the circumstellar habitable zone (CHZ) around a star; n _g: number of stars in the galactic habitable zone (GHZ); f _i: fraction of habitable planets where life originates; f _c: fraction of habitable planets which develops complex life forms; f _l: percentage of a planet's life time during which complex life forms exist; f _m: fraction of planets with a big natural satellite; f _j: fraction of planetary systems with Jovian-like planets; f _me: fraction of planets with a critically low frequency of biological mass extinctions.

The CHZ is generally defined as the region around a star where liquid water can exist on the surface of an Earth-like planet during a long period of time (see Huang, Reference Huang1959; Shklovsky and Sagan, Reference Shklovsky and Sagan1966; Hart, Reference Hart1979). The GHZ concept has been introduced by Gonzalez et al. (Reference Gonzalez, Brownlee and Ward2001) although Marochnik and Mukhin (Reference Marochnik, Mukhin, Ambartsumyan, Kardashev and Troitskii1986) already mentioned it. It is defined as the Milky Way region where an Earth-like planet can retain liquid water on its surface and provide a long-term habitat for animal-like aerobic life. This region would have the form of an annulus inserted in the galactic disk plane, and its limits would be set by several galactic-scale astrophysical processes likely to be related to the habitability of Earth-like planets. Its location would be time-dependent due to the increasing metallicity of interstellar medium (ISM). The inner limit would be set by high-energy events such as frequent supernovae explosions and gamma ray bursts (GRBs), the higher metallicity derived from them leading to orbital decay of planets as well as severe gravitational perturbations of Oort cloud comets, contributing to an increase of comet impacts frequency, which cause biological mass extinctions and complex life major perturbations. The outer GHZ limit would be imposed by the ISM chemical evolution, in particular by the thin galactic disk radial metallicity gradient (−0.07 dex kpc⁻¹ according to Rolleston et al., Reference Rolleston, Smartt, Dufton and Ryans2000). The GHZ would be placed in the Milky Way's thin disk, slowly migrating outward with time as the ISM metallicity increases.

It is very interesting to observe that a very similar concept to GHZ can also be derived not from chemical abundances arguments but from purely dynamical considerations. Spiral arms can only exist between the so-called Lindblad's ressonances. In the Milky Way case, the internal Lindblad ressonance radius is 2.5 kpc, while the external ressonance radius is 11 kpc from the galactic nucleus. Between these two radii, we have the co-rotation radius, that is, the distance from the galactic centre where the frequency of stellar galactocentric revolution equals the spiral arms rotation frequency. Star-forming rates as well as supernovae explosion rates would tend to be minimal within that region, and not surprisingly our Sun inhabits this galactic region! Lépine (Reference Lépine2008) refers to this zone as the ‘Galaxy's Green Belt’.

Ward and Brownlee emphasize that the quantity of terms in their equation is still far from complete, and that many factors included are pooly known. Obviously, the more factors are added, the more uncertain will be the final calculation result, and more probably N _RE will tend to low values. But they consider that, even by now, Rare Earth equation status is such that it permits to affirm that the most probable value for N _RE is exceedingly small. So the Ward–Brownlee equation would explain Fermi's Paradox while disagreeing the Humility Principle, favouring a highly pessimistic view concerning the existence of self-conscious intelligent beings in the universe.

The Grinspoon's equation

Grinspoon (Reference Grinspoon2003) suggests an equation to estimate the formation rate of what he calls ‘immortal civilizations’, that is, those ‘truly intelligent’ and really evolved technological societies. He considers a civilization like our own as a ‘proto-intelligent’ culture. Naturally those highly evolved civilizations would represent a possibly small fraction of the total number of civilizations residing in the Galaxy. Grinspoon's equation may be written as follows:

(4)$$V_{{\rm IC}} = R_\ast \times f_{\rm p} \times n_{\rm e} \times f_{\rm l} \times f_{\rm i} \times f_{{\rm IC}}, \;$$

where we have V _IC: formation rate of ‘immortal civilizations’; R_*: star formation rate in the Galaxy; f _p: fraction of stars with planets; n _e: number of habitable planets per star; f _l: fraction of habitable planets where life originates; f _i: fraction of planets with life where intelligence appears; f _IC: fraction of civilizations successful in their transition to ‘immortality’.

The first five factors in the right side of equation (4) are identical to the corresponding factors in Drake's equation (equation (2)). Assuming f _IC = 0.001 Grinspoon concludes that a new ‘immortal’ civilization is born each 2 × 10³ yr somewhere in the Galaxy. If f _IC = 0.00001, the rate would be 5 × 10⁻⁶ civ yr⁻¹. He argues that ‘immortal’ civilizations formation rate could be of order 1 civ Myr⁻¹ so that, in a Galaxy 10¹⁰ yr old, we would have something between 10⁴ and 5 × 10⁶ highly evolved technological societies. All of those numbers strongly violate Fermi's Paradox, constituting a vigorous defense of the Humility Principle and the optimistic view about the chances of self-conscious intelligence emerging in the cosmos.

Is intelligence really an evolutionary advantage?

This is an absolutely fundamental question for SETI. Sagan (Reference Sagan1977) pointed out that in general, intelligent beings survive better and produce more offspring than stupid ones. According to him, ‘smarter is better’. But this is not sure. We remark that, even here on Earth, we have millions of species of complex life forms which never developed neuronal cells: the Plantae kingdom.

Mayr (Reference Mayr1994, Reference Mayr2004) affirms that about 10⁹ live species have appeared on Earth since life beginning about 3.5–3.8 Gyr ago. If Sagan was correct, we would hope to find millions of highly intelligent species. But this is not the case. Photoreceptor structures (eyes) and bioluminescence have both high adaptive value and independently evolved many times in the Animalia kingdom. His conclusion is that high-level intelligence evolution is not deterministic in the Darwinian evolution context, so contradicting the Humility Principle. Many biologists think like Mayr (see, e.g. Ornstein, Reference Ornstein1982).

This important question is still far from be elucidated. As we shall see later in this paper, additionally one must distinguish between intelligence and technological civilizations. The first one may be much more abundant than the last in the universe.

An alternative intelligence equation

In this section, we propose another equation to estimate the probable quantity of SCITSs in the observable universe. We begin with HUDF – The Hubble Ultra Deep Field (Beckwith et al., Reference Beckwith, Steven, Stiavelli, Koekemoer, Caldwell, Ferguson, Hook, Lucas, Bergeron, Corbin, Jogee, Panagia, Robbert, Royle, Somerville and Sosey2006). From a recent new HUDF analysis, Conselice et al. (Reference Conselice, Wilkinson, Duncan and Mortlock2016) estimated the total number of galaxies within the radius of the observable universe as (1.4–2.7) × 10¹². Let us adopt 2 × 10¹² galaxies. From this total, we exclude elliptical, lenticular, irregular, peculiar, interacting and those with active galactic nuclei galaxies, since they probably have little astrobiological importance concerning extraterrestrial high-level intelligence. This is due to several reasons, such as supernovae frequency, star-forming rates, low ISM metallicity, dominant stellar populations, etc.

Galaxy morphologies show an environmental dependence (e.g. Deng et al., Reference Deng, He and Wen2009). Early-type galaxies tend to reside in the densest regions of the universe such as clusters of galaxies cores, while late-type ones are more common in low-density regions. There is also time dependence, that is, a relation with the age of the cosmos. The number of spiral galaxies has increased by a factor of 2.3 during the past 6 Gyr (Delgado-Serrano et al., Reference Delgado-Serrano, Hammer, Yang, Puech, Flores and Rodrigues2010). For a sample of nearby galaxies, they have found that spiral galaxies represent 72% of the total population of galaxies, confirming the results by Hammer et al. (Reference Hammer, Flores, Elbaz, Zheng, Liang and Cesarsky2005). Here we will adopt 80% as the percentage of spiral galaxies.

How many stars do we find in a typical spiral galaxy? It is generally mentioned that the Milky Way has (100–400) × 10⁹ stars, although the most common stellar type, red dwarfs, are difficult to detect. Considering that our Galaxy may harbour (400 ± 200) × 10⁹ stars, we adopt 10¹¹ stars.

The next step is to know how many solar-like stars exist in a typical spiral galaxy. A fraction around 10% is commonly to be found, and here we will conservatively assume 0.05.

Now we can calculate the approximate number of sun-like stars in all spiral galaxies within the radius of the observable universe:

$$2 \times 10^{12} \times 0.8 \times 10^{11} \times 0.05 = 8 \times 10^{21}\,{\rm sun}-{\rm like}\,{\rm stars}.$$

We are considering only sun-like type stars, excluding red dwarfs based on the common occurrence of UV flares in those stars. Red dwarfs may have Earth-like planets orbiting within the limits of their narrow CHZs as shown by Proxima Centauri (Anglada-Escudé et al., Reference Anglada-Escudé, Amado P, Barnes, Berdiñas, Butler, Coleman, de La Cueva, Dreizler, Endl, Giesers, Jeffers, Jenkins, Jones, Kiraga, Kürster, López-González M, Marvin, Morales, Morin, Nelson, Ortiz, Ofir, Paardekooper, Reiners, Rodríguez, Rodríguez-López, Sarmiento, Strachan, Tsapras, Tuomi and Zechmeister2016) and TRAPPIST-1 (Gillon et al., Reference Gillon, Jehin, Lederer, Delrez, de Wit, Burdanov, van Grootel, Burgasser, Triaud, Opiton, Demory, Sahu, Gagliuffi, Magain and Queloz2016) but, although this fact probably does not hampers the existence of primitive simple life forms, it certainly does not favour the evolution of complex high-level intelligent live beings.

What fraction of this sample of 8 × 10²¹ solar-like stars will harbour SCITSs? Surely not all of them. But probably how many? Let us define a new factor, P _c, the SCITS's formation probability. We then write:

(5)$${N}^{\prime} = 8 \times 10^{21}.P_{\rm c}, \;$$

where N′ is the probable total number of SCITSs in the observable universe. The mean number of civilizations per spiral galaxy N is, of course:

(6)$$N = 6.25 \times 10^{{-}13} \times {N}^{\prime}.$$

Applying the restriction imposed by Fermi's Paradox (N ≈ 1) we obtain:

(7)$$P_{\rm c} = 2 \times 10^{{-}10}.$$

We see that one can write:

(8)$${N}^{\prime} = n_{\rm G} \times f_{{\rm SG}} \times n_{\rm S} \times f_\odot{\times} P_{\rm c}, \;$$

where N′: probable total number of SCITSs in the observable universe; n _G: estimated total number of galaxies in the observable universe; f _SG: fraction of spiral galaxies; n _S: total number of stars in a typical spiral galaxy; f _ʘ: fraction of solar-like stars in a typical spiral galaxy; P _c: SCITS's formation probability factor.

Note that equation (8) eliminates the main problem inherent to all equations we have briefly revised in this paper, because all relevant parameters – either known or unknown – that can influence N’ or N are encapsulated in a single factor, P _c. Equation (8) contains just five terms, four of them being astrophysical in nature and all are reasonably well known. The only troublesome factor is P _c but, even so, it may be calculated with the help of Fermi's Paradox restriction, its value resulting known with only an uncertainty by a factor of 10², as we shall see in the sequence.

For P _c values ≤10⁻⁹–10⁻¹⁰, Fermi's Paradox is not violated. Indeed, these values correspond to an upper limit for P _c. It is interesting to note that Frank and Sullivan (Reference Frank and Sullivan2016) did find what could be interpreted as a lower limit for P _c: ≈10⁻²⁴.

As P _c is very small we can conclude that the formation of SCITSs is an extremely improbable event in the universe. This strongly points to the fact that intelligence is not a Darwinian evolutionary advantage.

Our estimate for P _c is also compatible with biological evidences. In the Earth's case, we have several intelligent species such as whales, dolphins, apes and the Homo sapiens, among several others, but only one – we – constructed a technological civilization. Mayr (Reference Mayr1993, Reference Mayr2004) cites the total number of species that appeared on Earth since life began as (1–50) × 10⁹. Inverting this quantity, we get 10⁻⁹ to 10⁻¹⁰, our estimated value for P _c. Additional evidence comes from KEPLER mission results, which have permitted to estimate as about 40 × 10⁹ the probable number of exoEarths in CHZs in our Galaxy (Petigura et al., Reference Petigura, Howard and Marcy2013). Again, if we are the only civilization in the Galaxy, we have (40 × 10⁹)⁻¹≈10⁻¹¹, roughly compatible with our P _c value. Also Tipler (Reference Tipler1980) has concluded that ‘the probability of the evolution of creatures with the technological capability of interstellar communication within five billion years after the development of life on an Earth-like planet is less than 10⁻¹⁰’.

In addition to P _c value known within an uncertainty by a factor of 10², we can also observe that some factors in equation (8) would admit slightly different values. For example, probably a better estimate of the real number of stars in a typical spiral galaxy could be of order of 10¹², not 10¹¹. In fact, this seems to be the case in some nearby spiral galaxies, such as NGC 224 (M 31, the Andromeda Galaxy). We then would have about 10 civilizations per spiral galaxy.

But it is necessary to distribute these few civilizations through time. Although Milky Way formation has begun over 13 Gyr ago and the age of the galactic halo is about 11.4 Gyr, the galactic component of astrobiological interest, namely, the galactic disk, is more recent. An estimate for the age of thick disk is 10 Gyr (Sharma et al., Reference Sharma, Stello, Bland-Hawthorn, Hayden, Zinn, Kallinger, Hon, Asplund, Buder, Silva, D'Orazi, Freeman, Kos, Lewis, Lin, Lind, Martell, Simpson, Wittenmyer, Zucker, Zwitter, Bedding, Chen, Čotar, Esdaile, Horner, Huber, Kafle, Khanna, Li, Ting, Nataf, Nordlander, Saadon, Traven, Wright and Wyse2019). The thick disk is older than the thin disk, and less metal-rich too. We will consider a 10 Gyr old galactic disk. If we distribute ten civilizations through a 10 Gyr timelapse, we obtain a rough estimate of the civilizations formation rate, probably also valid for other typical spiral galaxies: ≈1 civ Gyr⁻¹.

The next question is: what is the mean lifespan for a civilization? Characteristic time lifes for biological species are usually considered to be around 10⁶–10⁷ years. None of the Cambrian (about 0.5 Gyr ago) animal species exist today (Margulis and Sagan, Reference Margulis and Sagan1998). Ward and Brownlee (Reference Ward and Brownlee2000) note that about 3/4 of all live species extinguish every 10⁸ years. During the Cenozoic Era, the mean lifespan of Mammalia class species was always less than 3 Myr. Blair et al. (Reference Blair, Norris, Troup, Twardy, Wellington, Williams, Wright and Zadnik1992) have deduced an upper limit of 10⁸ yr for the civilizations lifetime. Let us be optimistic and assume this number. If so, we can immediately conclude that we are alone, because if the mean rate of SCITS's formation, dN'/dt ≈ 1 civ Gyr⁻¹ and the maximum duration of a civilization is about 0.1 Gyr, then in a typical spiral galaxy two or more civilizations never will coexist. Of course the civilization formation rate will be a function of the ISM metallicity, slowly increasing as the Galaxy becomes older and more metal-rich, but these changes much probably will not alter significantly the above conclusion.

The timescale to develop self-conscious intelligence

It has been generally presumed (e.g. Westby and Conselice, Reference Westby and Conselice2020) that time needed to develop high-level self-conscious intelligence is of the order 5 Gyr, based on what happened here on Earth. However, we remark that, during about 80% of all time life exists on our planet, there were practically only unicellular or very simple multicellular organisms. The great life diversification event occurred about 0.5 Gyr ago (the Cambrian explosion). From that moment until now, life evolved from microbes to self-conscious intelligent beings. Thus, all one can say, by applying the Humility Principle, is that we know the time needed to self-conscious intelligence appearance within a factor of ten (0.5–5 Gyr). We call these two extreme time intervals, the ‘short’ scale and the ‘long’ scale, respectively.

Basically considering the Milky Way history, surely there was an initial period during which the ISM metallicity was too low in order to permit the formation of Earth-like planets, as well as life forms. We refer to this as the Azoic Galactic Period (AGP). It starts about 12 Gyr ago with the formation of the galactic spherical component (halo), characterized by ISM metal content Z ≈ 0.01–0.02 Z _ʘ, and ends about 10 Gyr ago, when the metallicity of galactic thin disk reaches the critical value Z ≥ 0.1 Z _ʘ (Olejak et al., Reference Olejak, Belczynski, Bulik and Sobolewska2020). This metal-enrichment level is the minimum needed in order to allow Earth-like planets formation (Johnson and Li, Reference Johnson and Li2012). The Galactic thick disk probably has little astrobiological interest because it typically has significantly lower metallicity than the thin disk (Freeman, Reference Freeman, Block, Freeman and Puerari2010). It was formed between 9 and 11 Gyr ago.

Following AGP, we have an era during which only primitive live organisms exist. The duration of this era may have been from 0.5 to 5 Gyr. If one assumes the short timescale, the first SCITS in the Galaxy would have appeared about 9.5 Gyr ago. In the long timescale, that event was about 5 Gyr ago. Therefore, the Intelligence Galactic Era (IGE) would extend from 9.5 to 5 Gyr ago until now. Figures 1 and 2 show schematically both timescales. Between the short and long life timescales scenarios, obviously there is a multitude of possible intermediary situations. The intelligence evolution could require a different time in each planet where it appears but probably the short and long timescales represent the extreme limits. It seems difficult to imagine that high-level self-conscious intelligence could evolve from microbes in less than about 0.5 Gyr. On the other hand, for a planet orbiting a star like our Sun, intelligence could not demand more than about 6 Gyr to evolve, because we know that in about 1.1 Gyr from now all life on Earth will be destroyed due to a 10% natural increase in solar luminosity (Sackmann et al., Reference Sackmann, Boothroyd and Kraemer1993) which will cause a moist greenhouse global catastrophe, according to a cloud-free climate model of the Earth calculated by Kasting (Reference Kasting1988). However, the exact choice of intelligent life timescales does not significantly affect the general situation concerning the probable small number of SCITSs in the Galaxy now, that is, the pessimistic scenario always prevails.

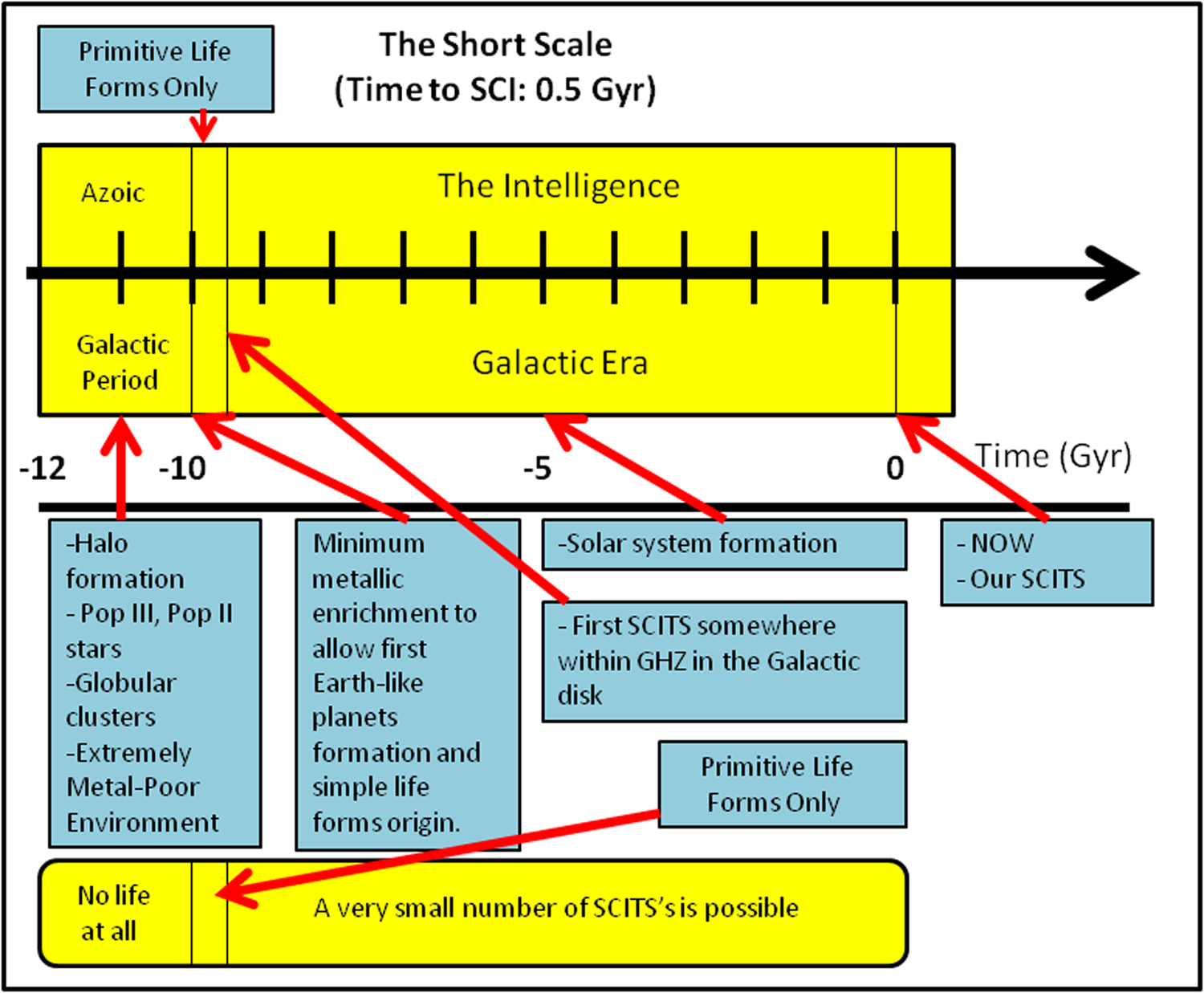
Figure 1. A possible timeline for self-conscious intelligent life in the Galaxy (short scale, see text).

Figure 2. A possible timeline for self-conscious intelligent life in the Galaxy (long scale, see text).

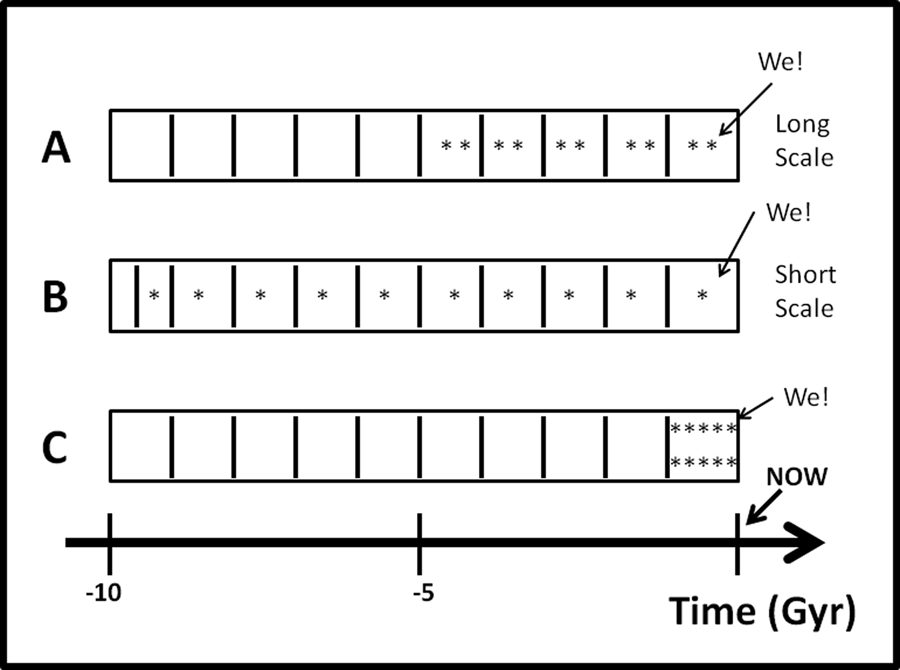
In order to be convinced of this, look at Figure 3, where we represent three different time distributions for SCITS's evolution in our Galaxy. Scenario A shows ten civilizations equally distributed over time for the long scale described in the text. Scenario B is the same, but for the short scale. In scenario C, we consider that ten civilizations evolved more or less simultaneously during the last 1 Gyr.

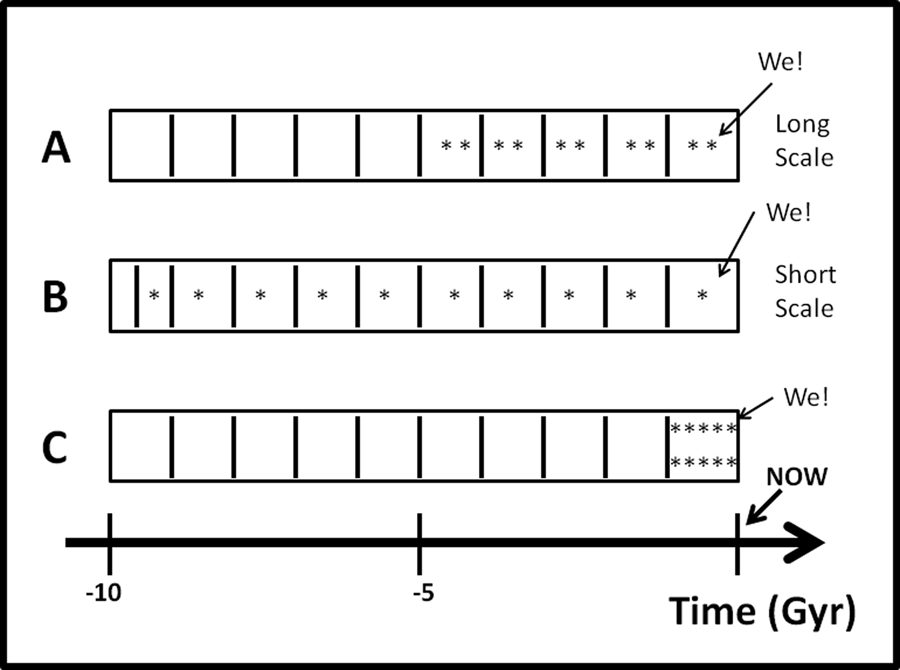
Figure 3. Three speculative scenarios for time distribution of SCITSs in the Milky Way Intelligence Galactic Era (IGE).

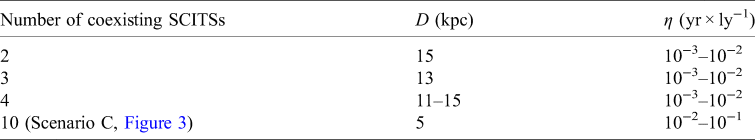
Table 1 shows the mean distances between SCITSs for different numbers of coexisting civilizations, assuming a homogeneous distribution in the galactic disk and all of them appearing near the co-rotation radius (7.5 kpc) in the middle of GHZ.

Table 1. Mean distances and contactability factor

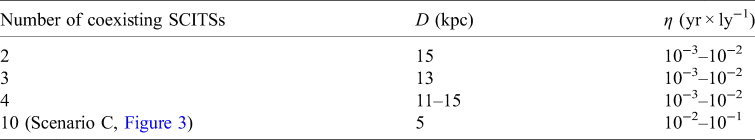
Mean distances D and contactability factor η calculated for L = 10² yr and L = 10³ yr, for different numbers of coexisting SCITSs homogeneously distributed near the co-rotation radius (7.5 kpc) in the Milky Way GHZ.

Quillfeldt (Reference Quillfeldt, Galante, Silva, Rodrigues, Horvath and Avellar2016) introduced the contactability factor η, as a measuring of positive contact chance among SCITSs, defined as:

(9)$$\eta \equiv \displaystyle{L \over D}, \;$$

where L is the duration of the communicating phase of a civilization measured in years, and D is the mean distance between two civilizations measured in light-years. If η >> 1, contact chances would be larger. In Table 1, we have also calculated η for L = 10² yr and L = 10³ yr. We see that η is always <<1 regardless the adopted scenario.

SCITSs classifications

In this section, we briefly review Kardashev's, Tang and Chang's and Grinspoon's classifications for extraterrestrial civilizations proposed in the literature.

SCITS's classification: an alternative proposal

The SCITS's classification schemes discussed in the previous section consider very restricted aspects, such as energy consumption rates or energy sources, and therefore say little about the possible evolution of a SCITS. We suggest here an alternative general and possibly more fundamental classification scheme for SCITSs. Let's distinguish five basic types:

• P – Primitive societies;

• Y – Young societies;

• A – Adult societies;

• S – Senior societies (?);

• D – Degenerate societies.

Degenerate societies

This group would include decaying civilizations experiencing a wiping out process caused by a large diversity of reasons: failure at transition from young stage to adult phase, annihilation by natural catastrophes (e.g. by collision of an asteroid or comet with the home planet), or astrophysical events (e.g. a supernova explosion or GRB occurring near their star). This class could also include those societies that have disintegrated as a consequence of a cultural shock with an alien culture (although, in our opinion, this possibility may be practically non-existent). Although one can expect to find a great diversity of degenerated civilizations, probably the most common candidates would be primitive or young societies whose evolution has been aborted.

Table 2 shows the probable correspondences among the different SCITS's classifications we have discussed in this paper.

Table 2. SCITSs classifications

(* ‘proto-intelligent’ cultures; + ‘truly’ intelligent cultures; ? doubtfull correspondence).

Correspondence among the different SCITS's classification schemes discussed in the text.

The five civilization groups defined in this section may suggest a natural sequence of evolution. However, this could not be always the case.

Even in those societies that do overcome the primitive stage, there is the risk of social stagnation, or even of evolutionary status regression. Such risks may be higher for primitive or young societies, perhaps smaller to adult or senior cultures.

Of course, theoretically, a civilization could skip stages, mainly in the case of a positive contact with an alien culture belonging to a more advanced group. However, such contacts could also result in stagnation, regression or even disintegration of the lower culture, configuring a destructive cultural shock.

On the other hand, the chances of a successful contact between two civilizations belonging to very different groups could be very small, and the subsequent utility of such an event doubtful. As an example, consider a hypothetical contact of a young society with a senior culture: besides very unlikely, it could even not be perceived and understood at all. The most useful intersocial contacts would tend to occur between adult civilizations, or between senior societies, or still, with less probability, between an adult civilization and a senior culture. We also mention that, given the probable very short life expectancy for young civilizations, indirect radio or optical contact chances between two young cultures would be negligible.

The relatively high step separating a typical young society from a consolidated adult alien culture could perhaps help to explain Fermi's Paradox, based on the extreme cultural difficulty (not to say impossibility) in recognizing the existence of the most advanced civilization.

Our own civilization's current status corresponds to 0.8 in Kardashev's classification, Tang and Chang's 3, Grinspoon's 1, and Y in the scheme proposed in this section. For short: 0.8/3/1/Y.

Evolutionary paths for SCITSs

The astrosociological classification presented in the previous section is useful in order to visualize and to understand what may probably happen to the SCITS's life and evolution. While primitive stage may last for, perhaps, 10⁴–10⁵ years, the young phase is probably short (10²–10³ years) and intense, rapidly conducting to a critical situation which we will call ‘the bottle neck’. This is happening with human civilization on Earth right now. Adult and senior societies may persist for 10⁴–10⁸ years or, perhaps, even more, but the most common types of SCITSs in a typical spiral galaxy probably are primitive and/or young cultures. Adult and senior civilizations may represent a very low percentage (about 1–10%) of all existing SCITSs, if they ever exist at all. We note that if adult and/or senior societies do not exist, then there would be no interstellar travellers!

Figure 4 shows schematically the possible evolutionary alternatives available to SCITs in the classification framework discussed in the present section.

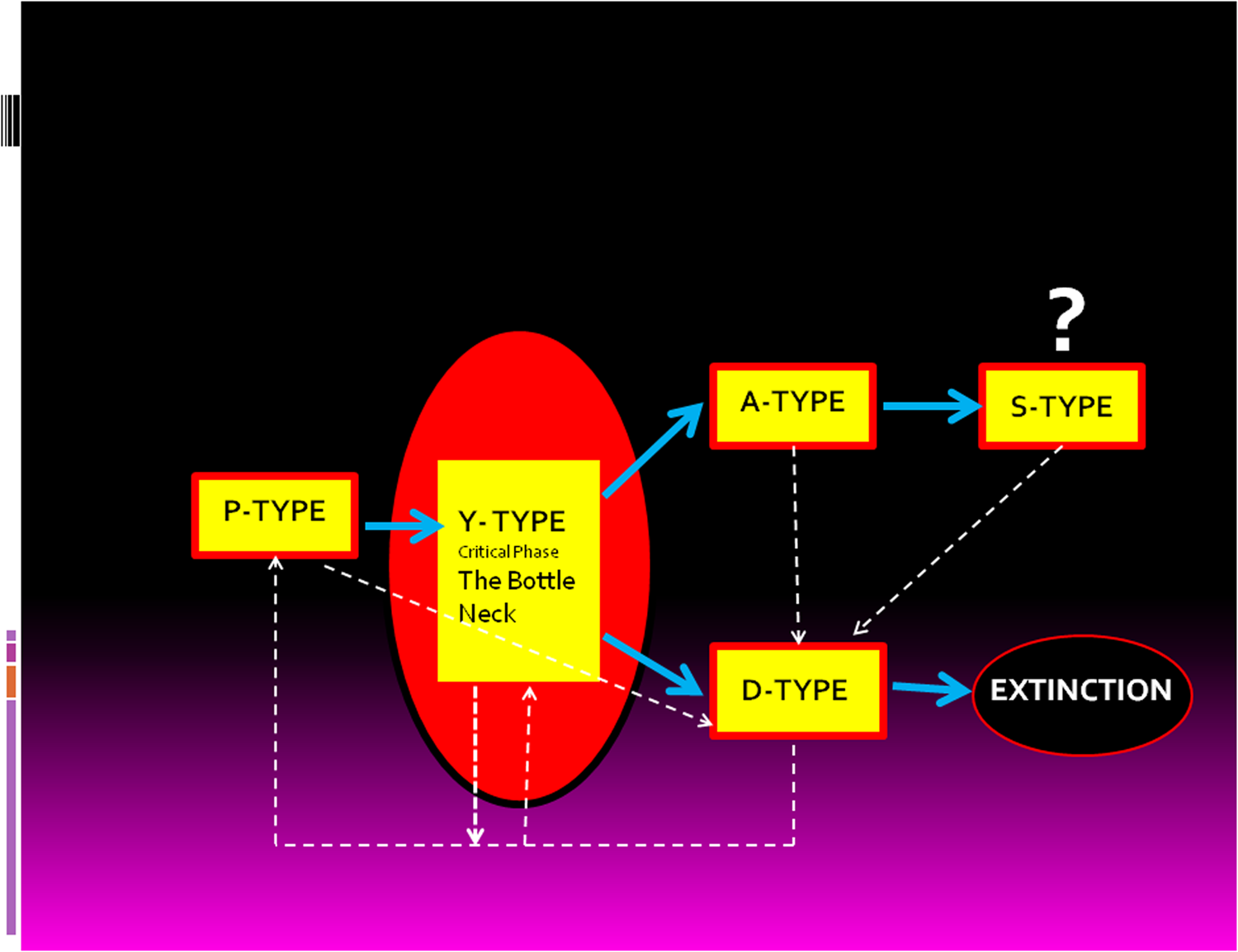
Figure 4. Probable life cycles for self-conscious intelligent technological societies in the universe.

As already mentioned, we suspect that young phase survival probability is strongly dependent on the equilibrium between technological development and what we call ‘ethical’ development of a society. Such an opinion is also sustained by Grinspoon (Reference Grinspoon2003) and Hawking (Reference Hawking2018), among others. Grinspoon (Reference Grinspoon2003) uses the expression ‘spiritual development’ as a synonym for ethical development.

We now introduce rather freely the ethical-technological ratio, R, defined as:

(10)$$R\equiv \displaystyle{{{\rm level}\,{\rm of}\,{\rm ethical}\,{\rm advancement}} \over {{\rm level}\,{\rm of}\,{\rm technological}\,{\rm advancement}}}.$$

This ‘quantity’, although highly subjective, helps to clarify the possible evolutionary paths available to a young society, once it is experiencing its critical phase (Figure 5).

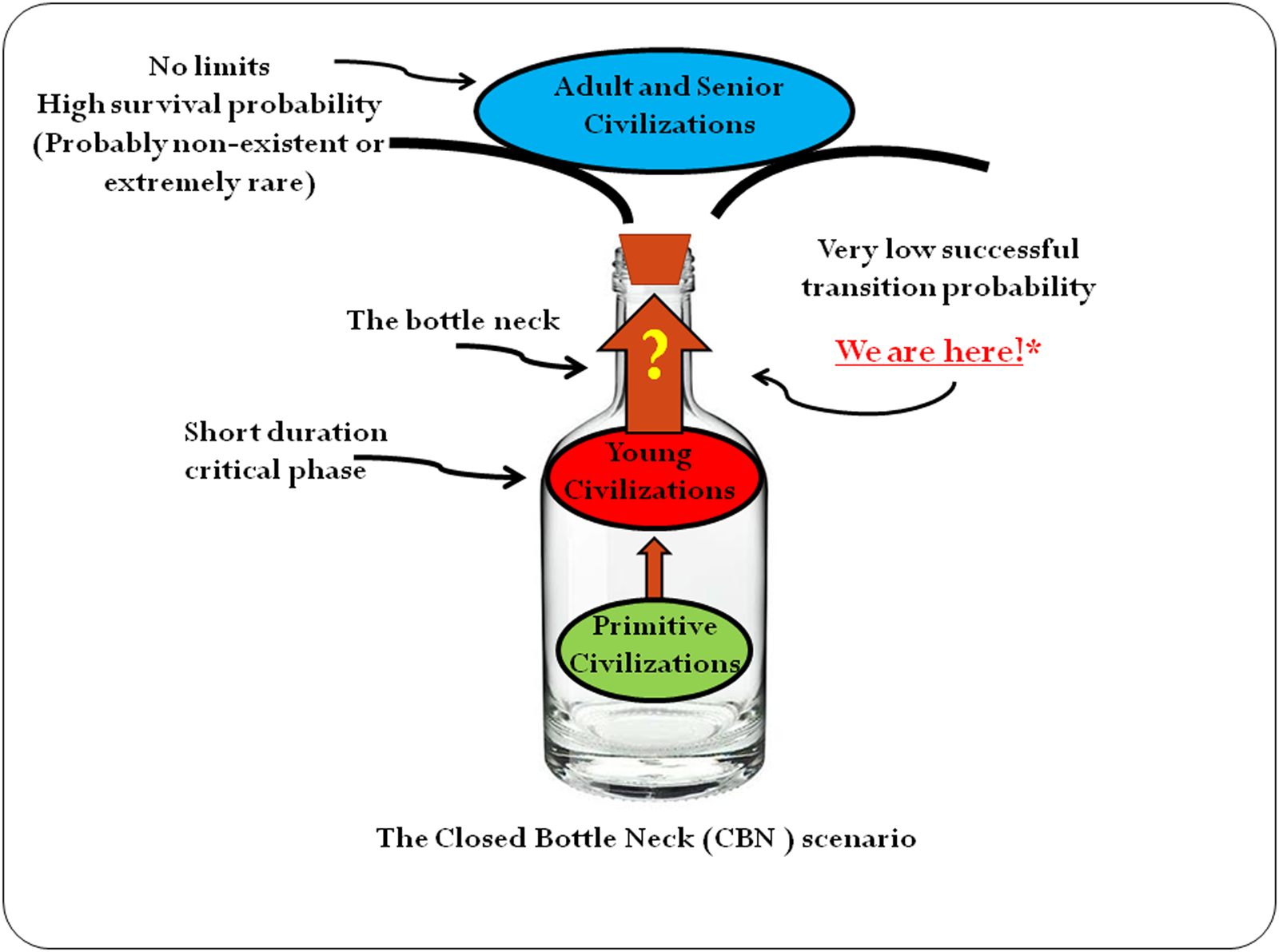
Figure 5. A (metaphorical) illustration of the Closed Bottle Neck (CBN) scenario for self-conscious intelligent technological societies evolution in the universe.

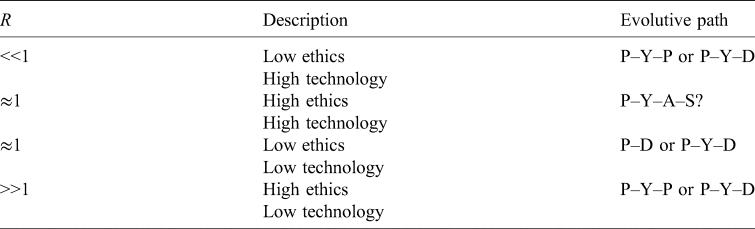
If a civilization may be generically classified as highly technologically evolved but primitive in ethical terms, then R << 1 and the chances of being well succeeded surpassing the critical young phase are not good. In this case, a civilization will probably extinguish (class D) or, at a first moment, retrocede to a more primitive stage. If, on the contrary, a civilization shows a great ethical advancement but little technological sophistication (R >> 1), success chances are also small. The ideal case would be R ≈ 1, with high-level both ethical and technological, but due to a lot of reasons (including genetic and cultural factors influencing the behaviour) that situation could tend to be rare. Table 3 summarizes the possibilities.

Table 3. Evolutionary scenarios

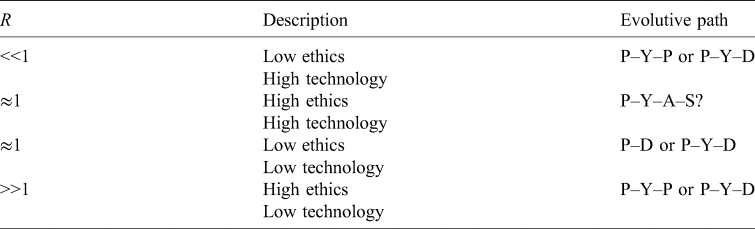
Speculative evolutionary scenarios for SCITSs as a function of R, the ethical/technological rate parameter as defined in the text.

When trying to quantify R, one could assign value 1 to designate a high ethical or high technological evolution, and a near zero, say 0.1, for low ethical or technological development. So, our civilization would be something like R ≈ 0.1. This would indicate a very low survival probability.

It is also important to add that the heterogeneity degree of a society with respect to the ethical advancement may be pivotal in determining its future. A more ethically homogeneous society would have more survival chances than an ethically very heterogeneous civilization. Technological heterogeneity does not seem to be so influential.

The closed bottle neck and Fermi's paradox

The ‘Great Silence’ (Brin, Reference Brin1983) and Fermi's Paradox may be well understood and reasonably explained with the astrosociological considerations contained in this paper. We have seen that civilizations formation rate in a typical spiral galaxy as the Milky Way is probably extremely low, of order 1 civ Gyr⁻¹. Also, if civilizations's typical lifetime has an upper limit of about 10⁸ years, then two civilizations never coexist in the Galaxy. If civilizations are no exit evolutionary paths, as has been suggested by dos Santos (2019, private communication), then virtually none of the scarce civilizations would surpass the young critical phase.

This is equivalent to say that the bottle neck is closed. As a consequence, there are no adult civilizations and therefore no interstellar flights, no von Neumann's self-reproducing machines, nor Galaxy colonization.

They are not here because they never existed, but because they are extremely rare and never evolve into A stage. In a typical spiral galaxy, the rare formed SCITSs probably enter into a P–Y–P closed loop, perhaps more than once before completely extinguishing.

This does not mean necessarily that adult or even senior civilizations never form in the universe. Their formation probability may be almost zero, but universe is so large that it is almost certain they would exist somewhere. But their rarity surely would imply that we should survey a very large number of galaxies in order to find them. Then, negative results like those by Gray and Mooley (Reference Gray and Mooley2017) would not be surprising. They could even be beyond our cosmological horizon, an idea already proposed by Wesson (Reference Wesson1990), thus providing an extended scale, cosmological resolution of the Fermi's Paradox. Obviously, in this case, direct and indirect contact chances would be null, not to speak about the cultural barrier, which would become negligible the possibilities of a positive intelligible contact between a young and an adult or senior distant extragalactic society.

The Drake's equation revisited

At this point, we return to Drake's equation (equation (2)). As already stated, factors f _i, f _c and L remain largely uncertain and controversial still today. Using the recent values for the other factors suggested in that section, we can write Drake's equation as follows:

(11)$$N_{\rm D} = 10 \times f_{\rm i} \times f_{\rm c} \times L.$$

Let us consider L = 10³ yr. This presumably would be a reasonable value to the short life expectancy of a Y society. Then we have:

(12)$$N_{\rm D} = 10^4 \times f_{\rm i} \times f_{\rm c}.$$

A very important point to remember is that even a high-level intelligence does not imply a technological civilization! Based on this, we can write factor f _i as:

(13)$$f_{\rm i} = k \times P_{\rm c}, \;$$

where k is a proportionality factor (k > 1) and P _c is our SCITS's probability formation factor introduced earlier.

We have pointed out that 10⁻¹¹ ≤ P _c ≤ 10⁻⁹. Here, we optimistically consider P _c = 10⁻⁹. Substituting into (13) we get:

(14)$$N_{\rm D} = 10^{{-}5} \times k \times f_{\rm c}$$

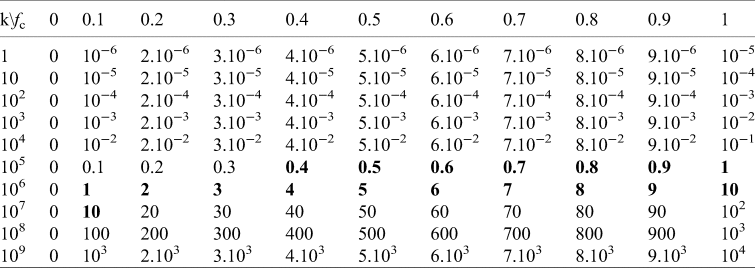
so that N _D = N _D (k,f _c). Table 4 presents some values for N _D considering 1 ≤ k ≤ 10⁹ and 0 ≤ f _c ≤ 1 selected values.

Table 4. Some reasonable Drake's equation solutions

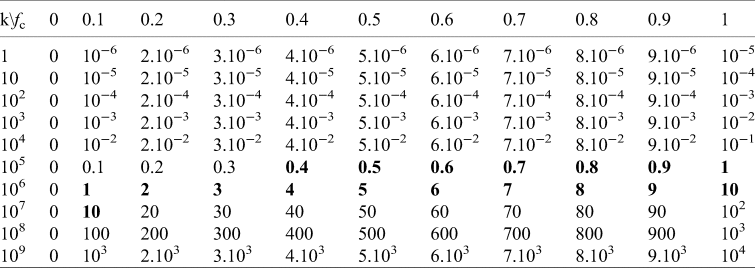
Drake's equation solutions N _D values for 1 ≤ k ≤ 10⁹ and 0 ≤ f _c ≤ 1 as discussed in the text.

From Table 4 we see that only those bold-written values for N _D are approximately compatible with Fermi's Paradox (N _D ≈ N ≈ 1–10). Those values correspond to 10⁵ ≤ k ≤ 10⁷ and 0.4 ≤ f _c ≤ 1, seeming to indicate that original values adopted for f _c (0.1–0.2) were probably underestimated. Therefore, it seems that a good fraction of technological societies is well succeeded in developing interstellar communication capability, that is, basically they dominate electromagnetic theory, a fundamental characteristic of young (type Y) societies, probably suggesting that the transition P–Y is relatively common. It also seems to suggest that it is highly probable that civilizations concomitantly develop nuclear and other advanced technologies, opening a potential way for self-annihilation.

The failure of SETI programs until now (in the sense of detecting positive extraterrestrial artificial radio or optical signals) and the great number of exoplanets found in recent years, combined with Fermi's Paradox restriction seem to imply that the great majority of technological intelligent societies do not overcome the bottle neck, thus corroborating the idea that civilizations would be no exit evolutionary paths (dos Santos, 2019, private communication).

This could also indicate that in a typical spiral galaxy like the Milky Way, we have only primitive (type P), young (type Y) and degenerated (type D) societies, not adult (type A) and/or senior (type S) civilizations. As we have pointed out earlier, P, Y or D societies do not develop interstellar flight, so they never will colonize the Galaxy.

Another interesting aspect is that the acceptable values for k are high (10⁵–10⁷). A possible interpretation is that intelligence (even if rare) may be much more common than technological civilizations, an idea also suggested by dos Santos (2019, private communication). Again, this also points to the probable extreme rarity of extraterrestrial civilizations.

We also emphasize that, the time duration L of the interstellar communicating phase for a typical civilization is not large, because of the short duration of the young evolutionary phase. The direct consequences from this to SETI traditional radio and optical searches are that the probability of intercepting a signal coming from an extraterrestrial civilization would be very small. This is so because a communicating civilization would not produce a stuffed radio signals expanding spherical bubble, but instead a thin hollow spherical shell of signals propagating through space. When we consider the short emitter's active transmission time and the enormous distances between the loci of two civilizations (typically of order 10⁴ ly or larger), it can be concluded that the contacting chances are negligible.

Now, returning to the Drake's equation, let us use as typical values k = 10⁶ and f _c = 0.5. We then get N _D = 5, a very low number, indeed, but still compatible with Fermi's Paradox, if one considers the short lifetime very probably typical for intelligent technological societies. As shown in Table 5, where we explore extreme as well as mean values for k and f _c one must have, 1 ≤ N _D ≤ 10, in order to do not violate Fermi's Paradox restriction.

Table 5. Drake's equation solutions

Drake's equation solutions N _D for different reasonable choices of k and f _c. Bold values do not violate Fermi's Paradox if SCITS's formation rate ≈1 civ Gyr⁻¹ and life expectance L′ ≤ 10⁸ yr, within the CBN scenario. For k = 10⁷ and f _c = 0.4, N _D is approximately the same as found by Westby and Conselice (Reference Westby and Conselice2020), that is, 36 (+175; −32) civilizations within our Galaxy.

As N _D is small, we can write N_D ≈ N and, adopting 10 Gyr as the astrobiological useful age of our Galaxy, we get a rough estimate to the mean civilization formation rate, very probably also valid for other typical spiral galaxies: 0.5 civ Gyr⁻¹. This value is about the same result we have found previously (1 civ Gyr⁻¹).

We can also estimate the mean spatial density of civilizations in the galactic disk. Using a mean thickness of 1 kpc and a diameter of 30 kpc results in 0.01 civ kpc⁻³. This number could be even minor by a factor of 10, if we consider that the Milky Way galactic disk can be 60 kpc in diameter, as suggested recently by López-Corredoira et al. (Reference López-Corredoira, Prieto, Garzón, Wang, Liu and Deng2018). Even considering only the GHZ, we would have just 0.05 civ kpc⁻³.

In terms of population dynamics theory, we suggest that the typical situation for a spiral galaxy would be a permanent start up or latent phase, that is, the civilizations formation rate is approximately equal to their death rate (1 civ Gyr⁻¹), the size of population (i.e. the total number of coexisting civilizations) never changes significantly, and it is always much less than the total capacity of the system. It seems there is no perspective of going into a positive linear or exponential growing phase: the number of civilizations existing in a typical spiral galaxy would be in a permanent homeostatic low regime. Finally, we remark that, if N is normally very small, of the order of unity, then Fermi's Paradox results dissolved. A similar conclusion was expressed by Sandberg et al. (Reference Sandberg, Drexler and Ord2018).

Why there should be a bottle neck?

The CBN concept derives directly from the fact that civilizations are complex dynamical and permanently unstable systems. Complex systems theory teaches us that such a system becomes increasingly fragile as its complexity grows. Strongly susceptible to a chaotic behaviour from the butterfly effect, technological civilizations may be ever building their proper ruin. Their evolution would tend to behave as a kind of cancer, whose out of control cell growing kills its host organism. This justifies the view that civilizations are no exit evolutionary pathways. In such a framework, one could hope to find only primitive (type P), or young (type Y), or degenerated (type D) societies in the universe, no adult (type A) or senior (type S) cultures, as we have already mentioned. However, we remember that this assertive is statistical in nature: eventually some Y society could succeed and become an A civilization, in spite of this event being highly improbable. If such a fact indeed occurs in a given galaxy, then that galaxy possibly could be effectively colonized.

Casti (Reference Casti2011) gives a long list of so-called ‘X-Events’, which can destroy a technological society. Pamlin and Armstrong (Reference Pamlin and Armstrong2015) do basically the same thing. Those events are mostly endogenous in nature, that is, they are deflagrated by the civilization itself. We can distinguish four basic types of endogenous X-Events: environmental collapse, nuclear self-annihilation, technological disruption and demographic explosion. Another category of X-Events, namely, those exogenous, includes natural cosmic phenomena such asteroid/comet impacts, nearby supernova explosions and GRBs, which could also affect the fate of a civilization. As the primitive stage in the life of a SCITS tends to be much longer than the young phase, and also because of the low level of technological sophistication, the major hazards to a P-type society would be offered by natural exogenous X-Events.

If the majority of civilizations in a given galaxy evolve within the GHZ limits, this fact could tend to minimize the danger from exogenous X-Events such as supernovae and GRBs, although probably not so for asteroid/comet impacts.

Considering the extremely short duration of a society's young phase, exogenous X-Events would not practically interfere, because their typical occurrence timescales are of order 10⁶ yr or more. On the other hand, endogenous X-Events would be important contributors to a Y-type society collapse. For a general view about the most probable evolutionary paths for SCITSs, see Figure 6.

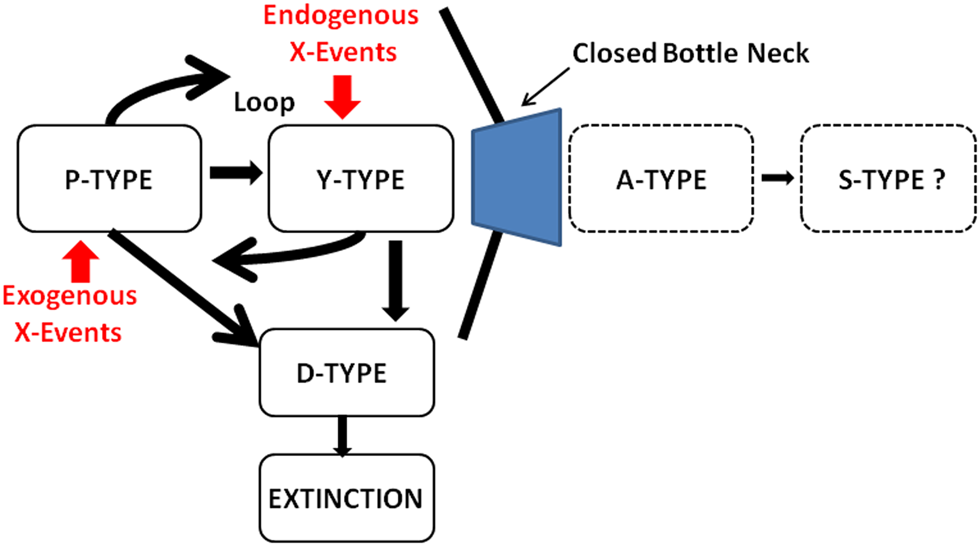
Figure 6. Most probable life cycles for self-conscious intelligent technological societies in the universe, according to the Closed Bottle Neck (CBN) model detailed in the text.

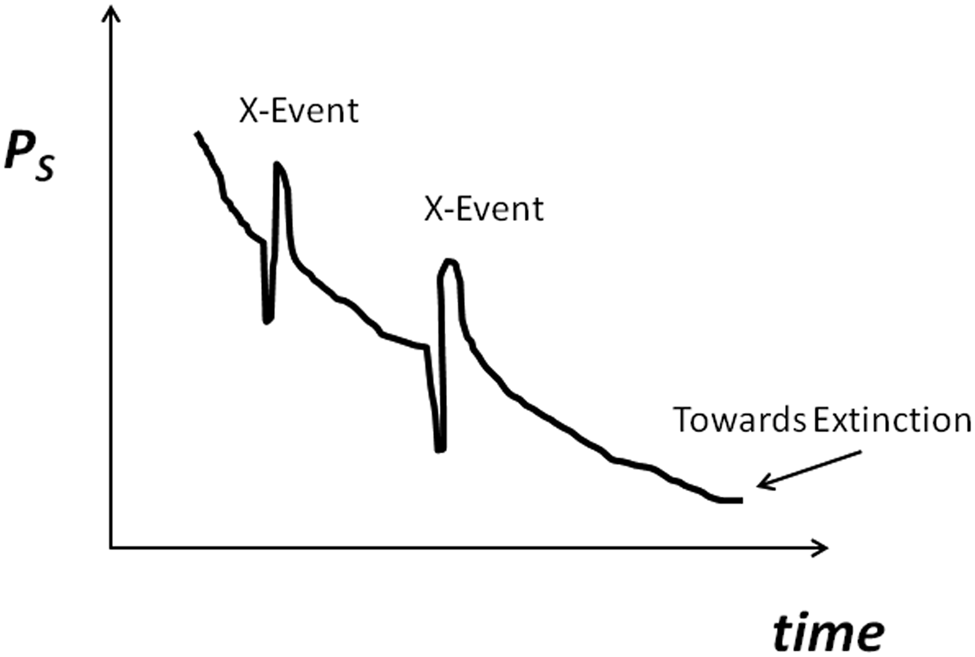
It is worth mentioning that the ‘ethical-technological ratio’ R introduced earlier can act as a modulating factor of the complexity-fragility binomial. A high ethical-low technological (R >> 1) situation would tend to reduce the fragility of the system to endogenous technological X-Events, but not against exogenous natural X-Events, while a low ethical-high technology (R << 1) case could increase the complexity of the system and so its fragility mainly to endogenous X-Events, while contributing to reduce the fragility against exogenous natural X-Events. Mathematically speaking, the survival probability P _s of a SCITS could perhaps be modelled by a decreasing exponential function of time. If a civilization enters into a P–Y–P evolutionary loop, then a qualitative representation of P _s as a function of time could be as shown in Figure 7.

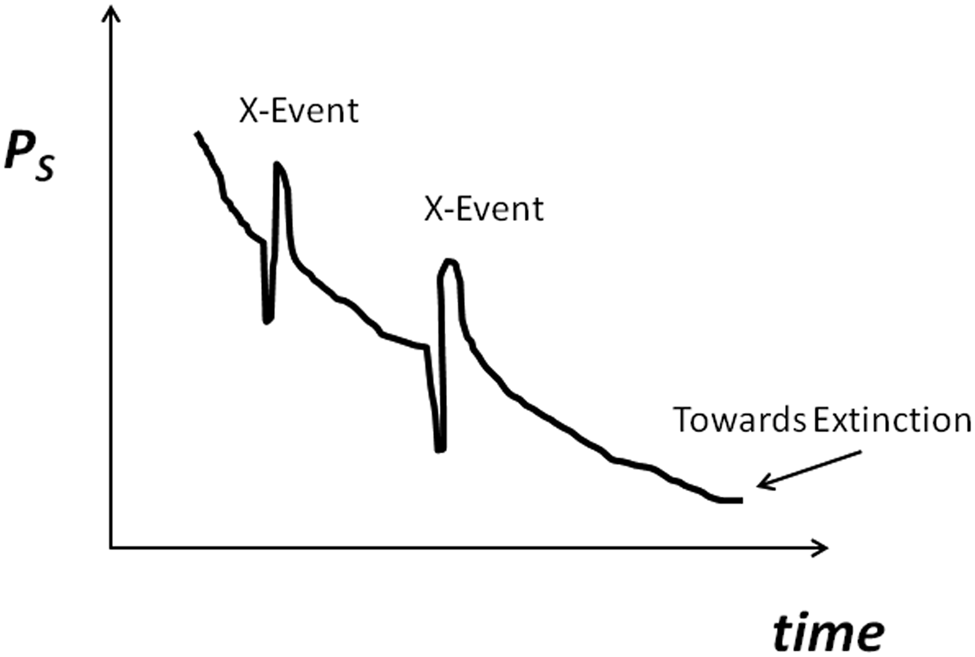
Figure 7. Qualitative graphical representation of the survival probability P _s as a function of time for a self-conscious intelligent technological society caught in a P–Y–P evolutionary loop, showing the occurrence of two hypothetical X-Events.

The fact our 10 Gyr old Galaxy is not yet colonized enables us to determine an upper limit for the survival probability P _s. Adopting a civilizations formation rate ≈1 civ Gyr⁻¹, one must have P_s ≤ 0.1. This would imply a mean colonization time t _col of the same order of the astrobiological useful age of the Galaxy, that is, about 10 Gyr. So, in this case, the time needed for the Galaxy to become colonized is comparable to the Galaxy's age. If P _s ≤ 0.01, that time would be larger by a factor of 10, about ten times the current age of the Universe! It is easy to see that t _col is proportional to the reciprocal of the survival probability P _s, and that R ≈ P _s. Clearly, P _s can not be high, and this reinforces the CBN scenario.

Perhaps the battle between complexity and fragility could have a different behaviour tendency in a highly-evolved society like A- or S-type, but the big problem would be how to survive until reaching those advanced stages.