Hostname: page-component-6dbcb7884d-vqfl6 Total loading time: 0 Render date: 2025-02-14T08:10:20.933Z Has data issue: false hasContentIssue false
  • English
  • Français

Overconvergent modular forms are highest-weight vectors in the Hodge-Tate weight zero part of completed cohomology

Part of: Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  02 March 2021

Sean Howe
Sean Howe*
Affiliation:
Department of Mathematics, University of Utah, Salt Lake City, UT, USA84112; E-mail: sean.howe@utah.edu.

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We construct a $(\mathfrak {gl}_2, B(\mathbb {Q}_p))$ and Hecke-equivariant cup product pairing between overconvergent modular forms and the local cohomology at 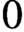$0$ of a sheaf on 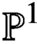$\mathbb {P}^1$, landing in the compactly supported completed $\mathbb {C}_p$-cohomology of the modular curve. The local cohomology group is a highest-weight Verma module, and the cup product is non-trivial on a highest-weight vector for any overconvergent modular form of infinitesimal weight not equal to 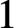$1$. For classical weight 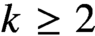$k\geq 2$, the Verma has an algebraic quotient 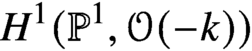$H^1(\mathbb {P}^1, \mathcal {O}(-k))$, and on classical forms, the pairing factors through this quotient, giving a geometric description of ‘half’ of the locally algebraic vectors in completed cohomology; the other half is described by a pairing with the roles of $H^1$ and $H^0$ reversed between the modular curve and $\mathbb {P}^1$. Under minor assumptions, we deduce a conjecture of Gouvea on the Hodge-Tate-Sen weights of Galois representations attached to overconvergent modular forms. Our main results are essentially a strict subset of those obtained independently by Lue Pan, but the perspective here is different, and the proofs are short and use simple tools: a Mayer-Vietoris cover, a cup product, and a boundary map in group cohomology.

MSC classification

Secondary: 11F33: Congruences for modular and $p$-adic modular forms
Type
Number Theory
Information
Forum of Mathematics, Sigma , Volume 9 , 2021 , e17
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2021. Published by Cambridge University Press

References

Bhatt, Bhargav and Scholze, Peter. Prisms and prismatic cohomology. arXiv:1905.08229, 2019.Google Scholar
Birkbeck, Christopher, Heuer, Ben, and Williams, Chris. Overconvergent Hilbert modular forms via perfectoid modular varieties. arXiv:1902.03985, 2019.Google Scholar
Boxer, George and Pilloni, Vincent. Higher Hida and Coleman theories on the modular curve. arXiv:2002.06845, 2020.Google Scholar
Chojecki, Przemyslaw, Hansen, D., and Johansson, C.. Overconvergent modular forms and perfectoid Shimura curves. Doc. Math., 22:191–262, 2017.Google Scholar
Emerton, Matthew. A local-global compatibility conjecture in the $p$-adic Langlands programme for ${\mathrm{GL}}_{2/\mathbb{Q}}$. Pure Appl. Math. Q., 2(2, Special Issue: In honor of John H. Coates. Part 2):279–393, 2006.CrossRefGoogle Scholar
Emerton, Matthew. On the interpolation of systems of eigenvalues attached to automorphic Hecke eigenforms. Invent. Math., 164(1):1–84, 2006.CrossRefGoogle Scholar
Emerton, Matthew. Local-global compatibility in the $p$-adic Langlands programme for ${\mathrm{GL}}_{2/\mathbb{Q}}$. Preprint, available: http://www.math.uchicago.edu/~emerton/pdffiles/lg.pdf, 2011.Google Scholar
Faltings, Gerd. Hodge-Tate structures and modular forms. Math. Ann., 278(1-4):133–149, 1987.CrossRefGoogle Scholar
Gouvêa, Fernando Q.. Continuity properties of $p$-adic modular forms. In Elliptic curves and related topics, volume 4 of CRM Proc. Lecture Notes, pages 85–99. Amer. Math. Soc., Providence, RI, 1994.CrossRefGoogle Scholar
Howe, Sean. Overconvergent modular forms and the $p$-adic Jacquet-Langlands correspondence. PhD thesis, University of Chicago, 2017.Google Scholar
Howe, Sean. A unipotent circle action on $p$-adic modular forms. Trans. Amer. Math. Soc. Ser. B, 7:186–226, 2020.CrossRefGoogle Scholar
Kedlaya, Kiran S. and Liu, Ruochuan. Relative p-adic Hodge theory, ii: Imperfect period rings. arXiv:1602.06899, 2016.Google Scholar
Yasuo, Morita. Analytic representations of ${\mathrm{SL}}_2$ over a $p$-adic number field. II. Automorphic forms of several variables (Katata, 1983), 282–297, Progr. Math., 46, Birkhäuser Boston, Boston, MA, 1984.Google Scholar
Pan, Lue. On locally analytic vectors of the completed cohomology of modular curves. arXiv:2008.07099, 2020.Google Scholar
Pilloni, Vincent. Overconvergent modular forms. Ann. Inst. Fourier (Grenoble), 63(1):219–239, 2013.CrossRefGoogle Scholar
Schneider, Peter and Teitelbaum, Jeremy. Locally analytic distributions and $p$-adic representation theory, with applications to ${\mathrm{GL}}_2$. J. Amer. Math. Soc., 15(2):443–468, 2002.CrossRefGoogle Scholar
Scholze, Peter. $p$-adic Hodge theory for rigid-analytic varieties. Forum Math. Pi, 1:e1, 77, 2013.CrossRefGoogle Scholar
Scholze, Peter. On torsion in the cohomology of locally symmetric varieties. Ann. of Math. (2), 182(3):945–1066, 2015.CrossRefGoogle Scholar
The Stacks Project Authors. Stacks Project. https://stacks.math.columbia.edu, 2018.Google Scholar