Hostname: page-component-6bf8c574d5-qdpjg Total loading time: 0 Render date: 2025-02-14T09:18:54.686Z Has data issue: false hasContentIssue false
  • English
  • Français

Flexibility of measure-theoretic entropy of boundary maps associated to Fuchsian groups

Part of: Measure-theoretic ergodic theory Other groups of matrices Dynamical systems with hyperbolic behavior Low-dimensional dynamical systems

Published online by Cambridge University Press:  14 April 2021

ADAM ABRAMS ,
SVETLANA KATOK  and
ILIE UGARCOVICI
ADAM ABRAMS*
Affiliation:
Institute of Mathematics, Polish Academy of Sciences, Warsaw00656, Poland
SVETLANA KATOK
Affiliation:
Department of Mathematics, The Pennsylvania State University, University Park, PA16802, USA (e-mail: sxk37@psu.edu)
ILIE UGARCOVICI
Affiliation:
Department of Mathematical Sciences, DePaul University, Chicago, IL60614, USA (e-mail: iugarcov@depaul.edu)
*
e-mail: the.adam.abrams@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a closed, orientable, compact surface S of constant negative curvature and genus 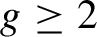$g \geq 2$ , we study the measure-theoretic entropy of the Bowen–Series boundary map with respect to its smooth invariant measure. We obtain an explicit formula for the entropy that only depends on the perimeter of the 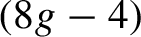$(8g-4)$ -sided fundamental polygon of the surface S and its genus. Using this, we analyze how the entropy changes in the Teichmüller space of S and prove the following flexibility result: the measure-theoretic entropy takes all values between 0 and a maximum that is achieved on the surface that admits a regular $(8g-4)$ -sided fundamental polygon. We also compare the measure-theoretic entropy to the topological entropy of these maps and show that the smooth invariant measure is not a measure of maximal entropy.

Keywords

Fuchsian groupsboundary mapsentropyTeichmüller space

MSC classification

Primary: 37D40: Dynamical systems of geometric origin and hyperbolicity (geodesic and horocycle flows, etc.) 37E10: Maps of the circle
Secondary: 28D20: Entropy and other invariants 20H10: Fuchsian groups and their generalizations
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 42 , Issue 2: Anatole Katok Memorial Issue Part 1: Special Issue of Ergodic Theory and Dynamical Systems , February 2022 , pp. 389 - 401
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

In memory of Tolya

References

Abrams, A. and Katok, S.. Adler and Flatto revisited: cross-sections for geodesic flow on compact surfaces of constant negative curvature. Studia Math. 246 (2019), 167–202.10.4064/sm171020-1-3CrossRefGoogle Scholar
Abrams, A., Katok, S. and Ugarcovici, I.. Rigidity of topological entropy of boundary maps associated to Fuchsian groups, Preprint, 2021, arxiv.org/abs/2101.10271.Google Scholar
Adler, R. and Flatto, L.. Geodesic flows, interval maps, and symbolic dynamics. Bull. Amer. Math. Soc. 25(2) (1991), 229–334.10.1090/S0273-0979-1991-16076-3CrossRefGoogle Scholar
Birman, J. and Series, C.. Dehn’s algorithm revisited, with applications to simple curves on surfaces. Combinatorial Group Theory and Topology (AM-111). Princeton University Press, Princeton, NJ, 1987, pp. 451–478.10.1515/9781400882083-023CrossRefGoogle Scholar
Bonahon, F.. The geometry of Teichmüller space via geodesic currents. Invent. Math. 92 (1988), 139–162.10.1007/BF01393996CrossRefGoogle Scholar
Bowen, R. and Series, C.. Markov maps associated with Fuchsian groups. Inst. Hautes Études Sci. Publ. Math. 50 (1979), 153–170.10.1007/BF02684772CrossRefGoogle Scholar
Fenchel, W. and Nielsen, J.. Discontinuous Groups of Isometries in the Hyperbolic Plane (de Gruyter Studies in Mathematics, 29). Ed. Schmidt, A. L.. Walter de Gruyter & Co., Berlin, 2003.Google Scholar
Forni, G. and Matheus, C.. Introduction to Teichmüller theory and its applications to dynamics of interval exchange transformations, flows on surfaces and billiards. J. Mod. Dyn. 8 (2013), 271–436.10.3934/jmd.2014.8.271CrossRefGoogle Scholar
Funar, L.. Lecture Notes for the Summer School ‘Géométries à courbure négative ou nulle, groupes discrets et rigidités’. Institut Fourier, Université de Grenoble, June–July 2004.Google Scholar
Hopf, E.. Fuchsian groups and ergodic theory. Trans. Amer. Math. Soc. 39(2) (1936), 299–314.10.1090/S0002-9947-1936-1501848-8CrossRefGoogle Scholar
Katok, A. and Hasselblatt, B.. Introduction to the Modern Theory of Dynamical Systems. Cambridge University Press, Cambridge, 1995.10.1017/CBO9780511809187CrossRefGoogle Scholar
Katok, S. and Ugarcovici, I.. Structure of attractors for boundary maps associated to Fuchsian groups. Geom. Dedicata 191 (2017), 171–198. Errata: 198 (2019), 189–191.10.1007/s10711-017-0251-zCrossRefGoogle Scholar
Ku, H.-T., Ku, M.-C. and Zhang, X.-M.. Isoperimetric inequalities on surfaces of constant curvature. Canad. J. Math. 49 (1997), 1162–1187.10.4153/CJM-1997-057-xCrossRefGoogle Scholar
Maskit, B.. New parameters for Fuchsian groups of genus $2$ . Proc. Amer. Math. Soc. 127 (1999), 3643–3652.10.1090/S0002-9939-99-04973-4CrossRefGoogle Scholar
Misiurewicz, M. and Ziemian, K.. Horseshoes and entropy for piecewise continuous piecewise monotone maps. From Phase Transitions to Chaos. World Scientific Publishing, River Edge, NJ, 1992, pp. 489–500.10.1142/9789814355872_0036CrossRefGoogle Scholar
Schmutz Schaller, P.. Teichmüller space and fundamental domains of Fuchsian groups. Enseign. Math. 45 (1999), 169–187.Google Scholar
Sullivan, D.. The density at infinity of a discrete group of hyperbolic motions. Inst. Hautes Études Sci. Publ. Math. 50 (1979), 171–202.10.1007/BF02684773CrossRefGoogle Scholar
Thurston, W. P.. Three-Dimensional Geometry and Topology. Princeton University Press, Princeton, NJ, 1997.10.1515/9781400865321CrossRefGoogle Scholar
Weiss, B.. On the work of Roy Adler in ergodic theory and dynamical systems. Symbolic Dynamics and its Applications (New Haven, CT, 1991) (Contemporary Mathematics, 135). American Mathematical Society, Providence, RI, 1992, pp. 19–32.Google Scholar
Zieschang, H., Vogt, E. and Coldewey, H. D.. Surfaces and Planar Discontinuous Groups (Lecture Notes in Mathematics, 835). Springer, Berlin, 1980.10.1007/BFb0089692CrossRefGoogle Scholar