In this paper we establish some constraints on the eigenvalues for the action of a self map of a proper variety on its $\ell$-adic cohomology. The essential ingredients are a trace formula due to Fujiwara, and the theory of weights.

The Chabauty–Kim method allows one to find rational points on curves under certain technical conditions, generalising Chabauty’s proof of the Mordell conjecture for curves with Mordell–Weil rank less than their genus. We show how the Chabauty–Kim method, when these technical conditions are satisfied in depth 2, may be applied to bound the number of rational points on a curve of higher rank. This provides a non-abelian generalisation of Coleman’s effective Chabauty theorem.

We show that the virtual cohomological dimension of a Coxeter group is essentially the regularity of the Stanley–Reisner ring of its nerve. Using this connection between geometric group theory and commutative algebra, as well as techniques from the theory of hyperbolic Coxeter groups, we study the behavior of the Castelnuovo–Mumford regularity of square-free quadratic monomial ideals. We construct examples of such ideals which exhibit arbitrarily high regularity after linear syzygies for arbitrarily many steps. We give a doubly logarithmic bound on the regularity as a function of the number of variables if these ideals are Cohen–Macaulay.

Geoffrey Robinson conjectured in 1996 that the $p$-part of character degrees in a $p$-block of a finite group can be bounded in terms of the center of a defect group of the block. We prove this conjecture for all primes $p\neq 2$ for all finite groups. Our argument relies on a reduction by Murai to the case of quasi-simple groups which are then studied using deep results on blocks of finite reductive groups.

We generalize work by Bourgain and Kontorovich [On the local-global conjecture for integral Apollonian gaskets, Invent. Math. 196 (2014), 589–650] and Zhang [On the local-global principle for integral Apollonian 3-circle packings, J. Reine Angew. Math. 737, (2018), 71–110], proving an almost local-to-global property for the curvatures of certain circle packings, to a large class of Kleinian groups. Specifically, we associate in a natural way an infinite family of integral packings of circles to any Kleinian group 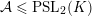${\mathcal{A}}\leqslant \text{PSL}_{2}(K)$ satisfying certain conditions, where 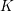$K$ is an imaginary quadratic field, and show that the curvatures of the circles in any such packing satisfy an almost local-to-global principle. A key ingredient in the proof is that 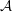${\mathcal{A}}$ possesses a spectral gap property, which we prove for any infinite-covolume, geometrically finite, Zariski dense Kleinian group in 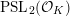$\operatorname{PSL}_{2}({\mathcal{O}}_{K})$ containing a Zariski dense subgroup of 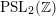$\operatorname{PSL}_{2}(\mathbb{Z})$.

Given a free unitary quantum group $G=A_{u}(F)$, with $F$ not a unitary $2\times 2$ matrix, we show that the Martin boundary of the dual of $G$ with respect to any $G$-${\hat{G}}$-invariant, irreducible, finite-range quantum random walk coincides with the topological boundary defined by Vaes and Vander Vennet. This can be thought of as a quantum analogue of the fact that the Martin boundary of a free group coincides with the space of ends of its Cayley tree.

In this paper, we prove that the set of all $F$-pure thresholds on a fixed germ of a strongly $F$-regular pair satisfies the ascending chain condition. As a corollary, we verify the ascending chain condition for the set of all $F$-pure thresholds on smooth varieties or, more generally, on varieties with tame quotient singularities, which is an affirmative answer to a conjecture given by Blickle, Mustaţǎ and Smith.

We prove that the complete $L$-function associated to any cuspidal automorphic representation of $\operatorname{GL}_{2}(\mathbb{A}_{\mathbb{Q}})$ has infinitely many simple zeros.