Let X be a smooth projective curve. We consider the dual reductive pair , 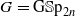over X, where H splits on an étale two-sheeted covering . Let BunG (respectively, BunH) be the stack of G-torsors (respectively, H-torsors) on X. We study the functors FG and FH between the derived categories D(BunG) and D(BunH), which are analogs of the classical theta-lifting operators in the framework of the geometric Langlands program. Assume n=m=1 and H nonsplit, that is, with connected. We establish the geometric Langlands functoriality for this pair. Namely, we show that FG :D(BunH)→D(BunG) commutes with Hecke operators with respect to the corresponding map of Langlands L-groups LH→LG. As an application, we calculate Waldspurger periods of cuspidal automorphic sheaves on BunGL2 and Bessel periods of theta-lifts from 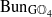to 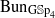. Based on these calculations, we give three conjectural constructions of certain automorphic sheaves on 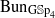(one of them makes sense for -modules only).