Hostname: page-component-745bb68f8f-5r2nc Total loading time: 0 Render date: 2025-02-11T17:16:34.920Z Has data issue: false hasContentIssue false
  • English
  • Français

On s-semipermutable or s-quasinormally Embedded Subgroups of Finite Groups

Published online by Cambridge University Press:  20 November 2018

Qingjun Kong  and
Xiuyun Guo
Qingjun Kong
Affiliation:
Department of Mathematics, Tianjin Polytechnic University, Tianjin 300387, People’s Republic of China e-mail: shkqj2929@163.com
Xiuyun Guo
Affiliation:
Department of Mathematics, Shanghai University, Shanghai 200444, People’s Republic of China
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Suppose that $G$ is a finite group and 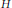$H$ is a subgroup of $G$. 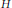$H$ is said to be $s$-semipermutable in $G$ if 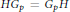$H{{G}_{p}}\,=\,{{G}_{p}}H$ for any Sylow $p$-subgroup 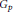${{G}_{p}}$ of $G$ with 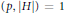$\left( p,\,\left| H \right| \right)\,=\,1$; $H$ is said to be $s$-quasinormally embedded in $G$ if for each prime $p$ dividing the order of $H$, a Sylow $p$-subgroup of $H$ is also a Sylow $p$-subgroup of some $s$-quasinormal subgroup of $G$. In every non-cyclic Sylow subgroup $P$ of $G$ we fix some subgroup $D$ satisfying 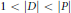$1\,<\,\left| D \right|\,<\,\left| P \right|$ and study the structure of $G$ under the assumption that every subgroup 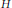$H$ of $P$ with $\left| H \right|\,=\,\left| D \right|$ is either $s$-semipermutable or $s$-quasinormally embedded in $G$. Some recent results are generalized and unified.

Keywords

20D1020D20s-semipermutable subgroups-quasinormally embedded subgroupsaturated formation
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 58 , Issue 4 , 01 December 2015 , pp. 799 - 807
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Ballester-Bolinches, A. and Pedraza-Aquilera, M. C., Sufficient conditions for supersolvability of finite groups. J. Pure Appl. Algebra 127(1998), 113–118. http://dx.doi.Org/10.1016/S0022-4049(96)00172-7 Google Scholar
[2] Deskins, W. E., On quasinormal subgroups of finite groups. Math. Z. 82(1963), 125–132. http://dx.doi.Org/10.1007/BF01111801 Google Scholar
[3] Huppert, B., Endliche Gruppen I. Springer-Verlag, Berlin-Heidelberg-New York, 1967.Google Scholar
[4] Huppert, B. and Blackburn, N., Finite Groups III. Springer-Verlag, Berlin-New York, 1982.Google Scholar
[5] Han, Z., On s-semipermutable subgroups of finite groups and p-nilpotency. Proc. Indian Acad. Sci. (Math. Sci.) 120(2010), 141–148. http://dx.doi.Org/10.1007/s12044-010-0026-z Google Scholar
[6] Kegel, O. H., Sylow Gruppen und subnormalteiler endlicher Gruppen. Math. Z. 78( 1962), 205–221. http://dx.doi.Org/10.1007/BF01195169 Google Scholar
[7] Li, S., Shen, Z., and Liu, J. et at, The influence of SS-quasinormality of some subgroups on the structure of finite groups. J. Algebra 319(2008), 4275^287. http://dx.doi.Org/10.1016/j.jalgebra.2008.01.030 Google Scholar
[8] Li, Y., Wei, H., and Wang, Y The influence ofir-quasinormality of some subgroups of a finite group. Arch. Math. 81(2003), 245–252. http://dx.doi.Org/10.1007/s00013-003-0829-6 Google Scholar
[9] Li, Y and Wang, Y, The influence of minimal subgroups on the structure of a finite group. Proc. Amer. Math. Soc. 131(2002), 337–341. http://dx.doi.Org/10.1090/S0002-9939-02-06547-4 Google Scholar
[10] Skiba, A. N., On weakly s-permutable subgroups of finite groups. J. Algebra 315(2007), 192–209. http://dx.doi.Org/10.1016/j.jalgebra.2007.04.025 Google Scholar
[11] Schmid, P., Subgroups permutable with all Sylow subgroups. J. Algebra 207(1998), 285–293. http://dx.doi.Org/10.1006/jabr.1998.7429 Google Scholar
[12] Wang, L. and Wang, Y, On s-semipermutable maximal and minimal subgroups of Sylow p-groups of finite groups. Comm. Algebra 34(2006), 143–149. http://dx.doi.Org/10.1080/0092 7870500346081 Google Scholar
[13] Wei, H. and Wang, Y, On c* -normality and its properties. J. Group Theory 10(2007), 211–223.Google Scholar
[14] Wei, X. and Guo, X., On finite groups with prime-power order S-quasinormally embedded subgroups. Monatsh. Math. 162(2011), 329–339. http://dx.doi.Org/10.1007/s00605-009-0175-2 Google Scholar
[15] Zhang, Q. and Wang, L., The influence of s-semipermutable subgroups on the structure of a finite group. Acta Math. Sinica 48(2005), 81–88.Google Scholar