Published online by Cambridge University Press: 20 November 2018
Let 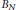 ${{B}_{N}}$ be the unit ball in
${{B}_{N}}$ be the unit ball in 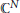 ${{\mathbb{C}}^{N}}$ and let
${{\mathbb{C}}^{N}}$ and let  $f$ be a function holomorphic and
$f$ be a function holomorphic and  ${{L}^{2}}$-integrable in
${{L}^{2}}$-integrable in 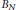 ${{B}_{N}}$. Denote by
${{B}_{N}}$. Denote by 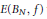 $E\left( {{B}_{N}},\,f \right)$ the set of all slices of the form
$E\left( {{B}_{N}},\,f \right)$ the set of all slices of the form 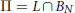 $\Pi \,=\,L\,\cap \,{{B}_{N}}$, where
$\Pi \,=\,L\,\cap \,{{B}_{N}}$, where 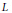 $L$ is a complex one-dimensional subspace of
$L$ is a complex one-dimensional subspace of 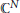 ${{\mathbb{C}}^{N}}$, for which
${{\mathbb{C}}^{N}}$, for which  $f{{|}_{\Pi }}$ is not
$f{{|}_{\Pi }}$ is not  ${{L}^{2}}$-integrable (with respect to the Lebesgue measure on L). Call this set the exceptional set for
${{L}^{2}}$-integrable (with respect to the Lebesgue measure on L). Call this set the exceptional set for  $f$. We give a characterization of exceptional sets which are closed in the natural topology of slices.
$f$. We give a characterization of exceptional sets which are closed in the natural topology of slices.