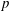Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-02-11T14:53:41.184Z Has data issue: false hasContentIssue false
  • English
  • Français

Character Codegrees of Maximal Class $p$-groups

Part of: Representation theory of groups

Published online by Cambridge University Press:  25 September 2019

Sarah Croome  and
Mark L. Lewis
Sarah Croome
Affiliation:
Department of Mathematical Sciences, Kent State University, Kent, OH 44242, United States Email: scroome@kent.edulewis@math.kent.edu
Mark L. Lewis
Affiliation:
Department of Mathematical Sciences, Kent State University, Kent, OH 44242, United States Email: scroome@kent.edulewis@math.kent.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 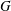$G$ be a 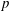$p$-group and let 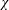$\unicode[STIX]{x1D712}$ be an irreducible character of 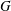$G$. The codegree of 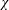$\unicode[STIX]{x1D712}$ is given by $|G:\,\text{ker}(\unicode[STIX]{x1D712})|/\unicode[STIX]{x1D712}(1)$. If 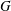$G$ is a maximal class 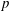$p$-group that is normally monomial or has at most three character degrees, then the codegrees of $G$ are consecutive powers of $p$. 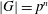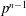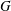If $|G|=p^{n}$ and $G$ has consecutive $p$-power codegrees up to $p^{n-1}$, then the nilpotence class of $G$ is at most 2 or $G$ has maximal class.

Keywords

codegreep-groupmaximal class

MSC classification

Primary: 20C15: Ordinary representations and characters
Secondary: 20D15: Nilpotent groups, $p$-groups
Type
Article
Information
Canadian Mathematical Bulletin , Volume 63 , Issue 2 , June 2020 , pp. 328 - 334
Copyright
© Canadian Mathematical Society 2019

References

Berkovich, Y., Groups of prime power order. Vol. 1. De Gruyter Expositions in Mathematics, 46, De Gruyter, Berlin, 2008. https://doi.org/10.1515/9783110208238.512Google Scholar
Bosma, W., Cannon, J., and Playoust, C., The magma algebra system I: The user language. J. Symbolic Comput. 24(1997), 235–265. https://doi.org/10.1006/jsco.1996.0125Google Scholar
Du, N. and Lewis, M. L., Codegrees and nilpotence class of p-groups. J. Group Theory 19(2016), 561–567. https://doi.org/10.1515/jgth-2015-0039Google Scholar
Isaacs, I. M., Character theory of finite groups. AMS Chelsea Publishing, Providence, RI, 2006. https://doi.org/10.1090/chel/359Google Scholar
Mann, A., Character degrees of some p-groups. 2016. arxiv:1602.04689Google Scholar
Qian, G., Wang, Y., and Wei, H., Co-degrees of irreducible characters in finite groups. J. Algebra 312(2007), 946–955. https://doi.org/10.1016/j.jalgebra.2006.11.001Google Scholar
Slattery, M. C., Maximal class p-groups with large character degree gaps. Arch. Math. (Basel) 105(2015), 501–507. https://doi.org/10.1007/s00013-015-0836-4Google Scholar
Slattery, M. C., Character degrees of normally monomial maximal class 5-groups. Character theory of finite groups. Contemp. Math., 524, American Mathematical Society, Providence, RI, 2010, pp. 153–159. https://doi.org/10.1090/conm/524/10354Google Scholar