No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
A fundamental idea in toric topology is that classes of manifolds with well-behaved torus actions (simply, toric spaces) are classified by pairs of simplicial complexes and (non-singular) characteristic maps. In a previous paper, the authors provided a new way to find all characteristic maps on a simplicial complex  $K(J)$ obtainable by a sequence of wedgings from
$K(J)$ obtainable by a sequence of wedgings from 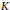 $K$.The main idea was that characteristic maps on
$K$.The main idea was that characteristic maps on 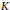 $K$ theoretically determine all possible characteristic maps on a wedge of
$K$ theoretically determine all possible characteristic maps on a wedge of 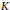 $K$.
$K$.
We further develop our previous work for classification of toric spaces. For a star-shaped simplicial sphere 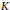 $K$ of dimension
$K$ of dimension 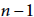 $n-1$ with
$n-1$ with  $m$ vertices, the Picard number Pic
$m$ vertices, the Picard number Pic $(K)$ of
$(K)$ of 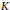 $K$ is
$K$ is 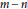 $m-n$. We call
$m-n$. We call 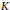 $K$ a seed if
$K$ a seed if 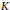 $K$ cannot be obtained by wedgings. First, we show that for a fixed positive integer
$K$ cannot be obtained by wedgings. First, we show that for a fixed positive integer 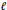 $\ell $, there are at most finitely many seeds of Picard number
$\ell $, there are at most finitely many seeds of Picard number 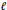 $\ell $ supporting characteristic maps. As a corollary, the conjecture proposed by V. V. Batyrev in is solved affirmatively.
$\ell $ supporting characteristic maps. As a corollary, the conjecture proposed by V. V. Batyrev in is solved affirmatively.
Secondly, we investigate a systematicmethod to find all characteristic maps on  $K(J)$ using combinatorial objects called (realizable) puzzles that only depend on a seed
$K(J)$ using combinatorial objects called (realizable) puzzles that only depend on a seed 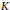 $K$. These two facts lead to a practical way to classify the toric spaces of fixed Picard number.
$K$. These two facts lead to a practical way to classify the toric spaces of fixed Picard number.