Hostname: page-component-745bb68f8f-s22k5 Total loading time: 0 Render date: 2025-02-10T07:43:32.474Z Has data issue: false hasContentIssue false
  • English
  • Français

Twists of Shimura Curves

Published online by Cambridge University Press:  20 November 2018

James Stankewicz
James Stankewicz*
Affiliation:
Mathematics and Computer Science, Wesleyan University, Middletown, CT, USA. e-mail: stankewicz@gmail.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Consider a Shimura curve 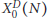$X_{0}^{D}\left( N \right)$ over the rational numbers. We determine criteria for the twist by an Atkin–Lenher involution to have points over a local field. As a corollary we give a new proof of the theorem of Jordan and Livné on ${{\mathbf{Q}}_{p}}$ points when 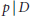$p|D$ and for the first time give criteria for ${{\mathbf{Q}}_{p}}$ points when 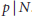$p|N$. We also give congruence conditions for roots modulo $p$ of Hilbert class polynomials.

Keywords

11G1814G3511G1511G10Shimura curvescomplex multiplicationmodular curveselliptic curves
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 66 , Issue 4 , 01 August 2014 , pp. 924 - 960
Copyright
Copyright © Canadian Mathematical Society 2014

Footnotes

The author was partially supported by NSF VIGRE grant DMS-0738586 and the University of Georgia Dissertation completion award.

References

[BLR90] Bosch, Siegfried, Lütkebohmert, Werner, and Raynaud, Michel, Néron models. Ergeb. Math. Grenzgeb. 21, Springer-Verlag, Berlin, 1990.Google Scholar
[CES03] Conrad, Brian, Edixhoven, Bas, and Stein, William, J1(p) has connected fibers. Doc. Math. 8(2003), 331–408 (electronic).Google Scholar
[Cla03] Clark, Pete L., Rational Points on Atkin–Lehner Quotients of Shimura Curves. Ph.D. thesis, Harvard, 2003.Google Scholar
[Cox89] Cox, David A., Primes of the form x2 + ny2. AWiley-Interscience Publication, John Wiley & Sons, Inc., New York, 1989.Google Scholar
[Del69] Deligne, Pierre, Variétés abéliennes ordinaires sur un corps fini. Invent. Math. 8(1969), 238–243. http://dx.doi.org/10.1007/BF01406076 CrossRefGoogle Scholar
[Eic56] Eichler, Martin, Modular correspondences and their representations. J. Indian Math. Soc. (N.S.) 20(1956), 163–206.Google Scholar
[GR91] Gonzàlez Rovira, Josep, Equations of hyperelliptic modular curves. Ann. Inst. Fourier (Grenoble) 41(1991), 779–795. http://dx.doi.org/10.5802/aif.1273 CrossRefGoogle Scholar
[GR04] González, Josep and Victor Rotger, , Equations of Shimura curves of genus two. Int. Math. Res. Not. 14(2004), 661–674.CrossRefGoogle Scholar
[Hel07] Helm, David, On maps between modular Jacobians and Jacobians of Shimura curves. Israel J. Math. 160(2007), 61–117. http://dx.doi.org/10.1007/s11856-007-0056-0 CrossRefGoogle Scholar
[JL85] Jordan, Bruce W. and LivnÉ, Ron A., Local Diophantine properties of Shimura curves. Math. Ann. 270(1985), 235–248. http://dx.doi.org/10.1007/BF01456184 CrossRefGoogle Scholar
[Kan11] Kani, Ernst, Products of CM elliptic curves. Collect. Math. 62(2011), 297–339. http://dx.doi.org/10.1007/s13348-010-0029-1 CrossRefGoogle Scholar
[KR08] Kontogeorgis, Aristides and Rotger, Victor, On the non-existence of exceptional automorphisms on Shimura curves. Bull. London Math. Soc. 40(2008), 363–374. http://dx.doi.org/10.1112/blms/bdn012 CrossRefGoogle Scholar
[Kur79] Kurihara, Akira, On some examples of equations defining Shimura curves and the Mumford uniformization. J. Fac. Sci. Univ. Tokyo Sect. IA Math. 25(1979), 277–300.Google Scholar
[Lan87] Lang, Serge, Elliptic functions. Second edition, Graduate Texts in Math. 112, Springer-Verlag, New York, 1987.Google Scholar
[Liu02] Liu, Qing, Algebraic geometry and arithmetic curves. Oxford Graduate Texts in Math. 6, Oxford University Press, Oxford, 2002.Google Scholar
[Lor11] Lorenzini, Dino J., Wild Models of Curves. http://www.math.uga.edu/_lorenz/Paper2.pdf Google Scholar
[Mes72] Messing, William, The crystals associated to Barsotti–Tate groups: with applications to abelian schemes. Lecture Notes in Math. 264, Springer-Verlag, Berlin, 1972.Google Scholar
[Mil86] Milne, James S., Jacobian varieties. In: Arithmetic geometry (Storrs, Conn., 1984), Springer, New York, 1986, 167–212.CrossRefGoogle Scholar
[Mol12] Molina, Santiago, Ribet bimodules and the specialization of Heegner points. Israel J. Math. 189(2012), 1–38. http://dx.doi.org/10.1007/s11856-011-0172-8 CrossRefGoogle Scholar
[Neu99] Neukirch, Jürgen, Algebraic number theory. Grundlehren Math.Wiss. 322, Springer-Verlag, Berlin, 1999.Google Scholar
[Ogg74] Ogg, Andrew P., Hyperelliptic modular curves. Bull. Soc. Math. France 102(1974), 449–462.CrossRefGoogle Scholar
[Ogg83] Ogg, Andrew P., Real points on Shimura curves. In: Arithmetic and geometry, Vol. I, Progr. Math. 35(1983), 277–307.Google Scholar
[Ogg85] Ogg, Andrew P., Mauvaise réduction des courbes de Shimura. In: Séminaire de théorie des nombres (Paris 1983–84), Progr. Math. 59(1985), 199–217.Google Scholar
[Ozm12] Ozman, Ekin, Local Points on Quadratic Twists of X0(N). Acta Arith. 152(2012), 323–348. http://dx.doi.org/10.4064/aa152-4-1 CrossRefGoogle Scholar
[Piz76] Pizer, Arnold, On the arithmetic of quaternion algebras. Acta Arith. 31(1976), 61–89.CrossRefGoogle Scholar
[Rib89] Ribet, Kenneth A., Bimodules and abelian surfaces. In: Algebraic number theory, Adv. Stud. Pure Math. 17(1989), 359–407.Google Scholar
[RS11] Ribet, Ken and Stein, William, Lectures on Modular Forms and Hecke Operators. http://wstein.org/books/ribet-stein/main.pdf, 2011.Google Scholar
[RSY05] Rotger, Victor, Skorobogatov, Alexei, and Yafaev, Andrei, Failure of the Hasse principle for Atkin–Lehner quotients of Shimura curves over ℚ. Mosc. Math. J. 5(2005), 463–476, 495.Google Scholar
[S+] Stein, W. A. et al., Sage Mathematics Software (Version 4.8). The Sage Development Team, 2012. http://www.sagemath.org Google Scholar
[Sad10] Sadek, Mohammad, On Quadratic Twists of Hyperelliptic Curves. http://arxiv.org/abs/1010.0732, 2010.Google Scholar
[Shi67] Shimura, Goro, Construction of class fields and zeta functions of algebraic curves. Ann. of Math. (2) 85(1967), 58–159. http://dx.doi.org/10.2307/1970526 CrossRefGoogle Scholar
[Shi71] Shimura, Goro, Introduction to the arithmetic theory of automorphic functions. Kanô Memorial Lectures, No. 1, Publications of the Mathematical Society of Japan 11, Iwanami Shoten, Publishers, Tokyo, 1971.Google Scholar
[Shi79] Shioda, Tetsuji, Supersingular K3 surfaces. In: Algebraic geometry (Proc. Summer Meeting, Univ. Copenhagen, Copenhagen, 1978), Lecture Notes in Math. 732(1979), 564–591.Google Scholar
[Vie77] Viehweg, Eckart, Invarianten der degenerierten Fasern in lokalen Familien von Kurven. J. Reine Angew. Math. 293/294(1977), 284–308.Google Scholar
[Vig80] France, Marie Vignéras, , Arithmétique des algèbres de quaternions. Lecture Notes in Math. 800, Springer, Berlin, 1980.Google Scholar