Published online by Cambridge University Press: 20 November 2018
Write 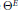 ${{\Theta }^{E}}$ for the stable discrete series character associated with an irreducible finite-dimensional representation
${{\Theta }^{E}}$ for the stable discrete series character associated with an irreducible finite-dimensional representation 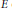 $E$ of a connected real reductive group
$E$ of a connected real reductive group  $G$. Let
$G$. Let 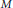 $M$ be the centralizer of the split component of a maximal torus
$M$ be the centralizer of the split component of a maximal torus  $T$, and denote by
$T$, and denote by 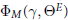 ${{\Phi }_{M}}\left( \gamma ,\,{{\Theta }^{E}} \right)$ Arthur’s extension of
${{\Phi }_{M}}\left( \gamma ,\,{{\Theta }^{E}} \right)$ Arthur’s extension of 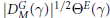 $|D_{M}^{G}\,\left( \gamma \right)|{{\,}^{1/2}}\,{{\Theta }^{E}}\,\left( \gamma \right)$ to
$|D_{M}^{G}\,\left( \gamma \right)|{{\,}^{1/2}}\,{{\Theta }^{E}}\,\left( \gamma \right)$ to  $T\left( \mathbb{R} \right)$. In this paper we give a simple explicit expression for
$T\left( \mathbb{R} \right)$. In this paper we give a simple explicit expression for 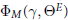 ${{\Phi }_{M}}\left( \gamma ,\,{{\Theta }^{E}} \right)$ when
${{\Phi }_{M}}\left( \gamma ,\,{{\Theta }^{E}} \right)$ when  $\gamma $ is elliptic in
$\gamma $ is elliptic in  $G$. We do not assume
$G$. We do not assume  $\gamma $ is regular.
$\gamma $ is regular.