Hostname: page-component-745bb68f8f-l4dxg Total loading time: 0 Render date: 2025-02-06T22:44:36.083Z Has data issue: false hasContentIssue false
  • English
  • Français

A REACTION–DIFFUSION–ADVECTION EQUATION WITH COMBUSTION NONLINEARITY ON THE HALF-LINE

Part of: Parabolic equations and systems Partial differential equations

Published online by Cambridge University Press:  02 July 2018

FANG LI Open the ORCID record for FANG LI [Opens in a new window] ,
QI LI Open the ORCID record for QI LI [Opens in a new window]  and
YUFEI LIU Open the ORCID record for YUFEI LIU [Opens in a new window]
FANG LI
Affiliation:
Mathematics and Science College, Shanghai Normal University, Shanghai 200234, China email lifwx@shnu.edu.cn
QI LI*
Affiliation:
Mathematics and Science College, Shanghai Normal University, Shanghai 200234, China email 2313591524@qq.com, 1000443603@smail.shnu.edu.cn
YUFEI LIU
Affiliation:
Mathematics and Science College, Shanghai Normal University, Shanghai 200234, China email 1000421212@smail.shnu.edu.cn
*
✉2313591524@qq.com, 1000443603@smail.shnu.edu.cn
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

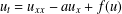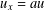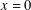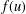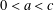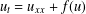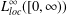We study the dynamics of a reaction–diffusion–advection equation $u_{t}=u_{xx}-au_{x}+f(u)$ on the right half-line with Robin boundary condition $u_{x}=au$ at $x=0$, where $f(u)$ is a combustion nonlinearity. We show that, when $0<a<c$ (where $c$ is the travelling wave speed of $u_{t}=u_{xx}+f(u)$), $u$ converges in the $L_{loc}^{\infty }([0,\infty ))$ topology either to 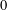$0$ or to a positive steady state; when 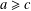$a\geq c$, a solution $u$ starting from a small initial datum tends to $0$ in the 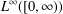$L^{\infty }([0,\infty ))$ topology, but this is not true for a solution starting from a large initial datum; when 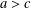$a>c$, such a solution converges to 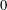$0$ in 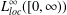$L_{loc}^{\infty }([0,\infty ))$ but not in 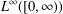$L^{\infty }([0,\infty ))$ topology.

Keywords

reaction–diffusion equationcombustion equationinitial boundary value problemasymptotic behaviour

MSC classification

Primary: 35K57: Reaction-diffusion equations
Secondary: 35K15: Initial value problems for second-order parabolic equations 35B40: Asymptotic behavior of solutions
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 98 , Issue 2 , October 2018 , pp. 277 - 285
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

This research was partly supported by NSFC (Nos. 11701374 and 11671262).

References

Angenent, S. B., ‘The zero set of a solution of a parabolic equation’, J. reine angew. Math. 390 (1988), 79–96.Google Scholar
Chen, X., Lou, B., Zhou, M. and Giletti, T., ‘Long time behavior of solutions of a reaction–diffusion equation on unbounded intervals with Robin boundary conditions’, Ann. Inst. H. Poincaré Anal. Non Linéaire 33 (2016), 67–92.Google Scholar
Cui, R., Lam, K.-Y. and Lou, Y., ‘Dynamics and asymptotic profiles of steady states of an epidemic model in advective environments’, J. Differential Equations 263 (2017), 2343–2373.Google Scholar
Cui, R. and Lou, Y., ‘A spatial SIS model in advective heterogeneous environments’, J. Differential Equations 261 (2016), 3305–3343.Google Scholar
Du, Y. and Lou, B., ‘Spreading and vanishing in nonlinear diffusion problems with free boundaries’, J. Eur. Math. Soc. 17 (2015), 2673–2724.Google Scholar
Du, Y., Lou, B. and Zhou, M., ‘Nonlinear diffusion problems with free boundaries: convergence, transition speed, and zero number argument’, SIAM J. Math. Anal. 47 (2015), 3555–3584.Google Scholar
Du, Y. and Matano, H., ‘Convergence and sharp thresholds for propagation in nonlinear diffusion problems’, J. Eur. Math. Soc. 12 (2010), 279–312.Google Scholar
Fašangová, E. and Feireisl, E., ‘The long-time behavior of solutions of parabolic problems on unbounded intervals: the influence of boundary conditions’, Proc. Roy. Soc. Edinburgh Sect. A 129 (1999), 319–329.Google Scholar
Gu, H., Lin, Z. and Lou, B., ‘Different asymptotic spreading speeds induced by advection in a diffusion problem with free boundaries’, Proc. Amer. Math. Soc. 143 (2015), 1109–1117.Google Scholar
Gu, H., Lou, B. and Zhou, M., ‘Long time behavior of solutions of Fisher–KPP equation with advection and free boundaries’, J. Funct. Anal. 269 (2015), 1714–1768.Google Scholar
Lou, Y. and Zhou, P., ‘Evolution of dispersal in advective homogeneous environment: the effect of boundary conditions’, J. Differential Equations 259 (2015), 141–171.Google Scholar
Matano, H., ‘Convergence of solutions of one-dimensional semilinear parabolic equations’, J. Math. Kyoto Univ. 18 (1978), 221–227.Google Scholar
Zlatoš, A., ‘Sharp transition between extinction and propagation of reaction’, J. Amer. Math. Soc. 19 (2006), 251–263.Google Scholar