Let $M=(\begin {smallmatrix}\rho ^{-1} & 0 \\0 & \rho ^{-1} \\\end {smallmatrix})$ be an expanding real matrix with $0<\rho <1$, and let ${\mathcal D}_n=\{(\begin {smallmatrix} 0\\ 0 \end {smallmatrix}),(\begin {smallmatrix} \sigma _n\\ 0 \end {smallmatrix}),(\begin {smallmatrix} 0\\ \gamma _n \end {smallmatrix})\}$ be digit sets with $\sigma _n,\gamma _n\in \{-1,1\}$ for each $n\ge 1$. Then the infinite convolution

$$ \begin{align*}\mu_{M,\{{\mathcal D}_n\}}=\delta_{M^{-1}{\mathcal D}_1}\ast\delta_{M^{-2}{\mathcal D}_2}\ast\cdots\end{align*} $$

is called a Moran–Sierpinski measure. We give a necessary and sufficient condition for 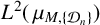$L^2(\,\mu _{M,\{{\mathcal D}_n\}})$ to admit an infinite orthogonal set of exponential functions. Furthermore, we give the exact cardinality of orthogonal exponential functions in 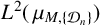$L^2(\,\mu _{M,\{{\mathcal D}_n\}})$ when $L^2(\,\mu _{M,\{{\mathcal D}_n\}})$ does not admit any infinite orthogonal set of exponential functions based on whether $\rho $ is a trinomial number or not.