Hostname: page-component-6dbcb7884d-q4sxc Total loading time: 0 Render date: 2025-02-13T18:46:57.361Z Has data issue: false hasContentIssue false
  • English
  • Français

PARITY BIAS IN FUNDAMENTAL UNITS OF REAL QUADRATIC FIELDS

Part of: Computational number theory Diophantine equations Algebraic number theory: global fields

Published online by Cambridge University Press:  03 February 2025

FLORIAN BREUER Open the ORCID record for FLORIAN BREUER [Opens in a new window]  and
CAMERON SHAW-CARMODY Open the ORCID record for CAMERON SHAW-CARMODY [Opens in a new window]
FLORIAN BREUER*
Affiliation:
School of Information and Physical Sciences, University of Newcastle, Callaghan, New South Wales 2308, Australia
CAMERON SHAW-CARMODY
Affiliation:
School of Information and Physical Sciences, University of Newcastle, Callaghan, New South Wales 2308, Australia e-mail: cameron.shaw-carmody@newcastle.edu.au
*
e-mail: florian.breuer@newcastle.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

We compute primes $p \equiv 5 \bmod 8$ up to $10^{11}$ for which the Pellian equation $x^2-py^2=-4$ has no solutions in odd integers; these are the members of sequence A130229 in the Online Encyclopedia of Integer Sequences. We find that the number of such primes $p\leqslant x$ is well approximated by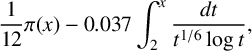

$$ \begin{align*}\frac{1}{12}\pi(x) - 0.037\int_2^x \frac{dt}{t^{1/6}\log t},\end{align*} $$

where $\pi (x)$ is the usual prime counting function. The second term shows a surprising bias away from membership of this sequence.

Keywords

Pellian equationfundamental unitprimeOEIS A130229

MSC classification

Primary: 11D09: Quadratic and bilinear equations
Secondary: 11R27: Units and factorization 11Y65: Continued fraction calculations
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , First View , pp. 1 - 4
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was supported by the Alexander von Humboldt Foundation. The second author was supported by a 2020–2021 Vacation Research Scholarship from the Australian Mathematical Sciences Institute (AMSI).

References

Breuer, F., ‘Periods of Ducci sequences and odd solutions to a Pellian equation’, Bull. Aust. Math. Soc. 100 (2019), 201–205.CrossRefGoogle Scholar
Breuer, F., ‘Multiplicative orders of Gauss periods and the arithmetic of real quadratic fields’, Finite Fields Appl. 73 (2021), Article no. 101848.CrossRefGoogle Scholar
Jacobson, M. J. and Williams, H. C., Solving the Pell Equation, CMS Books in Mathematics (Springer, New York, 2009).CrossRefGoogle Scholar
Lam, S. T., Pitrou, A. and Seibert, S., ‘Numba: a LLVM-based Python JIT compiler’, in: LLVM’15: Proceedings of the Second Workshop on the LLVM Compiler Infrastructure in HPC, ACM Digital Library (ed. Finkel, H.) (Association for Computing Machinery, New York, 2015), Article no. 7, 6 pages.Google Scholar
The On-line Encyclopedia of Integer Sequences , entry #A130229, https://oeis.org/A130229.Google Scholar
The Sage Developers, SageMath, the Sage Mathematics Software System (Version 9.3), 2021. https://www.sagemath.org.Google Scholar
Xue, J., Yang, T.-C. and Yu, C.-F., ‘Numerical invariants of totally imaginary quadratic $\mathbb{Z}\,[\sqrt{p}]$ -orders’, Taiwanese J. Math. 20(4) (2016), 723–741.CrossRefGoogle Scholar